On the Stable Eigenvalues of Perturbed Anharmonic Oscillators in Dimension Two

Abstract

We study the asymptotic behavior of the spectrum of a quantum system which is a perturbation of a spherically symmetric anharmonic oscillator in dimension 2. We prove that a large part of its eigenvalues can be obtained by Bohr–Sommerfeld quantization rule applied to the normal form Hamiltonian and also admits an asymptotic expansion at infinity. The proof is based on the generalization to the present context of the normal form approach developed in Bambusi et al. (Commun Part Differ Equ 45:1–18, 2020) (see also Parnovski and Sobolev in Invent Math 181(3):467–540, 2010) for the particular case of ${\mathbb {T}}^d$.

Appendices

Appendix A: Proof of Lemma 4.5

We start by proving the following easy lemma

Lemma A.1

The function $a_r$ defined by (4.4) is analytic on the domain

$$\begin{aligned} 0<\left| L\right| <\left( \frac{2\ell }{\ell +1}E\right) ^{\frac{\ell +1}{2\ell }},\quad E>0; \end{aligned}$$

(A.1)

Proof

The effective Hamiltonian $h_0^*(r,p_r,L)$ defined by (4.3) is an analytic function except at $r=0$. Furthermore, for $L\not =0$, $h^*$, as a function of $r,p_r$ is a submersion, except on the level surface of level $E=|L|^{\frac{\ell +1}{2\ell }}$. So, outside this domain the level surface depend analytically on both E and L. Since $a_r$ is just the normalized area contained in the level surface, it also depends analytically on E, L in the considered domain. $\square$

We now study the behavior of $a_r$ as $L\rightarrow 0$. To this end we apply the method of the residues to the integral defining it. Precisely, we prove the following Lemma

Lemma A.2

Define

$$\begin{aligned} \rho := \frac{{L}^2}{E^{\frac{(\ell +1)}{\ell }}}, \end{aligned}$$

(A.2)

then there exists $\rho _*$ and a function f(E, L), analytic in the domain

$$\begin{aligned} E>0, \quad 0\le \rho <\rho _*, \end{aligned}$$

(A.3)

s.t.

$$\begin{aligned} a_r = -\frac{1}{2} |L| + f(E, L). \end{aligned}$$

(A.4)

Proof

Performing the change of variables $r^2 =s,$ in the integral defining $a_r$ one has

$$\begin{aligned} {a_r}= \frac{\sqrt{2}}{2 \pi } \int _{s_m}^{s_M} \frac{1}{s}\sqrt{-p(s, E, {L})}\ d s, \end{aligned}$$

(A.5)

with

$$\begin{aligned} {p(s, E, {L}) = \frac{s^{\ell +1}}{2\ell } - Es + \frac{{L}^2}{2}} \end{aligned}$$

(A.6)

and $s_m = s_m(E, {L}),$ $s_M=s_M(E, {L})$ the two positive solutions of the equation ${p(s, E, {L}) = 0.}$

We now study the zeroes of $p(s, E, {L})$. To this aim we observe that, since p is a polynomial in s with coefficients depending on E and ${L}$, it has $\ell +1$ complex roots which of course depend continuously on E and L. Actually there is more structure: indeed $s_*(E,L)$ is a root of p if and only if $t_*=s_*E^{-1/\ell }$ is a root of

$$\begin{aligned} {{\tilde{p}}}(t) = \frac{t^{\ell +1}}{2\ell } - t + \frac{1}{2}\rho , \end{aligned}$$

(A.7)

which is a function of $\rho$ only.

Since we are interested in a neighborhood of $\rho =0$, we start by remarking that, for $\rho =0$ the roots of ${{\tilde{p}}}$ are

$$\begin{aligned} t_0(0) = 0, \quad \left( t_j(0)\right) ^{\ell } = 2 \ell \quad \forall j = 1, \dots , \ell . \end{aligned}$$

(A.8)

To be determined we take $t_1(0):=(2\ell )^{1/\ell }$ to be the positive real root of $2\ell$ and the other roots in counterclockwise order. Correspondingly we will have

$$\begin{aligned} E^{1/\ell }t_0(\rho )=s_m(E,L),\quad E^{1/\ell }t_1(\rho )=s_M(E,L). \end{aligned}$$

Denote

$$\begin{aligned} d:=\min _{j\not =l}\left| t_j(0)-t_l(0)\right| , \end{aligned}$$

then there exists $\rho _*$ s.t., for $\rho <\rho _*$ one has

$$\begin{aligned} \min _{j\not =l}\left| t_j(\rho )-t_l(\rho )\right| \ge d/2 \end{aligned}$$

and correspondingly

$$\begin{aligned} \min _{j\not =l}\left| s_j(E, {L}) - s_{l}(E, {L})\right| \ge \frac{d}{2} E^{\frac{1}{\ell }} \end{aligned}$$

(A.9)

The function to be integrated in (A.5) is

$$\begin{aligned} F(z) := \frac{\sqrt{2}}{2\pi }\frac{1}{z} \sqrt{- p(z, E, {L})}, \end{aligned}$$

(A.10)

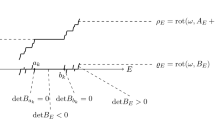
In order to make it holomorphic we cut ${\mathbb {C}}$ along the segments $b_j$ joining $s_{2(j-1)}(E, {L})$ with $s_{2 j - 1}(E, {L})$, $j=1,\ldots ,\lceil \frac{\ell }{2} \rceil$; if $\ell$ is odd, there is a last cut $b_{\lceil \frac{\ell }{2} \rceil }$, which is the half-line parallel to the real axis joining $s_{\ell }(E, {L})$ with $\infty$. Remark that $b_1$ is the interval of integration in which we are interested.

We are now ready to choose the curve over we integrate to apply the method of the residue. To this end we define

$$\begin{aligned} \mathtt{M} := \max _{j=1,..,\ell , \rho <\rho _*} {\text {Re}} \left( t_j(\rho )\right) , \end{aligned}$$

(A.11)

and take the curve $\Gamma _\varepsilon$ described in Fig. 1, with

$$\begin{aligned} \begin{aligned} a = \left( -\frac{d}{4}- \mathrm{i}\frac{d}{4}\right) E^{\frac{1}{\ell }}, \quad b= \left( 2\mathtt{M} - \mathrm{i}\frac{d}{4} \right) E^{\frac{1}{\ell }},\\ c =\left( 2 \mathtt{M} + \mathrm{i}\frac{d}{4}\right) E^{\frac{1}{\ell }}, \quad d = \left( -\frac{d}{4} + \mathrm{i}\frac{d}{4}\right) E^{\frac{1}{\ell }}. \end{aligned} \end{aligned}$$

(A.12)

We also denote $\gamma _R$ the boundary of the rectangle abcd. With this notation we have

$$\begin{aligned} \begin{aligned} 2 a_r&= \lim _{\varepsilon \rightarrow 0} \int _{\Gamma _\varepsilon } F(z)\ d z - \int _{\gamma _R} F(z)\ d z\\&= 2 \pi \mathrm{i}\text {Res}(F, 0) - \int _{\gamma _R} F(z)\ d z=-|{L}| - \int _{\gamma _R} F(z)\ d z. \end{aligned} \end{aligned}$$

(A.13)

To get the thesis just define

$$\begin{aligned} f(E,L):= - \int _{\gamma _R} F(z)\ d z, \end{aligned}$$

and remark that this is analytic in the considered domain. $\square$

Fig. 1

Representation of the path $\Gamma _\varepsilon$. The radius of the circles is $\varepsilon$, and the points a, b, c, d are defined as in (A.12)

Joining the results of these two lemmas one gets

Corollary A.3

There exists function $f=f(E,L)$ analytic in the domain

$$\begin{aligned} \left\{ (E, L)\ \left| \ E>0,\ |L| < \left( \frac{2\ell }{\ell + 1} E\right) ^{\frac{\ell +1}{2 \ell }}\right. \right\} , \end{aligned}$$

(A.14)

such that

$$\begin{aligned} a_r(E, L) = -\frac{1}{2}|L| + f(E, L). \end{aligned}$$

(A.15)

We finally prove Lemma 4.5:

Proof of Lemma 4.5

Let

$$\begin{aligned} a_1 := {\left\{ \begin{array}{ll} a_r &{} \text {if } L\ge 0\\ a_r - L &{}\text {if } L< 0. \end{array}\right. } \end{aligned}$$

(A.16)

then, by Corollary A.15, there exists a function f analytic in the domain (A.14) s.t.

$$\begin{aligned} a_1=-\frac{L}{2}+f(E,L), \end{aligned}$$

so that $a_1$ itself is analytic in such a domain. This concludes the proof of Items (1) and (2).

We come to the homogeneity properties. Take $L\not =0$, then by (4.4) and performing the change of variables $r = |L|^{\frac{1}{\ell +1}} x$ in (4.4), one sees that

$$\begin{aligned} a_r = \frac{\sqrt{2 E}}{\pi } |L|^{\frac{1}{\ell +1}} F(\rho ), \quad F(\rho ) = \int _{x_m}^{x_M} \sqrt{1-\rho \left( \frac{1}{2x^2} + \frac{x^{2\ell }}{2\ell }\right) }\,d x, \end{aligned}$$

with $\rho$ as in (A.7) and $x_m, x_M$ solving the equation $\displaystyle {\rho \frac{x^{2\ell }}{2\ell } + \rho \frac{1}{2x^2} -1 = 0.}$ As a consequence, for any $\lambda >0$ $a_r$ satisfies

$$\begin{aligned} a_r(\lambda ^{\frac{2\ell }{\ell +1}}E, \lambda L) = \lambda ^{\frac{\ell }{\ell +1}} \sqrt{2 E} \lambda ^{\frac{1}{\ell +1}} |L| F(\rho ) =\lambda a_r(E, L), \end{aligned}$$

also $a_1$ defined as in (A.16) satisfies

$$\begin{aligned} a_1(\lambda ^{\frac{2\ell }{\ell +1}}E, \lambda L) = \lambda a_r(E, L). \end{aligned}$$

(A.17)

Since $h_0$ and $a_2$ as in (4.2) are quasi-homogeneous functions of $x, \xi$, one immediately deduces the quasi-homogeneity of $a_1$. The homogeneity of $h_0$ as a function of a also immediately follows from (A.17). We still have to consider the case $L=0$. In this case the result immediately follows by continuity, by taking the limit of the functions to this set. $\square$

Appendix B: Proof of Lemma 5.12

First we prove the following

Lemma B.1

Let $\Pi ^*:=a^{-1}(\mathop {\Pi }^\circ )$ and suppose $f\in C^{\infty }({\mathbb {R}}^2)$ is such that $f\circ \phi ^{\varphi }_a=f$, $\forall \varphi$ then there exists ${{\tilde{f}}}\in C^{\infty }(\mathop \Pi ^{\circ })$ s.t. $f={{\tilde{f}}}\circ a$ on $\Pi ^*$.

Proof

Introducing action angle coordinates $(a,\varphi )$, which by the standard theory (see e.g. [Dui80]) are smooth and globally defined on the set $\Pi ^*$, the function f is a $C^\infty$ function of $(a,\varphi )$ which however does not depend on $\varphi$. This is the wanted function ${{\tilde{f}}}$.

$\square$

Lemma B.2

There exist angle variables $\varphi$ that are quasi-homogeneous of degree 0 as functions on $\Pi ^*$, namely they satisfy

$$\begin{aligned} \varphi (\lambda x, \lambda ^\ell \xi ) = \varphi (x, \xi ) \quad \forall (x, \xi ) \in \Pi ^*,\ \forall \lambda \in {\mathbb {R}}^+. \end{aligned}$$

Proof

Let ${\varphi } = (\varphi _1, \varphi _2): \Pi ^* \rightarrow {\mathbb {T}}^2$ be such that $(a, {\varphi })$ are global action angle coordinates. For any choice of ${{\bar{a}}}\in \mathop \Pi ^{\circ }$ with $\Vert {{\bar{a}}}\Vert =1$, let $(x_0, \xi _0) \in \Pi ^*$ be such that $a(x_0,\xi _0)={{\bar{a}}}$ and $\varphi (x_0, \xi _0) = 0$. Remark that it exists because the action $\phi _a^{\varphi }$ on the level surfaces of a is transitive. Furthermore $(x_0,\xi _0)$ is a function of a only. For $(x, \xi ) \in \Pi ^*$, define $\lambda \in {\mathbb {R}}^+$ and $({{\widetilde{x}}},\widetilde{\xi })$ by

$$\begin{aligned} (x, \xi ) = (\lambda {\tilde{x}}, \lambda ^\ell {\tilde{\xi }}), \quad \text {and}\quad \Vert a({\widetilde{x}}, {\widetilde{\xi }})\Vert = 1. \end{aligned}$$

(B.1)

Observe that with this definition $\lambda$ is a function of the actions a only. Then this implies that the function ${\tilde{\varphi }} = ({\tilde{\varphi }}_1, {\tilde{\varphi }}_2): \Pi ^* \rightarrow {\mathbb {T}}^2$ given by

$$\begin{aligned} {\tilde{\varphi }}(x, \xi ) := \varphi (x, \xi ) - \varphi (\lambda {x_0}, \lambda ^{\ell } {\xi _0}) \end{aligned}$$

(B.2)

still defines angle coordinates conjugated to the actions a on the set $\Pi ^*$. This is due to the fact that $\varphi (\lambda {x_0},\lambda ^\ell \xi _0)$ is a function of the actions only. Remark also that

$$\begin{aligned} {{\tilde{\varphi }}}(\lambda x_0,\lambda ^\ell \xi _0) =0,\quad \forall \lambda >0. \end{aligned}$$

From now on we use only the angles ${{\tilde{\varphi }}}$, so we omit the tildes.

We are now going to prove that these angles are homogeneous functions of degree 0 on $\Pi ^*$.

To this aim, we define for $\mu >0$ and $j = 1,2$

$$\begin{aligned} \varphi _{\mu , j}(x, \xi ):= \varphi _j(\mu x, \mu ^{\ell }\xi ) \end{aligned}$$

First of all, we observe that since $(a, \varphi )$ are canonically conjugated variables, one has $\displaystyle {\left\{ a_j;\varphi _i\right\} = \delta _{i, j}}$, so one has

$$\begin{aligned} \begin{aligned} \left\{ a_i;\varphi _{\mu , j}\right\} (x, \xi ) = \mu ^{\ell }\partial _{x} a_i (x, \xi ) \cdot \partial _{\xi } \varphi _{j} (\mu x, \mu ^{\ell }\xi ) - \mu \partial _{\xi } a_i (x, \xi ) \cdot \partial _{x} \varphi _{j} (\mu x, \mu ^{\ell } \xi ). \end{aligned} \end{aligned}$$

Since $a_i$ is quasi-homogeneous of degree $\ell +1,$ $\forall (x, \xi ) \in \Pi ^*$ one has

$$\begin{aligned} \begin{aligned} \mu ^{\ell }\partial _{x} a_i (x, \xi ) = \partial _{x} a_i(\mu x, \mu ^{\ell } \xi ), \\ \mu \partial _{\xi } a_i (x, \xi ) = \partial _{\xi }a_i(\mu x, \mu ^{\ell } \xi ), \end{aligned} \end{aligned}$$

one also obtains

$$\begin{aligned} \begin{aligned} \left\{ a_i;\varphi _{\mu , j}\right\} (x, \xi ) = \left\{ a_i;\varphi _{ j}\right\} (\mu x, \mu ^{\ell }\xi ) \equiv \delta _{i,j}. \end{aligned} \end{aligned}$$

(B.3)

Thus, using action angle coordinates to compute Poisson Brackets, one has

$$\begin{aligned} \left\{ a_j;\varphi _{\mu ,j}\right\} \equiv \frac{\partial \varphi _{\mu ,j}}{\partial \varphi _j} =1, \end{aligned}$$

therefore there exist functions $f_{\mu , j}(a)$, depending on the actions only, such that

$$\begin{aligned} {\varphi }_{\mu , j} - {\varphi }_{j} = f_{\mu , j}(a). \end{aligned}$$

To prove that $f_{\mu ,j}$ is identically zero, fix a value ${{\bar{a}}}$ of a, corresponding to some point $({{\bar{x}}},{{\bar{\xi }}})\in \Pi ^*$ define ${\lambda }:=\Vert {{\bar{a}}}\Vert ^{\frac{1}{\ell +1}}$, then we have

$$\begin{aligned} f_{\mu , j}(a({{\bar{x}}},{{\bar{\xi }}}))&=f_{\mu , j}\left( \lambda ^{\ell +1}a\left( \frac{{{\bar{x}}}}{ \lambda },\frac{{{\bar{\xi }}}}{\lambda ^\ell }\right) \right) =f_{\mu , j}(\lambda ^{\ell +1}{a({x_0},{\xi _0})}) \\&=f_{\mu , j}\left( a\left( {\lambda } {{x_0}}, {\lambda ^\ell } {{\xi _0}}\right) \right) = {\varphi }_{\mu , j}\left( {\lambda } x_0, {\lambda ^\ell } \xi _0\right) - {\varphi }_{j} \left( {\lambda } x_0, {\lambda ^\ell } \xi _0\right) \\&=0-0=0. \end{aligned}$$

$\square$

Corollary B.3

Let ${\check{x}}, {\check{\xi }}: \Pi ^\circ \times {\mathbb {T}}^2\rightarrow \Pi ^*$ be the functions expressing the Cartesian coordinates in terms of the action angle coordinates, namely s.t.

$$\begin{aligned} ({\check{x}}, {\check{\xi }}) \circ (a, \varphi ) = \text {Id}, \quad (a, \varphi ) \circ ({\check{x}}, {\check{\xi }}) = \text {Id}. \end{aligned}$$

Then the following holds:

$$\begin{aligned} {\check{x}} (\lambda ^{\ell +1}a, \varphi ) = \lambda {\check{x}}(a, \varphi ), \quad {\check{\xi }} (\lambda ^{\ell +1}a, \varphi ) = \lambda ^{\ell } {\check{\xi }}(a, \varphi ) \quad \forall \lambda \in {\mathbb {R}}^+,\ (a, \varphi ) \in \Pi ^\circ \times {\mathbb {T}}^2.\nonumber \\ \end{aligned}$$

(B.4)

We now prove Lemma 5.12:

Proof

By Lemma B.1, there exists ${\tilde{f}} \in C^\infty (\mathop \Pi ^{\circ })$ such that $f = {\tilde{f}} \circ a$. Passing to action angle variables on the set $\Pi ^*$, one has

$$\begin{aligned} {\tilde{f}}(a) ={f} ({\check{x}}(a, \varphi ), {\check{\xi }}(a, \varphi )) \end{aligned}$$

First we prove the estimates for $a\in \mathop \Pi ^{\circ }$. Consider for example

$$\begin{aligned} \partial _{a_1} {\tilde{f}} = \partial _x {f} \cdot \partial _{a_1} {\check{x}} + \partial _\xi {f} \cdot \partial _{a_1} {{\check{\xi }}} \end{aligned}$$

thus, using ${f} \in S_{AN,\delta }^{m},$ and the estimates

$$\begin{aligned} \left| \partial _a^{\beta }{\check{x}}(a, \varphi )\right| \lesssim _{} \langle a \rangle ^{\frac{1}{\ell + 1} - |\beta |}, \quad \left| \partial _a^{\beta }{{\check{\xi }}}(a, \varphi )\right| \lesssim _{} \langle a \rangle ^{\frac{1}{\ell + 1} - |\beta |}. \end{aligned}$$

which follow from (B.4) we can estimate such a quantity. One gets for $a \in \Pi ^\circ$

$$\begin{aligned} | \partial _a {\tilde{f}}| \lesssim \langle a \rangle ^{m-\delta _1 -\frac{\ell }{\ell + 1}} + \langle a \rangle ^{m-\delta _2 -\frac{1}{\ell + 1}}\simeq \langle a\rangle ^{m-\varsigma }. \end{aligned}$$

Iterating and studying the other derivatives, one also has that $\forall \alpha \in {\mathbb {N}}^2$, implies

$$\begin{aligned} | \partial _a^\alpha {\tilde{f}}| \lesssim \langle a \rangle ^{m-\varsigma |\alpha |}, \end{aligned}$$

but just for $a \in \Pi ^\circ$. To get a symbol defined on the whole of ${\mathbb {R}}^2$, we consider again the cones ${{\mathcal {V}}}$ and ${{\mathcal {C}}}$ and the cutoff function $\Psi$ supported in ${{\mathcal {V}}}$ and equal to one in ${{\mathcal {C}}}$, and just consider the function

$$\begin{aligned} f_c(a):={{\tilde{f}}}(a)\Psi (a)(1-\chi (\left\| a\right\| )), \end{aligned}$$

which has all the claimed properties. $\square$