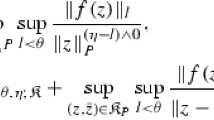Abstract
We study a general family of space–time discretizations of the KPZ equation and show that they converge to its solution. The approach we follow makes use of basic elements of the theory of regularity structures (Hairer in Invent Math 198(2):269–504, 2014) as well as its discrete counterpart (Hairer and Matetski in Discretizations of rough stochastic PDEs, 2015. arXiv:1511.06937). Since the discretization is in both space and time and we allow non-standard discretization for the product, the methods mentioned above have to be suitably modified in order to accommodate the structure of the models under study.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Bahouri, H., Chemin, J.-Y., Danchin, R.: Fourier analysis and nonlinear partial differential equations, vol. 343 of Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences]. Springer, Heidelberg (2011). https://doi.org/10.1007/978-3-642-16830-7
Bertini, L., Giacomin,G.: StochasticBurgers andKPZ equations fromparticle systems.Commun. Math. Phys. 183(3), 571–607 (1997). https://doi.org/10.1007/s002200050044
Blondel, O., Gonçalves, P.C., Simon, M.: Convergence to the stochastic Burgers equation from a degenerate microscopic dynamics. Electron. J. Probab. 21, Paper No. 69, 25 (2016). https://doi.org/10.1214/16-EJP15
Brenner, S.C., Scott, L.R.: The mathematical theory of the element methods, vol. 15 of Texts in Applied Mathematics. Springer, New York, third ed., (2008) https://doi.org/10.1007/978-0-387-75934-0
Corwin, I., Shen, H.: Open ASEP in the weakly asymmetric regime (2016). arXiv:1610.04931
Corwin, I., Shen, H., Tsai, L.-C.: ASEP(q,j) converges to the KPZ equation (2016). arXiv:1602.01908
Corwin, I., Tsai, L.-C.: KPZ equation limit of higher-spin exclusion processes. Ann. Probab. 45(3), 1771–1798 (2017). https://doi.org/10.1214/16-AOP1101
Chandra, A., Weber, H.: Stochastic PDEs, regularity structures, and interacting particle systems (2015). arXiv:1508.03616
Daubechies, I.: Ten lectures on wavelets, vol. 61 of CBMS-NSF Regional Conference Series in Applied Mathematics. Society for Industrial and Applied Mathematics (SIAM), Philadelphia, PA (1992). https://doi.org/10.1137/1.9781611970104
Diehl, J., Gubinelli, M., Perkowski, N.: The Kardar-Parisi-Zhang equation as scaling limit of weakly asymmetric interacting Brownian motions. Commun. Math. Phys. 354(2), 549–589 (2017). https://doi.org/10.1007/s00220-017-2918-6
Dembo,A., Tsai, L.-C.:Weakly asymmetric non-simple exclusion process and the Kardar-Parisi-Zhang equation. Commun. Math. Phys. 341(1), 219–261 (2016). https://doi.org/10.1007/s00220-015-2527-1
Franco T., Gonçalves P., Simon M.: Crossover to the stochastic Burgers equation for the WASEP with a slow bond. Commun. Math. Phys. 346(3), 801–838 (2016) https://doi.org/10.1007/s00220-016-2607-x
Friz, P.K., Hairer, M.: A course on rough paths. Universitext. Springer, Cham, With an introduction to regularity structures (2014). https://doi.org/10.1007/978-3-319-08332-2
Funaki T., Hoshino M.: A coupled KPZ equation, its two types of approximations and existence of global solutions. J. Funct. Anal. 273(3), 1165–1204 (2017) https://doi.org/10.1016/j.jfa.2017.05.002
Gärtner J.: Convergence towards Burgers’ equation and propagation of chaos for weakly asymmetric exclusion processes. Stoch. Process. Appl. 27(2), 233–260 (1988) https://doi.org/10.1016/0304-4149(87)90040-8
Gubinelli, M., Imkeller, P., Perkowski, N.: Paracontrolled distributions and singular PDEs. Forum Math. Pi 3, e6, 75. (2015). https://doi.org/10.1017/fmp.2015.2
Gonçalves, P., Jara, M.: Universality of KPZ equation (2010). arXiv:1003.4478
Gubinelli M., Jara M.: Regularization by noise and stochastic Burgers equations. Stoch. Partial Differ. Equ. Anal. Comput. 1(2), 325–350 (2013) https://doi.org/10.1007/s40072-013-0011-5
Gonçalves P., Jara M.: Nonlinear fluctuations of weakly asymmetric interacting particle systems. Arch. Ration. Mech. Anal. 212(2), 597–644 (2014) https://doi.org/10.1007/s00205-013-0693-x
Gonçalves P., Gonçalves P.: Stochastic Burgers equation from long range exclusion interactions. Stoch. Process. Appl. 127(12), 4029–4052 (2017) https://doi.org/10.1016/j.spa.2017.03.022
Gonçalves P., Jara M., Simon M.: Second order Boltzmann-Gibbs principle for polynomial functions and applications. J. Stat. Phys. 166(1), 90–113 (2017) https://doi.org/10.1007/s10955-016-1686-6
Gubinelli, M., Perkowski, N.: Energy solutions of KPZ are unique. J. Am. Math. Soc. https://doi.org/10.1090/jams/889
Gubinelli, M., Perkowski, N.: The Hairer–Quastel universality result in equilibrium (2016). arXiv:1602.02428
Gubinelli M., Perkowski N.: KPZ reloaded. Commun. Math. Phys. 349(1), 165–269 (2017) https://doi.org/10.1007/s00220-016-2788-3
Gubinelli M.: Controlling rough paths. J. Funct. Anal. 216(1), 86–140 (2004) https://doi.org/10.1016/j.jfa.2004.01.002
Hairer M.: Solving the KPZ equation. Ann. Math. (2) 178(2), 559–664 (2013) https://doi.org/10.4007/annals.2013.178.2.4 arXiv:1109.6811
Hairer M.: A theory of regularity structures. Invent. Math. 198(2), 269–504 (2014) https://doi.org/10.1007/s00222-014-0505-4 arXiv:1303.5113
Hairer M.: Introduction to regularity structures. Braz. J. Probab. Stat. 29(2), 175–210 (2015) https://doi.org/10.1214/14-BJPS241
Hairer, M., Matetski, K.: Discretisations of rough stochastic PDEs (2015). arXiv:1511.06937
Hoshino, M.: Paracontrolled calculus and Funaki-Quastel approximation for the KPZ equation (2016). arXiv:1605.02624
Hairer, M., Quastel, J.: A class of growth models rescaling to KPZ (2015). arXiv:1512.07845
Hairer M., Shen H.: A central limit theorem for the KPZ equation. Ann. Probab. 45(6B), 4167–4221 (2017) https://doi.org/10.1214/16-AOp1162
Kallenberg, O.: Foundations of modern probability. Probability and its Applications (New York). Springer-Verlag, New York, second ed., (2002)
Kardar M., Parisi G., Zhang Y.-C.: Dynamic scaling of growing interfaces. Phys. Rev. Lett. 56(9), 889–892 (1986)
Labbé C.: Weakly asymmetric bridges and the KPZ equation. Commun. Math. Phys. 353(3), 1261–1298 (2017) https://doi.org/10.1007/s00220-017-2875-0
Lyons T.J.: Differential equations driven by rough signals. Rev. Mat. Iberoamericana 14(2), 215–310 (1998) https://doi.org/10.4171/RMI/240
Meyer, Y.: Wavelets and operators, vol. 37 of Cambridge Studies in Advanced Mathematics. Cambridge University Press, Cambridge, Translated from the 1990 French original by D. H. Salinger (1992)
Mueller C.: On the support of solutions to the heat equation with noise. Stoch. Stoch. Rep. 37(4), 225–245 (1991)
Nelson E.: The free Markoff field. J. Funct. Anal. 12, 211–227 (1973) https://doi.org/10.1016/0022-1236(73)90025-6
Nualart, D.: The Malliavin calculus and related topics. Probability and its Applications (New York). Springer-Verlag, Berlin, second ed. (2006)
Sasamoto T., Spohn H.: Superdiffusivity of the 1D lattice Kardar-Parisi-Zhang equation. J. Stat. Phys. 137(5-6), 917–935 (2009)
Walsh, J.B.: An introduction to stochastic partial differential equations. In École d’été de probabilités de Saint-Flour, XIV—1984, vol. 1180 of Lecture Notes in Math., 265–439. Springer, Berlin, (1986). https://doi.org/10.1007/BFb0074920
Zabusky N.J., Kruskal M.D.: Interaction of “solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15, 240–243 (1965) https://doi.org/10.1103/PhysRevLett.15.240
Zhu, R., Zhu, X.: Approximating three-dimensional Navier–Stokes equations driven by space–time white noise (2014). arXiv:1409.4864
Zhu, R., Zhu, X.: Lattice approximation to the dynamical \({\phi_3^4}\) model (2015). arXiv:1508.05613
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by H. Spohn
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Cannizzaro, G., Matetski, K. Space–Time Discrete KPZ Equation. Commun. Math. Phys. 358, 521–588 (2018). https://doi.org/10.1007/s00220-018-3089-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00220-018-3089-9