Stationary Random Metrics on Hierarchical Graphs Via ${(\min,+)}$-type Recursive Distributional Equations

Abstract

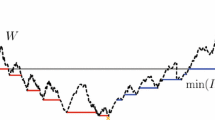
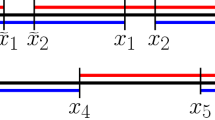
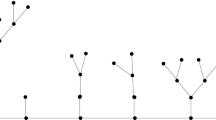
This paper is inspired by the problem of understanding in a mathematical sense the Liouville quantum gravity on surfaces. Here we show how to define a stationary random metric on self-similar spaces which are the limit of nice finite graphs: these are the so-called hierarchical graphs. They possess a well-defined level structure and any level is built using a simple recursion. Stopping the construction at any finite level, we have a discrete random metric space when we set the edges to have random length (using a multiplicative cascade with fixed law ${m}$). We introduce a tool, the cut-off process, by means of which one finds that renormalizing the sequence of metrics by an exponential factor, they converge in law to a non-trivial metric on the limit space. Such limit law is stationary, in the sense that glueing together a certain number of copies of the random limit space, according to the combinatorics of the brick graph, the obtained random metric has the same law when rescaled by a random factor of law ${m}$ . In other words, the stationary random metric is the solution of a distributional equation. When the measure m has continuous positive density on ${\mathbf{R}_{+}}$, the stationary law is unique up to rescaling and any other distribution tends to a rescaled stationary law under the iterations of the hierarchical transformation. We also investigate topological and geometric properties of the random space when m is log-normal, detecting a phase transition influenced by the branching random walk associated to the multiplicative cascade.