Abstract
In a recent work (Feischl et al. in Eng Anal Bound Elem 62:141–153, 2016), we analyzed a weighted-residual error estimator for isogeometric boundary element methods in 2D and proposed an adaptive algorithm which steers the local mesh-refinement of the underlying partition as well as the multiplicity of the knots. In the present work, we give a mathematical proof that this algorithm leads to convergence even with optimal algebraic rates. Technical contributions include a novel mesh-size function which also monitors the knot multiplicity as well as inverse estimates for NURBS in fractional-order Sobolev norms.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
1.1 Isogeometric analysis
The central idea of isogeometric analysis (IGA) is to use the same ansatz functions for the discretization of the partial differential equation at hand, as are used for the representation of the problem geometry. Usually, the problem geometry \({\varOmega }\) is represented in CAD by means of non-uniform rational B-splines (NURBS), T-splines, or hierarchical splines. This concept, originally invented in [32] for finite element methods (IGAFEM) has proved very fruitful in applications; see also the monograph [9].
Since CAD directly provides a parametrization of the boundary \(\partial {\varOmega }\), this makes the boundary element method (BEM) the most attractive numerical scheme, if applicable (i.e., provided that the fundamental solution of the differential operator is explicitly known). However, compared to the IGAFEM literature, only little is found for isogeometric BEM (IGABEM). The latter has first been considered for 2D BEM in [36] and for 3D BEM in [40]. Unlike standard BEM with piecewise polynomials which is well-studied in the literature, cf. the monographs [39, 41] and the references therein, the numerical analysis of IGABEM is widely open. We refer to [35, 37, 38, 42] for numerical experiments, to [44] for fast IGABEM with \({\mathcal {H}}\)-matrices, and to [31] for some quadrature analysis. To the best of our knowledge, a posteriori error estimation for IGABEM, however, has only been considered for simple 2D model problems in the recent own works [24, 25]. The present work extends the techniques from standard BEM to non-polynomial ansatz functions. The remarkable flexibility of the IGA ansatz functions to manipulate their smoothness properties motivates the development of a new adaptive algorithm which does not only automatically adapt the mesh-width, but also the continuity of the IGA ansatz function to exploit the additional freedoms and the full potential of IGA. This is the first algorithm which simultaneously steers the resolution and the smoothness of the ansatz functions, and, it may thus be a first step to a full hpk-adaptive algorithm.
For standard BEM with discontinuous piecewise polynomials, a posteriori error estimation and adaptive mesh-refinement are well understood. We refer to [1, 11, 12] for weighted-residual error estimators and to [19, 22] for recent overviews on available a posteriori error estimation strategies. Moreover, optimal convergence of mesh-refining adaptive algorithms has recently been proved for polyhedral boundaries [20, 21, 26] as well as smooth boundaries [27]. The work [2] allows to transfer these results to piecewise smooth boundaries; see also the discussion in the review article [8].
While this work focusses on adaptive IGABEM, adaptive IGAFEM is considered, e.g., in [16, 43]. A rigorous error and convergence analysis in the frame of adaptive IGAFEM is first found in [5] which proves linear convergence for some adaptive IGAFEM with hierarchical splines for the Poisson equation, and optimal rates are announced for some future work.
1.2 Model problem
We develop and analyze an adaptive algorithm for the following model problem: Let \({\varOmega }\subset \mathbb {R}^2\) be a Lipschitz domain with \(\mathrm{diam}({\varOmega })<1\) and \({\varGamma }\subseteq \partial {\varOmega }\) be a compact, piecewise smooth part of its boundary with finitely many connected components. We consider the weakly-singular boundary integral equation
where the right-hand side f is given and the density \(\phi \) is sought. We note that (1.1) for \({\varGamma }=\partial {\varOmega }\) is equivalent to the Laplace–Dirichlet problem
To approximate \(\phi \), we employ a Galerkin boundary element method (BEM) with ansatz spaces consisting of p-th order NURBS. The convergence order for uniform partitions of \({\varGamma }\) is usually suboptimal, since the unknown density \(\phi \) may exhibit singularities, which are stronger than the singularities in the geometry. In [24], we analyzed a weighted-residual error estimator and proposed an adaptive algorithm which uses this a posteriori error information to steer the h-refinement of the underlying partition as well as the local smoothness of the NURBS across the nodes of the adaptively refined partitions. It reflects the fact that it is a priori unknown, where the singular and smooth parts of the density \(\phi \) are located and where approximation by nonsmooth resp. smooth functions is required. In [24], we observed experimentally that the proposed algorithm detects singularities and possible jumps of \(\phi \) and leads to optimal convergence behavior. In particular, we observed that the proposed adaptive strategy is also superior to adaptive BEM with discontinuous piecewise polynomials in the sense that our adaptive NURBS discretization requires less degrees of freedom to reach a prescribed accuracy.
1.3 Contributions
We prove that the adaptive algorithm from [24] is rate optimal in the sense of [8]: Let \(\mu _\ell \) be the weighted-residual error estimator in the \(\ell \)-th step of the adaptive algorithm. First, the adaptive algorithm leads to linear convergence of the error estimator, i.e., \(\mu _{\ell +n} \le Cq^n\mu _\ell \) for all \(\ell ,n\in \mathbb {N}_0\) and some independent constants \(C>0\) and \(0<q<1\). Moreover, for sufficiently small marking parameters, i.e. aggressive adaptive refinement, the estimator decays even with the optimal algebraic convergence rate. Here, the important innovation is that the adaptive algorithm does not only steer the local refinement of the underlying partition (as is the case in the available literature, e.g., [8, 20, 21, 26, 27]), but also the multiplicity of the knots. In particular, the present work is the first available optimality result for adaptive algorithms in the frame of isogeometric analysis. Additionally, we can prove at least plain convergence if the adaptive algorithm is driven by the Faermann estimator \(\eta _\ell \) analyzed in [25] instead of the weighted-residual estimator \(\mu _\ell \), which generalizes a corresponding result for standard adaptive BEM [23].
Technical contributions of general interest include a novel mesh-size function \(h\in L^\infty ({\varGamma })\) which is locally equivalent to the element length (i.e., \(h|_T \simeq \mathrm{length}(T)\) for all elements T), but also accounts for the knot multiplicity. Moreover, for \(0<\sigma <1\), we prove a local inverse estimate \(\Vert h^{\sigma }{\varPsi }\Vert _{L^2({\varGamma })} \le C\,\Vert {\varPsi }\Vert _{\widetilde{H}^{-\sigma }({\varGamma })}\) for NURBS on locally refined meshes. Similar estimates for piecewise polynomials are shown in [15, 29, 30], while [3] considers NURBS but integer-order Sobolev norms only.
Throughout, all results apply for piecewise smooth parametrizations \(\gamma \) of \({\varGamma }\) and discrete NURBS spaces. In particular, the analysis thus covers the NURBS ansatz used for IGABEM, where the same ansatz functions are used for the discretization of the integral equation and for the resolution of the problem geometry, as well as spline spaces and even piecewise polynomials on the piecewise smooth boundary \({\varGamma }\) which can be understood as special NURBS.
1.4 Outline
The remainder of this work is organized as follows: Sect. 2 fixes the notation and provides the necessary preliminaries. This includes, e.g., the involved Sobolev spaces (Sect. 2.2), the functional analytic setting of the weakly-singular integral equation (Sect. 2.3), the assumptions on the parametrization of the boundary \({\varGamma }\) (Sect. 2.4), the discretization of the boundary (Sect. 2.5), the mesh-refinement strategy (Sect. 2.6), B-splines and NURBS (Sect. 2.7), and the IGABEM ansatz spaces (Sect. 2.8). Section 3 states our adaptive algorithm (Algorithm 3.1) from [24] and formulates the main theorems on linear convergence with optimal rates for the weighted-residual estimator \(\mu _\ell \) (Theorem 3.2) and on plain convergence for the Faermann estimator \(\eta _\ell \) (Theorem 3.4). The linear convergence for the \(\mu _\ell \)-driven algorithm is proved in Sect. 4. The proof requires an inverse estimate for NURBS in a fractional-order Sobolev norm (Proposition 4.1) as well as a novel mesh-size function for B-spline and NURBS discretizations (Proposition 4.2) which might be of independent interest. The proof of optimal convergence behaviour is given in Sect. 5. In Sect. 6, we show convergence for the \(\eta _\ell \)-driven algorithm.
For the empirical verification of the optimal convergence behavior of Algorithm 3.1 for \(\mu _\ell \)- as well as \(\eta _\ell \)-driven adaptivity and a comparison of IGABEM and standard BEM with discontinuous piecewise polynomials, we refer to the numerous numerical experiments in our preceding work [24].
2 Preliminaries
2.1 General notation
Throughout, \(|\cdot |\) denotes the absolute value of scalars, the Euclidean norm of vectors in \(\mathbb {R}^2\), the measure of a set in \(\mathbb {R}\) (e.g., the length of an interval), or the arclength of a curve in \(\mathbb {R}^{2}\). The respective meaning will be clear from the context. We write \(A\lesssim B\) to abbreviate \(A\le cB\) with some generic constant \(c>0\) which is clear from the context. Moreover, \(A\simeq B\) abbreviates \(A\lesssim B\lesssim A\). Throughout, mesh-related quantities have the same index, e.g., \(\mathcal {N}_\star \) is the set of nodes of the partition \(\mathcal {T}_\star \), and \(h_\star \) is the corresponding local mesh-width etc. The analogous notation is used for partitions \(\mathcal {T}_+\) resp. \(\mathcal {T}_\ell \) etc.
2.2 Sobolev spaces
For any measurable subset \({{\varGamma }_0}\subseteq {\varGamma }\), let \(L^2({{\varGamma }_0})\) denote the Lebesgue space of all square integrable functions which is associated with the norm \(\Vert u\Vert _{L^2({{\varGamma }_0})}^2:=\int _{{\varGamma }_0} |u(x)|^2\,dx\). We define for any \(0<\sigma \le 1\) the Hilbert space
associated with the Sobolev–Slobodeckij norm
with
where \(\partial _\gamma \) denotes the arclength derivative. For finite intervals \(I\subseteq \mathbb {R}\), we use analogous definitions. By \(\widetilde{H}^{-\sigma }({{\varGamma }_0})\), we denote the dual space of \(H^{\sigma }({{\varGamma }_0})\), where duality is understood with respect to the extended \(L^2({{\varGamma }_0})\)-scalar product, i.e.,
We note that \(H^{\sigma }({\varGamma })\subset L^2({\varGamma })\subset \widetilde{H}^{-\sigma }({\varGamma })\) form a Gelfand triple and all inclusions are dense and compact. Amongst other equivalent definitions of \(H^{\sigma }({{\varGamma }_0})\) are for example interpolation techniques. All these definitions provide the same space of functions but different norms, where norm equivalence constants depend only on \({{\varGamma }_0}\); see, e.g., the monographs [33, 34] and the references therein. Throughout our proofs, we shall use the Sobolev–Slobodeckij norm (2.2), since it is numerically computable.
2.3 Weakly-singular integral equation
It is known [33, 34] that the weakly-singular integral operator \(V:\widetilde{H}^{-1/2}({\varGamma })\rightarrow H^{1/2}({\varGamma })\) from (1.1) is a symmetric and elliptic isomorphism if \(\mathrm{diam}({\varOmega })<1\) which can always be achieved by scaling. For a given right-hand side \(f\in H^{1/2}({\varGamma })\), the strong form (1.1) is thus equivalently stated by
and the left-hand side defines an equivalent scalar product on \(\widetilde{H}^{-1/2}({\varGamma })\). In particular, the Lax–Milgram lemma proves existence and uniqueness of the solution \(\phi \in \widetilde{H}^{-1/2}({\varGamma })\). Additionally, \(V:L^2({\varGamma })\rightarrow H^1({\varGamma })\) is well-defined, linear, and continuous.
In the Galerkin boundary element method, the test space \(\widetilde{H}^{-1/2}({\varGamma }_{})\) is replaced by some discrete subspace \(\mathcal {X}_{\star }\subset {L^{2}({\varGamma }_{})}\subset \widetilde{H}^{-1/2}({\varGamma }_{})\). Again, the Lax–Milgram lemma guarantees existence and uniqueness of the solution \({\varPhi }_\star \in \mathcal {X}_\star \) of the discrete variational formulation
Below, we shall assume that \(\mathcal {X}_\star \) is linked to a partition \(\mathcal {T}_\star \) of \({\varGamma }\) into a set of connected segments.
2.4 Boundary parametrization
Let \({\varGamma } = \bigcup _i{\varGamma }_i\) be decomposed into its finitely many connected components \({\varGamma }_i\). Since the \({\varGamma }_i\) are compact and piecewise smooth as well, it holds
see, e.g., [25, Section 2.2]. The usual piecewise polynomial and NURBS basis functions have connected support and are hence supported by some single \({\varGamma }_i\) each. Without loss of generality and for the ease of presentation, we may therefore assume throughout that \({\varGamma }\) is connected. All results of this work remain valid for non-connected \({\varGamma }\).
We assume that either \({\varGamma }=\partial {\varOmega }\) is parametrized by a closed continuous and piecewise two times continuously differentiable path \(\gamma :[a,b]\rightarrow {\varGamma }\) such that the restriction \(\gamma |_{[a,b)}\) is even bijective, or that \({\varGamma }\subsetneqq \partial {\varOmega }\) is parametrized by a bijective continuous and piecewise two times continuously differentiable path \(\gamma :[a,b]\rightarrow {\varGamma }\). In the first case, we speak of closed \({\varGamma }=\partial {\varOmega }\), whereas the second case is referred to as open \({\varGamma }\subsetneqq \partial {\varOmega }\).
For closed \({\varGamma }=\partial {\varOmega }\), we denote the \((b-a)\)-periodic extension to \(\mathbb {R}\) also by \(\gamma \). For the left and right derivative of \(\gamma \), we assume that \(\gamma ^{\prime _\ell }(t)\ne 0\) for \(t\in (a,b]\) and \(\gamma ^{\prime _r}(t)\ne 0\) for \(t\in [a,b)\). Moreover we assume that \(\gamma ^{\prime _\ell }(t) +c\gamma ^{\prime _r}(t)\ne 0\) for all \(c>0\) and \(t\in [a,b]\) resp. \(t\in (a,b)\). Finally, let \(\gamma _L:[0,L]\rightarrow {\varGamma }\) denote the arclength parametrization, i.e., \(|\gamma _L^{\prime _\ell }(t)| = 1 = |\gamma _L^{\prime _r}(t)|\), and its periodic extension. Elementary differential geometry yields bi-Lipschitz continuity
where \(C_{{\varGamma }}>0\) depends only on \({\varGamma }\). A proof is given in [28, Lemma 2.1] for closed \({\varGamma }=\partial {\varOmega }\). For open \({\varGamma }\subsetneqq \partial {\varOmega }\), the proof is even simpler.
Let \(I\subseteq [a,b]\). If \({\varGamma }=\partial {\varOmega }\) is closed and \(|I|\le \frac{3}{4} L\) resp. if \({\varGamma }\subsetneqq \partial {\varOmega }\) is open, the bi-Lipschitz continuity (2.7) implies
2.5 Boundary discretization
In the following, we describe the different quantities which define the discretization.
Nodes \(\varvec{z_j=\gamma (\check{z}_j)\in \mathcal {N}_\star }.\) Let \(\mathcal {N}_\star :=\big \{z_j\,:\,j=1,\dots ,n\big \}\) and \(z_0:=z_n\) for closed \({\varGamma }=\partial {\varOmega }\) resp. \(\mathcal {N}_\star :=\big \{z_j\,:\,j=0,\dots ,n\big \}\) for open \({\varGamma }\subsetneqq \partial {\varOmega }\) be a set of nodes. We suppose that \(z_j=\gamma (\check{z}_j)\) for some \(\check{z}_j\in [a,b]\) with \(a=\check{z}_0<\check{z}_1<\check{z}_2<\dots <\check{z}_n=b\) such that \(\gamma |_{[\check{z}_{j-1},\check{z}_j]}\in C^2([\check{z}_{j-1},\check{z}_j])\).
Multiplicity \(\varvec{\#z_j}\) and knots \(\varvec{\mathcal {K}_\star },\varvec{\check{\mathcal {K}}_\star }.\) Let \(p\in \mathbb {N}_0\) be some fixed polynomial order. Each node \(z_j\) has a multiplicity \(\#z_j\in \{1,2\dots , p+1\}\) with \(\#{z}_{0}=\#z_{n}=p+1\). This induces knots
with \(k=1\) resp. \(k=0\) and corresponding knots \(\check{\mathcal {K}}_\star :=\gamma |_{(a,b]}^{-1}(\mathcal {K}_\star )\) resp. \(\check{\mathcal {K}}_\star :=\gamma ^{-1}(\mathcal {K}_\star )\) on the parameter domain [a, b].
Elements, partition \(\varvec{\mathcal {T}_\star },\) and \(\varvec{[T]},\) \(\varvec{[\mathcal {T}_\star }].\) Let \(\mathcal {T}_\star =\{T_1,\dots ,T_n\}\) be a partition of \({\varGamma }\) into compact and connected segments \(T_j=\gamma (\check{T}_j)\) with \(\check{T}_j=[\check{z}_{j-1},\check{z}_j]\). We define
where \(z_{T,1}=z_{j-1}\) and \(z_{T,2}=z_j\) are the two nodes of \(T=T_j\).
Local mesh-sizes \(\varvec{h_{\star ,T}},\) \(\varvec{\check{h}_{\star ,T}}\) and \(\varvec{h_\star },\) \(\varvec{\check{h}_\star }.\) The arclength of each element \(T\in \mathcal {T}_\star \) is denoted by \(h_{\star ,T}\). We define the local mesh-width function \(h_\star \in L^\infty ({\varGamma })\) by \(h_\star |_T=h_{\star ,T}\). Additionally, we define for each element \(T\in \mathcal {T}_\star \) its length \(\check{h}_{\star ,T}:=|\gamma ^{-1}(T)|\) with respect to the parameter domain [a, b]. This gives rise to \(\check{h}_\star \in L^\infty ({\varGamma })\) with \(\check{h}_\star |_T=\check{h}_{\star ,T}\). Note that the lengths \(h_{\star ,T}\) and \(\check{h}_{\star ,T}\) of an element T are equivalent, where the equivalence constants depend only on \(\gamma \).
Local mesh-ratios \( \varvec{\check{\kappa }_\star }.\) We define the local mesh-ratio by
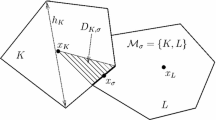
Patches \(\varvec{\omega _\star (z)},\) \(\varvec{\omega _\star (U)},\) \(\varvec{\omega _\star ({\mathcal {U}})},\) and \(\varvec{\bigcup \mathcal U}.\) For each set \(U\subseteq {\varGamma }\), we inductively define for \(m\in \mathbb {N}_0\) (Fig. 1)
For nodes \(z\in {\varGamma }\), we abbreviate \(\omega _\star (z)=:\omega _\star (\{z\}).\) For each set \({\mathcal {U}}\subseteq [\mathcal {T}_\star ]\), we define
and
2.6 Mesh-refinement
Suppose that we are given a deterministic mesh-refinement strategy \(\mathtt{ref}(\cdot )\) such that, for each mesh \([\mathcal {T}_{\star }]\) and an arbitrary set of marked nodes \(\mathcal M_\star \subseteq \mathcal {N}_\star \), the application \([\mathcal {T}_{+}] := \mathtt{ref}([\mathcal {T}_\star ],\mathcal M_\star )\) provides a mesh in the sense of Sect. 2.5 such that, first, the marked nodes belong to the union of the refined elements, i.e., \(\mathcal M_\star \subset \bigcup ([\mathcal {T}_\star ]{\setminus } [\mathcal {T}_{+}])\), and, second, the knots \(\mathcal {K}_\star \) form a subsequence of the knots \(\mathcal {K}_{+}\). The latter implies the estimate
since \([\mathcal {T}_\star ]{\setminus }[\mathcal {T}_+]\) is the set of all elements in which a new knot is inserted and one new knot can be inserted in at most 2 elements of the old mesh, i.e., at the intersection of 2 elements.
We write \([\mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ])\), if there exist finitely many meshes \([\mathcal {T}_1],\dots ,[\mathcal {T}_\ell ]\) and subsets \(\mathcal M_{j}\subseteq \mathcal {N}_{j}\) of the corresponding nodes such that \([\mathcal {T}_\star ] = [\mathcal {T}_1]\), \([\mathcal {T}_{+}]=[\mathcal {T}_\ell ]\), and \([\mathcal {T}_{j}] = \mathtt{ref}([\mathcal {T}_{j-1}],\mathcal M_{j-1})\) for all \(j=2,\dots ,\ell \), where we formally allow \(m=1\), i.e., \([\mathcal {T}_\star ]=[\mathcal {T}_{1}]\in \mathtt{ref}([\mathcal {T}_\star ])\).
For the proof of our main result, we need the following assumptions on \(\mathtt{ref}(\cdot )\).
Assumption 2.1
For an arbitrary initial mesh \([\mathcal {T}_0]\) and \([\mathbb {T}]:=\mathtt{ref}([\mathcal {T}_0])\), we assume that the mesh-refinement strategy satisfies the properties (M1)–(M3):
-
(M1)
There exists a constant \(\check{\kappa }_{\max }\ge 1\) such that the local mesh-ratios (2.11) are uniformly bounded
$$\begin{aligned} \check{\kappa }_\star \le \check{\kappa }_{\max }\quad \text {for all }[\mathcal {T}_\star ]\in [\mathbb {T}]. \end{aligned}$$(2.13) -
(M2)
For all \([\mathcal {T}_\star ],[\mathcal {T}_{+}]\in [\mathbb {T}]\), there is a common refinement \([\mathcal {T}_\star \oplus \mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ]){\cap {\mathtt{ref}}}([\mathcal {T}_{+}])\) such that the knots \(\mathcal {K}_\star \oplus \mathcal {K}_{+}\) of \([\mathcal {T}_\star \oplus \mathcal {T}_{+}]\) satisfy the overlay estimate
$$\begin{aligned} |\mathcal {K}_\star \oplus \mathcal {K}_{+}|\le |\mathcal {K}_\star |+|\mathcal {K}_{+}|-|\mathcal {K}_0|. \end{aligned}$$(2.14) -
(M3)
Each sequence \([\mathcal {T}_\ell ]\in [\mathbb {T}]\) of meshes generated by successive mesh-refinement, i.e., \([\mathcal {T}_j] = \mathtt{ref}([\mathcal {T}_{j-1}],\mathcal M_{j-1})\) for all \(j \in \mathbb {N}\) and arbitrary \(\mathcal M_j \subseteq \mathcal {N}_j,\) satisfies
$$\begin{aligned} |\mathcal {K}_\ell |-|\mathcal {K}_0|\le C_\mathrm{mesh}\sum _{j=0}^{\ell -1} |\mathcal M_j|\quad \text {for }\ell \in \mathbb {N}, \end{aligned}$$(2.15)where \(C_\mathrm{mesh}>0\) depends only on \([\mathcal {T}_0]\).
These assumptions are especially satisfied for pure h-refinement based on local bisection [1] as well as for the concrete strategy used in [24, 25]. The latter strategy looks as follows: Let \([\mathcal {T}_\star ]\in [\mathbb {T}]\). Let \(\mathcal M_\star \subseteq \mathcal {N}_\star \) be a set of marked nodes. To get the refined mesh \([\mathcal {T}_{+}]:=\mathtt{ref}([\mathcal {T}_\star ],\mathcal M_\star )\), we proceed as follows:
-
(i)
If both nodes of an element \(T\in \mathcal {T}_\star \) belong to \(\mathcal M_\star \), the element T will be marked.
-
(ii)
For all other nodes in \(\mathcal M_\star \), the multiplicity will be increased if it is less or equal to \(p+1\), otherwise the elements which contain one of these nodes \(z\in \mathcal M_\star \), will be marked.
-
(iii)
Recursively, mark further elements \(T'\in \mathcal {T}_\star \) for refinement if there exists a marked element \(T\in \mathcal {T}_\star \) with \(T\cap T'\ne \emptyset \) and \(\check{h}_{\star ,T'}>\check{\kappa }_0 \check{h}_{\star ,T}\).
-
(iv)
Refine all marked elements \(T\in \mathcal {T}_\star \) by bisection and hence obtain \([\mathcal {T}_+]\).
According to [1], the proposed recursion in step (iii) terminates and the generated partition \(\mathcal {T}_+\) guarantees (M1) with \(\check{\kappa }_{\max }=2\check{\kappa }_0\). The following proposition shows that also the assumptions (M2)–(M3) are satisfied.
Proposition 2.2
The proposed refinement strategy \(\mathtt{ref}(\cdot )\) used in [24, 25] satisfies Assumption 2.1, where \(\check{\kappa }_{\max }=2\check{\kappa }_0\) and \(C_\mathrm{mesh}\) depends only on the initial partition of the parameter domain, i.e., \(\mathcal {T}_0\) transformed onto [a, b].
Proof
For any partition \(\mathcal {T}_\star \) of \({\varGamma }\) and any subset of marked elements \(\mathcal {S}_\star \subseteq \mathcal {T}_\star \), let \(\widetilde{\mathtt{ref}}(\mathcal {T}_\star ,\mathcal {S}_\star )\) be the partition obtained from the recursive bisection in step (iii)–(iv) above. This local h-refinement procedure has been analyzed in [1]. According to [1, Theorem 2.3], the recursion is well-defined and guarantees \(\check{\kappa }_\star \le 2\check{\kappa }_0\) for all \(\mathcal {T}_\star \in \widetilde{\mathtt{ref}}(\mathcal {T}_0)\).
To see (M2), [1, Theorem 2.3] guarantees the existence of some coarsest common refinement \(\mathcal {T}_\star \widetilde{\oplus }\mathcal {T}_+\in \widetilde{\mathtt{ref}}(\mathcal {T}_\star )\cap \widetilde{\mathtt{ref}}(\mathcal {T}_+)\) such that
The corresponding nodes just satisfy \(\mathcal {N}_\star \oplus \mathcal {N}_{+}=\mathcal {N}_\star \cup \mathcal {N}_{+}\). There exists a finite sequence of meshes \(\mathcal {T}_\star ={\widetilde{\mathcal {T}}}_1,{\widetilde{\mathcal {T}}}_2={\widetilde{\mathtt{ref}}} ({\widetilde{\mathcal {T}}}_1,\mathcal {S}_1),\dots ,{\widetilde{\mathcal {T}}}_\ell ={\widetilde{\mathtt{ref}}} ({\widetilde{\mathcal {T}}}_{\ell -1},\mathcal {S}_{\ell -1})=\mathcal {T}_\star \widetilde{\oplus }\mathcal {T}_{+}\) with suitable \(\mathcal {S}_j\subseteq \mathcal {T}_j\) for \(j=1,\dots ,\ell -1\). If we define \(\mathcal M_j\subseteq \mathcal {N}_j\) as the set of all nodes in \(\mathcal {S}_j\), we see that the sequence \([\mathcal {T}_\star ]=[\mathcal {T}_1],[\mathcal {T}_2]=\mathtt{ref}([\mathcal {T}_1],\mathcal M_1),\dots [\mathcal {T}_\ell ]= \mathtt{ref}([\mathcal {T}_{\ell -1},\mathcal M_{\ell -1})\) satisfies \(\mathcal {T}_j={\widetilde{\mathcal {T}}}_j\) for \(j=1,\dots \ell \). By repetitively marking one single node, we obtain from \([\mathcal {T}_\ell ]\) a mesh \([\mathcal {T}_\star \oplus \mathcal {T}_+]\) with nodes \(\mathcal {N}_\star \oplus \mathcal {N}_+=\mathcal {N}_\star \cup \mathcal {N}_+\) and \(\# z=\max (\#_\star z,\#_+z)\), where \(\#_\star \) resp. \(\#_+\) denote the multiplicity in \(\mathcal {K}_\star \) resp. \(\mathcal {K}_+\) and, e.g., \(\#_+ z:=0\) if \(z\in \mathcal {N}_\star {\setminus }\mathcal {N}_+\). There obviously holds
Moreover, \([\mathcal {T}_\star \oplus \mathcal {T}_+]\) is clearly a refinement of \([\mathcal {T}_+]\) as well.
Finally we consider (M3). As before we have \(\mathcal {T}_1={\widetilde{\mathtt{ref}}}(\mathcal {T}_0,\mathcal {S}_0),\dots ,\) \(\mathcal {T}_\ell ={\widetilde{\mathtt{ref}}}(\mathcal {T}_{\ell -1},\mathcal {S}_{\ell -1})\) for suitable \(\mathcal {S}_{j}\subseteq \mathcal {T}_j\), \(j=0,\dots ,\ell -1\). Note that there holds \(|\mathcal {S}_j|\le 2|\mathcal M_j|\). We denote \(|\#_j|:=|\mathcal {K}_{j+1}|-|\mathcal {K}_{j}|-(|\mathcal {N}_{j+1}|-|\mathcal {N}_j|)\) as the number of multiplicity increases during the j-th refinement. There holds
and hence
The term \(|\mathcal {T}_\ell |-|\mathcal {T}_0|\) can be estimated by \(C\sum _{j=0}^{\ell -1} |\mathcal {S}_j|\) with some constant \(C>0\) which depends only on the initial partition of the parameter domain, see [1, Theorem 2.3], and hence by \(2C\sum _{j=0}^{\ell -1}|\mathcal M_j|\). The estimate \(|\#_j|\le |\mathcal M_j|\) concludes the proof with \(C_\mathrm{mesh}=2C+1\). \(\square \)
2.7 B-splines and NURBS
Throughout this subsection, we consider knots \(\check{\mathcal {K}}:=(t_i)_{i\in \mathbb {Z}}\) on \(\mathbb {R}\) with multiplicity \(\#t_i\) which satisfy \(t_{i-1}\le t_{i}\) for \(i\in \mathbb {Z}\) and \(\lim _{i\rightarrow \pm \infty }t_i=\pm \infty \). Let \(\check{\mathcal {N}}:=\big \{t_i\,:\,i\in \mathbb {Z}\big \}=\big \{\check{{z}}_{j}\,:\,j\in \mathbb {Z}\big \}\) denote the corresponding set of nodes with \(\check{{z}}_{j-1}<\check{{z}}_{j}\) for \(j\in \mathbb {Z}\). For \(i\in \mathbb {Z}\), the i-th B-spline of degree p is defined inductively by (Fig. 2)
where, for \(t\in \mathbb {R}\),
We also use the notations \(B_{i,p}^{\check{\mathcal {K}}}:=B_{i,p}\) and \(\beta _{i,p}^{\check{\mathcal {K}}}:=\beta _{i,p}\) to stress the dependence on the knots \(\check{\mathcal {K}}\). The following lemma collects some basic properties of B-splines.
Lemma 2.3
Let \(I=[a,b)\) be a finite interval and \(p\in \mathbb {N}_0\). Then, the following assertions (i)–(vi) hold :
-
(i)
The set \(\big \{B_{i,p}|_I\,:\,i\in \mathbb {Z}, B_{i,p}|_I\ne 0\big \}\) is a basis for the space of all right-continuous \(\check{\mathcal {N}}\)-piecewise polynomials of degree lower or equal p on I which are, at each knot \(t_i,\) \(p-\#t_i\) times continuously differentiable if \(p-\#t_i\ge 0\).
-
(ii)
For \(i\in \mathbb {Z},\) \(B_{i,p}\) vanishes outside the interval \([t_{i-1},t_{i+p})\). It is positive on the open interval \((t_{i-1},t_{i+p})\).
-
(iii)
For \(i\in \mathbb {Z},\) \(B_{i,p}\) is completely determined by the \(p+2\) knots \(t_{i-1},\dots ,t_{i+p}\).
-
(iv)
The B-splines of degree p form a (locally finite) partition of unity, i.e.,
$$\begin{aligned} \sum _{i \in \mathbb {Z}} B_{i,p}=1\quad \text {on }\mathbb {R}. \end{aligned}$$(2.17)
Proof
The proof of (i) is found in [14, Theorem 6], and (ii)–(iii) are proved in [14, Section 2]. (iv) is proved in [14, page 9–10]. \(\square \)
In addition to the knots \(\check{\mathcal {K}}=(t_i)_{i\in \mathbb {Z}}\), we consider positive weights \(\mathcal {W}:=(w_i)_{i\in \mathbb {Z}}\) with \(w_i>0\). For \(i\in \mathbb {Z}\) and \(p\in \mathbb {N}_0\), we define the i-th NURBS by
We also use the notation \(R_{i,p}^{\check{\mathcal {K}},\mathcal {W}}:=R_{i,p}\). Note that the denominator is locally finite and positive.
For any \(p\in \mathbb {N}_0\), we define the B-spline space
as well as the NURBS space
2.8 Ansatz spaces
Let \([\mathcal {T}_0]\) be a given initial mesh with corresponding knots \(\mathcal {K}_0\) such that \(h_0 \le |{\varGamma }|/4\) for closed \({\varGamma }=\partial {\varOmega }\). We set \([\mathbb {T}]:=\mathtt{ref}([\mathcal {T}_0])\). Suppose that \(\mathcal {W}_0=(w_{i})_{i=1-p}^{N-p}\) are given initial weights with \(N=|\mathcal {K}_0|\) for closed \({\varGamma }=\partial {\varOmega }\) resp. \(N=|\mathcal {K}_0|-(p+1)\) for open \({\varGamma }\subsetneqq \partial {\varOmega }\).
If \({\varGamma }=\partial {\varOmega }\) is closed, we extend the transformed knot sequence \(\check{\mathcal {K}}_0=(t_i)_{i=1}^N\) arbitrarily to \((t_i)_{i\in \mathbb {Z}}\) with \(t_{-p}=\dots =t_0=a\), \(t_i\le t_{i+1}\), \(\lim _{i\rightarrow \pm \infty }t_i=\pm \infty \) and \(\mathcal {W}_0=(w_i)_{i\in \mathbb {Z}}\) with \(w_i>0\). For the extended sequences, we also write \(\check{\mathcal {K}}_0\) and \(\mathcal {W}_0\) and set
If \({\varGamma }_{}\subsetneqq \partial {\varOmega }\) is open, we extend the sequences \(\check{\mathcal {K}}_0=(t_i)_{i=-p}^N\) and \(\mathcal {W}_0\) arbitrarily to \((t_i)_{i\in \mathbb {Z}}\) with \(t_i\le t_{i+1}\), \(\lim _{i\rightarrow \pm \infty }t_i=\pm \infty \) and \(\mathcal {W}_0=(w_i)_{i\in \mathbb {Z}}\) with \(w_i>0\). This allows to define
Due to Lemma 2.3, this definition does not depend on how the sequences are extended.
Let \([\mathcal {T}_\star ]\in [\mathbb {T}]\) be a mesh with knots \(\mathcal {K}_\star \). Via knot insertion from \(\mathcal {K}_0\) to \(\mathcal {K}_\star \), one obtains unique corresponding weights \(\mathcal {W}_\star \). These are chosen such that the denominators of the NURBS functions do not change. In particular, this implies nestedness
where the spaces \(\mathcal {X}_\star \) resp. \(\mathcal {X}_+\) are defined analogously to (2.21)–(2.22). Moreover, the weights are just convex combinations of \(\mathcal {W}_0\), wherefore
For further details, we refer to, e.g., [25, Section 4.2].
3 Adaptive algorithm and main results
For each mesh \([\mathcal {T}_\star ]\in [\mathbb {T}]\), define the node-based error estimator
where the refinement indicators read
Here, we must additionally suppose \(f\in H^{1}({\varGamma })\) to ensure that \(\mu _\star \) is well-defined. It has been proved in [24] that \(\mu _\star \) is reliable, i.e.,
where \(C_\mathrm{rel}>0\) depends only on p, \(w_\mathrm{min}\), \(w_\mathrm{max}\), \(\gamma \), and \(\check{\kappa }_\mathrm{max}\). We note that the weighted-residual error estimator in the form \(\mu _\star \simeq \Vert h_\star ^{1/2}\partial _{\varGamma }(f-V{\varPhi }_\star )\Vert _{L^2({\varGamma })}\) goes back to the works [6, 13], where reliability (3.2) is proved for standard 2D BEM with piecewise constants on polyhedral geometries, while the corresponding result for 3D BEM is found in [12]. We consider the following adaptive algorithm which employs the Dörfler marking strategy (3.3) from [17] to single out nodes for refinement.
Algorithm 3.1
Input: Adaptivity parameter \(0<\theta <1\), \(C_\mathrm{mark}\ge 1\), polynomial order \(p\in \mathbb {N}_0\), initial mesh \([\mathcal {T}_0]\), initial weights \(\mathcal {W}_0\).
Adaptive loop: For each \(\ell =0,1,2,\dots \) iterate the following steps (i)–(iv):
-
(i)
Compute discrete approximation \({\varPhi }_\ell \in \mathcal {X}_\ell \) from Galerkin BEM.
-
(ii)
Compute refinement indicators \(\mu _\ell ({z})\) for all nodes \({z}\in \mathcal {N}_\ell \).
-
(iii)
Determine an up to the multiplicative constant \(C_\mathrm{mark}\) minimal set of nodes \(\mathcal M_\ell \subseteq \mathcal {N}_\ell \) such that
$$\begin{aligned} \theta \,\mu _\ell ^2 \le \sum _{{z}\in \mathcal M_\ell }\mu _\ell ({z})^2. \end{aligned}$$(3.3) -
(iv)
Generate refined mesh \([\mathcal {T}_{\ell +1}]:=\mathtt{ref}([\mathcal {T}_\ell ],\mathcal M_\ell )\).
Output: Approximate solutions \({\varPhi }_\ell \) and error estimators \(\mu _\ell \) for all \(\ell \in \mathbb {N}_0\).
Our main result is that the proposed algorithm is linearly convergent, even with the optimal algebraic rate. For a precise statement of this assertion, let \([\mathbb {T}_N]:=\big \{[\mathcal {T}_\star ]\in [\mathbb {T}]\,:\,|\mathcal {K}_\star |-|\mathcal {K}_0|\le N\big \}\) be the finite set of all refinements having at most N knots more than \([\mathcal {T}_0]\). Following [8], we introduce an estimator-based approximation class \(\mathbb {A}_s\) for \(s>0\): We write \(\phi \in \mathbb {A}_s\) if
In explicit terms, this just means that an algebraic convergence rate of \(\mathcal {O}(N^{-s})\) for the estimator is possible, if the optimal meshes are chosen. The following theorem is the main result of our work:
Theorem 3.2
Let \(f\in H^1({\varGamma }),\) so that the weighted-residual error estimator \(\mu _\ell \) from (3.1) is well-defined and that Algorithm 3.1 is driven by \(\mu _\ell .\) We suppose that the Assumption 2.1 on the mesh-refinement holds true. Then, for each \(0<\theta \le 1,\) there exist constants \(0<q_\mathrm{lin}<1\) and \(C_\mathrm{lin}>0\) such that Algorithm 3.1 is linearly convergent in the sense of
In particular, this implies convergence
Moreover, there is a constant \(0<\theta _\mathrm{opt}<1\) such that for all \(0<\theta <\theta _\mathrm{opt},\) there exists a constant \(C_\mathrm{opt}>0\) such that, for all \(s>0,\) it holds
The constants \(q_\mathrm{lin}, C_\mathrm{lin}\) depend only on \(p, w_{\min },w_{\max }, \gamma , \theta ,\) and \(\check{\kappa }_{\max }\) from (M1). The constant \(\theta _\mathrm{opt}\) depends only on \(p, w_{\min },w_{\max }, \gamma ,\) and (M1)–(M3), and \(C_\mathrm{opt}\) depends additionally on \(\theta \).
Remark 3.3
The proof of Theorem 3.2 reveals that linear convergence (3.5) only requires (M1), while optimal rates (3.7) rely on (3.5) and (M2)–(M3). Provided that there exists a constant \(C_\mathrm{son} > 0\) such that \(|\mathcal {K}_\star |\le C_\mathrm{son}|\mathtt{ref}([\mathcal {T}_\star ],\mathcal M_\star )|\) for all \([\mathcal {T}_\star ]\in [\mathbb {T}]\) and \(\mathcal M_\star \subseteq \mathcal {N}_\star \), also the converse implication in (3.7) holds true. The proof follows along the lines of [8, Proposition 4.15] and thus is left to the reader.
The proof of Theorem 3.2 is given in Sects. 4 and 5. The ideas essentially follow those of [8], where an axiomatic approach of adaptivity for abstract problems is found. We note, however, that [8] only considers h-refinement, while the present formulation of Algorithm 3.1 steers both, the h-refinement and the knot multiplicity increase.
If Algorithm 3.1 is steered by the Faermann estimator
with the refinement indicators
instead of \(\mu _\star \), we can prove at least plain convergence of the estimator to zero. In contrast to the weighted-residual estimator which requires additional regularity \(f\in H^1({\varGamma })\), the Faermann estimator \(\eta _\star \) allows a right-hand side \(f\in H^{1/2}({\varGamma })\). Moreover, \(\eta _\star \) estimator is efficient and reliable
where \(C_\mathrm{eff}>0\) depends only on \({\varGamma }\), while \(C_\mathrm{rel}>0\) depends additionally on \(p, \check{\kappa }_{\max }, w_{\min }, w_{\max }\) and \(\gamma \); see [25, Theorem 3.1 and 4.4]. This equivalence of error and estimator puts some interest on the following convergence theorem which is, however, weaker than the statement of Theorem 3.2.
Theorem 3.4
Let \(f\in H^{1/2}({\varGamma })\). We suppose that (M1) from Assumption 2.1 for the mesh-refinement holds. Then, for each \(0<\theta \le 1,\) Algorithm 3.1 steered by the Faermann estimator (3.8) is convergent in the sense of
According to (3.9), this is equivalent to
Remark 3.5
The statements of Theorems 3.2 and 3.4 remain valid, if only adaptive h-refinement is used, i.e., if Algorithm 3.1 does not steer the knot multiplicity.
4 Proof of Theorem 3.2, linear convergence (3.5)
As an auxiliary result, we need an inverse-type estimate for NURBS with respect to the fractional \(\widetilde{H}^{-1/2}({\varGamma })\)-norm. In the following, a result is stated and proved for the \(\widetilde{H}^{-\sigma }({\varGamma })\)-norm, where \(0<\sigma <1\). For piecewise polynomials, an analogous result is already found in [30, Theorem 3.6] resp. [29, Theorem 3.9]. Our proof is inspired by [15, Section 4.3], where a similar result is found for piecewise constant functions as well as for piecewise affine and globally continuous functions in 1D. For integer-order Sobolev norms, inverse estimates for NURBS are found in [3, Section 4], and (4.2) is proved in [2, Theorem 3.1] for piecewise polynomials.
Proposition 4.1
Let \([\mathcal {T}_\star ]\in [\mathbb {T}]\) and \(0<\sigma <1\). Then, there is a constant \(C_\mathrm{inv}>0\) such that
For \(\sigma =1/2,\) it holds
The constant \(C_\mathrm{inv}\) only depends on \(\check{\kappa }_{\max }, p, w_{\min }, w_{\max },\) \(\gamma ,\) and \(\sigma \).
Proof
The proof is done in four steps. First, we show that \(\Vert h_\star ^{\sigma }\psi \Vert _{L^2({\varGamma })}\lesssim \Vert \psi \Vert _{\widetilde{H}^{-\sigma }({\varGamma })}\) holds for all \(\psi \in L^2({\varGamma })\) which satisfy a certain assumption. In the second step, we prove an auxiliary result for polynomials which is needed in the third one, where we show that all \(\psi \in \mathcal {X}_\star \) satisfy the mentioned assumption. In the last step, we apply a recent result of [2], which then concludes the proof.
Step 1 Let \(\mathcal {X}\subset L^2({\varGamma })\) satisfy the following assumption: There exists a constant \(q \in (0,1)\) such that for all \(T\in \mathcal {T}_\star \) and all \(\psi \in \mathcal {X}\) there exists some connected subset \({\varDelta }(T,\psi ) \subseteq T\) of length \(|{\varDelta }(T,\psi )|\ge q|T|\) such that \(\psi \) does not change its sign on \({\varDelta }(T,\psi )\) and
Then, there exists a constant \(C>0\) which depends only on q and \(\check{\kappa }_\star \), such that
For a compact nonempty interval \([c,d]= I \subseteq [a,b]\), we define the bubble function
It obviously satisfies \(0\le P_I\le 1\) and \(\mathrm{supp}P_I=I\). A standard scaling argument proves
and
with generic constants \(C_1,C_2,C_3>0\) which do not depend on I. For each \(T\in \mathcal {T}_\star \), let \(I(T,\psi )\) be some interval with \(\gamma (I(T,\psi ))={\varDelta }(T,\psi )\). With the arclength parametrization \(\gamma _L\), we define, for all \(T\in \mathcal {T}_\star \), the functions \(P_{{\varDelta }(T,\psi )}:=P_{I(T,\psi )}\circ \gamma _L\) and the coefficients
Note that (4.4) and (4.5) hold for \(P_{{\varDelta }(T,\psi )}\) with I simply replaced by \({\varDelta }(T,\psi )\) and with \((\cdot )'\) replaced by the arclength derivative \(\partial _{\varGamma }\). By definition of the dual norm, it holds
First, we estimate the numerator in (4.7):
It remains to estimate the denominator in (4.7): We first note that it holds \(|u|_{H^{\sigma }(I)}^2\lesssim |I|^{1-\sigma }\Vert u'\Vert _{L^2(I)}\) for any interval \(I\subset \mathbb {R}\) of finite length and \(u\in H^1(I)\). This is already stated in [7, Lemma 7.4]. However, a detailed proof is given only for \(1/2<\sigma <1\). For \(0<\sigma \le 1/2\) this inequality can be shown exactly as in the proof of [24, Lemma 4.5], where only \(\sigma =1/2\) is considered. This, together with (2.8), implies for any connected \(\omega \subseteq {\varGamma }\) with \(|\omega |\le \frac{3}{4}|{\varGamma }|\) that
The hidden constant in (4.8) depends only on \(\sigma \) and \({\varGamma }\). Equation (4.8) is applicable for any node patch \(\omega _\star (z)\) since we assumed in Sect. 2.8 that \(h_0\le |{\varGamma }|/4\) if \({\varGamma }=\partial {\varOmega }\) With [18, Lemma 2.3], we hence see
This yields
where the hidden constant depends only on \(\check{\kappa }_{\max }, \sigma \), and \(\gamma \). With
we finish the first step.
Step 2 For some fixed polynomial degree \(p\in \mathbb {N}_0\), there exists a constant \(q_1\in (0,1)\) such that for all polynomials F of degree p on [0, 1] there exists some interval \(I\subseteq [0,1]\) of length \(|I|\ge q_1\) with
Instead of considering general polynomials \(\mathcal {P}^p([0,1])\) of degree p, it is sufficient to consider the following subset
Note that \(\mathcal {M}\) is a compact subset of \(L^{\infty }([0,1])\) and that differentiation \((\cdot )'\) is a continuous mapping on \(\mathcal {M}\) due to finite dimension. This especially implies boundedness \(\sup _{F\in \mathcal {M}} \Vert F'\Vert _{\infty }\le C_4<\infty \). We may assume \(C_4>2\). For given \(F\in \mathcal {M}\), we define the interval I as follows: Without loss of generality, we assume that the maximum of |F| is attained at some \(t_1\in [0,1/2]\) and that \(F(t_1)=1\). We set \(t_3:=t_1+C_4^{-1}\in (t_1,1]\) and \(t_2:=t_1+C_4^{-1}/2\in (t_1,3/4]\) and \(I:=[t_1,t_2]\). Then, \(|I|=1/(2C_4)\) and for all \(t\in I\) it holds
Altogether, we thus have
and conclude this step.
Step 3 We show that \(\mathcal {X}_\star \) satisfies the assumption of Step 1 and hence conclude \(\Vert h_\star ^{\sigma }{\varPsi }_\star \Vert _{L^2({\varGamma })}\lesssim \Vert {\varPsi }_\star \Vert _{\widetilde{H}^{-\sigma }({\varGamma })}\) for all \({\varPsi }_\star \in \mathcal {X}_\star \): Let \(\check{T}\subset [a,b]\) be the interval with \(\gamma (\check{T})=T\) and \(\check{\psi }:=\psi \circ \gamma |_{\check{T}}\). Since \(|I|\simeq |\gamma (I)|\) for any interval \(I\subseteq [a,b]\), where the hidden constants depend only on \(\gamma \), we just have to find a uniform constant \(q_2\in (0,1)\) and some interval \(I\subseteq \check{T}\) of length \(|I|\ge q_2 |\check{T}|\) with
The function \(\check{\psi }\) has the form F / w with a polynomial F of degree p and the weight function w, which is also a polynomial of degree p and which satisfies \(w_{\min }\le w\le w_{\max }\). Hence, (4.10) is especially satisfied if
After scaling to the interval [0, 1], we can apply Step 2 and conclude this step. Altogether, this proves (4.1).
Step 4 According to [2], it holds \(\Vert h_\star ^{1/2}\partial _{\varGamma }(V\psi )\Vert _{L^2({\varGamma })}\lesssim \Vert h_\star ^{1/2}\psi \Vert _{L^2({\varGamma })}+\Vert \psi \Vert _{\widetilde{H}^{-1/2}({\varGamma })}\) for all \(\psi \in L^2({\varGamma })\), where the hidden constant depends only on \({\varGamma }\), \(\gamma \), and \(\check{\kappa }_{\max }\). Together with Step 3, this shows (4.2). \(\square \)
The proof of linear convergence (3.5) will be done with the help of some auxiliary (and purely theoretical) error estimator \({\widetilde{\rho }}_\star \). The latter relies on the following definition of an equivalent mesh-size function which respects the multiplicity of the knots.
Proposition 4.2
Assumption 2.1 (M1) implies the existence of a modified mesh-size function \(\widetilde{h}:[\mathbb {T}]\rightarrow L^\infty ({\varGamma })\) with the following properties : There exists a constant \(C_\mathrm{wt}>0\) and \(0<q_\mathrm{ctr}<1\) which depend only on \(\check{\kappa }_{\max }, p\) and \(\gamma \) such that for all \([\mathcal {T}_\star ]\in [\mathbb {T}]\) and all refinements \([\mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ]),\) the corresponding mesh-sizes \(\widetilde{h}_\star :=\widetilde{h}([\mathcal {T}_\star ])\) and \(\widetilde{h}_{+}:=\widetilde{h}([\mathcal {T}_{+}])\) satisfy equivalence
reduction
as well as contraction on the patch of refined elements
Note that \(\omega _{+}( [\mathcal {T}_{+}]{\setminus }[\mathcal {T}_{\star }])=\omega _{{\star }}( [\mathcal {T}_{\star }]{\setminus }[\mathcal {T}_{+}]),\) which follows from \(\bigcup ([\mathcal {T}_+]{\setminus } [\mathcal {T}_\star ])=\bigcup ([\mathcal {T}_\star ]{\setminus }[\mathcal {T}_+])\) and the fact that the application of \(\omega _{+}\) resp. \(\omega _{\star }\) only adds elements of \(\mathcal {T}_{\star }{\cap {\mathcal {T}}}_{+}\).
Proof
For all \([\mathcal {T}_\star ]\in \mathbb {T}\), we define \(\widetilde{h}_\star \in L^\infty ({\varGamma })\) by
where \(0<q_1<1\) is fixed later. Clearly, \(\widetilde{h}_\star \simeq \check{h}_\star \), where the hidden equivalence constants depend only on \(\check{\kappa }_\star \), p, and \(q_1\). Let \(x\in {\varGamma }\). First, suppose \(x\not \in \omega _{+}([\mathcal {T}_{+}]{\setminus }[\mathcal {T}_\star ])\cup \mathcal {N}_{+}\), i.e., neither the element \([T]\in [\mathcal {T}_{+}]\) containing x nor its neighbors result from h-refinement or from multiplicity increase. Then, \(\widetilde{h}_{+}(x)=\widetilde{h}_\star (x)\). Second, suppose \(x\in \omega _{+}([\mathcal {T}_{+}]{\setminus }[\mathcal {T}_\star ]){\setminus }\mathcal {N}_{+}\), i.e., the element \([T']\in [\mathcal {T}_{+}]\) containing x or one of its neighbors result from h-refinement and/or multiplicity increase. If only multiplicity increase took place, we get
In the other case, consider the father \([T]\in [\mathcal {T}_\star ]\) of \([T']\), i.e., \(T'\subseteq T\). Note that
with a constant \(0<q_2<1\) which depends only on \(\check{\kappa }_{\max }\). Choose \(0<q_1<1\) sufficiently large such that
This choice yields \(\widetilde{h}_{+}(x)\le ({q_2}/q_1^{4p}) \cdot \widetilde{h}_\star (x)\), since \(\mathcal {N}_\star {\cap {\omega }}_\star (T)\) contains at most 4 nodes. Therefore, we conclude the proof with \(q_\mathrm{ctr}:=\max (q_1,{q_2}/q_1^{4p})\). \(\square \)
Remark 4.3
Note that the construction of \(\widetilde{h}_\star \) in Proposition 4.2 even ensures contraction \(\widetilde{h}_{+}|_{\omega _{+}(T)}\le q_\mathrm{ctr}\widetilde{h}_\star |_{\omega _{+}(T)}\) if \({[T]\in [\mathcal {T}_{+}]{\setminus } [\mathcal {T}_\star ]}\) is obtained by h-refinement, while the multiplicity of all nodes \(z\in \mathcal {N}_+{\cap {\omega }}_+(T)\) is arbitrarily chosen \(\#z\in \{1,\dots ,p+1\}\). In explicit terms, this allows for instance to set the multiplicity of all nodes \(z\in \mathcal {N}_+{\cap {\omega }}_+(T)\) to \(\#z:=1\), if T is obtained by h-refinement.\(\square \)
For any \([\mathcal {T}_\star ]\in [\mathbb {T}]\), we define the auxiliary estimator
which employs the novel mesh-size function \(\widetilde{h}_\star \) from Proposition 4.2. Obviously the estimators \(\mu _\star \) and \(\widetilde{\rho }_\star \) are locally equivalent
where the hidden constants depend only on \(\check{\kappa }_{\max }\), p, and \(\gamma \). The proof of the following lemma is inspired by [26, Proposition 3.2] resp. [8, Lemma 8.8], where only h-refinement is considered.
Lemma 4.4
(Estimator reduction of \(\widetilde{\rho })\) Algorithm 3.1 guarantees
The constants \(0<q_\mathrm{est}<1\) and \(C_\mathrm{est}>0\) depend only on \(\check{\kappa }_{\max },p,w_{\min },w_{\max },\gamma ,\) and \(\theta \).
Proof
The proof is done in several steps.
Step 1 With the inverse estimate (4.2), there holds the following stability property for any measurable \({\varGamma }_0\subseteq {\varGamma }\)
with a constant \(C>0\) which depends only on \(C_\mathrm{wt},C_\mathrm{inv}\), and \(\gamma \).
Step 2 With Proposition 4.2, we split the estimator into a contracting and into a non-contracting part
Step 1, the Young inequality, and Proposition 4.2 show, for arbitrary \(\delta >0\), that
Analogously, we get
Combining these estimates, we end up with
Step 3 Local equivalence (4.16) and the Dörfler marking (3.3) for \(\mu _\ell \) imply
where the hidden constants depend only on \(\check{\kappa }_\mathrm{max}\), p, and \(\gamma \). Hence, \(\widetilde{\rho }_\ell \) satisfies some Dörfler marking with a certain parameter \(0<\widetilde{\theta }<1\). With \(\mathcal M_\ell \subseteq \bigcup ([\mathcal {T}_\ell ]{\setminus } [\mathcal {T}_{\ell +1}])\), (4.18) hence becomes
By choosing \(\delta \) sufficiently small, we prove (4.17) with \(C_\mathrm{est}:=2(1+\delta ^{-1})C^2\) and \(q_\mathrm{est}:=(1+\delta )\big (1-(1-q_\mathrm{ctr})\widetilde{\theta }\big )<1\). \(\square \)
Proof of linear convergence (3.5)
Due to the properties of the weakly-singular integral operator V, the bilinear form \(A(\phi ,\psi ):=\langle V\phi \,;\,\psi \rangle _{{\varGamma }}\) defines even a scalar product, and the induced norm \(\Vert \psi \Vert _{V}:=A(\psi ,\psi )^{1/2}\) is an equivalent norm on \(\widetilde{H}^{-1/2}({\varGamma })\). According to nestedness of the ansatz spaces \(\mathcal {X}_{\ell }\subset \mathcal {X}_{\ell +1}\), the Galerkin orthogonality implies the Pythagoras theorem
Together with the estimator reduction (4.17) and reliability (3.2)
this implies the existence of \(0<\kappa ,\lambda <1\), which depend only on \(C_\mathrm{rel},C_\mathrm{est}\) and \(q_\mathrm{est}\), such that \({\varDelta }_\star :=\Vert \phi -{\varPhi }_\star \Vert _{V}^2+\lambda \,{\widetilde{\rho }}_\star ^{\,2} \simeq {\widetilde{\rho }}_\star ^{\,2}\) satisfies
see, e.g., [26, Theorem 4.1], while the original idea goes back to [10]. From this, we infer
and hence conclude the proof. \(\square \)
5 Proof of Theorem 3.2, optimal convergence (3.7)
As in the previous section, we define an auxiliary error estimator. For each \([\mathcal {T}_\star ]\in [\mathbb {T}]\), let
Note that the estimators \(\mu _\star \) and \(\rho _\star \) are again locally equivalent
where the hidden constant depends only on \(\check{\kappa }_{\max }\). Analogous versions of the next two lemmas are already proved in [26, Proposition 4.2 and 4.3] for h-refinement and piecewise constants; see also [8, Propostion 5.7] for discontinuous piecewise polynomials and h-refinement. The proof for Lemma 5.1 is essentially based on Proposition 4.1. The proof of Lemma 5.2 requires the construction of a Scott–Zhang type operator (5.9) which is not necessary in [8, 26], since both works consider discontinuous piecewise polynomials.
Lemma 5.1
(Stability of \(\rho \)) Let \([\mathcal {T}_\star ]\in [\mathbb {T}]\) and \([\mathcal {T}_+]\in \mathtt{ref}(\mathcal {T}_\star )\). For \(\mathcal {S}\subseteq \mathcal {T}_\star \cap \mathcal {T}_+\) there holds
where \(C_\mathrm{stab}>0\) depends only on the parametrization \(\gamma \) and the constant \(C_\mathrm{inv}\) of Proposition 4.1.
Proof
For all subsets \({\varGamma }_0\subseteq {\varGamma }\), it holds
The choice \({\varGamma }_0=\bigcup \mathcal {S}\) shows stability
and we conclude the proof. \(\square \)
Lemma 5.2
(Discrete reliability of \(\rho )\) There exist constants \(C_\mathrm{rel}, C_\mathrm{ref}>0,\) which depend only on \(\check{\kappa }_{\max }, p, w_{\min }, w_{\max },\) and \(\gamma ,\) such that for all refinements \([\mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ])\) of \([\mathcal {T}_\star ]\in [\mathbb {T}]\) there exists a subset \(\mathcal {R}_\star (\mathcal {T}_+)\subseteq \mathcal {T}_\star \) with
as well as
For the proof of Lemma 5.2, we need to introduce a Scott–Zhang type operator. Let \([\mathcal {T}_\star ]\in [\mathbb {T}]\) and \(\big \{R_{i,p}|_{[a,b\rangle }\,:\,i=1-p,\dots ,N-p\big \}\circ \gamma |_{[a,b\rangle }^{-1}\) be the basis of NURBS of \(\mathcal {X}_\star \), where “\(\rangle \)” stands for “)” if \({\varGamma }=\partial {\varOmega }\) is closed and for “]” if \({\varGamma }\subsetneqq \partial {\varOmega }\) is open. Here, N denotes the number of transformed knots \(\check{\mathcal {K}}_\star \) in (a, b]. With the corresponding B-splines there holds \(R_{i,p}=w_iB_{i,p} /w\), where \(w=\sum _{\ell \in \mathbb {Z}} w_\ell B_{\ell ,p}\) is the fixed denominator satisfying \(w_\mathrm{min}\le w\le w_\mathrm{max}\); see Sect. 2.8. In [4, Section 2.1.5], it is shown that, for \(i\in \{1-p,\dots ,N-p\}\), there exist dual basis functions \(B_{i,p}^*\in L^2(\mathrm{supp}B_{i,p})\) with
and
Define \(R_{i,p}^*:=B_{i,p}^* w/w_i\) with the denominator w from before, and \(\widehat{R}_{i,p}:=R_{i,p}|_{[a,b\rangle }\circ \gamma |_{[a,b\rangle }^{-1}\). For \(I\subseteq \{1-p,\dots ,N-p\}\), we define the following Scott–Zhang type operator
In [4, Section 3.1.2], a similar operator is considered for \(I= \{1-p,\dots ,N-p\}\), and [4, Proposition 2.2] proves an analogous version of the following lemma.
Lemma 5.3
The Scott–Zhang type operator (5.9) satisfies the following two properties :
-
(i)
Local projection property : For \(T\in \mathcal {T}_\star \) with \(\big \{i\,:\,T\subseteq \mathrm{supp}\widehat{R}_{i,p}\big \}\subseteq I\) and \(\psi \in L^2({\varGamma }),\) the inclusion \(\psi |_{\omega _\star ^{p}(T)} \in \mathcal {X}_\star |_{\omega _\star ^p(T)}:=\big \{\xi |_{\omega _\star ^p(T)}\,:\,\xi \in \mathcal {X}_\star \big \}\) implies \(\psi |_T=(P_{\star ,I}\psi )|_T\).
-
(ii)
Local \(L^2\)-stability : For \(\psi \in L^2({\varGamma })\) and \(T\in \mathcal {T}_\star ,\) there holds
$$\begin{aligned} \Vert P_{\star ,I}(\psi )\Vert _{L^2(T)}\le C_\mathrm{sz}\Vert \psi \Vert _{L^2(\omega _\star ^{p}(T))}, \end{aligned}$$where \(C_\mathrm{sz}\) depends only on \(\check{\kappa }_{\max }, p, w_{\max }\), and \(\gamma \).
Proof
All NURBS basis functions which are non-zero on T, have support in \(\omega _\star ^p(T)\). With this, (i) follows easily from the definition of \(P_{\star ,I}\). For stability (ii), we use \(0\le \widehat{R}_{i,p}\le 1\) and (5.8) to see
Overall, the hidden constants depend only on \(\check{\kappa }_{\max }, p, w_{\max }\), and \(\gamma \). \(\square \)
Proof of Lemma 5.2
We choose
We prove that
To see this, let \(T\in \mathcal {T}_\star \) with \(T\subseteq \overline{{\varGamma }{\setminus }\omega _\star ^{p}( [\mathcal {T}_\star ]{\setminus } [\mathcal {T}_{+}])}\). Then, \(\big \{i\,:\,T\subseteq \mathrm{supp}\widehat{R}_{i,p}\big \}\subseteq I\). It holds \(\omega _\star ^{p}(T)\subseteq \bigcup ([\mathcal {T}_\star ]\cap [\mathcal {T}_{+}])\). This implies that no new knots are inserted in \(\omega ^p_\star (T)\). With Lemma 2.3(i), it follows \(\mathcal {X}_+|_{\omega _\star ^p(T)}=\mathcal {X}_\star |_{\omega _\star ^p(T)}\) and, in particular, \(({\varPhi }_{+}-{\varPhi }_\star )|_{\omega _\star ^p(T)}\in \mathcal {X}_\star |_{\omega ^p_\star (T)}\). Hence Lemma 5.3(i) is applicable and proves \(P_{\star ,I}({\varPhi }_+-{\varPhi }_\star )|_T=({\varPhi }_+-{\varPhi }_\star )|_T\). For \(T\in \mathcal {T}_\star \) with \(T\subseteq \bigcup ([\mathcal {T}_\star ]{\setminus } [\mathcal {T}_+])\), the assertion follows immediately from the definition of \(P_{\star ,I}\), since \(\widehat{R}_{i,p}|_T=0\) for \(i\in I\).
Let \(\widetilde{\mathcal {N}}_\star :=\big \{z\in \mathcal {N}_\star \,:\,z\in \omega _\star ^{p}( [\mathcal {T}_\star ]{\setminus } [\mathcal {T}_{+}])\big \}\). For \(z\in \widetilde{\mathcal {N}}_\star \), let \(\varphi _z\) be the \(P^1\) hat function, i.e., \(\varphi _z(z')=\delta _{zz'}\) for all \(z'\in \mathcal {N}_\star \), \(\mathrm{supp}(\varphi _z)=\omega _\star (z)\), and \(\partial _{\varGamma } \varphi _z= \mathrm {const.}\) on \(T_{z,1}\) and \(T_{z,2}\), where \(\omega _\star (z)=T_{z,1}\cup T_{z,2}\) with \(T_{z,1},T_{z,2}\in \mathcal {T}_\star \). Because of Galerkin orthogonality and \(\sum _{z\in \widetilde{\mathcal {N}}_\star } \varphi _z=1\) on \(\omega _\star ^{p}( [\mathcal {T}_\star ]{\setminus } [\mathcal {T}_{+}])\), we see
We abbreviate \({\varSigma }:=\sum _{z\in \widetilde{\mathcal {N}}} \varphi _z (f-V{\varPhi }_\star )\) and estimate with (M1), Lemma 5.3(ii) and Proposition 4.1
as well as
So far, we thus have proved
Next, we use [25, Lemma 3.4], [24, Lemma 4.5], and \(|\partial _{\varGamma }\varphi _z|\simeq |\omega _\star (z)|^{-1}\) to estimate
It remains to consider the term \(\Vert h_\star ^{-1/2}(f-V{\varPhi }_\star )\Vert _{L^2(\omega ^{p+1}([\mathcal {T}_\star ]{\setminus } [\mathcal {T}_{+}]))}\) of (5.11) and (5.12). It holds
For any \(T\in \mathcal {T}_\star \), there is a function \(\psi _T\in \mathcal {X}_\star \) with connected support, \(T\subseteq \mathrm{supp}(\psi _T)\subseteq \omega _\star ^{\lceil p/2\rceil }(T)\) and \(\Vert 1-\psi _T\Vert _{L^2(\mathrm{supp}(\psi _T))}^2\le q\, |\mathrm{supp}(\psi _T)|\) with some \(q\,\in (0,1)\) which depends only on \(\check{\kappa }_\mathrm{max}, \gamma , p, w_\mathrm{min}\), and \(w_\mathrm{max}\); see [25, (A1)–(A2), Theorem 4.4]. We use some Poincaré inequality (see, e.g., [18, Lemma 2.5]) to see
The Galerkin orthogonality proves
Using (5.14), we therefore get
which implies
Hence, we are led to
With
we therefore conclude the proof. \(\square \)
Since we use a different mesh-refinement strategy, we cannot directly cite the following lemma from [8]. However, we may essentially follow the proof of [8, Proposition 4.12] verbatim. Details are left to the reader.
Lemma 5.4
(Optimality of Dörfler marking) Define
For all \(0<\overline{\theta }<\overline{\theta }_\mathrm{opt}\) there is some \(0<q_\mathrm{opt}<1\) such that for all refinements \([\mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ])\) of \([\mathcal {T}_\star ]\in [\mathbb {T}]\) the following implication holds true
The constant \(q_\mathrm{opt}\) depends only on \(\overline{\theta }\) and the constants \(C_\mathrm{stab}\) of Lemma 5.1 and \(C_\mathrm{rel}\) of Lemma 5.2. \(\square \)
The next lemma reads similarly as [8, Lemma 3.4]. Since we use a different mesh-refinement strategy and our estimator \(\rho \) does not satisfy the reduction axiom (A2), we cannot directly cite the result. However, the idea of the proof is the same. Indeed, one only needs a weaker version of the mentioned axiom.
Lemma 5.5
(Quasi-monotonicity of \(\rho )\) For all refinements \([\mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ])\) of \([\mathcal {T}_\star ]\in [\mathbb {T}],\) there holds
where \(C_\mathrm{mon}>0\) depends only on the parametrisation \(\gamma \) and the constants \(C_\mathrm{inv}\) of Proposition 4.1 and \(C_\mathrm{rel}\) of Lemma 5.2.
Proof
We split the estimator as follows
For the first sum, we use (5.4), \(\bigcup (\mathcal {T}_{+}{\setminus } \mathcal {T}_\star )=\bigcup (\mathcal {T}_\star {\setminus } \mathcal {T}_{+})\), and \(\check{h}_{+}\le \check{h}_\star \) to estimate
For the second sum, we use Lemma 5.1 to see
We end up with
Lemma 5.2 concludes the proof. \(\square \)
The optimality in Theorem 3.2 essentially follows from the following lemma. It was inspired by an analogous version from [8, Lemma 4.14].
Lemma 5.6
Suppose that \(\phi \in \mathbb {A}_s\) for some \(s>0\). Then, for all \(0<\overline{\theta }<\overline{\theta }_\mathrm{opt}\) there exist constants \(C_1,C_2>0\) such that for all meshes \([\mathcal {T}_\star ]\in [\mathbb {T}]\) there exists some refinement \([\mathcal {T}_{+}]\in \mathtt{ref}([\mathcal {T}_\star ])\) such that the corresponding set \(\mathcal {R}_\star (\mathcal {T}_+)\subseteq \mathcal {T}_\star \) from Lemma 5.2 satisfies
and
With the constants \(C_\mathrm{rel}, C_\mathrm{mon},\) and \(q_\mathrm{opt}\) from Lemmas 5.2, 5.4 and 5.5, it holds \(C_1=2C_\mathrm{rel}\) and \(C_2=(C_\mathrm{mon}q_\mathrm{opt}^{-1})^{1/2}\).
Proof
We set \(\alpha :=C_\mathrm{mon}^{-1} q_\mathrm{opt}\) with the constants of Lemma 5.4 and Lemma 5.5, and \(\delta ^2:=\alpha \rho _\star ^2\).
Step 1 There exists \([\mathcal {T}_\delta ]\in [\mathbb {T}]\) with
Let \(N\in \mathbb {N}_0\) be minimal with \((N+1)^{-s}\Vert \phi \Vert _{\mathbb {A}_s}\le \delta \). If \(N=0\), then \(\rho _0\le \Vert \phi \Vert _{\mathbb {A}_s}\le \delta \) and we can choose \([\mathcal {T}_\delta ]=[\mathcal {T}_0]\). If \(N>0\), minimality of N implies \(N^{-s}\Vert \phi \Vert _{\mathbb {A}_s}>\delta \) or equivalently \(N< \Vert \phi \Vert _{\mathbb {A}_s}^{1/s}\delta ^{-1/s}\). We choose \([\mathcal {T}_\delta ]\in [\mathbb {T}_N]\) such that
By the definition of \(\Vert \phi \Vert _{\mathbb {A}_s}\), we see
By the definition of \([\mathbb {T}_N]\), we see
Step 2 We consider the overlay \([\mathcal {T}_{+}]:= [\mathcal {T}_\star ]\oplus [\mathcal {T}_\delta ]\) of (M2). Quasi-monotonicity shows
Step 3 Finally, the assumptions on the refinement strategy are used. The overlay estimate and Step 1 give
Combining the last two estimates, we end up with
This proves (5.18) with \(C_1={2C_\mathrm{rel}}\) and \(C_2=\alpha ^{-1/2}\). By (5.20) we can apply Lemma 5.4 and see (5.19). \(\square \)
So far, we have only considered the auxiliary estimator \(\rho _\star \). In particular, we did not use Algorithm 3.1, but only the refinement strategy \(\mathtt{ref}(\cdot )\) itself. For the proof of optimal convergence (3.7), we proceed similarly as in [8, Theorem 8.4 (ii)].
Proof of (3.7)
Due to (5.2), there is a constant \(C\ge 1\) which depends only on \(\check{\kappa }_{\max }\) with \(\mu _\star ^2\le C\rho _\star ^2\) for all \([\mathcal {T}_\star ] \in [\mathbb {T}]\). We set \(\theta _\mathrm{opt}:= {\overline{\theta }_\mathrm{opt}}/{C}\) and \(\overline{\theta }:=C\,\theta \) and suppose that \(\theta \) is sufficiently small, namely, \(\theta <\theta _\mathrm{opt}\) and hence \(\overline{\theta }<\overline{\theta }_\mathrm{opt}\). Let \(\ell \in \mathbb {N}_0\) and \(j\le \ell \). Choose a refinement \([\mathcal {T}_{+}]\) of \([\mathcal {T}_j]\) as in Lemma 5.6. In particular, the set \(\mathcal {R}_j(\mathcal {T}_+)\) satisfies the Dörfler marking (5.20). According to (5.2), this implies
i.e., the set \(\mathcal {N}_j \cap \bigcup \mathcal {R}_j(\mathcal {T}_+)\) satisfies the Dörfler marking (3.3) from Algorithm 3.1. Since the chosen set \(\mathcal M_j\) of Algorithm 3.1 has essentially minimal cardinality, we see with (5.18) that
With the mesh-closure estimate of (M3), we get
Linear convergence (3.5) shows
Hence,
This concludes the proof. \(\square \)
6 Proof of Theorem 3.4, plain convergence (3.10)
To prove convergence of Algorithm 3.1 driven by the Faermann estimators \(\eta _\ell \), we apply an abstract result of [23, Section 2] which is recalled in the following: Let \({\mathcal {H}}\) be a Hilbert space with dual space \({\mathcal {H}}^*\) and \(V:{\mathcal {H}}\rightarrow {\mathcal {H}}^*\) be a linear elliptic operator and \(f\in {\mathcal {H}}^*\). Let \((\mathcal {X}_\ell (f))_{\ell \in \mathbb {N}_0}\) be a sequence of finite dimensional nested subspaces of \({\mathcal {H}}\), i.e., \(\mathcal {X}_\ell (f)\subseteq \mathcal {X}_{\ell +1}(f)\), with Galerkin approximations \({\varPhi }_\ell (f)\in \mathcal {X}_\ell (f)\) for the equation \(V\phi =f\). Further, let \((\mathcal {N}_\ell (f))_{\ell \in \mathbb {N}_0}\) be a sequence of arbitrary finite sets and
some heuristical error estimator, where we only require \(\eta _\ell (f,z)\ge 0\) for each \(z\in \mathcal {N}_\ell (f)\). Let \((\mathcal M_\ell (f))_{\ell \in \mathbb {N}_0}\) be a sequence of marked elements with \(\mathcal M_\ell (f)\subseteq \mathcal {N}_\ell (f)\) which satisfies the Dörfler marking, i.e.,
Additionally let
be an auxiliary error estimator with local contributions \({\widetilde{\rho }}_\ell (f,z)\ge 0\). Then, there holds the following convergence result.
Lemma 6.1
Suppose that \(D\subseteq {\mathcal {H}}^*\) is a dense subset of \({\mathcal {H}}^*\) such that for all \(f\in D\) and all \(\ell \in \mathbb {N}_0\) there is a set \(\mathcal {R}_\ell (f)\subseteq \mathcal M_\ell (f)\) such that the following assumptions (A1)–(A3) hold :
-
(A1)
\(\eta _\ell (f)\) is a local lower bound of \({\widetilde{\rho }}_\ell (f){:}\) There is a constant \(C_1>0\) such that
$$\begin{aligned} \eta _\ell (f,\mathcal M_\ell (f))\le C_1 {\widetilde{\rho }}_\ell (f,\mathcal {R}_\ell (f))\quad \text {for all }\ell \in \mathbb {N}_0. \end{aligned}$$ -
(A2)
\({\widetilde{\rho }}_\ell (f)\) is contractive on \(\mathcal {R}_\ell (f){:}\) There is a constant \(C_2\) such that for all \(\ell \in \mathbb {N}_0, m\in \mathbb {N}\) and all \(\delta >0,\) it holds
$$\begin{aligned}&C_2^{-1}{\widetilde{\rho }}_\ell (f,\mathcal {R}_\ell (f))^2\le {\widetilde{\rho }}_\ell (f)^2-\frac{1}{1+\delta }\, {\widetilde{\rho }}_{\ell +m}(f)^2+(1+\delta ^{-1})C_2\Vert {\varPhi }_{\ell +m}(f)\\&\quad -{\varPhi }_\ell (f)\Vert _{{\mathcal {H}}}^2. \end{aligned}$$
In addition, we suppose for all \(f\in {\mathcal {H}}^*\) validity of :
-
(A3)
\(\eta _\ell \) is stable on \(\mathcal M_\ell (f)\) with respect to f : there is a constant \(C_3>0\) such that, for all \(\ell \in \mathbb {N}_0\) and \(f'\in {\mathcal {H}}^*,\) it holds
$$\begin{aligned} |\eta _\ell (f,\mathcal M_\ell (f))-\eta _\ell (f',\mathcal M_\ell (f))|\le C_3 \Vert f-f'\Vert _{{\mathcal {H}}^*}. \end{aligned}$$
Then, there holds convergence \(\lim _{\ell \rightarrow \infty }\eta _\ell =0\) for all \(f\in {\mathcal {H}}^*\).
Proof of plain convergence (3.10)
We choose \({\mathcal {H}}=\widetilde{H}^{-1/2}({\varGamma })\), \({\mathcal {H}}^*=H^{1/2}({\varGamma })\), V the weakly-singular integral operator (1.1). Moreover, Algorithm 3.1 generates the transformed NURBS spaces \(\mathcal {X}_\ell (f)\), the set of nodes \(\mathcal {N}_\ell (f)\), the Faermann estimator \(\eta _\ell (f)\) and the set of marked nodes \(\mathcal M_\ell (f)\). We use the mesh-size function \(\widetilde{h}_\ell \) of Proposition 4.2 to define
if f is in the dense set \(D=H^1({\varGamma })\). We aim to apply Lemma 6.1 and show in the following that (A1)–(A2) hold for all \(f\in H^1({\varGamma })\) even with \(\mathcal {R}_\ell (f)=\mathcal M_\ell (f)\) and that (A3) holds for all \(f\in H^{1/2}({\varGamma })\). Then, Lemma 6.1 shows convergence (3.10) of the Faermann estimator.
-
(A1)
Of Lemma 6.1 follows immediately from [24, Theorem 4.3], where the constant \(C_1\) depends only on \(\check{\kappa }_{\max }, p\), and \(\gamma \).
-
(A3)
Can be proved exactly as in [23, Section 2.4] as \(\eta _\ell \) is efficient (see [25, Theorem 3.1]) and has a semi-norm structure. The constant \(C_3\) depends only on \({\varGamma }\).
The only challenging part is the proof of (A2) for fixed \(f\in H^1({\varGamma })\). We proceed similarly as in the proof of [23, Theorem 3.1]. In the following, we skip the dependence of f. The heart of the matter are the estimates \(\widetilde{h}_{\ell +1}\le q_\mathrm{ctr}\widetilde{h}_{\ell }\) on \(\omega _\ell (\mathcal M_\ell )\) and \(\widetilde{h}_{\ell +1}\le \widetilde{h}_\ell \) on \({\varGamma }\), which follow from Proposition 4.2 and
This shows
Hence, the estimator \({\widetilde{\rho }}_\ell \) satisfies
Here, the factor 1 / 2 on the left-hand side stems from the fact that each node patch consists (generically) of two elements. This fact also shows \(\Vert \widetilde{h}_\ell ^{\,1/2}\partial _{\varGamma }(f-V{\varPhi }_\ell )\Vert _{L^2({\varGamma })}^2=\widetilde{\rho }_\ell ^{\,2}/2\). The Young inequality \((c+d)^2\le (1+\delta )c^2+(1+\delta ^{-1})d^2\) for \(c,d\ge 0\), together with the triangle inequality shows
Finally, we use Proposition 4.1 and see
with a constant \(\widetilde{C}_\mathrm{inv}>0\) which depends only on \(C_\mathrm{inv}\) and \(h_\star \simeq \widetilde{h}_\star \). This yields
and concludes the proof of (A2) with \(C_2=\max (\frac{1}{1-q_\mathrm{ctr}},2 C_\mathrm{inv}^2)\). \(\square \)
References
Aurada, M., Feischl, M., Führer, T., Karkulik, M., Praetorius, D.: Efficiency and optimality of some weighted-residual error estimator for adaptive 2D boundary element methods. Comput. Methods Appl. Math. 13(3), 305–332 (2013)
Aurada, M., Feischl, M., Führer, T., Karkulik, M., Melenk, J.M., Praetorius, D.: Local inverse estimates for non-local boundary integral operators. Math. Comput. (2016) (accepted for publication), (preprint). arXiv:1504.04394
Bazilevs, Y., da Veiga, L.B., Cottrell, J.A., Hughes, T.J.R., Sangalli, G.: Isogeometric analysis: approximation, stability and error estimates for h-refined meshes. Math. Mod. Methods Appl. Sci. 16(07), 1031–1090 (2006)
Beirão da Veiga, L., Buffa, A., Sangalli, G., Vázquez, R.: Mathematical analysis of variational isogeometric methods. Acta Numer. 23, 157–287 (2014)
Buffa, A., Giannelli, C.: Adaptive isogeometric methods with hierarchical splines: error estimator and convergence. Math. Models Meth. Appl. Sci. 26(1), 1–25 (2016)
Carstensen, C.: An a posteriori error estimate for a first-kind integral equation. Math. Comput. 66(217), 139–155 (1997)
Carstensen, C., Faermann, B.: Mathematical foundation of a posteriori error estimates and adaptive mesh-refining algorithms for boundary integral equations of the first kind. Eng. Anal. Bound. Elem. 25(7), 497–509 (2001)
Carstensen, C., Feischl, M., Page, M., Praetorius, D.: Axioms of adaptivity. Comput. Math. Appl. 67(6), 1195–1253 (2014)
Cottrell, J.A., Hughes, T.J.R., Bazilevs, Y.: Isogeometric Analysis: Toward Integration of CAD and FEA. Wiley, Chichester (2009)
Cascon, J.M., Kreuzer, C., Nochetto, R.H., Siebert, K.G.: Quasi-optimal convergence rate for an adaptive finite element method. SIAM J. Numer. Anal. 46(5), 2524–2550 (2008)
Carstensen, C., Maischak, M., Praetorius, D., Stephan, E.P.: Residual-based a posteriori error estimate for hypersingular equation on surfaces. Numer. Math. 97(3), 397–425 (2004)
Carstensen, C., Maischak, M., Stephan, E.P.: A posteriori error estimate and \(h\)-adaptive algorithm on surfaces for Symm’s integral equation. Numer. Math. 90(2), 197–213 (2001)
Carstensen, C., Stephan, E.P.: Adaptive boundary element methods for some first kind integral equations. SIAM J. Numer. Anal. 33(6), 2166–2183 (1996)
de Boor, C.: B (asic)-spline basics. University of Wisconsin-Madison, Mathematics Research Center, Madison (1986)
Dahmen, W., Faermann, B., Graham, I.G., Hackbusch, W., Sauter, S.A.: Inverse inequalities on non-quasi-uniform meshes and application to the mortar element method. Math. Comput. 73(247), 1107–1138 (2004)
Dörfel, M.R., Jüttler, B., Simeon, B.: Adaptive isogeometric analysis by local \(h\)-refinement with T-splines. Comput. Methods Appl. Mech. Eng. 199(5–8), 264–275 (2010)
Dörfler, W.: A convergent adaptive algorithm for Poisson’s equation. SIAM J. Numer. Anal. 33(3), 1106–1124 (1996)
Faermann, B.: Localization of the Aronszajn–Slobodeckij norm and application to adaptive boundary element methods. I. The two-dimensional case. IMA J. Numer. Anal. 20(2), 203–234 (2000)
Feischl, M., Führer, T., Heuer, N., Karkulik, M., Praetorius, D.: Adaptive boundary element methods: a posteriori error estimators, adaptivity, convergence, and implementation. Arch. Comput. Methods Eng. 22, 309–389 (2015)
Feischl, M., Führer, T., Karkulik, M., Melenk, J.M., Praetorius, D.: Quasi-optimal convergence rates for adaptive boundary element methods with data approximation, part I: weakly-singular integral equation. Calcolo 51(4), 531–562 (2014)
Feischl, M., Führer, T., Karkulik, M., Melenk, J.M., Praetorius, D.: Quasi-optimal convergence rates for adaptive boundary element methods with data approximation, part II: hyper-singular integral equation. Electron. Trans. Numer. Anal. 44, 153–176 (2015)
Feischl, M., Führer, T., Karkulik, M., Praetorius, D.: ZZ-type a posteriori error estimators for adaptive boundary element methods on a curve. Eng. Anal. Bound. Elem. 38, 49–60 (2014)
Feischl, M., Führer, T., Mitscha-Eibl, G., Praetorius, D., Stephan, E.P.: Convergence of adaptive BEM and adaptive FEM-BEM coupling for estimators without \(h\)-weighting factor. Comput. Methods Appl. Math. 14(4), 485–508 (2014)
Feischl, M., Gantner, G., Haberl, A., Praetorius, D.: Adaptive 2D IGA boundary element methods. Eng. Anal. Bound. Elem. 62, 141–153 (2016)
Feischl, M., Gantner, G., Praetorius, D.: Reliable and efficient a posteriori error estimation for adaptive IGA boundary element methods for weakly-singular integral equations. Comput. Methods Appl. Mech. Eng. 290, 362–386 (2015)
Feischl, M., Karkulik, M., Melenk, J.M., Praetorius, D.: Quasi-optimal convergence rate for an adaptive boundary element method. SIAM J. Numer. Anal. 51(2), 1327–1348 (2013)
Gantumur, T.: Adaptive boundary element methods with convergence rates. Numer. Math. 124(3), 471–516 (2013)
Gantner, G.: Isogeometric adaptive BEM. Master’s thesis, Vienna University of Technology (2014)
Georgoulis, E.: Inverse-type estimates on hp-finite element spaces and applications. Math. Comput. 77(261), 201–219 (2008)
Graham, I.G., Hackbusch, W., Sauter, S.A.: Finite elements on degenerate meshes: inverse-type inequalities and applications. IMA J. Numer. Anal. 25(2), 379–407 (2005)
Heltai, L., Arroyo, M., DeSimone, A.: Nonsingular isogeometric boundary element method for Stokes flows in 3D. Comput. Methods Appl. Mech. Eng. 268, 514–539 (2014)
Hughes, T.J.R., Cottrell, J.A., Bazilevs, Y.: Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput. Methods Appl. Mech. Eng. 194(39–41), 4135–4195 (2005)
Hsiao, G.C., Wendland, W.L.: Boundary Integral Equations. Applied Mathematical Sciences, vol. 164. Springer, Berlin (2008)
McLean, W.: Strongly Elliptic Systems and Boundary Integral Equations. Cambridge University Press, Cambridge (2000)
Marussig, B., Zechner, J., Beer, G., Fries, T.-P.: Fast isogeometric boundary element method based on independent field approximation. Comput. Methods Appl. Math. 284, 458–488 (2015)
Politis, C., Ginnis, A.I., Kaklis, P.D., Belibassakis, K., Feurer, C.: An isogeometric BEM for exterior potential-flow problems in the plane. In: 2009 SIAM/ACM Joint Conference on Geometric and Physical Modeling, pp. 349–354. ACM (2009)
Peake, M.J., Trevelyan, J., Coates, G.: Extended isogeometric boundary element method (XIBEM) for two-dimensional Helmholtz problems. Comput. Methods Appl. Mech. Eng. 259, 93–102 (2013)
Simpson, R.N., Bordas, S.P.A., Trevelyan, J., Rabczuk, T.: A two-dimensional isogeometric boundary element method for elastostatic analysis. Comput. Methods Appl. Mech. Eng. 209(212), 87–100 (2012)
Sauter, S.A., Schwab, C.: Boundary Element Methods. Springer Series in Computational Mathematics, vol. 39. Springer, Berlin (2011). Translated and expanded from the 2004 German original
Scott, M.A., Simpson, R.N., Evans, J.A., Lipton, S., Bordas, S.P.A., Hughes, T.J.R., Sederberg, T.W.: Isogeometric boundary element analysis using unstructured T-splines. Comput. Methods Appl. Mech. Eng. 254, 197–221 (2013)
Steinbach, O.: Numerical Approximation Methods for Elliptic Boundary Value Problems. Springer, New York (2008). Translated from the 2003 German original
Takahashi, T., Matsumoto, T.: An application of fast multipole method to isogeometric boundary element method for Laplace equation in two dimensions. Eng. Anal. Bound. Elem. 36(12), 1766–1775 (2012)
Vuong, A.-V., Giannelli, C., Jüttler, B., Simeon, B.: A hierarchical approach to adaptive local refinement in isogeometric analysis. Comput. Methods Appl. Mech. Eng. 200(49–52), 3554–3567 (2011)
Zechner, J., Marussig, B., Beer, G., Fries, T.-P.: Isogeometric boundary element method with hierarchical matrices (2014) (preprint). arXiv:1406.2817
Acknowledgments
Open access funding provided by [TU Wien (TUW)]. The authors acknowledge support through the Austrian Science Fund (FWF) under Grant P27005 Optimal adaptivity for BEM and FEM-BEM coupling, P29096 Optimal isogeometric boundary element methods and the FWF doctoral school Dissipation and Dispersion in Nonlinear PDEs funded under Grant W1245. Moreover, MF was supported by the Australian Research Council under grant number DP160101755.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Feischl, M., Gantner, G., Haberl, A. et al. Optimal convergence for adaptive IGA boundary element methods for weakly-singular integral equations. Numer. Math. 136, 147–182 (2017). https://doi.org/10.1007/s00211-016-0836-8
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00211-016-0836-8