Skip to main content
SpringerLink
Log in

Rational pullbacks of Galois covers

  • Published:
Mathematische Zeitschrift Aims and scope Submit manuscript

Abstract

The finite subgroups of \(\mathrm{PGL}_2({\mathbb {C}})\) are shown to be the only finite groups G with this property: for some integer \(r_0\) (depending on G), all Galois covers \(X\rightarrow {\mathbb {P}}^1_{\mathbb {C}}\) of group G can be obtained by pulling back those with at most \(r_0\) branch points along non-constant rational maps \({\mathbb {P}}^1_{\mathbb {C}}\rightarrow {\mathbb {P}}^1_{\mathbb {C}}\). For \(G\subset \mathrm{PGL}_2({\mathbb {C}})\), it is in fact enough to pull back one well-chosen cover with at most 3 branch points. A consequence of the converse for inverse Galois theory is that, for \(G\not \subset \mathrm{PGL}_2({\mathbb {C}})\), letting the branch point number grow provides truly new Galois realizations \(F/{\mathbb {C}}(T)\) of G. Another application is that the “Beckmann–Black” property that “any two Galois covers of \({\mathbb {P}}^1_{\mathbb {C}}\) with the same group G are always pullbacks of another Galois cover of group G” only holds if \(G\subset \mathrm{PGL}_2({\mathbb {C}})\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. The term “regularly” will be fully justified with the general definition of “k-regular parametricity” for which the base field k is not necessarily algebraically closed (see Definition 2.1).

  2. Above and throughout the paper, the condition “\(G \subset \mathrm{PGL}_2({\mathbb {C}})\)” (resp., “\(G \not \subset \mathrm{PGL}_2({\mathbb {C}})\)”) really means that G is isomorphic (resp., is not isomorphic) to a subgroup of \(\mathrm{PGL}_2({\mathbb {C}})\).

  3. The case \(N=1\) is particularly significant as it supports Hilbert’s strategy to solve the Inverse Galois Problem by first producing a \({\mathbb {Q}}\)-regular Galois cover \(f:X\rightarrow {\mathbb {P}}^1_{\mathbb {Q}}\) of given group. It is known to hold for some groups: abelian, \(S_n\), \(A_n\), dihedral of order 2n with \(n>1\) odd, etc.

  4. which is the function field extension associated with \(f \otimes _k {\overline{k}} : X \otimes _k {\overline{k}} \rightarrow {\mathbb {P}}^1_{{\overline{k}}}\).

  5. in the sense that they correspond to \(e^{2i\pi /e_i}\) in the canonical isomorphism \(I_{{\mathfrak {P}}} \rightarrow \mu _{e_i} =\langle e^{2i\pi /e_i} \rangle \).

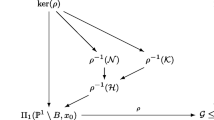
  6. Due to our definition of the categories \({{\textsf {H}}}_{G,r}(k)\) and \({{\textsf {H}}}_{G,r}(\mathbf{C})(k)\), it is the so-called inner version of Hurwitz spaces that we shall be working with.

  7. In particular, the degree of \(T_0\in k(U)\) (the maximum of numerator degree and denominator degree in coprime notation) is the same as the degree of the associated map \({\mathbb {P}}^1_k \rightarrow {\mathbb {P}}^1_k\).

  8. Here, a subset of a topological space is called constructible if it is a finite union of locally closed sets.

  9. In fact, \(|G|\in \{2,3,4,6\}\), since G is then a group of automorphisms of some elliptic curve, see [28, Chapter III, Theorem 10.1].

  10. Note here that equivalent covers have the same defining equations by definition, so that the term “defining equation for an element of \({\mathcal {H}}_{G,r}(\mathbf{C})(k)\)” is indeed well-defined.

  11. There may be a priori two different \(j_i\in \{1,\ldots ,r\}\) such that \({{\mathcal {C}}}_i\in \mathbf{C}_{f,T_0,j_i}\).

  12. Definition of “ample field” is recalled in Remark 1.4(a).

  13. In fact, the assumption on H to be non-solvable can be removed with a bit of extra effort. Moreover, if H is not a regular Galois group over k, then G is not either. Hence, Theorem 1.1(b) trivially fails.

  14. We note that, for \(R\ge 6\), this claim also follows for any choice of y from [7, Theorem 3.1(b-2)], since the latter implies that every pullback of a k-cover in \({{\textsf {H}}}_{G,R}({\mathbf{C}})\) has at least \(R+2\) branch points, and hence is not in \({{\textsf {H}}}_{G,R+1}(\mathbf{D}_y)(k).\)

References

  1. Arbarello, E., Cornalba, M.: Footnotes to a paper of Beniamino Segre: “On the modules of polygonal curves and on a complement to the Riemann existence theorem” (Italian) [Math. Ann. 100 (1928), 537–551; Jbuch 54, 685]. The number of \(g^{1}_{d}\)’s on a general \(d\)-gonal curve, and the unirationality of the Hurwitz spaces of \(4\)-gonal and \(5\)-gonal curves. Math. Ann., 256(3):341–362 (1981)

  2. Bary-Soroker, L., Fehm, A.: Open problems in the theory of ample fields. In Geometric and differential Galois theories, volume 27 of Sémin. Congr., pages 1–11. Soc. Math. France, Paris (2013)

  3. Dèbes, P., Deschamps, B.: The regular inverse Galois problem over large fields. In Geometric Galois actions, 2, volume 243 of London Math. Soc. Lecture Note Ser., pages 119–138. Cambridge Univ. Press, Cambridge (1997)

  4. Dèbes, P., Douai, J-C.: Algebraic covers: field of moduli versus field of definition. Ann. Sci. École Norm. Sup. (4), 30(3):303–338 (1997)

  5. Dèbes, P., König, J., Legrand, F., Neftin, D.: On parametric and generic polynomials with one parameter. J. Pure Appl. Algebra 225(10), 106717 (2021)

    Article  MathSciNet  Google Scholar 

  6. Dèbes, P., König, J., Legrand, F., Neftin, D.: Rational pullbacks of Galois covers. Manuscript (2018). arXiv:1807.01937v2

  7. Dèbes, P.: Groups with no parametric Galois realizations. Ann. Sci. Éc. Norm. Supér. (4), 51(1):143–179 (2018)

  8. Dèbes, P.: Méthodes topologiques et analytiques en théorie inverse de Galois : théorème d’existence de Riemann. (French). In Arithmétique de revêtements algébriques (Saint-Étienne, 2000), volume 5 of Sémin. Congr., pages 27–41. Soc. Math. France, Paris (2001)

  9. Dèbes, P.: Density results for Hilbert subsets. Indian J. Pure Appl. Math. 30(1), 109–127 (1999)

    MathSciNet  MATH  Google Scholar 

  10. Dèbes, P.: On the Malle conjecture and the self-twisted cover. Israel J. Math. 218(1), 101–131 (2017)

    Article  MathSciNet  Google Scholar 

  11. Dèbes, P., Legrand, F.: Specialization results in Galois theory. Trans. Am. Math. Soc. 365(10), 5259–5275 (2013)

    Article  MathSciNet  Google Scholar 

  12. Fried, M.D., Jarden, M.: Field arithmetic. Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics], 11. Springer-Verlag, Berlin, third edition, (2008). Revised by Jarden. xxiv+792 pp

  13. Fried, M.D.: Fields of definition of function fields and Hurwitz families-groups as Galois groups. Comm. Algebra 5(1), 17–82 (1977)

    Article  MathSciNet  Google Scholar 

  14. Fried, M.D., Völklein, H.: The inverse Galois problem and rational points on moduli spaces. Math. Ann. 290(4), 771–800 (1991)

    Article  MathSciNet  Google Scholar 

  15. Grothendieck, A.: Eléments de géométrie algébrique. IV. Etude locale des schémas et des morphismes de schémas. I. (French). Inst. Hautes Etudes Sci. Publ. Math., 20, 259 pp (1964)

  16. Jarden, M.: Algebraic patching. Springer Monographs in Mathematics. Springer, Heidelberg, 2011. xxiv+290 pp

  17. Jensen, C.U., Ledet, A., Yui, N.: Generic polynomials. Constructive Aspects of the Inverse Galois Problem. Mathematical Sciences Research Institute Publications, 45. Cambridge University Press. x+258 pp (2002)

  18. Jordan, C.: Recherches sur les substitutions. J. Liouville 17, 351–367 (1872)

    MATH  Google Scholar 

  19. König, J., Legrand, F.: Non-parametric sets of regular realizations over number fields. J. Algebra 497, 302–336 (2018)

    Article  MathSciNet  Google Scholar 

  20. König, J., Legrand, F., Neftin, D.: On the local behavior of specializations of function field extensions. Int. Math. Res. Not. IMRN 2019(9), 2951–2980 (2019)

    Article  MathSciNet  Google Scholar 

  21. Malle, G., Matzat, B.H.: Inverse Galois theory. Springer Monographs in Mathematics. Springer, Berlin. Second edition. xvii+532 pp (2018)

  22. Müller, P.: Finiteness results for Hilbert’s irreducibility theorem. Ann. Inst. Fourier (Grenoble) 52(4), 983–1015 (2002)

    Article  MathSciNet  Google Scholar 

  23. Mumford, D.: The red book of varieties and schemes, volume 1358 of Lecture Notes in Mathematics. Springer-Verlag, Berlin, Second, expanded edition, 1999. Includes the Michigan lectures (1974) on curves and their Jacobians, With contributions by Enrico Arbarello. x+306 pp

  24. Pop, F.: 1/2 Riemann existence theorem with Galois action. In Algebra and number theory (Essen, 1992), pages 193–218. de Gruyter, Berlin (1994)

  25. Pop, F.: Embedding problems over large fields. Ann. Math. (2), 144(1):1–34 (1996)

  26. Pop, F.: Little survey on large fields - old & new. In Valuation theory in interaction, EMS Ser. Congr. Rep., pages 432–463. Eur. Math. Soc., Zürich (2014)

  27. Sadi, B.: Descente effective du corps de définition des revêtements. Thèse Université Lille 1, (1999). https://ori-nuxeo.univ-lille1.fr/nuxeo/site/esupversions/73ccb929-17bf-4a20-abc6-0aac9072c8d3

  28. Silverman, J.H.: The arithmetic of elliptic curves, volume 106 of Graduate Texts in Mathematics. Springer, Dordrecht, second edition, (2009). xx+513 pp

  29. Völklein, H.: Groups as Galois groups. An introduction, volume 53 of Cambridge Studies in Advanced Mathematics. Cambridge University Press, Cambridge, (1996). xviii+248 pp

Download references

Acknowledgements

This work was supported in part by the Labex CEMPI (ANR-11-LABX-0007-01) and ISF grants No. 577/15 and No. 696/13.

Author information

Authors and Affiliations

  1. Laboratoire de Mathématiques Paul Painlevé, Université de Lille, 59655, Villeneuve d’Ascq Cedex, France

    Pierre Dèbes

  2. Department of Mathematics Education, Korea National University of Education, 28173, Cheongju, South Korea

    Joachim König

  3. Institut für Algebra, Fachrichtung Mathematik, TU Dresden, 01062, Dresden, Germany

    François Legrand

  4. Department of Mathematics, Technion, Israel Institute of Technology, Haifa, 32000, Israel

    Danny Neftin

Authors
  1. Pierre Dèbes
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Joachim König
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. François Legrand
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Danny Neftin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Pierre Dèbes.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dèbes, P., König, J., Legrand, F. et al. Rational pullbacks of Galois covers. Math. Z. 299, 1507–1531 (2021). https://doi.org/10.1007/s00209-021-02703-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00209-021-02703-z

Keywords

Mathematics Subject Classification

Search

Navigation