Dynamics of neuronal waves

Abstract

First of all, by studying the existence and stability of traveling wave fronts of the following nonlinear nonlocal model equation

$$u_t+au_x+u=\alpha\mathop{\int}_{\mathbb R}K(x-y)H(u(y,t)-\theta){\rm d}y +\beta\mathop{\int}_{\mathbb R}K(x-y)H(u(y,t)-\Theta){\rm d}y,$$

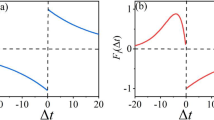
we derive relation between speed index function and stability index function for each of the waves. This model was derived when studying working memory in synaptically coupled neuronal networks, which incorporates low persistent activity rate θ and high saturating rate Θ. We will investigate dynamics of neuronal waves. For this purpose, we will be concerned with the equation for several different kinds of symmetric and asymmetric kernels and will compare speeds of the waves. Our analysis and results on the speed index functions facilitate our investigation on stability of the waves and the estimates of speeds. Secondly, we are concerned with standing waves of the nonlinear nonhomogeneous system of integral-differential equations

$$\begin{array}{lll} u_t+u+w& =&\alpha \mathop{\int}\limits_{\mathbb R}K(x-y)H(u(y,t)-\theta){\rm d}y\\ &&+\,\beta \mathop{\int}\limits_{\mathbb R}K(x-y)H(u(y,t)-\Theta){\rm d}y+{\mathcal I}(x,t),\\ w_t&=&\varepsilon(u-\gamma w),\end{array}$$

and the scalar equation

$$\begin{array}{lll} u_t+u&=&\alpha \mathop{\int}\limits_{\mathbb R}K(x-y)H(u(y,t)-\theta){\rm d}y\\ &&+\,\beta \mathop{\int}\limits_{\mathbb R}K(x-y)H(u(y,t)-\Theta){\rm d}y+{\mathcal I}(x,t).\end{array}$$