On the Triple Junction Problem without Symmetry Hypotheses

Abstract

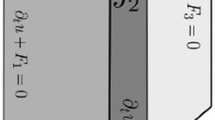
We investigate the Allen–Cahn system $\Delta u-W_u(u)=0$, $u:\mathbb {R}^2\rightarrow \mathbb {R}^2$, where $W\in C^2(\mathbb {R}^2,[0,+\infty ))$ is a potential with three global minima. We establish the existence of an entire solution u which possesses a triple junction structure. The main strategy is to study the global minimizer $u_\varepsilon$ of the variational problem $\min \int _{B_1} \left( \frac{\varepsilon }{2}\vert \nabla u\vert ^2+\frac{1}{\varepsilon }W(u) \right) \,\textrm{d}z$, $u=g_\varepsilon$ on $\partial B_1$ for some suitable boundary data $g_\varepsilon$. The point of departure is an energy lower bound that plays a crucial role in estimating the location and size of the diffuse interface. We do not impose any symmetry hypothesis on the solution or on the potential.

Appendices

Appendix A: Proof of Lemma 2.5

For sufficiently small $\varepsilon$, we want to construct an energy competitor $u_{test}\in W^{1,2}(B_1,\mathbb {R}^2)$ with the boundary condition $u_{test}\big \vert _{\partial B_1}=g_\varepsilon$ and show that

$$\begin{aligned} \int _{B_1}\left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\le \sigma _{12}+\sigma _{13}+\sigma _{23}+C\varepsilon , \end{aligned}$$

(A1)

for a positive constant C independent of $\varepsilon$.

Let $c_0$ be as in (19). Set

$$\begin{aligned} r_1{:}{=}c_0\varepsilon ,\quad r_2{:}{=}1-c_0\varepsilon . \end{aligned}$$

We also define the following angles that correspond to the locations of the three interfaces.

$$\begin{aligned} \varphi _{12}{:}{=}\frac{\pi }{2}+\frac{\alpha _2-\alpha _1}{2},\quad \varphi _{13}{:}{=}\frac{3\pi }{2}-\frac{\alpha _3}{2},\quad \varphi _{23}{:}{=}\frac{3\pi }{2}+\frac{\alpha _3}{2}. \end{aligned}$$

Set $\varphi _0$ as a fixed angle

$$\begin{aligned} \varphi _0{:}{=}\frac{1}{4}\min \{\alpha _1,\alpha _2,\alpha _3\}. \end{aligned}$$

We define

$$\begin{aligned}&\begin{aligned} S(s,t;\theta _1,\theta _2)&{:}{=}\{(r\cos \theta ,r\sin \theta ):s\le r\le t,\theta _1\le \theta \le \theta _2 \},\\ {}&\quad \text {if}\quad 0\le s<t\le 1,\quad 0\le \theta _1<\theta _2\le 2\pi . \end{aligned}\\&\begin{aligned} S(s,t;\theta _1,\theta _2)&{:}{=}\{(r\cos \theta ,r\sin \theta ):s\le r\le t,\theta \in [\theta _1,2\pi ]\cup [0,\theta _2] \},\\ {}&\quad \text {if}\quad 0\le s<t\le 1,\quad 0\le \theta _2<\theta _1\le 2\pi . \end{aligned} \end{aligned}$$

Recall that $U_{12}\in W_{loc}^{1,2}(\mathbb {R},\mathbb {R}^2)$ is a 1D minimizer of the minimization problem

$$\begin{aligned}&\min \int _{-\infty }^\infty \left( \frac{1}{2}\vert v'\vert ^2+W(v) \right) \,d\eta ,\\&\lim \limits _{x\rightarrow -\infty } v(\eta )=a_1,\quad \lim \limits _{\eta \rightarrow \infty } v(\eta )=a_2, \quad v(\mathbb {R})\subset \mathbb {R}^2\setminus \{a_1,a_2\}. \end{aligned}$$

The properties of this 1D minimizer play an important role in our construction of $u_{test}$.

Now we are ready to construct $u_{test}$. On $S(r_1,r_2; \varphi _{12}-\varphi _0,\varphi _{12}+\varphi _0)$, we set

$$\begin{aligned} u_{test}=U_{12}\left( \frac{r\sin (\varphi _{12}-\theta )}{\varepsilon }\right) \end{aligned}$$

By the exponential decay estimate for the minimizing connection $U_{12}$ (see [6, Proposition 2.4]), we have the following estimates for $u_{test}$ on $\partial S(r_1,r_2;\varphi _{12}-\varphi _0,\varphi _{12}+\varphi _0)$:

$$\begin{aligned}&\vert u_{test}(r,\varphi _{12}-\varphi _0)-a_2\vert \le Ke^{-\frac{kr}{\varepsilon }\sin {\varphi _0}},\quad \text {on}\quad \{r_1\le r\le r_2, \theta =\varphi _{12}-\varphi _0\}, \end{aligned}$$

(A2)

$$\begin{aligned}&\vert u_{test}(r,\varphi _{12}+\varphi _0)-a_1\vert \le Ke^{-\frac{kr}{\varepsilon }\sin {\varphi _0}},\quad \text {on}\quad \{r_1\le r\le r_2, \theta =\varphi _{12}+\varphi _0\}, \end{aligned}$$

(A3)

$$\begin{aligned}&\vert u_{test}(r_1,\theta )-a_1\vert \le Ke^{-kc_0\sin (\theta -\varphi _{12})},\quad \text {on}\quad \{r=r_1, \varphi _{12}\le \theta \le \varphi _{12}+\varphi _0\},\end{aligned}$$

(A4)

$$\begin{aligned}&\vert u_{test}(r_1,\theta )-a_2\vert \le Ke^{-kc_0\sin (\varphi _{12}-\theta )}, \quad \text {on}\quad \{r=r_1, \varphi _{12}-\varphi _0\le \theta \le \varphi _{12}\}, \end{aligned}$$

(A5)

$$\begin{aligned}&\vert u_{test}(r_2,\theta )-a_1\vert \le Ke^{-k\frac{r_2\sin (\theta -\varphi _{12})}{\varepsilon }}, \quad \text {on}\quad \{r=r_2, \varphi _{12}\le \theta \le \varphi _{12}+\varphi _0\},\end{aligned}$$

(A6)

$$\begin{aligned}&\vert u_{test}(r_2,\theta )-a_2\vert \le Ke^{-k\frac{r_2\sin (\varphi _{12}-\theta )}{\varepsilon }}, \quad \text {on}\quad \{r=r_2, \varphi _{12}-\varphi _0\le \theta \le \varphi _{12}\}. \end{aligned}$$

(A7)

Here K, k are constants that are independent of $\varepsilon$.

For calculating the energy in $S(r_1,r_2;\varphi _{12}-\varphi _0,\varphi _{12}+\varphi _0)$, it is convenient to rotate the coordinate system by setting

$$\begin{aligned} x'=\cos \frac{\alpha _2-\alpha _1}{2}x+\sin \frac{\alpha _2-\alpha _1}{2}y,\quad y'=-\sin \frac{\alpha _2-\alpha _1}{2}x+\cos \frac{\alpha _2-\alpha _1}{2}y. \end{aligned}$$

Note that $u_{test}$ only depends on the $x'$ variable, thus $\vert \partial _{y'} u_{test}\vert =0$. We compute the energy

$$\begin{aligned}&\int _{S(r_1,r_2,\varphi _{12}-\varphi _0,\varphi _{12}+\varphi _0)} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\nonumber \\&\quad \le \int _{r_1}^{r_2}\,\textrm{d}y'\int _{-\tan {\varphi _0}}^{\tan {\varphi _0}}\left( \frac{\varepsilon }{2}\vert \partial _{x'} u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}x'\nonumber \\&\quad \le (r_2-r_1)\sigma _{12}\nonumber \\&\quad \le (1-c_0\varepsilon )\sigma _{12}. \end{aligned}$$

(A8)

Similarly, we set, respectively,

$$\begin{aligned} u_{test}&=U_{31}\left( \frac{r\sin {(\varphi _{13}-\theta )}}{\varepsilon }\right) , \quad \text {on}\quad S(r_1,r_2;\varphi _{13}-\varphi _0,\varphi _{13}+\varphi _0),\\ u_{test}&=U_{23}\left( \frac{r\sin {(\varphi _{23}-\theta )}}{\varepsilon }\right) , \quad \text {on}\quad S(r_1,r_2;\varphi _{23}-\varphi _0,\varphi _{23}+\varphi _0). \end{aligned}$$

Note that if $\varphi _{23}+\varphi _0>2\pi$, we can replace it by $\varphi _{23}+\varphi _0-2\pi$. In particular, when $\theta =\varphi _{13}-\varphi _0$, it holds that

$$\begin{aligned} \vert u_{test}(r,\varphi _{13}-\varphi _0)-a_1\vert \le Ke^{-k\frac{r\sin {\varphi _0}}{\varepsilon }},\quad \text {on}\quad \{r_1\le r\le r_2,\,\theta =\varphi _{13}-\varphi _0\}. \end{aligned}$$

(A9)

With (A3) and (A9) we are able to define $u_{test}$ on $S(r_1,r_2;\varphi _{12}+\varphi _0,\varphi _{13}-\varphi _0)$ by

$$\begin{aligned} u_{test}(r,\theta ){:}{=} u_{test}(r,\varphi _{12}+\varphi _0)\frac{\varphi _{13}-\varphi _0-\theta }{\alpha _1-2\varphi _0}+u_{test}(r,\varphi _{13}-\varphi _0)\frac{\theta -(\varphi _{12}+\varphi _0)}{ \alpha _1-2\varphi _0}. \end{aligned}$$

This construction can be extended to $S(r_1,r_2; \varphi _{13}+\varphi _0,\varphi _{23}-\varphi _0)$ and $S(r_1,r_2;\varphi _{23}+\varphi _0, \varphi _{12}-\varphi _0)$ in the same way. The energy in $S(r_1,r_2;\varphi _{12}+\varphi _0,\varphi _{13}-\varphi _0)$ will be estimated in polar coordinates.

$$\begin{aligned}&\int _{S(r_1,r_2;\varphi _{12}+\varphi _0,\varphi _{13}-\varphi _0)} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\nonumber \\&\quad =\int _{\varphi _{12}+\varphi _0}^{\varphi _{13}-\varphi _0}\int _{r_1}^{r_2} \left( \frac{\varepsilon }{2}\left( \left| \frac{\partial u_{test}}{\partial r}\right| ^2+\frac{1}{r^2}\left| \frac{\partial u_{test}}{\partial \theta }\right| ^2\right) +\frac{1}{\varepsilon }W(u_{test}) \right) r\,dr\,d\theta . \end{aligned}$$

(A10)

Note that

$$\begin{aligned} \left| \frac{\partial u_{test}}{\partial r}\right| ^2&\le 2\left( \left| \frac{\partial u_{test}(r,\varphi _{12}+\varphi _0)}{\partial r}\right| ^2+\left| \frac{\partial u_{test}(r,\varphi _{13}-\varphi _0)}{\partial r}\right| ^2\right) \\&\le \frac{2\sin ^2{\varphi _0}}{\varepsilon ^2} \left( \left| U_{12}'\left( -\frac{r\sin {\varphi _0}}{\varepsilon }\right) \right| ^2+\left| U_{31}'\left( \frac{r\sin {\varphi _0}}{\varepsilon }\right) \right| ^2\right) \\&\le \frac{Ce^{-\frac{2kr}{\varepsilon }\sin {\varphi _0}}}{\varepsilon ^2},\\ \left| \frac{\partial u_{test}}{\partial \theta }\right| ^2&\le Ce^{-\frac{2kr}{\varepsilon }\sin {\varphi _0}},\quad W(u_{test}(r,\theta ))\le Ce^{-\frac{2kr}{\varepsilon }\sin {\varphi _0}}, \end{aligned}$$

where C is a universal constant.

Substituting these into (A10) yields

$$\begin{aligned}&\int _{S(r_1,r_2;\varphi _{12}+\varphi _0,\varphi _{13}-\varphi _0)} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\nonumber \\&\quad \le \int _{\varphi _{12}+\varphi _0}^{\varphi _{13}-\varphi _0} \!\!\int _{c_0\varepsilon }^{1-c_0\varepsilon } \left( \! \frac{\varepsilon }{2}\left( \! \frac{Ce^{-\frac{2kr}{\varepsilon }\sin {\varphi _0}}}{\varepsilon ^2}\!+\!\frac{Ce^{-\frac{2kr}{\varepsilon }\sin {\varphi _0}}}{r^2} \!\right) \!+\!\frac{1}{\varepsilon }Ce^{-\frac{2kr}{\varepsilon }\sin {\varphi _0}}\! \right) r\,\textrm{d}r\textrm{d}\theta \nonumber \\&\quad \le C(W,c_0)\varepsilon , \end{aligned}$$

(A11)

for some constant $C(W,c_0)$ that does not depend on $\varepsilon$.

Now that $u_{test}$ has been already defined on the annulus $S(r_1,r_2;0,2\pi )$, we proceed to define $u_{test}$ in the inner ball $B_{r_1}$ and the outer layer $S(r_2,1; 0,2\pi )$. First of all we take $u_{test}$ to be the harmonic extension in $B_{r_1}$, with respect to its boundary data.

$$\begin{aligned} \begin{aligned} \Delta&u_{test}=0 \text { in }B_{r_1},\\ u_{test}\big \vert _{\partial B_{r_1}}&\text { is given by the construction on }S(r_1,r_2;0,2\pi ). \end{aligned} \end{aligned}$$

It is not hard to verify that

$$\begin{aligned} \vert u_{test}\vert \le C,\quad \vert \nabla _T u_{test}\vert \le \frac{C}{\varepsilon } \quad \text {on}\quad \partial B_{r_1}, \end{aligned}$$

where $\nabla _T$ denotes the tangential derivative, $C=C(W,c_0)$ is a constant independent of $\varepsilon$. By elliptic regularity, $\vert u_{test}\vert$ and $\varepsilon \vert \nabla u_{test}\vert$ are also bounded by some universal constant C inside $B_{r_1}$. Then we have

$$\begin{aligned} \int _{B_{r_1}} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\le & {} \pi (c_0\varepsilon )^2\left( \frac{\varepsilon }{2} \left( \frac{C}{\varepsilon }\right) ^2+\frac{1}{\varepsilon }C\right) \nonumber \\\le & {} C(W,c_0)\varepsilon . \end{aligned}$$

(A12)

It remains to construct $u_{test}$ on the annulus $S(r_2,1;0,2\pi )$. Set

$$\begin{aligned} u_{test}(r,\theta )=\frac{1-r}{c_0\varepsilon } u_{test}(r_2,\theta )+\frac{r-r_2}{c_0\varepsilon }g_{\varepsilon }(\theta ), \quad r_2\le r\le 1, \quad \theta \in [0,2\pi ). \end{aligned}$$

(A13)

Here $g_\varepsilon$ is the boundary data on $\partial B_1$ defined by (19) and $u_{test}(r_2,\theta )$ is given by the construction of $u_{test}$ on $\partial S(r_1,r_2;0,2\pi )$. We have

$$\begin{aligned}&\int _{S(r_2,1;\varphi _{12}-\varphi _0,\varphi _{13}-\varphi _0)} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\nonumber \\&\quad =\left( \int _{\varphi _{12}-c_0\varepsilon }^{\varphi _{12}+c_0\varepsilon }+\int _{\varphi _{12}-\varphi _0}^{\varphi _{12}-c_0\varepsilon }+\int _{\varphi _{12}+c_0\varepsilon }^{\varphi _{12}+\varphi _0}+\int _{\varphi _{12}+\varphi _0}^{\varphi _{13}-\varphi _0} \right) \nonumber \\&\qquad \int _{r_2}^{1} \bigg ( \frac{\varepsilon }{2}\left( \left| \frac{\partial u_{test}}{\partial r}\right| ^2 +\frac{1}{r^2}\left| \frac{\partial u_{test}}{\partial \theta }\right| ^2\right) +\frac{1}{\varepsilon }W(u_{test}) \bigg )r\,\textrm{d}r\,\textrm{d}\theta . \end{aligned}$$

(A14)

We estimate each part separately. In $S(r_2,1;\varphi _{12}-c_0\varepsilon ,\varphi _{12}+c_0\varepsilon )$, it holds that

$$\begin{aligned}&\int _{\varphi _{12}-c_0\varepsilon }^{\varphi _{12}+c_0\varepsilon }\int _{r_2}^1 \left( \frac{\varepsilon }{2}\left( \left| \frac{\partial u_{test}}{\partial r}\right| ^2+\frac{1}{r^2}\left| \frac{\partial u_{test}}{\partial \theta }\right| ^2\right) +\frac{1}{\varepsilon }W(u_{test}) \right) r\,\textrm{d}r\,\textrm{d}\theta .\\&\quad \le 2\vert c_0\varepsilon \vert ^2 \cdot \left( \frac{\varepsilon }{2}\bigg \vert \frac{C}{\varepsilon }\bigg \vert ^2+\frac{1}{\varepsilon } C \right) \le C\varepsilon ; \end{aligned}$$

In $S(r_2,1; \varphi _{12}-\varphi _0,\varphi _{12}-c_0\varepsilon )\cup S(r_2,1; \varphi _{12}+c_0\varepsilon ,\varphi _{12}+\varphi _0)$, by (A6) and (A7) we have

$$\begin{aligned}&\left( \int _{\varphi _{12}-\varphi _0}^{\varphi _{12}-c_0\varepsilon }+\int _{\varphi _{12}+c_0\varepsilon }^{\varphi _{12}+\varphi _0}\right) \int _{r_2}^1 \left( \frac{\varepsilon }{2}\left( \left| \frac{\partial u_{test}}{\partial r}\right| ^2+\frac{1}{r^2}\left| \frac{\partial u_{test}}{\partial \theta }\right| ^2\right) +\frac{1}{\varepsilon }W(u_{test}) \right) r\,\textrm{d}r\,\textrm{d}\theta .\\&\quad \le \left( \int _{\varphi _{12}-\varphi _0}^{\varphi _{12}-c_0\varepsilon }+\int _{\varphi _{12}+c_0\varepsilon }^{\varphi _{12}+\varphi _0}\right) \int _{r_2}^1 \bigg ( \frac{\varepsilon }{2}\frac{\vert u_{test}(r_2,\theta )-g_\varepsilon (\theta )\vert ^2}{(c_0\varepsilon )^2}\\&\qquad +\frac{\varepsilon }{2r^2}\left( \left| \frac{\partial u_{test}(r_2,\theta )}{\partial \theta }\right| ^2+\left| \frac{\partial g_\varepsilon (\theta )}{\partial \theta }\right| ^2\right) +\frac{1}{\varepsilon } C\vert u_{test}(r_2,\theta )-g_\varepsilon (\theta )\vert ^2\bigg )r\,\textrm{d}r\,\textrm{d}\theta \\&\quad \le C\int _{c_0\varepsilon }^{\varphi _0} \int _{r_2}^1 \left( \frac{1}{\varepsilon } e^{-k\frac{\sin \theta }{\varepsilon }} \right) \,r\,\textrm{d}r\,\textrm{d}\theta \le C\varepsilon ; \end{aligned}$$

In $S(r_2,1;\varphi _{12}+\varphi _0,\varphi _{13}-\varphi _0)$, we note that

$$\begin{aligned} u_{test}(r, \theta )-a_1&= \frac{r-r_2}{c_0\varepsilon } \bigg ((u_{test}(r_2,\varphi _{12}+\varphi _0)-a_1)\frac{\varphi _{13}-\varphi _0-\theta }{\alpha _1-2\varphi _0}\nonumber \\&\quad +(u_{test}(r_2,\varphi _{13}-\varphi _0)-a_1)\frac{\theta -(\varphi _{12}+\varphi _0)}{ \alpha _1-2\varphi _0}\bigg ), \end{aligned}$$

(A15)

which implies that

$$\begin{aligned} \int _{\varphi _{12}+\varphi _0}^{\varphi _{13}-\varphi _0}\int _{r_2}^1 \left( \frac{\varepsilon }{2}\left( \left| \frac{\partial u_{test}}{\partial r}\right| ^2+\frac{1}{r^2}\left| \frac{\partial u_{test}}{\partial \theta }\right| ^2\right) +\frac{1}{\varepsilon }W(u_{test}) \right) r\,\textrm{d}r\,\textrm{d}\theta \le Ce^{-\frac{k}{\varepsilon }}\le C\varepsilon . \end{aligned}$$

Therefore, (A14) becomes

$$\begin{aligned} \int _{S(r_2,1;\varphi _{12}-\varphi _0,\varphi _{13}-\varphi _0)} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\le C\varepsilon . \end{aligned}$$

(A16)

Finally, using (A8), (A11), (A12), (A16) we conclude that

$$\begin{aligned} \int _{S(0,1;\varphi _{12}-\varphi _0,\varphi _{13}-\varphi _0)} \left( \frac{\varepsilon }{2}\vert \nabla u_{test}\vert ^2+\frac{1}{\varepsilon }W(u_{test}) \right) \,\textrm{d}z\le \sigma _{12}+ C(W,c_0)\varepsilon . \end{aligned}$$

The energies on $S(0,1;\varphi _{13}-\varphi _0,\varphi _{23}-\varphi _0)$ and $S(0,1;\varphi _{23}-\varphi _0,\varphi _{12}-\varphi _0)$ satisfy the same estimate. Adding them up leads to (20), which completes the proof.

Appendix B: The Minimization Problem (81)

Recall that

$$\begin{aligned}&E(\mu _1,\mu _2,y^*)\\&\quad = \bigg (\left[ \sin {\frac{\alpha _3}{2}}(\sigma _{13}+\sigma _{23})+\left( \mu _2-\mu _1+\sin {\frac{\alpha _2-\alpha _1}{2}}\right) (\sigma _{23}-\sigma _{13})\right] ^2\\&\qquad +\left[ \left( y^*+\cos {\frac{\alpha _3}{2}}\right) (\sigma _{13}+\sigma _{23})\right] ^2\bigg )^{\frac{1}{2}}\\&\qquad +\sigma _{12}\sqrt{(\mu _1+\mu _2)^2+\left( \cos {\frac{\alpha _2-\alpha _1}{2}}-y^*\right) ^2}, \end{aligned}$$

with the domains of $\mu _1,\,\mu _2,\,y^*$ given by

$$\begin{aligned}&\mu _1\in \left[ 0,\sin {\frac{\alpha _3}{2}}+\sin {\frac{\alpha _2-\alpha _1}{2}}-2c_0\varepsilon \right] ,\\&\mu _2\in \left[ 0,\sin {\frac{\alpha _3}{2}}-\sin {\frac{\alpha _2-\alpha _1}{2}}-2c_0\varepsilon \right] ,\\&y_*\in \left[ -\cos {\frac{\alpha _3}{2}}+c_0\varepsilon ,\cos {\frac{\alpha _2-\alpha _1}{2}}-c_0\varepsilon \right] . \end{aligned}$$

First, we prove that $\frac{\partial E}{\partial \mu _2}\ge 0$. It suffices to show that

$$\begin{aligned} \frac{\partial }{\partial \mu _2} \left( \sin {\frac{\alpha _3}{2}}(\sigma _{13}+\sigma _{23})+\left( \mu _2-\mu _1+\sin {\frac{\alpha _2-\alpha _1}{2}}\right) (\sigma _{23}-\sigma _{13}) \right) ^2\ge 0. \end{aligned}$$

Indeed, we have

$$\begin{aligned}&\frac{1}{2}\frac{\partial }{\partial \mu _2} \left( \sin {\frac{\alpha _3}{2}}(\sigma _{13}+\sigma _{23})+\left( \mu _2-\mu _1+\sin {\frac{\alpha _2-\alpha _1}{2}}\right) (\sigma _{23}-\sigma _{13}) \right) ^2\\&\quad =(\sigma _{23}-\sigma _{13})\cdot \left( \sin {\frac{\alpha _3}{2}}(\sigma _{13}+\sigma _{23})+\left( \mu _2-\mu _1+\sin {\frac{\alpha _2-\alpha _1}{2}}\right) (\sigma _{23}-\sigma _{13}) \right) \\&\quad \ge (\sigma _{23}-\sigma _{13})\left( \sin {\frac{\alpha _3}{2}}(\sigma _{13}+\sigma _{23})-\sin {\frac{\alpha _3}{2}}(\sigma _{23}-\sigma _{13}) \right) \\&\quad \ge 2\sigma _{13}\sin {\frac{\alpha _3}{2}}(\sigma _{23}-\sigma _{13})\\&\quad \ge 0, \end{aligned}$$

where we utilized $\sigma _{23}\ge \sigma _{13}$ and $\mu _2-\mu _1+\sin \frac{\alpha _2-\alpha _1}{2}\ge -\sin \frac{\alpha _3}{2}$.

Thus we can assume $\mu _2=0$. Set

$$\begin{aligned} \mu ^*{:}{=}\sin \frac{\alpha _2-\alpha _1}{2}-\mu _1. \end{aligned}$$

By (6) we consider the equivalent minimization problem

$$\begin{aligned} \begin{aligned}&\qquad \min {\ \tilde{E}(\mu ^*,y^*)}, \\ \text { such that }&\mu ^*\in \left[ -\sin {\frac{\alpha _3}{2}}+2c_0\varepsilon ,\sin \frac{\alpha _2-\alpha _1}{2}\right] ,\\&y_*\in \left[ -\cos {\frac{\alpha _3}{2}}+c_0\varepsilon ,\cos {\frac{\alpha _2-\alpha _1}{2}}-c_0\varepsilon \right] , \end{aligned} \end{aligned}$$

(B17)

where

$$\begin{aligned} \tilde{E}(\mu ^*,y^*)&= \left( \left( \sin {\frac{\alpha _3}{2}}(\sin \alpha _1+\sin \alpha _2)+\mu ^*(\sin \alpha _1-\sin \alpha _2)\right) ^2\right. \\&\left. \quad +\left( \left( y^*+\cos {\frac{\alpha _3}{2}}\right) (\sin \alpha _1+\sin \alpha _2)\right) ^2\right) ^{\frac{1}{2}}\\&\quad +\sin \alpha _3\left( \left( \sin \frac{\alpha _2-\alpha _1}{2}-\mu ^*\right) ^2+\left( \cos {\frac{\alpha _2-\alpha _1}{2}}-y^*\right) ^2\right) ^{\frac{1}{2}}. \end{aligned}$$

The objective is to prove that $\tilde{E}$ obtains its minimal value $\sin \alpha _1+\sin \alpha _2+\sin \alpha _3$ if and only if $y^*=\mu ^*=0$.

By (5),

$$\begin{aligned} \sin {\frac{\alpha _1+\alpha _2}{2}}=\sin {\frac{\alpha _3}{2}},\quad \cos {\frac{\alpha _1+\alpha _2}{2}}=-\cos {\frac{\alpha _3}{2}}. \end{aligned}$$

Using this and the Cauchy Schwartz inequality $\sqrt{a^2+b^2}\sqrt{c^2+d^2}\ge ac+bd$, we estimate

$$\begin{aligned}&\tilde{E}(\mu ^*,y^*)\\&\quad \ge \sin ^2{\frac{\alpha _3}{2}}(\sin \alpha _1+\sin \alpha _2)+\mu ^*\sin \frac{\alpha _3}{2}(\sin \alpha _1-\sin \alpha _2)\\&\qquad +\cos \frac{\alpha _3}{2}\left( y^*+\cos \frac{\alpha _3}{2}\right) (\sin \alpha _1+\sin \alpha _2)\\&\qquad \!+\!\sin \alpha _3\left( \left( \sin \frac{\alpha _2-\alpha _1}{2}-\mu ^*\right) \sin \frac{\alpha _2-\alpha _1}{2}+\left( \cos \frac{\alpha _2-\alpha _1}{2}-y^*\right) \cos \frac{\alpha _2-\alpha _1}{2} \!\right) \\&\quad =\sin \alpha _1+\sin \alpha _2+\sin \alpha _3. \end{aligned}$$

The equality holds if and only if

$$\begin{aligned}&\frac{\sin {\frac{\alpha _3}{2}}(\sin \alpha _1+\sin \alpha _2)+\mu ^*(\sin \alpha _1-\sin \alpha _2)}{\left( y^*+\cos \frac{\alpha _3}{2}\right) (\sin \alpha _1+\sin \alpha _2)}=\frac{\sin \frac{\alpha _3}{2}}{\cos \frac{\alpha _3}{2}}, \end{aligned}$$

(B18)

$$\begin{aligned}&\frac{\sin \frac{\alpha _2-\alpha _1}{2}-\mu ^*}{\cos \frac{\alpha _2-\alpha _1}{2}-y^*}=\frac{\sin \frac{\alpha _2-\alpha _1}{2}}{\cos \frac{\alpha _2-\alpha _1}{2}}. \end{aligned}$$

(B19)

Supposing that $y^*\ne 0$, then, by (B19),

$$\begin{aligned} \frac{\mu ^*}{y^*}=\frac{\sin \frac{\alpha _2-\alpha _1}{2}}{\cos \frac{\alpha _2-\alpha _1}{2}}. \end{aligned}$$

Substituting this into (B18) implies

$$\begin{aligned}&\frac{\sin \frac{\alpha _2-\alpha _1}{2}(\sin \alpha _1-\sin \alpha _2)}{\cos \frac{\alpha _2-\alpha _1}{2}(\sin \alpha _1+\sin \alpha _2)}=\frac{\sin \frac{\alpha _3}{2}}{\cos \frac{\alpha _3}{2}}\\&\quad \Rightarrow \tan ^2\frac{\alpha _2-\alpha _1}{2}=\tan ^2\frac{\alpha _3}{2}, \end{aligned}$$

which is impossible since $0\le \frac{\alpha _2-\alpha _1}{2}<\frac{\alpha _3}{2}<\frac{\pi }{2}$. Therefore in order to get the equality it must hold that $y^*=0$. Then by (B19) $\mu ^*=0$. The proof is completed.

$\square$

Appendix C: The Last Inequality in (84)

The objective is to show

$$\begin{aligned}&( \sigma _{12}+\sigma _{13}+\sigma _{23})^2\\&\quad < \left[ \left( \sin {\frac{\alpha _3}{2}}- \sin {\frac{\alpha _2-\alpha _1}{2}}\right) \sigma _{13} +\left( \sin {\frac{\alpha _3}{2}}+ \sin {\frac{\alpha _2-\alpha _1}{2}}\right) \sigma _{23} \right] ^2\\&\qquad +\left[ (\sigma _{13}+\sigma _{23})\left( \cos {\frac{\alpha _3}{2}}+\cos {\frac{\alpha _2-\alpha _1}{2}}\right) \right] ^2 \end{aligned}$$

Or by (6) equivalently,

$$\begin{aligned} ( \sin {\alpha _1}+\sin {\alpha _2}+\sin {\alpha _3})^2&< \bigg [ \left( \sin {\frac{\alpha _3}{2}}- \sin {\frac{\alpha _2-\alpha _1}{2}}\right) \sin {\alpha _2} \\&\quad +\left( \sin {\frac{\alpha _3}{2}}+ \sin {\frac{\alpha _2-\alpha _1}{2}}\right) \sin {\alpha _1} \bigg ]^2 \\&\quad +\left[ (\sin {\alpha _2}+\sin {\alpha _1})\left( \cos {\frac{\alpha _3}{2}}+\cos {\frac{\alpha _2-\alpha _1}{2}}\right) \right] ^2 \end{aligned}$$

We directly compute

$$\begin{aligned}&\left[ \sin {\frac{\alpha _3}{2}}(\sin \alpha _2+\sin \alpha _1)+\sin {\frac{\alpha _2-\alpha _1}{2}}(\sin \alpha _1-\sin \alpha _2) \right] ^2\\&\qquad +\left[ (\sin \alpha _1+\sin \alpha _2)\left( \cos {\frac{\alpha _3}{2}}+\cos {\frac{\alpha _2-\alpha _1}{2}}\right) \right] ^2-( \sin {\alpha _1}+\sin {\alpha _2}+\sin {\alpha _3})^2\\&\quad =4\left( \sin ^2\frac{\alpha _3}{2}\cos \frac{\alpha _2-\alpha _1}{2}+\sin ^2\frac{\alpha _2-\alpha _1}{2}\cos \frac{\alpha ^3}{2}\right) ^2\\&\qquad +4\sin ^2\frac{\alpha _3}{2}\cos ^2\frac{\alpha _2-\alpha _1}{2}\left( \cos \frac{\alpha _3}{2} +\cos \frac{\alpha _2-\alpha _1}{2}\right) ^2\\&\qquad -4\sin ^2\frac{\alpha _3}{2}\left( \cos \frac{\alpha _3}{2} +\cos \frac{\alpha _2-\alpha _1}{2}\right) ^2\\&\quad = 4\left( \sin ^2\frac{\alpha _3}{2}\cos \frac{\alpha _2-\alpha _1}{2}+\sin ^2\frac{\alpha _2-\alpha _1}{2}\cos \frac{\alpha ^3}{2}\right) ^2\\&\qquad -4\sin ^2\frac{\alpha _3}{2}\sin ^2\frac{\alpha _2-\alpha _1}{2}\left( \cos \frac{\alpha _3}{2} +\cos \frac{\alpha _2-\alpha _1}{2}\right) ^2\\&\quad =4\left( \sin ^2\frac{\alpha _3}{2}-\sin ^2\frac{\alpha _2-\alpha _1}{2}\right) ^2\\&\quad >0. \end{aligned}$$

$\square$

Appendix D: Flat Chains and Minimal Partition

We begin with flat chains and then particularize to partitions because it is in this more general setting where the compactness theorem and the monotonicity formula are developed in the literature. Flat chains over a finite coefficient group were introduced by Flemming [15], following Whitney [42, p. 152] and further developed and utilized by White [40, 41].

1.1 D.1 Flat Chains with Coefficients in a Group

Let G be an abelian group with norm $\vert \cdot \vert$, such that $\vert g\vert \ge 0$ with $\vert g \vert =0$ if and only if $g=0$, for all $g\in G$, and $\vert g+h\vert \le \vert g\vert +\vert h\vert$, for all $g,h\in G$. Then $(G,\vert \cdot \vert )$ is a metric space and we will assume that it is complete and separable. In our case G will be a finite group.

Fix $\mathbb {R}^n$ and a compact convex set $\mathbb {K}$ in $\mathbb {R}^n$. For each integer $k\ge 0$, consider the abelian group of all formal finite sums of the form $\sum g_iP_i$, where $g_i\in G$ and where $P_i$ is a k-dimensional oriented compact convex polyhedron in $\mathbb {K}$. We form the quotient group obtained by identifying gP with $-g\tilde{P}$, whenever P and $\tilde{P}$ coincide but have opposite orientations. Also, identify gP and $gP_1+gP_2$, whenever P can be subdivided into $P_1$ and $P_2$. The resulting abelian group $\mathcal {P}_k(\mathbb {K};G)$ is called the group of polyhedral k-chains on $\mathbb {K}$ with coefficients in G. Define the boundary homomorphism $\partial : \mathcal {P}_k\rightarrow \mathcal {P}_{k-1}$ by

$$\begin{aligned} \partial \left( \sum g_iP_i \right) {:}{=}\sum g_i\partial P_i. \end{aligned}$$

Note that any polyhedral k-chain T can be written as a linear combination $\sum _i g_i[P_i]$ of nonoverlapping polyhedra, that is, polyhedra with disjoint interiors. Then, the (Whitney) flat norm of the chain is defined to be

$$\begin{aligned} W(T)=\inf \limits _{Q}\{\mathbb {M}(T-\partial \Omega )+\mathbb {M}(Q)\}, \end{aligned}$$

where the infimum is over all polyhedral $k+1$-chains Q, and $\mathbb {M}$ (for mass) stands for measure. The flat norm makes $\mathcal {P}_k(\mathbb {K;G})$ into a metric space. The completion of this metric space is denoted by $\mathcal {F}_k(\mathbb {K},G)$ and its elements are called flat k-chains in $\mathbb {K}$ with coefficients in G. By uniform continuity, functionals such as the flat norm and operations such as addition and boundary extend in a unique way from polyhedral chains to flat chains. The mass norm in $\mathcal {P}_k(\mathbb {K},G)$ extends to a linear semicontinuous functional in $\mathcal {F}_k(\mathbb {K},G)$.

Suppose that every bounded closed subset of G is compact. A fundamental compactness theorem for flat chains asserts that, given any sequence $T_i\in \mathcal {F}_k(\mathbb {K},G)$ with $\mathbb {M}(T_i)$ and $\mathbb {M}(\partial T_i)$ uniformly bounded, there is a W-convergent subsequence.

1.2 D.2 Flat Chains of Top Dimension

Polyhedral n-chains in $\mathbb {R}^n$ with compact support can be identified with the set of piecewise-constant functions

$$\begin{aligned} g:\mathbb {R}^n\rightarrow G, \end{aligned}$$

that vanish outside a compact convex set $\mathbb {K}$. Here, two functions that differ only on a set of measure zero are regarded as the same. ‘Piecewise constant’ means locally constant except along a finite collection of hyperplanes. The identification is as follows. Any such $T\in \mathcal {F}_k(\mathbb {R}^n;G)$ can be written as

$$\begin{aligned} T=\sum g_i[P_i], \end{aligned}$$

where the $P_i$’s are nonoverlapping and inherit their orientations from $\mathbb {R}^n$. We can associate to T the function

$$\begin{aligned} g:\mathbb {R}^n\rightarrow G,\; \text { with } g(x)={\left\{ \begin{array}{ll} g_i, &{} x \text { is in the interior of }P_i,\\ 0, &{}x\text { is not in the interior of }P_i. \end{array}\right. } \end{aligned}$$

Note that the mass norm of T is equal to the $L^1$ norm of $g(\cdot )$. Also, since there are no nonzero $(n+1)$-chains in $\mathbb {R}^n$, we see from the definition of W that

$$\begin{aligned} W(T)=\mathbb {M}(T)=\int _{\mathbb {R}^n} \vert g(x) \vert \,\textrm{d}x. \end{aligned}$$

Consequently, the W-completion of the polyhedral chains (that is, the flat n-chains) is isomorphic to the $L^1$-completion of the piecewise-constant functions. Thus, the flat n-chains T on $\mathbb {R}^n$ with compact support can be identified with the $L^1_{loc}(\mathbb {R}^n;G)$ functions. The flat chains with $\mathbb {M}(\partial T)<+\infty$ correspond to the sets with finite perimeter (Caccioppoli sets). The BV norm of the function g above gives the perimeter, that is,

$$\begin{aligned} \Vert g\Vert _{BV}=\mathbb {M}(\partial T). \end{aligned}$$

The compactness for flat chains with

$$\begin{aligned} \mathbb {M}(T_n)+\mathbb {M}(\partial T_n)<C \end{aligned}$$

is equivalent in this setup to the compactness of the embedding

$$\begin{aligned} BV(\Omega )\Subset L^1(\Omega ),\quad \text {for}\quad \Omega \text { bounded.} \end{aligned}$$

The lower semicontinuity of $\mathbb {M}(\partial T)$ with respect to the W-norm is equivalent to the lower semicontinuity of the BV norm with respect to $L^1$.

1.3 D.3 Minimizing Partitions: The Group of Surface Tension Coefficients

The purpose next is the introduction of an appropriate group G so that for the flat chain $T=\sum g_iP_i$, where $P_i=A_i$, with $A=\{A_i\}$ a partition of U, there holds

(D20)

Here U is an open set, for example $U=\mathbb {R}^n$, $V\Subset U$, and

$$\begin{aligned} E(A;V)=\sum \sigma _{ij} \mathbb {M}(I_{ij}\cap V), \quad \text {where}\quad I_{ij}{:}{=}\partial A_i\cap \partial A_j. \end{aligned}$$

The partition A is a minimizing N-partition if given $V\Subset U$ and any N-parition $A'$ of U with

$$\begin{aligned} \bigcup \limits _{i=1}^N (A_i\Delta A_i')\Subset V, \end{aligned}$$

we have

$$\begin{aligned} E(A;V)\le E(A';V). \end{aligned}$$

Here $A_i\Delta A_i'=(A_i\cup A_i')\setminus (A_i\cap A_i')$.

First, assume that

$$\begin{aligned} \sigma _{ik}\le \sigma _{ij}+\sigma _{jk},\quad \text {for all}\quad i,j,k. \end{aligned}$$

(D21)

Let G be the free $\mathbb {Z}_2$-module with N generators $f_1,\ldots ,f_N$ (one for each phase). White [40] defines a norm in this group such that

$$\begin{aligned} \vert f_i-f_j\vert =\sigma _{ij}, \end{aligned}$$

and the $\mathbb {Z}_2$-module identifies

$$\begin{aligned} f_{i_1}-f_{j_1}=f_{i_1}+f_{j_1}. \end{aligned}$$

Utilizing this, it is easy to see in calculating $\partial T$, and $\mathbb {M}(\partial T)$, that (D20) holds. In this setup, given a partition of U into N measureable sets $A_1,\ldots ,A_N$, and $\mathbb {K}$ as above, we associate the flat n-chain

$$\begin{aligned} T=[g]_\mathbb {K}, \end{aligned}$$

where $[\cdot ]_\mathbb {K}$ denotes the isomorphism between $L^1_{loc}(\mathbb {R}^n,G)$ functions and flat n-chains with compact support, and

$$\begin{aligned} g(x)={\left\{ \begin{array}{ll} f_i, &{} \text {for}\quad x\in A_i\cap \mathbb {K},\\ 0, &{} \text {for}\quad x\not \in A_i\cap \mathbb {K}. \end{array}\right. } \end{aligned}$$

Note that if the $A_i$’s have piecewise-smooth boundaries, then (D20) holds. More generally, equation (D20) holds whenever the $A_i$’s are Caccioppoli sets, that is, whenever the flat chains have finite mass. Conversely, given any flat n-chain T, we can represent T as

$$\begin{aligned} T=[g]_\mathbb {K}, \end{aligned}$$

where $g\in L^1(U\cap \mathbb {K}; G)$. We note that in White [40] it is shown that the inequalities (D21) are no real restriction, in the sense that if they are violated, then one can define new coefficients $\sigma _{ij}^*$ out of the old, so that the infimum of E coincides with the infimum of $E^*$ (defined by replacing $\sigma _{ij}$ with $\sigma _{ij}^*$). Also, it is noted that (D21) is necessary for E to be linear semicontinuous with respect to the flat norm.

Concerning regularity of the minimizing partition, White [40] states that under strictness of the inequalities (D21), the singular set has dimension at most $n-2$, and therefore almost everywhere regularity holds. Moreover, he states that for the strongest Allard-type regularity theorem to hold, the strict triangle inequalities are both necessary and sufficient.

1.4 D.4 The Monotonicity Formula

The monotonicity formula holds for k-dimensional mass minimizing flat chains and states that $\frac{\mathbb {M}(T\arrowvert B_r(x))}{\omega _kr^k}$ is an increasing function of r, where $\omega _k$ is the volume of the k-dimensional unit ball (p. 43 in White [41]). Hence it also holds for n-dimensional minimizing flat chains that coincide with the minimizing partition.