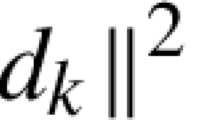Abstract
We present a general framework to study uniqueness, stability and reconstruction for infinite-dimensional inverse problems when only a finite-dimensional approximation of the measurements is available. For a large class of inverse problems satisfying Lipschitz stability we show that the same estimate holds even with a finite number of measurements. We also derive a globally convergent reconstruction algorithm based on the Landweber iteration. This theory applies to nonlinear ill-posed problems such as electrical impedance tomography (EIT), inverse scattering and quantitative photoacoustic tomography (QPAT), under the assumption that the unknown belongs to a finite-dimensional subspace. In particular, we derive Lipschitz stability estimates for EIT with a matrix approximation of the Neumann-to-Dirichlet map; for the inverse scattering problem with measurements of the scattering amplitude at a finite number of directions on \(S^2 \times S^2\); and for QPAT with a low-pass filter of the internal energy.
Similar content being viewed by others
References
Adcock, B., Hansen, A.C.: A generalized sampling theorem for stable reconstructions in arbitrary bases. J. Fourier Anal. Appl. 18(4), 685–716, 2012. https://doi.org/10.1007/s00041-012-9221-x.
Adcock, B., Hansen, A.C.: Generalized sampling and infinite-dimensional compressed sensing. Found. Comput. Math. 16(5), 1263–1323, 2016
Adcock, B., Hansen, A.C., Poon, C.: Beyond consistent reconstructions: optimality and sharp bounds for generalized sampling, and application to the uniform resampling problem. SIAM J. Math. Anal. 45(5), 3132–3167, 2013. https://doi.org/10.1137/120895846.
Adcock, B., Hansen, A.C., Poon, C., Roman, B.: Breaking the coherence barrier: a new theory for compressed sensing. Forum Math. Sigma, 2017. https://doi.org/10.1017/fms.2016.32.
Adler, J., Öktem, O.: Solving ill-posed inverse problems using iterative deep neural networks. Inverse Probl. 33(12), 124007, 2017
Adler, J., Öktem, O.: Learned primal-dual reconstruction. IEEE Trans. Med. Imaging 37(6), 1322–1332, 2018
Alberti, G.S., Arroyo, Á., Santacesaria, M.: Inverse problems on low-dimensional manifolds. arXiv preprint arXiv:2009.00574 (2020)
Alberti, G.S., Capdeboscq, Y.: Lectures on elliptic methods for hybrid inverse problems, Cours Spécialisés [Specialized Courses], vol. 25. Société Mathématique de France, Paris (2018)
Alberti, G.S., Santacesaria, M.: Calderón’s inverse problem with a finite number of measurements. Forum Math. Sigma, 2019. https://doi.org/10.1017/fms.2019.31.
Alberti, G.S., Santacesaria, M.: Calderón’s inverse problem with a finite number of measurements II: independent data. Appl. Anal., 2020
Alberti, G.S., Santacesaria, M.: Infinite dimensional compressed sensing from anisotropic measurements and applications to inverse problems in PDE. Appl. Comput. Harmon. Anal. 50, 105–146, 2021. https://doi.org/10.1016/j.acha.2019.08.002.
Alessandrini, G.: Stable determination of conductivity by boundary measurements. Appl. Anal. 27(1–3), 153–172, 1988
Alessandrini, G., Beretta, E., Vessella, S.: Determining linear cracks by boundary measurements: Lipschitz stability. SIAM J. Math. Anal. 27(2), 361–375, 1996. https://doi.org/10.1137/S0036141094265791.
Alessandrini, G., De Hoop, M.V., Gaburro, R.: Uniqueness for the electrostatic inverse boundary value problem with piecewise constant anisotropic conductivities. Inverse Probl. 33(12), 125013, 2017
Alessandrini, G., de Hoop, M.V., Gaburro, R., Sincich, E.: Lipschitz stability for the electrostatic inverse boundary value problem with piecewise linear conductivities. J. Math. Pures Appl. (9) 107(5), 638–664, 2017
Alessandrini, G., de Hoop, M.V., Gaburro, R., Sincich, E.: Lipschitz stability for a piecewise linear Schrödinger potential from local Cauchy data. Asymptot. Anal. 108(3), 115–149, 2018. https://doi.org/10.3233/asy-171457.
Alessandrini, G., Rondi, L.: Determining a sound-soft polyhedral scatterer by a single far-field measurement. Proc. Am. Math. Soc. 133(6), 1685–1691, 2005
Alessandrini, G., Vessella, S.: Lipschitz stability for the inverse conductivity problem. Adv. Appl. Math. 35(2), 207–241, 2005. https://doi.org/10.1016/j.aam.2004.12.002.
Ambrosetti, A., Prodi, G.: A Primer of Nonlinear Analysis, Cambridge Studies in Advanced Mathematics, vol. 34. Cambridge University Press, Cambridge (1995). Corrected reprint of the 1993 original.
Aronszajn, N.: Theory of reproducing kernels. Trans. Am. Math. Soc. 68, 337–404, 1950. https://doi.org/10.2307/1990404.
Arridge, S., Maass, P., Öktem, O., Schönlieb, C.B.: Solving inverse problems using data-driven models. Acta Numer. 28, 1–174, 2019. https://doi.org/10.1017/S0962492919000059.
Astala, K., Päivärinta, L.: Calderóns inverse conductivity problem in the plane. Ann. Math. 163, 265–299, 2006
Bacchelli, V., Vessella, S.: Lipschitz stability for a stationary 2D inverse problem with unknown polygonal boundary. Inverse Probl. 22(5), 1627, 2006
Bal, G.: Hybrid inverse problems and internal functionals. In: Inverse problems and applications: inside out. II, Math. Sci. Res. Inst. Publ., vol. 60, pp. 325–368. Cambridge Univ. Press, Cambridge (2013)
Bal, G., Jollivet, A., Jugnon, V.: Inverse transport theory of photoacoustics. Inverse Probl. 26(2), 025011, 2010. https://doi.org/10.1088/0266-5611/26/2/025011.
Bal, G., Ren, K.: Multi-source quantitative photoacoustic tomography in a diffusive regime. Inverse Probl. 27(7), 075003, 2011. https://doi.org/10.1088/0266-5611.
Bal, G., Uhlmann, G.: Inverse diffusion theory of photoacoustics. Inverse Probl. 26(8), 085010, 2010
Bao, G., Zhang, H., Zou, J.: Unique determination of periodic polyhedral structures by scattered electromagnetic fields. Trans. Am. Math. Soc. 363(9), 4527–4551, 2011
Barceló, J.A., Luque, T., Pérez-Esteva, S.: Characterization of Sobolev spaces on the sphere. J. Math. Anal. Appl. 491(1), 124240, 2020. https://doi.org/10.1016/j.jmaa.2020.124240.
Bellassoued, M., Jellali, D., Yamamoto, M.: Lipschitz stability for a hyperbolic inverse problem by finite local boundary data. Appl. Anal. 85(10), 1219–1243, 2006. https://doi.org/10.1080/00036810600787873.
Beretta, E., Francini, E.: Lipschitz stability for the electrical impedance tomography problem: the complex case. Commun. Partial Differ. Equ. 36(10), 1723–1749, 2011. https://doi.org/10.1080/03605302.2011.552930.
Beretta, E., Francini, E.: Global Lipschitz stability estimates for polygonal conductivity inclusions from boundary measurements. Appl. Anal., 2020. https://doi.org/10.1080/00036811.2020.1775819.
Beretta, E., Francini, E., Morassi, A., Rosset, E., Vessella, S.: Lipschitz continuous dependence of piecewise constant Lamé coefficients from boundary data: the case of non-flat interfaces. Inverse Probl. 30(12), 125005, 2014. https://doi.org/10.1088/0266-5611/30/12/125005.
Beretta, E., Francini, E., Vessella, S.: Determination of a linear crack in an elastic body from boundary measurements-Lipschitz stability. SIAM J. Math. Anal. 40(3), 984–1002, 2008. https://doi.org/10.1137/070698397.
Beretta, E., Francini, E., Vessella, S.: Uniqueness and Lipschitz stability for the identification of Lamé parameters from boundary measurements. Inverse Probl. Imaging 8, 611, 2014. https://doi.org/10.3934/ipi.2014.8.611.
Beretta, E., de Hoop, M.V., Faucher, F., Scherzer, O.: Inverse boundary value problem for the Helmholtz equation: quantitative conditional Lipschitz stability estimates. SIAM J. Math. Anal. 48(6), 3962–3983, 2016. https://doi.org/10.1137/15M1043856.
Beretta, E., de Hoop, M.V., Francini, E., Vessella, S.: Stable determination of polyhedral interfaces from boundary data for the Helmholtz equation. Commun. Partial Differ. Equ. 40(7), 1365–1392, 2015. https://doi.org/10.1080/03605302.2015.1007379.
Beretta, E., de Hoop, M.V., Francini, E., Vessella, S., Zhai, J.: Uniqueness and Lipschitz stability of an inverse boundary value problem for time-harmonic elastic waves. Inverse Probl. 33(3), 035013, 2017. https://doi.org/10.1088/1361-6420/aa5bef.
Beretta, E., de Hoop, M.V., Qiu, L.: Lipschitz stability of an inverse boundary value problem for a Schrödinger-type equation. SIAM J. Math. Anal. 45(2), 679–699, 2013. https://doi.org/10.1137/120869201.
Blåsten, E., Liu, H.: On corners scattering stably and stable shape determination by a single far-field pattern. Indiana Univ. Math. J. 70, 907–947, 2019
Blåsten, E., Liu, H.: Recovering piecewise constant refractive indices by a single far-field pattern. Inverse Probl. 36(8), 085005, 2020. https://doi.org/10.1088/1361-6420/ab958f.
Borcea, L.: Electrical impedance tomography. Inverse Probl. 18(6), R99–R136, 2002. https://doi.org/10.1088/0266-5611/18/6/201.
Bourgeois, L.: A remark on Lipschitz stability for inverse problems. C. R. Math. 351(5–6), 187–190, 2013
Brauchart, J.S., Dick, J.: A characterization of Sobolev spaces on the sphere and an extension of Stolarskys invariance principle to arbitrary smoothness. Constr. Approx. 38(3), 397–445, 2013. https://doi.org/10.1007/s00365-013-9217-z.
Calderón, A.P.: On an inverse boundary value problem. In: Seminar on Numerical Analysis and Its Applications to Continuum Physics (Rio de Janeiro, 1980), pp. 65–73. Soc. Brasil. Mat., Rio de Janeiro (1980)
Caro, P., García, A., Reyes, J.M.: Stability of the Calderón problem for less regular conductivities. J. Differ. Equ. 254(2), 469–492, 2013. https://doi.org/10.1016/j.jde.2012.08.018.
Caro, P., Rogers, K.M.: Global uniqueness for the Calderón problem with Lipschitz conductivities. In: Forum of Mathematics, Pi, vol. 4. Cambridge University Press (2016)
Cekić, M., Lin, Y.H., Rüland, A.: The Calderón problem for the fractional Schrödinger equation with drift. Calc. Var. Partial. Differ. Equ. 59, 1–46, 2020
Cheney, M., Isaacson, D., Newell, J.C.: Electrical impedance tomography. SIAM Rev. 41(1), 85–101, 1999. https://doi.org/10.1137/S0036144598333613.
Cheng, J., Nakamura, G.: Stability for the inverse potential problem by finite measurements on the boundary. Inverse Probl. 17(2), 273–280, 2001. https://doi.org/10.1088/0266-5611/17/2/307.
Cheng, J., Yamamoto, M.: Uniqueness in an inverse scattering problem within non-trapping polygonal obstacles with at most two incoming waves. Inverse Probl. 19(6), 1361, 2003
Clop, A., Faraco, D., Ruiz, A.: Stability of Calderóns inverse conductivity problem in the plane for discontinuous conductivities. Inverse Probl. Imaging 4(1), 49–91, 2010
Colton, D., Kress, R.: Inverse Aoustic and Electromagnetic Scattering Theory. Applied Mathematical Sciences, vol. 93, 3rd edn. Springer, New York (2013) https://doi.org/10.1007/978-1-4614-4942-3.
Dashti, M., Harris, S., Stuart, A.: Besov priors for Bayesian inverse problems. Inverse Probl. Imaging 6(2), 183–200, 2012. https://doi.org/10.3934/ipi.2012.6.183.
De Vito, E., Mücke, N., Rosasco, L.: Reproducing kernel Hilbert spaces on manifolds: Sobolev and diffusion spaces. Anal. Appl., 2020. https://doi.org/10.1142/S0219530520400114.
Friedman, A., Isakov, V.: On the uniqueness in the inverse conductivity problem with one measurement. Indiana Univ. Math. J. 38(3), 563–579, 1989
Gaburro, R., Sincich, E.: Lipschitz stability for the inverse conductivity problem for a conformal class of anisotropic conductivities. Inverse Probl. 31(1), 015008, 2015. https://doi.org/10.1088/0266-5611/31/1/015008.
Gilbarg, D., Trudinger, N.S.: Elliptic Partial Differential Equations of Second Order. Classics in Mathematics. Springer, Berlin (2001). Reprint of the 1998 edition.
Grasmair, M., Haltmeier, M., Scherzer, O.: Necessary and sufficient conditions for linear convergence of \(\ell ^1\)-regularization. Commun. Pure Appl. Math. 64(2), 161–182, 2011. https://doi.org/10.1002/cpa.20350.
Grasmair, M., Haltmeier, M., Scherzer, O.: The residual method for regularizing ill-posed problems. Appl. Math. Comput. 218(6), 2693–2710, 2011. https://doi.org/10.1016/j.amc.2011.08.009.
Haberman, B.: Uniqueness in Calderóns problem for conductivities with unbounded gradient. Commun. Math. Phys. 340(2), 639–659, 2015
Harrach, B.: Uniqueness and Lipschitz stability in electrical impedance tomography with finitely many electrodes. Inverse Probl. 35(2), 024005, 2019. https://doi.org/10.1088/1361-6420/aaf6fc.
Harrach, B.: Uniqueness, stability and global convergence for a discrete inverse elliptic Robin transmission problem. Numer. Math. 147, 29–70, 2020
Harrach, B., Meftahi, H.: Global uniqueness and Lipschitz-stability for the inverse Robin transmission problem. SIAM J. Appl. Math. 79(2), 525–550, 2019. https://doi.org/10.1137/18M1205388.
Hasanov Hasanoğlu, A., Romanov, V.G.: Introduction to Inverse Problems for Differential Equations. Springer, Cham (2017) https://doi.org/10.1007/978-3-319-62797-7.
de Hoop, M.V., Qiu, L., Scherzer, O.: Local analysis of inverse problems: Hölder stability and iterative reconstruction. Inverse Probl. 28(4), 045001, 2012. https://doi.org/10.1088/0266-5611/28/4/045001.
de Hoop, M.V., Qiu, L., Scherzer, O.: An analysis of a multi-level projected steepest descent iteration for nonlinear inverse problems in Banach spaces subject to stability constraints. Numer. Math. 129(1), 127–148, 2015. https://doi.org/10.1007/s00211-014-0629-x.
Hu, G., Salo, M., Vesalainen, E.: Shape identification in inverse medium scattering problems with a single far-field pattern. SIAM J. Math. Anal. 48(1), 152–165, 2016. https://doi.org/10.1137/15M1032958.
Isakov, V.: Inverse Problems for Partial Differential Equations. Applied Mathematical Sciences, vol. 127, 3rd edn. Springer, Cham (2017) https://doi.org/10.1007/978-3-319-51658-5.
Kaipio, J., Somersalo, E.: Statistical inverse problems: discretization, model reduction and inverse crimes. J. Comput. Appl. Math. 198(2), 493–504, 2007. https://doi.org/10.1016/j.cam.2005.09.027.
Kaltenbacher, B., Neubauer, A., Scherzer, O.: Iterative Regularization Methods for Nonlinear Ill-Posed Problems, Radon Series on Computational and Applied Mathematics, vol. 6. Walter de Gruyter GmbH & Co. KG, Berlin (2008) https://doi.org/10.1515/9783110208276.
Kekkonen, H., Lassas, M., Siltanen, S.: Analysis of regularized inversion of data corrupted by white Gaussian noise. Inverse Probl. 30(4), 045009, 2014. https://doi.org/10.1088/0266-5611/30/4/045009.
Kirsch, A.: An Introduction to the Mathematical Theory of Inverse Problems. Applied Mathematical Sciences, vol. 120, 2nd edn. Springer, New York (2011) https://doi.org/10.1007/978-1-4419-8474-6.
Kuchment, P., Kunyansky, L.: Mathematics of photoacoustic and thermoacoustic tomography. In: Handbook of Mathematical Methods in Imaging, vol. 1, 2, 3, pp. 1117–1167. Springer, New York (2015)
Kuchment, P., Steinhauer, D.: Stabilizing inverse problems by internal data. Inverse Probl. 28(8), 084007, 2012
Lassas, M., Saksman, E., Siltanen, S.: Discretization-invariant Bayesian inversion and Besov space priors. Inverse Probl. Imaging 3(1), 87–122, 2009. https://doi.org/10.3934/ipi.2009.3.87.
Lechleiter, A., Rieder, A.: Newton regularizations for impedance tomography: convergence by local injectivity. Inverse Prob. 24(6), 065009, 2008. https://doi.org/10.1088/0266-5611/24/6/065009.
Li, B.Z., Ji, Q.H.: Sampling analysis in the complex reproducing kernel Hilbert space. Eur. J. Appl. Math. 26(1), 109–120, 2015
Liu, H., Petrini, M., Rondi, L., Xiao, J.: Stable determination of sound-hard polyhedral scatterers by a minimal number of scattering measurements. J. Differ. Equ. 262(3), 1631–1670, 2017. https://doi.org/10.1016/j.jde.2016.10.021.
Lucas, A., Iliadis, M., Molina, R., Katsaggelos, A.K.: Using deep neural networks for inverse problems in imaging: beyond analytical methods. IEEE Signal Process. Mag. 35(1), 20–36, 2018
Mairal, J., Bach, F., Ponce, J.: Task-driven dictionary learning. IEEE Trans. Pattern Anal. Mach. Intell. 34(4), 791–804, 2011
Mandache, N.: Exponential instability in an inverse problem for the Schrödinger equation. Inverse Probl. 17(5), 1435, 2001
McCann, M.T., Jin, K.H., Unser, M.: Convolutional neural networks for inverse problems in imaging: a review. IEEE Signal Process. Mag. 34(6), 85–95, 2017
Mittal, G., Giri, A.K.: Iteratively regularized Landweber iteration method: convergence analysis via Hölder stability. Appl. Math. Comput. 392, 125744, 2021. https://doi.org/10.1016/j.amc.2020.125744.
Mueller, J.L., Siltanen, S.: Linear and Nonlinear Inverse Problems with Practical Applications. Computational Science & Engineering, vol. 10. Society for Industrial and Applied Mathematics (SIAM), Philadelphia (2012) https://doi.org/10.1137/1.9781611972344.
Nachman, A.I.: Global uniqueness for a two-dimensional inverse boundary value problem. Ann. Math. 143, 71–96, 1996
Ongie, G., Jalal, A., Metzler, C.A., Baraniuk, R.G., Dimakis, A.G., Willett, R.: Deep learning techniques for inverse problems in imaging. IEEE J. Sel. Areas Inf. Theory 1(1), 39–56, 2020. https://doi.org/10.1109/JSAIT.2020.2991563.
Rondi, L.: A remark on a paper by Alessandrini and Vessella. Adv. Appl. Math. 36(1), 67–69, 2006
Rüland, A., Sincich, E.: Lipschitz stability for the finite dimensional fractional Calderón problem with finite Cauchy data. Inverse Probl. Imaging 13(5), 1023–1044, 2019
Seidman, T.I.: Nonconvergence results for the application of least-squares estimation to ill-posed problems. J. Optim. Theory Appl. 30(4), 535–547, 1980. https://doi.org/10.1007/BF01686719.
Stefanov, P.: Stability of the inverse problem in potential scattering at fixed energy. Ann. Inst. Fourier (Grenoble) 40(4), 867–884, 1990
Stefanov, P., Uhlmann, G., Vasy, A., Zhou, H.: Travel time tomography. Acta Math. Sin. (Engl. Ser.) 35(6), 1085–1114, 2019. https://doi.org/10.1007/s10114-019-8338-0.
Sylvester, J., Uhlmann, G.: A global uniqueness theorem for an inverse boundary value problem. Ann. Math. 125, 153–169, 1987
Uhlmann, G.: Electrical impedance tomography and Calderóns problem. Inverse Probl. 25(12), 123011, 2009. https://doi.org/10.1088/0266-5611/25/12/123011.
Wang, L.V., Hu, S.: Photoacoustic tomography: in vivo imaging from organelles to organs. Science 335(6075), 1458–1462, 2012. https://doi.org/10.1126/science.1216210.
Yarotsky, D.: Error bounds for approximations with deep ReLU networks. Neural Netw. 94, 103–114, 2017
Acknowledgements
The authors are members of the Gruppo Nazionale per l’Analisi Matematica, la Probabilità e le loro Applicazioni (GNAMPA) of the Istituto Nazionale di Alta Matematica (INdAM). The authors would like to thank Otmar Scherzer for explaining some useful details of [66].
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Additional information
Communicated by C. Le Bris
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
G.S. Alberti is partially supported by the UniGE starting grant “Curiosity”. M. Santacesaria is partially supported by a INdAM—GNAMPA Project 2019. This material is based upon work supported by the Air Force Office of Scientific Research under award number FA8655-20-1-7027.
Appendix: A Local Convergence of Landweber Iteration
Appendix: A Local Convergence of Landweber Iteration
For the sake of completeness, we describe the convergence result used in Section 3.1. We present a simplified version of [66, Theorem 3.2] that is sufficient for our scopes.
Let X and Y be Hilbert spaces, \(A\subseteq X\) be an open set, \(K\subseteq A\) be a compact set and \(F:A\rightarrow Y\) be such that
-
1.
\(F\in C^1(A,Y)\) and \(F':A\rightarrow {\mathcal {L}}_c(X,Y)\) is Lipschitz continuous, namely
$$\begin{aligned}&\Vert F'(x_1)-F'(x_2)\Vert _{X\rightarrow Y}\leqq L\Vert x_1-x_2\Vert _X,\qquad x_1,x_2\in A,\\&\Vert F'(x)\Vert _{X\rightarrow Y}\leqq \hat{L},\qquad x\in A, \end{aligned}$$for some \(L,\hat{L}>0\);
-
2.
\(F^{-1}\) is Lipschitz continuous, namely
$$\begin{aligned} \Vert x_1-x_2\Vert _X \leqq C\Vert F(x_1)-F(x_2)\Vert _Y,\qquad x_1,x_2\in A, \end{aligned}$$for some \(C>0\).
Proposition 2
There exist \(\rho ,\mu >0\) and \(c\in (0,1)\) such that the following is true. Take \(x^{\dagger }\in K\) and let \(y=F(x^{\dagger })\). If \(x_0\in K\) satisfies
then the iterates \((x_k)\) of the Landweber iteration
converge to \(x^{\dagger }\) and satisfy
Remark The constants \(\rho \), c and \(\mu \) are given explicitly in [66] as functions of the a priori data. For instance, the constant \(\rho \), which measures how close to \(x^{\dagger }\) the initial guess \(x_0\) needs to be, may be chosen as
and the step-size \(\mu \) needs to satisfy
The statement provided in [66] requires F to be weakly sequentially closed, but a close look to the proof indicates that this assumption may be dropped, since in this case the existence of the minimizer \(x^{\dagger }\) is granted.
Rights and permissions
About this article
Cite this article
Alberti, G.S., Santacesaria, M. Infinite-Dimensional Inverse Problems with Finite Measurements. Arch Rational Mech Anal 243, 1–31 (2022). https://doi.org/10.1007/s00205-021-01718-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00205-021-01718-4