Detection and isolation of abnormal sub-module in an offshore MMC-HVDC system

Abstract

The cost of downtime and maintenance of any component of the offshore HVDC transmission system is higher than its equivalent onshore one. To prevent this, it is essential to detect any sign of hardware degradation and replace it with a healthy component. This paper presents a new way of detecting any possible deterioration of sub-module capacitance based on the measured feedback on the MMC-HVDC system. Here, the capacitor voltages are estimated using an analytical model and compared with the measured voltages. Initially, the method is verified with an independent sub-module during charging, closed-loop control, and discharge periods. It is then extended to a complete MMC-HVDC system during its operation. The simulation results demonstrate that the proposed method identifies the abnormal sub-module, isolates it, and replaces with a healthy, redundant module, without affecting the normal operation of the MMC-HVDC system.

Appendix

1.1 Derivation of analytical method

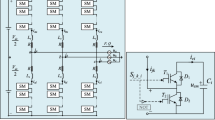
Time domain solution to the state equation is derived using the final and initial values of the states and their derivatives. Derivation for nth order dynamic solution with application to MMC-HVDC system is presented in detail in another work [30], by the present authors. To make this paper self-explanatory, some of the details from this are covered here.

Consider state space matrix of sub-module with 3 states:

$$\dot{{\varvec{X}}}({\varvec{t}})={\varvec{A}}{\varvec{X}}({\varvec{t}})+{\varvec{B}}{\varvec{U}}({\varvec{t}})$$

(19)

There are two sets of initial and final conditions of time domain solution, one set from (19) and the other set from inverse Laplace form. The final solution is obtained by equating both sets of initial conditions. The initial value of first-order derivative is obtained (19) as written as follows:

$$\dot{{\varvec{X}}}\left(0\right)=\frac{d{\varvec{X}}\left({\varvec{t}}\right)}{dt}\boldsymbol{ }\left(t\to 0\right)={\varvec{A}}{\varvec{X}}\left(0\right)+{\varvec{B}}{\varvec{U}}\left(0\right)$$

(20)

Continue to take derivative treating input to be constant and substitute the initial value:

$$\begin{aligned}\ddot{{\varvec{X}}}\left(0\right)&=\frac{{d}^{2}X\left(t\right)}{d{t}^{2}}\left(t\to 0\right) ={\varvec{A}}\left({\varvec{A}}{\varvec{X}}\left(0\right)+{\varvec{B}}{\varvec{U}}\left(0\right)\right)\end{aligned}$$

(21)

$$\begin{aligned}\,\,\,\,\,\,\,\,\,\,={{\varvec{A}}}^{2}{\varvec{X}}\left(0\right)+{\varvec{A}}{\varvec{B}}{\varvec{U}}\left(0\right)\end{aligned}$$

In the steady state, the derivative becomes zero; therefore, final value from (19) for given input remains the same and is given by:

$${\varvec{X}}\left(\boldsymbol{\infty }\right)=-{{\varvec{A}}}^{-1}{\varvec{B}}{\varvec{U}}\left(0\right)$$

(22)

The second set of initial and final values of time domain solution is obtained from inverse Laplace form. Consider time domain solution, either in the eigenvalues (23) or with time constants (24):

$$\left[\begin{array}{c}{x}_{1}\left(T\right)\\ {x}_{2}\left(T\right)\\ {x}_{3}\left(T\right)\end{array}\right]=\left[\begin{array}{c}{c}_{10}\\ {c}_{20}\\ {c}_{30}\end{array}\right]+\left[\begin{array}{ccc}\begin{array}{c}{c}_{11}\\ {c}_{21}\\ {c}_{31}\end{array}& \begin{array}{c}{c}_{21}\\ {c}_{22}\\ {c}_{23}\end{array}& \begin{array}{c}{c}_{31}\\ {c}_{32}\\ {c}_{33}\end{array}\end{array}\right]\left[\begin{array}{c}{exp}^{\left({r}_{1}T\right)}\\ {exp}^{\left({r}_{2}T\right)}\\ {exp}^{\left({r}_{3}T\right)}\end{array}\right]$$

(23)

$$\left[\begin{array}{c}{x}_{1}\left(T\right)\\ {x}_{2}\left(T\right)\\ {x}_{3}\left(T\right)\end{array}\right]=\left[\begin{array}{c}{c}_{10}\\ {c}_{20}\\ {c}_{30}\end{array}\right]+\left[\begin{array}{ccc}\begin{array}{c}{c}_{11}\\ {c}_{21}\\ {c}_{31}\end{array}& \begin{array}{c}{c}_{21}\\ {c}_{22}\\ {c}_{23}\end{array}& \begin{array}{c}{c}_{31}\\ {c}_{32}\\ {c}_{33}\end{array}\end{array}\right]\left[\begin{array}{c}{exp}^{\left(-T/{\tau }_{1}\right)}\\ {exp}^{\left(-T/{\tau }_{2}\right)}\\ {exp}^{\left(-T/{\tau }_{3}\right)}\end{array}\right]$$

(24)

Substituting final value in (24) as t → $\infty$ reduces to:

$$X\left(\infty \right)=\left[\begin{array}{c}{x}_{1}\left(\infty \right)\\ {x}_{2}\left(\infty \right)\\ {x}_{3}\left(\infty \right)\end{array}\right]=\left[\begin{array}{c}{c}_{10}\\ {c}_{20}\\ {c}_{30}\end{array}\right]$$

(25)

Initial condition of (23) obtained by substituting t → $0$, shown as in:

$$\left[\begin{array}{c}{x}_{1}\left(0\right)\\ {x}_{2}\left(0\right)\\ {x}_{3}\left(0\right)\end{array}\right]=\left[\begin{array}{c}{x}_{1}\left(\infty \right)\\ {x}_{2}\left(\infty \right)\\ {x}_{3}\left(\infty \right)\end{array}\right]+\left[\begin{array}{ccc}\begin{array}{c}{c}_{11}\\ {c}_{21}\\ {c}_{31}\end{array}& \begin{array}{c}{c}_{21}\\ {c}_{22}\\ {c}_{23}\end{array}& \begin{array}{c}{c}_{31}\\ {c}_{32}\\ {c}_{33}\end{array}\end{array}\right]\left[\begin{array}{c}1\\ 1\\ 1\end{array}\right]$$

(26)

Initial value of first-order derivative of (23) calculated and simplified to:

$$\frac{d}{dt}\left[\begin{array}{c}{x}_{1}\left(t\to 0\right)\\ {x}_{2}\left(t\to 0\right)\\ {x}_{3}\left(t\to 0\right)\end{array}\right]=\left[\begin{array}{ccc}\begin{array}{c}{c}_{11}\\ {c}_{21}\\ {c}_{31}\end{array}& \begin{array}{c}{c}_{21}\\ {c}_{22}\\ {c}_{23}\end{array}& \begin{array}{c}{c}_{31}\\ {c}_{32}\\ {c}_{33}\end{array}\end{array}\right]\left[\begin{array}{c}{r}_{1}\\ {r}_{2}\\ {r}_{3}\end{array}\right]$$

(27)

Initial value of second-order derivative of (23) calculated from:

$$\frac{{d}^{2}}{{dt}^{2}}\left[\begin{array}{c}{x}_{1}\left(t\to 0\right)\\ {x}_{2}\left(t\to 0\right)\\ {x}_{3}\left(t\to 0\right)\end{array}\right]=\left[\begin{array}{ccc}\begin{array}{c}{c}_{11}\\ {c}_{21}\\ {c}_{31}\end{array}& \begin{array}{c}{c}_{21}\\ {c}_{22}\\ {c}_{23}\end{array}& \begin{array}{c}{c}_{31}\\ {c}_{32}\\ {c}_{33}\end{array}\end{array}\right]\left[\begin{array}{c}{{r}_{1}}^{2}\\ {{r}_{2}}^{2}\\ {{r}_{3}}^{2}\end{array}\right]$$

(28)

Separating coefficients of ${c}_{ij}$ from (26), (27) and (28) and simplifying by sending eigenvalue matrix on right hand side gives:

$$\left[\begin{array}{ccc}\begin{array}{c}{c}_{11}\\ {c}_{21}\\ {c}_{31}\end{array}& \begin{array}{c}{c}_{21}\\ {c}_{22}\\ {c}_{23}\end{array}& \begin{array}{c}{c}_{31}\\ {c}_{32}\\ {c}_{33}\end{array}\end{array}\right]=\left[\begin{array}{ccc}{\varvec{X}}\left(0\right)-{\varvec{X}}\left(\boldsymbol{\infty }\right)\vdots & \dot{{\varvec{X}}}\left(0\right)\vdots & \ddot{{\varvec{X}}}\left(0\right)\end{array}\right]{\varvec{G}}$$

(29)

where ${\varvec{G}}={\left[\begin{array}{ccc}\begin{array}{c}1\\ 1\\ 1\end{array}& \begin{array}{c}{r}_{1}\\ {r}_{2}\\ {r}_{3}\end{array}& \begin{array}{c}{{r}_{1}}^{2}\\ {{r}_{2}}^{2}\\ {{r}_{3}}^{2}\end{array}\end{array}\right]}^{-1}$

The result of (25) and (29) substituted into (23) to obtain the following:

$${\varvec{X}}\left({\varvec{T}}\right)={\varvec{X}}\left(\boldsymbol{\infty }\right)+\left[\begin{array}{ccc}{\varvec{X}}\left(0\right)-{\varvec{X}}\left(\boldsymbol{\infty }\right)\vdots & \dot{{\varvec{X}}}\left(0\right)\vdots & \ddot{{\varvec{X}}}\left(0\right)\end{array}\right].{\varvec{G}}.{\varvec{E}}$$

(30)

where ${\varvec{E}}=\left[\begin{array}{c}{exp}^{\left({r}_{1}T\right)}\\ {exp}^{\left({r}_{2}T\right)}\\ {exp}^{\left({r}_{3}T\right)}\end{array}\right]$

The final values and initial values of the derivative with state space matrices $A$ and $B$ are shown in (20) to (22) or (${\varvec{X}}\left(\boldsymbol{\infty }\right),\boldsymbol{ }\dot{{\varvec{X}}}\left(0\right),\boldsymbol{ }\ddot{{\varvec{X}}}\left(0\right))$. These final values and initial values in (30) are replaced with values from in (20) to (22) to simplify further to:

$$\begin{aligned}&{\varvec{X}}\left({\varvec{T}}\right)={-{\varvec{A}}}^{-1}{\varvec{B}}{\varvec{U}}\left(0\right)\\ &\quad+\left[\begin{array}{cc}{\varvec{X}}\left(0\right){-{\varvec{A}}}^{-1}{\varvec{B}}{\varvec{U}}\left(0\right)\vdots &{\varvec{A}}{\varvec{X}}\left(0\right)+{\varvec{B}}{\varvec{U}}\left(0\right)\end{array}\right]{\varvec{K}}\end{aligned}$$

(31)

where ${\varvec{K}}={\varvec{G}}.{\varvec{E}}$ ${\varvec{E}}=\left[\begin{array}{c}{exp}^{\left({r}_{1}T\right)}\\ {exp}^{\left({r}_{2}T\right)}\\ {exp}^{\left({r}_{3}T\right)}\end{array}\right]$

The above equation is required to separate the coefficients of ${\varvec{X}}\left(0\right)$ and ${\varvec{U}}\left(0\right)$ to form simplified and final solution.

$${\varvec{X}}\left(1\right)={\varvec{X}}\left({\varvec{T}}\right)={\varvec{P}}\boldsymbol{ }{\varvec{X}}\left(0\right)+{\varvec{Q}}\boldsymbol{ }{\varvec{U}}\left(0\right)$$

(32)

The calculated coefficients of ${\varvec{X}}\left(0\right)$ and ${\varvec{U}}\left(0\right)$, ${\varvec{P}}$ and Q respectively are given by:

$$P\left(i,j\right)=A(i,j).K\left(2\right)+ {A}^{2}(i,j).K\left(3\right)\quad {\text{if}}\; i\ne j$$

(33)

$$\quad\quad\quad\,\,\, =K\left(1\right)+A(i,j)K\left(2\right)+{A}^{2}(i,j)K\left(3\right) \quad {\text{if}}\; i=j$$

$$\begin{aligned} Q\left(i,j\right)&=-{B}^{-1}A(i,j)\left(K\left(1\right)-1\right)+B(i,j)K\left(2\right)\\ &\quad +AB(i,j)K\left(3\right)\end{aligned}$$

(34)

The changes in electrical characteristics result in changes in values of matrix elements as matrices A and B derived using circuit parameter R, L and C. The matrices of P and Q are functions of matrices A, B, and K. Any change in parameter R, L and C results in change in the element in matrices of P and Q. The next state value ${\varvec{X}}\left(1\right)$ depends upon the matrices P and Q; therefore, it also reflects the same in the measurement signature. The order of the $X\left(0\right)$ is 3 in this paper.