Abstract
It is known that, when dealing with 50–60 Hz electromagnetic interference problems between power/railway lines and metallic pipelines/telecommunication cables, an important role is played by the soil modeling. To this aim, this paper presents a novel algorithm devoted to the construction of an equivalent two-layer soil model starting from the combined use of data available from different kinds of earth conductivity maps: on one side, the ones related to the geological characteristics relevant to the area of interest and, on the other side, the ones based on radio electric propagation measurements. From these data and by means of a suitable algorithm, the parameters characterizing the equivalent two-layer soil model are obtained.





Similar content being viewed by others
References
IEEE (2000) Std 80-2000: guide for safety in AC substation grounding. IEEE
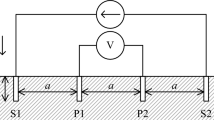
IEEE (2012) Std 81-2012: IEEE guide for measuring earth resistivity, ground impedance, and earth surface potentials of a grounding system. IEEE
EPRI (1982) Report EL-2699: transmission Line Grounding, vol 1, EPRI
Federal Communication Commission (2020) M3 map of effective ground conductivity in the United States for AM broadcast stations. https://www.fcc.gov/media/radio/m3-ground-conductivity-map
ITU-R (2015) Recommendation ITU-R P.832-4: World atlas of ground conductivities. ITU
Morgan RR, Maxwell EL (1965) Omega navigational system conductivity map. Report 54-F-1, Office of Naval Research, Washington
Morgan RR (1968) Worldwide VLF effective conductivity map. Office of Naval Research, Washington
Morgan RR (1968) Preparation of a worldwide VLF Effective conductivity map. Office of Naval Research, Washington
EPRI (2006) Guide for transmission line grounding—a roadmap for design, testing, and remediation: part 1—theory book. EPRI
Tsiamitros DA, Papagiannis GK, Dokopoulos PS (2005) Equivalent Resistivity approximation of two-layer earth structures for earth return impedance calculations. In: 2005 powertech conference proceedings, St. Petersburg, pp 1–7
Tsiamitros DA, Papagiannis GK, Dokopoulos PS (2007) Homogeneous earth approximation of two-layer earth structures: an equivalent resistivity approach. IEEE Trans Power Deliv 22(1):658–666
Paulino JOS, Barbosa CF, Boaventura WC (2012) Modeling two-layers soil for lightning induced-voltage calculation. In: 4th SBSE simposio Brasileiro de sistemas elétricos, Goiânia, Goias, Brasil
Paulino JOS, Barbosa CF, Boaventura WC (2013) An approximate expression for the equivalent resistivity of a two-layer soil. In: 2013 international symposium on lightning protection (XII SIPDA), Belo Horizonte, Brazil
Martins-Britto AG, Lopes F, Rondineau S (2020) Multi-layer earth structure approximation by a homogeneous conductivity soil for ground return impedance calculations. IEEE Trans Power Deliv 35(2):881–891
Martins-Britto AG, Moraes CM, Lopes F, Rondineau S (2020) Low-frequency electromagnetic coupling between a traction line and an underground pipeline in multilayered soil. In: 5th workshop on communication networks and power systems (WCNPS 2020), Brasilia, Brazil
Martins-Britto AG, Moraes CM, Lopes F (2021) Inductive interferences between a 500kV power line and a pipeline with a complex approximation layout and multi-layered soil. Electr Power Syst Res 196
Alipio R, Visacro S (2014) Modeling the frequency dependence of electrical parameters of soil. IEEE Trans Electromagn Compat 56(5):1163–1171
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1
The purpose of Appendix 1 is to give some information about the frequency dependence of the soil conductivity; Alipio and Visacro [17] on the basis of many measurements, proposed the following empirical formula that describes the soil conductivity σ(f) in function of the frequency in the range [100 Hz, 4 MHz]:
where σ(f) is expressed in S/m and σ0 is the value of the conductivity at 100 Hz expressed in S/m. In Fig.
6, we have plotted the value of the ratio σ(f)/σ0 for different values of σ0; we can notice that till to about 10 kHz the value of the ratio is always very close to 1 and increases more rapidly for lower values of σ0 so meaning that soils which are poorly conductive at power frequencies become more conductive at 1 MHz even by a factor 2–3.
One could take into account of (10) in order to evaluate σ1 and D when solving system (7). Table
3 shows some results obtained when considering σgeo constant and when considering it as function of the frequency according to (10) with σgeo = σ0. The quantities σ1AV and DAV are the first layer conductivity and thickness obtained by taking into account of (10).
By looking at Table 3, one can see that by considering σ2 constant or variable in function of the frequency according to (10), has influence mainly on the result of the first layer thickness.
Appendix 2
The scope of Appendix 2 is to justify formula (4).
Starting from formula (2), we may write:
The left-hand side of (11) represents a real and positive number, so the following inequality must hold:
Moreover, in (3) that represents a real positive quantity, the argument of the logarithm must be greater than 1; thus, it has to be:
By combining together (12) and (13), one finally obtains the following inequality:
In order to solve (14), we have to distinguish two cases:
Case 1: σ2 < σe(f).
One can verify that (14) is fulfilled for σ1 > σe(f).
Case 2: σ2 > σe(f).
One can verify (14) is fulfilled for σ1 < σe(f).
Thus, one has the relationships in (4).
Appendix 3
Appendix 3 is devoted to a concise demonstration of conditions (8a) and (8b) able to guarantee the existence of the solution of system (7).
For convenience, let us introduce the expressions that represent the first layer thickness in function of σ1, σ2 and of the measured equivalent conductivity σe at 1 MHz and 10 kHz, respectively:
The solution of system (7) is given by the intersection of the curves (15) and (16) and is represented by the couple (σ1, D) where both σ1 and D must be positive.
Having in mind (4), in order that both the curves (15) and (16) are positive, one of the two following conditions must be fulfilled:
-
I.
σ2 < min(σe1MHz, σe10kHz) AND σ1 > max(σe1MHz, σe10kHz)
or
-
II.
σ2 > max(σe1MHz, σe10kHz) AND σ1 < min(σe1MHz, σe10kHz)
Nevertheless, these two conditions are necessary but not sufficient in order to have the intersection of the curves (15) and (16); in fact, we have the following sub-cases:
Case Ia: σ2 < σe1MHz < σe10kHz.
One can verify that it is always D10kHz(σ1) > D1MHz(σ1) for any value of σ1; for σ1 → ∞ both the curves tend to 0. In this case no intersection exists.
Case Ib: σ2 < σe10kHz < σe1MHz.
Let us put into evidence the following points:
-
the curve D1MHz(σ1) has a vertical asymptote for σ1 = σe1MHz while curve D10kHz(σ1) has a vertical asymptote for σ1 = σe10kHz; being σe10kHz < σe1MHz, one has that in a very small neighborhood at the right of σe1MHz we have that D1MHz(σ1) → ∞ while D10kHz(σ1) has a finite value; this means that D1MHz(σ1) > D10kHz(σ1)
-
the following the relation holds:
$$ \mathop {\lim }\limits_{{\sigma_{1} \to \;\infty }} \quad \frac{{D_{1{\rm MHz}} \left( {\sigma_{1} } \right)}}{{D_{10{\rm kHz}} \left( {\sigma_{1} } \right)}} = \frac{{\sqrt {\sigma_{2} } - \sqrt {\sigma_{e1{\rm MHz}} } }}{{\sqrt {\sigma_{2} } - \sqrt {\sigma_{e10{\rm kHz}} } }}\sqrt {\frac{{f_{2} }}{{f_{1} }}} $$(17)
If the above limit given by (17) is < 1, it means that it exists a particular value σ1* such that D1MHz(σ1) < D10kHz(σ1) for σ1 > σ1*. By taking into account of the first item, we can conclude that: in the interval (σe1MHz, σ1*), one has D1MHz(σ1) > D10kHz(σ1) while in the interval (σ1*, ∞), one has D1MHz(σ1) < D10kHz(σ1).
This implies that the two curves have an intersection; thus, system (7) has solution provided that condition (8b) (which derives from (17) and from (9) with σ2 = σgeo) is fulfilled.
Case IIa: σ2 > σe1MHz > σe10kHz.
One can verify that always D10kHz(σ1) > D1MHz(σ1) for any value of σ1; for σ1 → 0+ both the curves tend to the horizontal asymptote given by (5) with Dasym(104) > Dasym(106). In this case no intersection exists.
Case IIb: σ2 > σe10kHz > σe1MHz.
Let us put into evidence the following points:
-
The curve D1MHz(σ1) has a vertical asymptote for σ1 = σe1MHz while curve D10kHz(σ1) has a vertical asymptote for σ1 = σe10kHz; being σe1MHz < σe10kHz, one has that in a very small neighborhood at the left of σe1MHz we have that D1MHz(σ1) → ∞ while D10kHz(σ1) has a finite value; this means that D1MHz(σ1) > D10kHz(σ1).
-
The following the relation holds:
$$ \mathop {\lim }\limits_{{\sigma_{1} \to \;0^{ + } }} \quad \frac{{D_{10{\rm kHz}} \left( {\sigma_{1} } \right)}}{{D_{1{\rm MHz}} \left( {\sigma_{1} } \right)}} = \frac{{\sqrt {\sigma_{2} } - \sqrt {\sigma_{e10{\rm kHz}} } }}{{\sqrt {\sigma_{2} } - \sqrt {\sigma_{e1{\rm MHz}} } }}\sqrt {\frac{{f_{1} }}{{f_{2} }}} \sqrt {\frac{{\sigma_{e1{\rm MHz}} }}{{\sigma_{e10{\rm kHz}} }}} $$(18)
If the above limit given by (18) is > 1 it means that it exists a particular value σ1* such that D1MHz(σ1) < D10kHz(σ1) for σ1 < σ1*. By taking into account of the first item, we can conclude that: in the interval (σ1*, σe1MHz), one has D1MHz(σ1) > D10kHz(σ1) while in the interval (0, σ1*), one has D1MHz(σ1) < D10kHz(σ1).
This implies that the two curves have an intersection; thus, system (7) has solution provided that condition (8a) (which derives from (18) and from (9) with σ2 = σgeo) is fulfilled.
Rights and permissions
About this article
Cite this article
Lucca, G. Construction of a two-layer soil model from earth conductivity maps. Electr Eng 104, 1349–1359 (2022). https://doi.org/10.1007/s00202-021-01390-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00202-021-01390-7