Skip to main content
SpringerLink
Log in

On Rothschild–Stiglitz as Competitive Pooling

  • Exposita Note
  • Published:
Economic Theory Aims and scope Submit manuscript

Abstract

Dubey and Geanakoplos (Q J Econ 117:1529–1570, 2002) have developed a theory of competitive pooling, which incorporates adverse selection and signaling into general equilibrium. By recasting the Rothschild–Stiglitz model of insurance in this framework, they find that a separating equilibrium always exists and is unique.

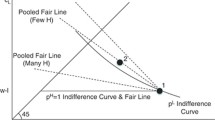
We prove that their uniqueness result is not a consequence of the framework, but rather of their definition of refined equilibria. When other types of perturbations are used, the model allows for many pooling allocations to be supported as such: in particular, this is the case for pooling allocations that Pareto dominate the separating equilibrium.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Dubey P., Geanakoplos J. (2002) Competitive pooling: Rothschild Stiglitz reconsidered. Q J Econ 117, 1529–1570

    Article  Google Scholar 

  • Gale D. (1992) A Walrasian theory of markets with adverse selection. Rev Econ Stud 59, 229–255

    Article  Google Scholar 

  • Gale D. (1996) Equilibria and Pareto optima of markets with adverse selection. Econ Theory 7, 207–235

    Google Scholar 

  • Gale D. (2001) Signaling in markets with two-sided adverse selection. Econ Theory 18, 391–414

    Article  Google Scholar 

  • Hellwig M. (1987) Some recent developments in the theory of competition in markets with adverse selection. Eur Econ Rev 31, 319–325

    Article  Google Scholar 

  • Kohlberg E., Mertens J.F. (1986) On the strategic stability of equilibria. Econometrica 54, 1003–1038

    Article  Google Scholar 

  • Rothschild M., Stiglitz J. (1976) Equilibrium in competitive insurance markets: an essay on the economics of imperfect information. Q J Econ 90, 629–649

    Article  Google Scholar 

  • Selten R. (1975) Reexamination of the perfectness concept for equilibrium points in extensive Games. Int J Game Theory 4, 25–55

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. CREI and Universitat Pompeu Fabra, 25–27 Ramon Trias Fargas, Barcelona, 08005, Spain

    Alberto Martin

Authors
  1. Alberto Martin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alberto Martin.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Martin, A. On Rothschild–Stiglitz as Competitive Pooling. Economic Theory 31, 371–386 (2007). https://doi.org/10.1007/s00199-006-0089-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00199-006-0089-3

Keywords

JEL Classification Numbers

Search

Navigation