1 Introduction

Providing reliable predictions of shock interference phenomena is the key in the design of high-speed vehicles. Shock waves that are generated by the nose of the fuselage may interact with other shocks emanating from the different components of the vehicle, e.g., wings, inlet cowls, etc. The interaction between shock waves often leads to severe heating and pressure loads acting on the surfaces of the vehicle, affecting performance and airworthiness, and may impose additional thermal protection system requirements that increase the weight of the vehicle. The occurrence of shock wave interference can also be encountered in the flow path of the propulsion system of high-speed vehicles, where the flow is heated up before entering the system. At hypervelocity conditions, non-equilibrium physical processes such as finite-rate chemistry and internal energy exchange greatly influence the interaction mechanisms and the aerothermodynamic interactions with the surfaces of the vehicle. The problem of non-equilibrium shock interference has been widely studied in the literature, but due to its complexity and multi-physics nature, it is not yet fully understood. Most of the existing literature focuses on the Earth’s atmosphere, thus only considering air and/or nitrogen flows. However, space missions to Mars have increased the need for better understanding of carbon-dioxide-dominated atmospheres, for which the literature is scarce.

The first systematic study on shock interference mechanisms was carried out by Edney [1], who conducted experiments on the impingement of an oblique shock over a bow shock in front of a cylinder. By changing the location of this intersection, distinct shock interference patterns were identified and classified into six different shock interaction types using a classical shock-polar approach. The pioneering work of Edney was eventually adopted to classify shock interference patterns over other geometries such as the double-wedge case considered in this paper. The latter geometry is particularly relevant since it is found inside the propulsive systems of space vehicles. Furthermore, it represents regions in the proximity of control surfaces and/or the fuselage-canopy area. Inviscid flow over double wedges under the perfect gas assumption was numerically studied by Olejniczak et al. [2] in order to identify the shock interaction patterns resulting from increasing the angle of the second wedge. The transition between type VI and type V (transition types VI–V), as well as the six-shock and seven-shock configurations of type V, was investigated in more detail by Halder et al. [3]. Different possible configurations of the type V interaction, including the transition between a regular reflection and a Mach reflection (RR–MR transition), were numerically explored by Hu et al. [4] by changing the angle of the second wedge. Unsteady behaviour in flows over double wedges was first reported by Ben-Dor et al. [5]. Self-induced oscillations giving rise to extremely high pressure loads were found in the type V shock interaction pattern for a range of angles of the second wedge. The oscillatory wave patterns, associated with the interaction of shock waves with the slip layers emanating from the triple points, were investigated by Hu et al. [4].

In hypersonic flight conditions, temperatures tend to exceed the threshold for triggering real-gas effects, such as thermodynamic relaxation and chemical reactions. An accurate representation of such aerothermodynamic environments can therefore only be achieved by accounting for thermochemical non-equilibrium. Tchuen et al. [6, 7] confirmed the necessity of re-analysing shock interactions with thermochemical non-equilibrium when hypersonic conditions are considered. By comparison with the perfect gas model, it was concluded that real-gas effects significantly influence the shock structure and the surface pressure. In the case of flow of air over a \(15^{\circ }\)–\(45^{\circ }\) double-wedge configuration, it was observed that taking into account non-equilibrium effects caused the interaction pattern to change from a type V to a type VI interaction [6]. A similar tendency was found for a \(15^{\circ }\)–\(60^{\circ }\) configuration, for which the shock layer thickness decreased significantly, even though the wave structure remained the same [7]. Focusing instead on the transition types VI–V, Li et al. [8] also studied the effects of using a real-gas model. It was found that the inclusion of non-equilibrium effects results in a delay of this transition, with respect to the critical value of the second-wedge angle.

Several other studies have focused exclusively on studying shock interactions by accounting for these effects [9,10,11,12,13]. Atkins and Deiterding [13] modelled thermochemical non-equilibrium in an accurate and robust way by coupling the Mutation++ thermochemistry library [14] (Multicomponent Thermodynamic And Transport properties for IONized gases in C++) to an adaptive mesh refinement solver. A double-wedge geometry with a type V interaction pattern was used as a test case to demonstrate the solver’s ability to address non-equilibrium flow features in a highly resolved manner. The RR–MR transition in the type V interaction was studied in detail in Refs. [10,11,12], and, overall, it was concluded that the dominant cause for this transition is the migration and collision of the two triple points.

The previously mentioned studies do not extend into the \(\hbox {CO}_2\) flows of interest in this work. \(\hbox {CO}_2\) is a triatomic molecule showing a rather different behaviour from \(\hbox {N}_2\) and O\(_2\) molecules with respect to the energy redistribution among the internal degrees of freedom. This results in a characteristic vibrational temperature that is lower than in the case of air, with a non-negligible impact on the non-equilibrium processes. Using the two-temperature model developed for air with some extensions and modifications, Candler [15] studied a Martian atmospheric entry flow considering a \(\hbox {CO}_2\)–\(\hbox {N}_2\) composition. Results showed that, due to the very fast vibrational relaxation of \(\hbox {CO}_2\), there is very little thermal non-equilibrium. Additionally, at energies below the threshold for CO dissociation, the flow is nearly in chemical equilibrium and, at higher energies, CO dissociation leads to strong chemical non-equilibrium. The two-temperature non-equilibrium model for \(\hbox {CO}_2\) was developed by Park [16]. The application to a re-entry simulation of a stagnation-line flow confirmed the conclusions drawn by Candler. In Ref. [17], it was shown that comparisons between the two-temperature model for \(\hbox {CO}_2\) and the more detailed state-to-state approach showed a good agreement. Furthermore, validation work was carried out by Liao et al. [18] by comparing experimental measurements of shock stand-off distances over hypersonic spheres in a \(\hbox {CO}_2\) flow to the corresponding numerical simulations. It was concluded that, under the studied conditions, the two-temperature model is applicable.

To the best of the authors’ knowledge, the literature discussing the identification of shock wave interference patterns and the associated aerothermal loads in the case of \(\hbox {CO}_2\)-dominated flows is still not fully consolidated. Windisch et al. [19] simulated the intersection between an oblique shock and a bow shock in front of a cylinder with a \(\hbox {CO}_2\)-dominated flow and observed the type VII interaction. A comparison with the same test case but considering instead a \(\hbox {N}_2\) mixture revealed that the post-shock temperatures were significantly lower for the gas model of the Martian atmosphere, resulting in a higher fluid density and a much smaller shock stand-off distance. Moreover, thermal non-equilibrium was nearly non-existent, as expected. Recently, the authors have taken a first step to study shock interference patterns in \(\hbox {CO}_2\) flows over double wedges [20]. It was shown that, for the simulated conditions, the shock patterns in \(\hbox {CO}_2\) flows differ from the ones obtained for air. Likely due to the much faster vibrational relaxation of \(\hbox {CO}_2\) molecules, lower temperatures and higher densities were obtained, which resulted in smaller shock angles and flow patterns that were more attached to the wall. Further work is required to understand shock interference phenomena in non-equilibrium flows primarily composed of \(\hbox {CO}_2\). The purpose of the present study is to extend our previous work and conduct a systematic study where the impact of the freestream temperature is further assessed. Due to shock/boundary-layer interactions, viscous effects play a large role in this type of flows and can greatly influence the structure of the flow features [6, 7]. The additional length scales introduced by a viscous model make the shock interaction analysis very difficult, and an inviscid model can be a good starting point for the fundamental study of shock interaction patterns with focus on the gas dynamics. Therefore, the non-equilibrium high-Mach-number inviscid flow over a double-wedge configuration is simulated with two different sets of freestream conditions. Shock interference patterns over double wedges when a \(\hbox {CO}_2\)-dominated flow is considered are evaluated and compared to the case of air. Furthermore, in order to better assess non-equilibrium effects, the same simulations are run with a calorically perfect ideal gas model.

The remainder of this paper is organized as follows. The governing equations are presented with emphasis on the physical modelling of finite-rate chemistry and thermal non-equilibrium. Solvers and numerical methods employed in this work are described, as well as validation tests. The computational analysis of flows over double wedges is presented, including a grid convergence study, a study of the shock interference patterns with changes in the freestream temperature and a comparison between different mixtures with a focus on the non-equilibrium effects. Finally, the main conclusions are summarized in the last section.

2 Physical and numerical models

2.1 Physical modelling and governing equations

The system of governing equations is obtained following classical approaches presented in the literature for an inviscid, chemically reacting, non-equilibrium flow [21]. The two-temperature model by Park [22] is used, where the translational energy mode is assumed to be at equilibrium with the rotational one, and the vibrational energy mode is assumed to be at equilibrium with the electronic one. In a compact form, the system can be described as

$$\begin{aligned} \frac{\partial \mathbf {U}}{\partial t} + \nabla \cdot \mathbf {F}(\mathbf {U}) = \mathbf {Q}(\mathbf {U}), \end{aligned}$$
(1)

where the vectors of conservative variables, convective fluxes, and source terms are given by

$$\begin{aligned} {\mathbf {U}} = \left\{ \begin{array}{c} \rho _1 \\ \vdots \\ \rho _{n_\mathrm{s}} \\ \rho \mathbf {u}\\ \rho e \\ \rho e^{\text {ve}} \end{array} \right\} , ~ ~ \mathbf {F} = \left\{ \begin{array}{c} \rho _1 \mathbf {u} \\ \vdots \\ \rho _{n_\mathrm{s}} \mathbf {u} \\ \rho \mathbf {u} \otimes \mathbf {u}+ p {\bar{I}} \\ \rho \mathbf {u} h \\ \rho \mathbf {u} e^{\text {ve}} \end{array} \right\} , ~ ~ {\mathbf {Q}} = \left\{ \begin{array}{c} \dot{\omega }_1 \\ \vdots \\ \dot{\omega }_{n_\mathrm{s}} \\ 0 \\ 0 \\ \dot{\Omega } \end{array} \right\} , \end{aligned}$$
(2)

and \(\mathbf {u}\) is the flow velocity vector, \(\rho \) is the density of the mixture, \(\rho _s\) is the density of species s, p is the static pressure, e and \(e^{\text {ve}}\) are, respectively, the total energy per unit mass and the vibrational energy per unit mass for the mixture, h is the total enthalpy per unit mass, \(\dot{\omega }_s\) is the chemical source energy of species s, index s denotes the \(s{\text {th}}\) chemical species, and \(n_\mathrm{s}\) is the total number of species.

Calculating the non-equilibrium thermodynamic state and source terms is necessary to close the system of governing equations that describes hypersonic flows. This is achieved by means of coupling with appropriate multi-temperature thermochemistry models. The equations presented below describe the implementation of the two-temperature model for a mixture composed of neutral species, provided by the Mutation++ library [14]. Each individual species s is assumed to behave as an ideal gas. Hence, the total pressure of the mixture p is defined by Dalton’s Law as the summation of the partial pressures associated with each species \(p_s\), determined by the ideal gas law,

$$\begin{aligned} p = \sum _{s=1}^{n_\mathrm{s}} p_s = \sum _{s=1}^{n_\mathrm{s}} \rho _s \frac{R_\text {u}}{M_s} T_\text {{tr}}, \end{aligned}$$
(3)

where \(R_\text {u}\) is the universal gas constant, \(M_s\) is the molar mass of species s, and \(T_{\text {tr}}\) is the trans-rotational temperature. The total specific energy of the flow e is given as the sum of the internal and kinetic energies:

$$\begin{aligned} e = \sum _{s=1}^{n_\mathrm{s}} c_s e_s + \frac{1}{2}u^2, \end{aligned}$$
(4)

where \(c_s\) is the mass fraction of species s, u is the magnitude of the flow velocity vector, and \(e_s\) is the specific internal energy of the species, given by the sum of the energy of formation and the contribution of each internal mode (t-translational, r-rotational, v-vibrational, e-electronic),

$$\begin{aligned} e_s = e_s^{\text {t}} \left( T_{\text {tr}}\right) + e_s^{\text {r}} \left( T_{\text {tr}}\right) + e_s^{\text {v}} \left( T_{\text {ve}}\right) + e_s^{\text {e}} \left( T_{\text {ve}}\right) + e_s^0. \end{aligned}$$
(5)

Using a combination of statistical thermodynamics and quantum mechanics, the internal mode energies are defined on the basis of the rigid-rotor harmonic oscillator model (RRHO) as:

$$\begin{aligned} e_s^{\text {t}} \left( T_{\text {tr}}\right)= & {} \frac{3}{2}\frac{R_\text {u}}{M_s}T_{\text {tr}}, \end{aligned}$$
(6)
$$\begin{aligned} e_s^{\text {r}} \left( T_{\text {tr}}\right)= & {} {\left\{ \begin{array}{ll} \frac{R_\text {u}}{M_s}T_{\text {tr}},&{} \text {for linear molecules,} \\ 0, &{} \text {for atoms,} \end{array}\right. } \end{aligned}$$
(7)
$$\begin{aligned} e_s^{\text {v}} \left( T_{\text {ve}}\right)= & {} {\left\{ \begin{array}{ll} \frac{R_\text {u}}{M_s} \sum \limits _{v}\frac{\theta _{v,s}^{\text {v}}}{\exp \left( \theta _{v,s}^{\text {v}}/T_{\text {ve}}\right) -1},&{} \text {for molecules,} \\ 0, &{} \text {for atoms,} \end{array}\right. } \end{aligned}$$
(8)
$$\begin{aligned} e_s^{\text {e}} \left( T_{\text {ve}}\right)= & {} \frac{R_\text {u}}{M_s}\frac{\sum \nolimits _{i} g_{i,s} \theta _{i,s}^{\text {e}} \exp \left( -\theta _{i,s}^\text {e}/T_{\text {ve}}\right) }{\sum \nolimits _{i} g_{i,s} \exp \left( -\theta _{i,s}^{\text {e}}/T_{\text {ve}}\right) }, \end{aligned}$$
(9)

where \(\theta _{v,s}^{\text {v}}\) is the characteristic vibrational temperature of species s and vibrational mode v, \(g_{i,s}\) and \(\theta _{i,s}^{\text {e}}\) are the degeneracy and characteristic electronic temperature, respectively, at energy level i for species s. The formation energy \(e_s^0\) is referenced at the standard state conditions of 298.15 K and 1 atm.

The conservation equation for the mass of the mixture is replaced by a mass conservation equation for each species in the gas, incorporating a production/destruction term that results from chemical activity. The chemical source term \(\dot{\omega }_s\) is given by

$$\begin{aligned} \dot{\omega }_s = M_s \sum _{r=1}^{n_\mathrm{r}} \left( \nu ''_{s,r} - \nu '_{s,r}\right) \left[ k_{\text {f},r} {\displaystyle \prod _{j=1}^{n_\mathrm{s}}} \hat{\rho }_j^{\nu '_{j,r}} - k_{\text {b},r} { \displaystyle \prod _{j=1}^{n_\mathrm{s}}} \hat{\rho }_j^{\nu ''_{j,r}} \right] , \end{aligned}$$
(10)

where \(n_\mathrm{r}\) and \(n_\mathrm{s}\) are, respectively, the number of reactions and the number of species, \(\nu _s'\) is the forward reaction stoichiometry coefficient, \(\nu _s''\) is the backward reaction stoichiometry coefficient, \(\hat{\rho _j}\) is the molar density, \(k_{\text {f},r}\) is the forward reaction rate, and \(k_{\text {b},r}\) is the backward reaction rate. The forward reaction rate for each reaction r is defined according to the modified Arrhenius equation

$$\begin{aligned} k_{\text {f},r} = A_rT_\text {c}^{N_r}\exp \left( {-\frac{\theta _r}{T_\text {c}}}\right) , \end{aligned}$$
(11)

where the coefficients \(A_r\), \(\theta _r\), and \(N_r\) are obtained from experimental data and are, respectively, the reaction rate constant, the activation temperature, and an exponent. The backward reaction rates \(k_{\text {b},r}\) are determined from the equilibrium reaction rates, \(k_{\text {b},r} = k_{\text {f},r}/k_{\text {eq},r}\) for every reaction r. The equilibrium reaction rates \(k_{\text {eq},r}\) are determined as a function of the Gibbs free energy. The temperature \(T_\text {c}\) is the controlling temperature determined by Park’s two-temperature model [22]:

  • Dissociation reactions

    \(T_\text {c} = \sqrt{T_{\text {tr}}T_\text {ve}}\) for the forward rate; \(T_\text {c} = T_{\text {tr}}\) for the backward rate;

  • Exchange reactions

    \(T_{\text {c}}= T_{\text {tr}}\).

In the two-temperature model, the energy transfer mechanisms, that determine the change in vibrational energy of the mixture, are accounted for in the source term vector. The source term \(\dot{\Omega }\) is defined as the sum of the vibrational-to-translational energy transfer and energy exchanges due to chemical activity,

$$\begin{aligned} \dot{\Omega } =\sum _{s=1}^{n_\mathrm{s}} \dot{\Omega }_s^{\text {t-r:v-e}} + \dot{\Omega }_s^{\text {c:v}} + \dot{\Omega }_s^{\text {c:e}}. \end{aligned}$$
(12)

The term \(\dot{\Omega }^{\text {t-r}:\text {v-e}}\) concerns the rate of energy exchange between the translational and vibrational energy modes, following the Landau–Teller model [23]

$$\begin{aligned} \dot{\Omega }_s^{\text {t-r:v-e}} = \rho _s \frac{e^\text {v}_s (T) - e^\text {v}_s \left( T_\text {v}\right) }{\tau _s^{\text {V-T}}}. \end{aligned}$$
(13)

The vibrational relaxation time of each species, \(\tau _s^{\text {V-T}}\), is given by the Millikan and White empirical formula [24] and the Park correction [22],

$$\begin{aligned} \tau ^{\text {V-T}}_{s} = \tau _{s}^{\text {MW}}+\tau _{s}^{\text {P}}, \end{aligned}$$
(14)

where \(\tau _{s}^{\text {MW}}\), the Millikan and White relaxation time of species s, depends on the vibrational relaxation times of the interactions with collision partners r and the corresponding molar fractions \(X_r\), as follows:

$$\begin{aligned} \tau _s^{\text {MW}}= & {} \left( \sum _{r=1}^{n_\mathrm{s}} \frac{X_r}{ \tau _{s-r}^{\text {MW}}}\right) ^{-1}, \end{aligned}$$
(15)
$$\begin{aligned} \tau _{s-r}^{\text {MW}}= & {} \exp \left( A_{s,r} \left( T^{-\frac{1}{3}} - B_{s,r}\right) - 18.42 \right) \left( \frac{p}{101325} \right) ^{-1} [\mathrm{s}].\nonumber \\ \end{aligned}$$
(16)

The Park correction is given by

$$\begin{aligned} \tau _{s}^{\text {P}} = \left( N_s \sigma _s \sqrt{\frac{8R_\text {u}T_{\text {tr}}}{\pi M_s}} \right) ^{-1}, \end{aligned}$$
(17)

where r denotes the \(r{\text {th}}\) species, \(X_r\) is the molar fraction, \(N_s\) is the number density, and \(\sigma _s\) is an effective cross section for vibrational relaxation. The change in vibrational-electronic energy of the mixture due to the production/destruction of species is accounted for in the terms \(\dot{\Omega }_s^{\text {c:v}}\) and \(\dot{\Omega }_s^{\text {c:e}}\), given by

$$\begin{aligned} \dot{\Omega }_s^{\text {c:v}} = c_1 \dot{\omega }_s e_s^{\text {v}}, \dot{\Omega }_s^{\text {c:e}} = \dot{\omega }_s e_s^{\text {e}}. \end{aligned}$$
(18)

The coupling between vibrational energy modes and finite-rate chemistry follows a simple non-preferential dissociation model, for which it is assumed that molecules are destroyed or created at the average vibrational energy of the cell; therefore, \(c_1 = 1\).

2.2 Solvers and numerical methods

The open-source SU2 softwareFootnote 1 suite [25] written in C++ and Python is the basis for this study. It is built specifically for the analysis of partial differential equations (PDEs) and PDE-constrained optimization problems on unstructured meshes with the state-of-the-art numerical methods. The solver is rapidly establishing itself within the open-source community as a prominent software for multi-physics analysis and design, including the simulation of chemically reactive and non-equilibrium flows [20, 26] with the development of SU2-NEMO (Non-Equilibrium MOdels solver). The approach adopted for numerical discretization is based on classical literature on the subject. A finite-volume edge-based formulation is used with the AUSM scheme [27] (advection upstream splitting method) together with MUSCL (monotonic upstream-centred scheme for conservation laws) and standard limiting procedures. A dual time-stepping approach with a second-order backward-difference discretization of the time derivative terms is adopted to address unsteadiness.

The closure of the governing equations for the system of interest is achieved by means of coupling with appropriate thermochemistry models (presented in Sect. 2.1). In this regard, the implementation in the present work extended SU2-NEMO by linking to the Mutation++ libraryFootnote 2 [14] that provides efficient algorithms for the computation of thermodynamic, transport (viscosity, thermal conductivity, and diffusion) and chemical kinetic gas properties. The library has been designed for robustness over a wide range of temperatures and its accuracy in dealing with multi-temperature models, with the following constraints in mind: (1) high fidelity of the physical models, ensuring that the laws of thermodynamics are satisfied and that results are validated against the existing experimental data, (2) low computational cost, (3) a modern, object-oriented, extensible framework, and (4) detailed in-source and user’s guide documentation in order to facilitate model improvement and collaboration.

2.3 Validation of thermochemical non-equilibrium models

Both the SU2 software and the Mutation++ library have been extensively validated [14, 25]. To validate the additional numerical implementation that concerns the link between these two codes, the canonical problem of a zero-dimensional adiabatic heat bath was chosen. This type of test case allows to isolate the vibrational-to-translational energy transfer together with vibration-chemistry coupling from the CFD (computational fluid dynamics) simulation, with the purpose of validating the thermochemical relaxation process.

The case of heat bath with a chemically reacting mixture of standard five-species air (\(\hbox {N}_{2}, \hbox {O}_{2}, \hbox {N}, \hbox {O}, \mathrm{and} \hbox { NO}\)) in thermal non-equilibrium was tested. The initial number density is set to 10\(^{25}\) m\(^3\)/s with molar fractions \(\hbox {N}_2\): 0.79 and O\(_2\): 0.21. The initial translational and vibrational temperatures are 15,000 K and 300 K, respectively. The mechanism of reaction is taken from Park [28, 29], and a non-preferential model is considered. A time step of \(1.0\times 10^{-10}\) s is used in the simulation. Figure 1 shows the evolution of (a) translational and vibrational temperatures and (b) species molar fractions with time. Results obtained with SU2-NEMO are compared with the ones provided by another hypersonic CFD code, the Michigan Aerothermal Navier–Stokes Solver LeMANS [30]. A fair agreement is obtained between the two different codes. A perfect match is obtained for the equilibrium temperatures and molar fractions, whereas a 3% discrepancy of vibrational temperature is observed at its peak value, approximately for the same instant of time at which the largest discrepancies of molar fractions are observed. As expected from a non-preferential dissociation model that is used in both solvers, the maximum in vibrational temperature occurs at an instant of time where \(T_{\text {tr}}=T_{\text {ve}}\). This model assumes that the molecules are destroyed or created at the average vibrational energy of the cell, which causes the vibrational-to-translational energy transfer to govern the thermochemical relaxation process. Mathematically, the vibration-chemistry coupling term \(\dot{\Omega }^{{\text {c:v-e}}}\) is much smaller than the term \(\dot{\Omega }^{\text {t-r:v-e}}\) provided by the Landau–Teller model, for which \(\frac{\mathrm{d}T_\mathrm{ve}}{\mathrm{d}t} = 0\) at the same time that \(T_{\text {tr}}=T_{\text {ve}}\). The slight discrepancies also are expected, since there are some differences in the modelling, namely Arrhenius law coefficients in the reaction mechanism as well as different thermodynamic database and handling of the electronic excitation.

Fig. 1
figure 1

Adiabatic thermal bath simulation for an air five-species mixture. Code-to-code comparison between SU2-NEMO and LeMANS

Full size image

3 Computational analysis

In this section, shock interaction patterns in non-equilibrium flows over double-wedge geometries are discussed for different mixtures and values of the freestream temperature. For the flow of air, a mixture with five species (\(\hbox {N}_2\), O\(_2\), N, O, and NO) is considered, and the reaction mechanism is taken from the widely used references of Park [28, 29]. For the \(\hbox {CO}_2\)–\(\hbox {N}_2\) flow, a mixture with 10 species (C, N, O, C\(_2\), \(\hbox {N}_2\), O\(_2\), CN, CO, NO, and \(\hbox {CO}_2\)) is used, and the reaction mechanism is taken from [16]. Following the approach of Candler [15], only \(\hbox {CO}_2\) and \(\hbox {N}_2\) are considered as initial species. At the conditions studied, ionization is assumed not to be important for the flow features.

Fig. 2
figure 2

Double-wedge geometry, computational domain, and boundary conditions

Full size image

The geometry and computational domain used in the study are illustrated in Fig. 2. Both wedges have the same length, i.e., \(L_1/L_2 = 1\). The first wedge has an angle of \(\theta _1 = 15^{\circ }\), and the second wedge has an angle of \(\theta _2 = 45^{\circ }\). An inviscid wall boundary condition is imposed along the walls, supersonic outflow is used at the exit, and a farfield boundary condition is imposed at the remaining boundary. The freestream conditions are summarized in Table 1. For all simulations, the freestream Mach number and pressure are, respectively, \(M_\infty = 9 \) and \(p_\infty = 390\) Pa. The initial mass fractions are \(Y_{\!\text {N}_2} = 0.77\) and \(Y_{\!\text {O}_2} = 0.23\) for air and \(Y_{\!\text {CO}_2} = 0.95\) and \(Y_{\!\text {N}_2} = 0.05\) for the \(\hbox {CO}_2\)–\(\hbox {N}_2\) flow. It is assumed that the freestream flow is in thermal equilibrium, \(T_{\text {tr},\infty }=T_{\text {ve},\infty }=T_\infty \). Two different values of freestream temperature are simulated: \(T_\infty = 300\) K and \(T_\infty = 1000\) K. The first case aims at simulating the occurrence of shock wave interference in a situation typical of external aerodynamics, in the proximity of control surfaces and/or the fuselage-canopy area, where the flow has been previously heated up by the vehicle nose shock. The higher value of freestream temperature simulates the interference phenomena in the case of internal aerodynamics of a propulsive system, for which the flow undergoes a series of shock waves before entering the system. To better understand the impact of non-equilibrium effects in this study, these simulations were also run using a calorically perfect ideal gas model—with \(\gamma = 1.4\) for air and \(\gamma = 1.29\) for the \(\hbox {CO}_2\)–\(\hbox {N}_2\) mixture. Under the calorically perfect ideal gas assumption, the type of interaction that occurs depends only on the relevant non-dimensional parameters (which for inviscid flow are freestream Mach number \(M_\infty \), wedge length ratio \(L_1/L_2\), specific heat ratio \(\gamma \), wedge angles \(\theta _1\) and \(\theta _2\)) [2], and therefore, only one value of freestream temperature was used, \(T_\infty = 300\) K. The next three subsections present: Sect. 3.1 a grid independence study, Sect. 3.2 numerical results for air and \(\hbox {CO}_2\)–\(\hbox {N}_2\) flows, and Sect. 3.3 the discussion of the results with respect to the non-equilibrium effects and its impact on the shock interaction patterns.

Table 1 Simulation parameters
Full size table

3.1 Grid independence

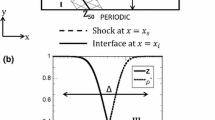
Shock interactions result in complex flow structures that can only be accurately captured using very fine grids. In order to avoid the consequent excessive computational times, adaptive mesh refinement is employed in this work, so that mesh resolution is increased only where it is needed. The baseline two-dimensional grid is an unstructured mesh composed only of triangular elements. Nodes are clustered near the walls and in the region where the interaction is expected to occur. Figure 3 shows the grid independence study for the case of an air five-species mixture with \(T_{\text {tr},\infty }=T_{\text {ve},\infty } = 1000\) K. Results show the effects of different grid sizes on the (a) normalized surface pressure profile as well as (b), (c) normalized translational and vibrational temperatures, respectively, as a function of the normal distance from the wall \(\delta \), at a position on the surface of the second wedge where the most complex flow features are observed. For the scope of this paper, it is considered that grid convergence is achieved, since the discrepancies observed between the two most refined meshes are very small and they have no impact in the flow features associated with different shock patterns. A similar approach is used for all the different cases. For each one of the cases, the most refined grid is chosen. Table 2 shows the characteristic cell size (normalized by the length of the wedge) of the grids that are used in the numerical study. For each grid, the cell size is measured at two different locations of interest for the shock pattern, indicated, later in the paper, in Figs. 4 and 7: (1) shock wave and (2) contact discontinuity

Table 2 Characteristic cell size and number of nodes of final grids (locations 1 and 2 indicated in Figs. 4 and 7)
Full size table
Fig. 3
figure 3

Grid convergence study for the case of an air five-species mixture with \(T_\infty = 1000\, \hbox {K}\)

Full size image

3.2 Shock interaction patterns

Fig. 4
figure 4

Air flow over \(15^{\circ }\)–\(45^{\circ }\) double wedge. Normalized pressure \(p/p_\infty \) (left), numerical schlieren (right); from top to bottom: calorically perfect ideal gas, non-equilibrium with \(T_\infty = 300\, \hbox {K}\), \(T_\infty = 1000\, \hbox {K}\)

Full size image
Fig. 5
figure 5

Air flow over \(15^{\circ }\)–\(45^{\circ }\) double wedge. Translational temperature \(T_\text {tr}\) (left), vibrational temperature \(T_\text {ve}\) (right); from top to bottom: non-equilibrium with \(T_\infty = 300\, \hbox {K}\), \(T_\infty = 1000\, \hbox {K}\)

Full size image

In the first set of test cases, a mixture of air five species is considered. Figure 4 shows the normalized pressure and a numerical schlieren of the flowfield, and Fig. 5 shows translational and vibrational temperatures. Using a calorically perfect ideal gas model, a type V interaction with a nine-shock configuration is obtained. The schematic provided in Fig. 6a is used to analyse the pattern obtained numerically. This interaction is characterized by four triple points. The upper triple point UTP is the intersection between the first oblique shock, the bow shock, and the transmitted shock SW3. The interaction of shock SW3 and the oblique shock generated in the corner between the two wedges—shock SW2—results in a Mach reflection denoted by MS2, which gives rise to the mid-triple point MTP (connecting SW3, MS2, and SW4). The lower triple point LTP concerns what is usually described as a lambda shock interaction, connecting MS2, the oblique shock from the corner between the two wedges SW2 and shock SW5. For the given freestream conditions and geometry, the flow is not able to meet both pressure equilibrium and flow tangency constraints with an incident and a reflected shock at the wall, which results in the reflection of shock SW5 on the surface of the second wedge as a normal shock in a second Mach reflection, giving rise to shock SW6 and the wall triple point WTP. From the upper and mid-triple points, UTP and MTP, respectively, two slip layers, SL1 and SL2 are emitted, bounding a thick contact surface. Furthermore, a third slip layer SL3 is emitted from the lower triple point LTP. Just behind the Mach reflection between the mid-triple and lower triple points, MTP and LTP, respectively, slip layers SL2 and SL3 assemble a converging stream tube. Shock SW4 is reflected on the slip layer SL1 as an expansion wave, turning the slip layer SL1 upward. Further downstream, this expansion wave impinges on the slip layer SL2, which is turned upward and lets the stream tube bounded by SL2 and SL3 diverge. This converging–diverging stream tube is a typical feature of the type V nine-shock configuration. The reflection of shock SW6 on the slip layer SL3 emits an expansion wave that makes SL3 turn upward. The flow between this contact discontinuity and the surface of the second wedge then undergoes a series of alternating isentropic compression and expansion waves, behaving as an underexpanded jet with regard to the adjacent flow region.

For a freestream temperature of \(T_\infty = 300\, \hbox {K}\), a type V interaction with a six-shock configuration is obtained. The type V interaction is characterized by one triple point P, as shown in the schematic illustrated in Fig. 6b. Point P is where the nose shock and the bow shock intersect, from which a transmitted shock PT emerges in a Mach reflection structure. The latter shock interacts with the oblique shock generated by the corner between the two wedges (shock TB), causing shock PT to reflect as shock TU (dark blue to red regions in the normalized pressure plot shown in Fig. 4) and shock TB to reflect as shock TR (light blue to yellow/green regions in the normalized pressure plot shown in Fig. 4) in a regular reflection structure. The resulting peak in surface pressure can be identified in Fig. 9. The reflection of these two shocks from opposite families is one of the key features in the type V six-shock interaction. Downstream of the corner between the two wedges, shock TR undergoes a regular reflection on the surface of the second wedge and gives rise to shock RQ that can be observed in the pressure contours in the transition from light blue to green/yellow, in Fig. 4. Furthermore, a slip layer—evident in the temperature plots shown in Fig. 5—emanates from the triple point P. Shock TU reflects on the slip layer as an expansion wave that, in turn, merges with the reflected shock RQ, which further downstream is reflected by the slip layer as an expansion wave. The two expansion fans can be seen in the normalized pressure plots, where the contours change from yellow to green, light blue, and dark blue.

Fig. 6
figure 6

Schematics of shock interaction patterns obtained in this study

Full size image

As the freestream temperature is raised to \(T_\infty = 1000\, \hbox {K}\), the shock interaction pattern completely changes and a type VI interaction is obtained, with a schematic shown in Fig. 6c. In this type of interaction, the flow is purely supersonic and oblique shock waves originating in the two corners directly intersect in point P, resulting in the appearance of a contact discontinuity that separates the flow that has passed through both shocks from the one that has passed through the second shock BP only. An expansion fan is emitted from the oblique shock intersection and reflected on the surface of the second wedge (transition of contours from red to green and blue). Some of the reflected expansion waves are deflected as they cross the slip layer and intersect with the oblique shock PS, weakening it. The remaining expansion waves are instead reflected by the contact discontinuity as compression waves, recompressing the fluid along the surface of the second wedge (closed light blue pressure contours).

Fig. 7
figure 7

\(\hbox {CO}_2\)–\(\hbox {N}_2\) flow over \(15^{\circ }\)–\(45^{\circ }\) double wedge. Normalized pressure \(p/p_\infty \) (left), numerical schlieren (right); from top to bottom: calorically perfect ideal gas, non-equilibrium with \(T_\infty = 300\, \hbox {K}\), \(T_\infty = 1000\, \hbox {K}\)

Full size image
Fig. 8
figure 8

\(\hbox {CO}_2\)–\(\hbox {N}_2\) flow over \(15^{\circ }\)–\(45^{\circ }\) double wedge. Translational temperature \(T_\text {tr}\) (left), vibrational temperature \(T_\text {ve}\) (right); from top to bottom: non-equilibrium with \(T_\infty = 300\, \hbox {K}\), \(T_\infty = 1000\, \hbox {K}\)

Full size image
Fig. 9
figure 9

Comparison of normalized surface pressure profile

Full size image

In the second set of test cases, a mixture with \(\hbox {CO}_2\), \(\hbox {N}_2\), and eight other species is considered (C, N, O, C\(_2\), \(\hbox {N}_2\), O\(_2\), CN, CO, NO, and \(\hbox {CO}_2\)). Figures 7 and 8 show the shock interaction patterns obtained for this mixture. In this case, except for the non-equilibrium simulation with \(T_\infty = 1000\) K, the patterns obtained numerically are very different from the case of air. For the case of a calorically perfect ideal gas, a type V with a six-shock configuration is obtained. A description of this type of interaction has already been given for the case of non-equilibrium air with \(T_\infty = 300\) K. A supersonic type VI—for which the wave structure has already been described for the case of non-equilibrium air with \(T_\infty = 1000\) K—is obtained for \(T_\infty = 300\) K and \(T_\infty = 1000\) K. The differences in flow features observed between these three cases are relatively small. The shock angles differ slightly, and the interaction pattern is more attached to the wall as the freestream temperature is increased from 300 K to 1000 K in the case of \(\hbox {CO}_2\). These differences can be quantitatively identified in terms of normalized surface pressure in Fig. 9. Despite the disparities in surface pressure just behind the corner shock wave, there is no impact on the shock interaction pattern. The effect of non-equilibrium processes on the flow pressure behind the corner shock is discussed in the next section.

3.3 Non-equilibrium effects

Modelling the flow as a calorically perfect ideal gas does not account for chemical reactions and implies, among other things, that the energies associated with different molecular degrees of freedom are in equilibrium, and thus, only one temperature needs to be considered. However, in hypersonic conditions, thermal and chemical non-equilibrium effects play a significant role and cause the flow features to be significantly influenced by the values of freestream temperature, as shown in this study. With the increase in freestream temperature, the impact of non-equilibrium effects is more pronounced, as the shock interaction pattern differs from the one obtained with a calorically perfect ideal gas model. Overall, the flow becomes warmer, and a larger part of the energy that is transformed across a shock wave, from kinetic to internal, goes towards the excitation of the vibrational degrees of freedom of molecules, potentially up to the point of dissociation. As a result, when non-equilibrium effects are accounted for and as the freestream temperature increases, the shock waves become weaker (this is reflected in overall smaller shock angles or length of normal shocks/Mach stems) despite the pattern observed and independently of the mixture in question. The results obtained numerically are consistent with this trend: for both air and \(\hbox {CO}_2\)–\(\hbox {N}_2\) flows, for a higher freestream temperature, thermal non-equilibrium is stronger just behind the shocks (Figs. 5 and 8). However, vibrational relaxation is faster, which causes the flowfield to be, overall, closer to thermal equilibrium and the shocks to become weaker.

Fig. 10
figure 10

Air flow over \(15^{\circ }\)–\(45^{\circ }\) double wedge. Mass fractions for O (left) and NO (right); \(T_\infty = 300\, \hbox {K}\) (top) and \(T_\infty = 1000\, \hbox {K}\) (bottom)

Full size image
Fig. 11
figure 11

\(\hbox {CO}_2\)–\(\hbox {N}_2\) flow over \(15^{\circ }\)–\(45^{\circ }\) double wedge. Mass fractions for CO (left) and O (right); \(T_\infty = 1000\, \hbox {K}\)

Full size image

Nonetheless, the flow is also extremely sensitive to the atomic and molecular structure and characteristics of the mixture considered, and their impact on thermodynamic properties. Accordingly, for \(T_\infty = 300\, \hbox {K}\), the two mixtures result in completely different shock interaction patterns. For the case of air, looking at Fig. 5, it is interesting to notice that the shock interaction pattern changes significantly, as well as the degree of thermal non-equilibrium and the chemical activity (shown in Fig. 10). In contrast, the shock interaction patterns for the \(\hbox {CO}_2\)–\(\hbox {N}_2\) flows do not change as much. The smaller impact of freestream temperature on the shock interaction patterns of a \(\hbox {CO}_2\)-dominated flow is consistent with the fact that the vibrational characteristic temperature of \(\hbox {CO}_2\) is significantly lower than the one of \(\hbox {N}_2\) and O\(_2\) molecules of the air mixture; therefore, vibrational relaxation is much faster, and overall, the flow is prone to be closer to a state of equilibrium. For the higher value of freestream temperature, \(T_\infty = 1000\, \hbox {K}\), the two mixtures result in the same interaction pattern and very similar shapes. Both flows have a very thin layer (not visible in the case of \(\hbox {CO}_2\)–\(\hbox {N}_2\)) of strong thermal non-equilibrium behind the curved shock, but overall both flowfields are very close to thermal equilibrium.

Figure 10 shows the percentage mass fractions for O and NO species in the case of the air flow, test cases \(T_\infty = 300\) K and \(T_\infty = 1000\) K. Figure 11 shows the percentage mass fractions for CO and O species in the \(\hbox {CO}_2\)–\(\hbox {N}_2\) flow, test case \(T_\infty = 1000\) K. For the remaining test case, \(T_\infty = 300\, \hbox {K}\) with the \(\hbox {CO}_2\)–\(\hbox {N}_2\) mixture, no results are shown since the flow is frozen. For the case of air, as shown in Fig. 10, chemical activity is triggered for \(T_\infty = 300\, \hbox {K}\), with some O\(_2\) and \(\hbox {N}_2\) dissociation, and also NO formation, taking place. As the freestream temperature is increased to \(T_\infty = 1000\, \hbox {K}\), the occurrence of chemical reactions increases substantially—with the maximum mass fraction of O changing from 3.3% to 21.5%, and the maximum mass fraction of NO changing from 0.9% to 7.5%. For \(T_\infty = 1000\) K, it can be observed that NO is almost non-existent in the regions where there is more atomic oxygen and that as it decreases from red to yellow regions in the figure, NO formation begins to occur (blue to orange to red regions). Figure 11 shows the percentage of mass fractions for CO and O species in the case of the \(\hbox {CO}_2\)–\(\hbox {N}_2\) flow, test case \(T_\infty = 1000\) K. Only at this value of freestream temperature, there seems to be enough energy to break the bonds of \(\hbox {CO}_2\) molecules, resulting in a maximum mass fraction of 20.5% of CO and 9.3% of atomic oxygen. However, this energy is not sufficient to trigger CO dissociation, as there are no C atoms in the flowfield. Even though for the higher freestream temperature the shock waves for this mixture become weaker, the maximum pressure behind the oblique shock generated in the corner between the two wedges increases (Fig. 9). As dissociation starts to occur, the density jump across the oblique shock increases, and consequently, the pressure jump increases as well. For air, on the other hand, the shock pattern changes significantly, and the associated maximum pressure decreases considerably.

As explained in Sect. 2.1, a simple non-preferential dissociation model is employed to address the chemical–vibrational coupling. The latter model assumes that molecules can be dissociated from all vibrational states, which may be valid for situations where the freestream kinetic energy per unit mass is much larger than the dissociation energies of the molecules [21]. In preferential dissociation, on the other hand, it is considered that molecules dissociate more easily when vibrationally more excited. Molecules must “ladder climb” from lower to higher vibrational states to be dissociated. As a result, dissociation reactions are delayed, and vibrational temperature tends to be lower during the relaxation process, when compared to non-preferential dissociation [20]. It is likely that using a preferential dissociation model in this study would have had an impact on the results. It is assumed that less dissociation would occur and that, with lower vibrational temperatures, stronger thermal non-equilibrium would be obtained for each case. According to what is observed in this study, that would mean delaying the trend that has been shown as temperature rises. Further studies employing this model would be required to understand whether this would have a significant impact on the shape of the interactions patterns or even change them.

Fig. 12
figure 12

Study of shock interaction patterns in air (red circles) and \(\hbox {CO}_2\)–\(\hbox {N}_2\) (blue circles) flows, with respect to freestream temperature \(T_\infty \)

Full size image

4 Conclusions

A numerical study was performed to understand how shock interference mechanisms in hypersonic flows change when a mixture other than air is considered. A Mach 9 flow over a \(15^{\circ }\)–\(45^{\circ }\) double-wedge geometry was simulated for air and a \(\hbox {CO}_2\)–\(\hbox {N}_2\) flow, and results were compared. Furthermore, to assess the influence of high-temperature effects for different conditions, a study of the shock interaction pattern was conducted with respect to two different values of the freestream temperature: \(T_\infty = 300\, \hbox {K}\) and \(T_\infty = 1000\, \hbox {K}\). Figure 12 summarizes the results obtained in this study. It is shown that the freestream temperature has a greater impact on the shock interaction pattern in the flow of air than in \(\hbox {CO}_2\)-dominated flow. For different values of the freestream temperature, there is a great change in the wave structure for the flow of air, whereas for the \(\hbox {CO}_2\)–\(\hbox {N}_2\) flow the change of pattern does not occur. It is overall observed that, due to the much faster vibrational relaxation of \(\hbox {CO}_2\) molecules, the flows with a \(\hbox {CO}_2\)–\(\hbox {N}_2\) mixture are closer to thermal equilibrium, and the patterns obtained are characterized by weaker shocks. However, at very high temperatures, thermal relaxation is fast in both mixtures and chemical reactions play a significant role. At a freestream temperature of 1000 K, the shock interaction pattern obtained is the same. Future work is looking into including viscous effects in the study, to understand how these patterns change when shock/boundary-layer interaction also plays a role. Employing a preferential dissociation model in this study and assessing its influence could also be considered.