Market dynamics, dynamic resource management and environmental policy in the context of (strong) sustainability

Abstract

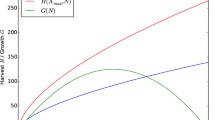
In this paper, we investigate the relationship between market dynamics, dynamic resource management and environmental policy. In contrast to static market entry games, this paper draws attention to the effects of market dynamics on resource dynamics et vice versa, because (1) we show that feedback processes are necessary for obtaining a better understanding of what drives the dynamics between the evolution of common-pool resources and the number of harvesters and more importantly, (2) this analysis provides an environment discussing sustainability in an appropriate inasmuch dynamic way. The paper makes the following points: based on a co-evolutionary model, which incorporates resource and market dynamics simultaneously, it is shown that an increasing number of harvesters does not necessarily imply a lower stock of the common-pool resource in the long run. Further it is shown that a tax-scheme establish an output-sharing solution for coping with the overuse of common-pool resources. This results is in contrast to the prevailing literature, which mainly discusses tax-schemes and out-sharing as substitutes rather than as complements for solving the commons-problem. This conclusion holds even if we additionally assume harvesting-cost-reducing technological progress. On the other side if policy interventions ceased, strong resource sustainability in the sense of resource conservation is not possible, given technological progress is a relevant issue.

Appendix

1.1 Steady states

Table 1 Summary of the derived steady state conditions for system (8)

Proof of the global stability of the obtained steady states of system (8)

Remarks

In this section, we prove the local as well as the global stability of the identified fixed-points of system (8). First, we prove the local stability of the obtained steady states of the non-linear system (8). It is known that for a hyperbolic fixed-point (the real part of the Eigenvalues are nonzero), the flow of the linearized fixed-point is homeomorphic to the non-linear flow (sufficiently close to the fixed-point). Before we proceed, we transform system (8) which consists of three differential equations to a system of two differential equations. We can follow this way because we know that Eq. 5 is a first order autonomous differential equation with the solution given by Eq. 6. Inserting expression (6) into Eq. 8 leads to

$$\label{equ:dynamic2} \left\{\begin{array}{lcl}\dot{N}&=&\xi N\left[1-\displaystyle\frac{N}{M}\right]-\left[\sum_{h}s_{h}f_{h}(N)\right]\\ \dot{s}_{i}&=&s_{i}(1-s_{i})(\pi_{i}-\pi_{j}).\\ \end{array}\right. $$

(17)

We obtain this system assuming C _h  = C _fix,h that is t→ ∞. The Jacobian matrix of system (17) reads as:

$$Jac\equiv\left[\begin{array}{ll}\displaystyle\frac{\partial{\dot{N}}}{\partial N}&\displaystyle\frac{\partial{\dot{N}}}{\partial s_{i}}\\ \displaystyle\frac{\partial{\dot{s_{i}}}}{\partial N}&\displaystyle\frac{\partial{\dot{s_{i}}}}{\partial s_{i}}\\ \end{array}\right]= $$

(18)

$$=\left[\begin{array}{cc}-\xi\left(\displaystyle\frac{N}{M}\right)&-N(e_{i}-e_{j})\\ s_{i}(1-s_{i})\displaystyle\frac{\Theta}{(1+N)N}&(1-2s_{i})\Theta \end{array}\right],$$

(19)

with $\Theta\equiv\frac{N}{N+1}\left[p(e_{i}-e_{j})-e_{i}c_{i}+e_{j}c_{j}\right]=\pi_{i}-\pi_{j}.$ Hence, Θ is the payoff difference between the profit streams of firm i and j.

Proof of Proposition 1

We start with the proof of the local stability of equilibrium A ₁,A ₂,A ₃ and A ₄ by evaluating the Jacobian matrix at A ₁,A ₂,A ₃ and A ₄ each. Table 2 shows the Eigenvalues and their respective signs for each fixed-point.

Table 2 Summary of the derived steady state conditions for system (8)

The sign of the second Eigenvalue χ ₂ for the steady states A ₁,A ₂,A ₃ and A ₄ is clearly determined to be negative due to the fact that N > 0. However, the sign of the first Eigenvalue for A ₁ and A ₂ is not determined ex ante: It could be positive, zero or negative.

If the sign is positive, we obtain a saddle path for A ₁ and A ₂. If χ ₁ and χ ₂ are negative, A ₁ and A ₂ define a stable node. Given the Eigenvalues are zero, we obtain a later line equilibrium because the Jacobian matrix turns out to be singular.

From Proposition 1, it follows that s _i  = 1 which leads to the conclusion that A ₁ and A ₂ are strictly negative because Θ > 0 which stems directly from π _i  > π _j . The first Eigenvalue for the fixed-points A ₃ and A ₄ is clearly negative due to the fact that e _i  > e _j . Therefore, the fixed-points A ₁,A ₂,A ₃ and A ₄ are locally stable.

The next point is to show that the equilibrium points A ₁,A ₂,A ₃ and A ₄ are asymptotically globally stable. This can be done by ruling out closed orbits. Referring to the literature, two important results provide sufficient conditions that rule out the possibility of periodic solutions: the Benedixon’s and Dulac’s criterion, whereas it turns out that the Benedixon’s criterion can be traced back to the Dulac’s criterion as a special case.²⁶

Theorem 1

(Dulac’s criterion) Assume that $\dot{\boldsymbol{x}}=\boldsymbol{f}(\boldsymbol{x})$ is a continuously differentiable vector field, on a simply connected open subset of $\mathcal{R}\times\mathcal{R}$ . If there exists a continuously differentiable, real-valued function $k(\boldsymbol{x})$ with $\{N,s_{i}\}\in\boldsymbol{x}$ such that $\nabla\cdot(k\dot{\boldsymbol{x}})$ has one sign throughout $\mathcal{R}$ , there are no periodic orbits of the autonomous system in $\mathcal{R}$ .

Proof

Assume that there is a closed orbit $\mathcal{C}$ in the simple connected region $\mathcal{R}$. Let $\mathcal{D}$ denote the interior of $\mathcal{C}$. When $\mathcal{C}$ is transversed counterclockwise, Green’s Theorem in the plane gives the following identity:

$$\label{eq:Green}\int\int_{\mathcal{D}}\:\nabla\:\cdot(k\dot{\boldsymbol{x}})\:d\mathcal{D}=\oint_{\mathcal{C}} (k\dot{\boldsymbol{x}}\cdot \boldsymbol{n})\:dl, $$

(20)

where $\boldsymbol{n}$ is the outward normal and dl is the element of arc length along $\mathcal{C}$. The integral on the left hand’s side of Eq. 20 must be nonzero, because $\nabla\:(k\dot{x})$ has one sign in $\mathcal{R}$. The line integral on the right hand side of Eq. 20 equals to zero because $\mathcal{C}$ is a trajectory. Thus, the tangent vector of $\mathcal{C}$, $\dot{\boldsymbol{x}}$, is orthogonal to $\boldsymbol{n}$ which leads to $\dot{\boldsymbol{x}}\boldsymbol{n}=0$. This contradiction implies that no such $\mathcal{C}$ can exist.□

The problem which comes along with applying the Dulac’s and Benedixon’s criterion is that it is sometimes pretty hard determining an appropriate weighting function $k(\boldsymbol{x})$, because there is no algorithm providing such a function. With respect to system (17), we choose $k(\boldsymbol{x})=1$ ²⁷ which, as we will see, is a good choice helping us to provide a sufficient condition for the exclusion of periodic orbits.

Applying Dulac’s criterion, we can write:

$$\label{eq:dulac1} \nabla\cdot(k\dot{\boldsymbol{x}})=\frac{\partial}{\partial s_{i}}\left(k\dot{s_{i}}\right)+\frac{\partial}{\partial N}\left(k\dot{N}\right). $$

(21)

Using the steady state condition s _i  = 1 associated with the steady states A ₁,A ₂,A ₃ and A ₄, we can evaluate expression (21) further as:

$$ \label{eq:dulac2} \nabla\cdot(k\dot{\boldsymbol{x}})=-\frac{\xi N}{M}+(1-2s_{i})\Theta=-\frac{\xi N}{M}-\Theta<0 $$

(22)

Expression (22) turns out to be strictly negative, given N > 0 by assumption and Θ > 0 because of the fact that π _i  > π _j . Hence, $\nabla(k\dot{\boldsymbol{x}})$ does not change sign in $\mathcal{D}$. Since the region s _i  = 1 and N > 0 is simply connected and k(·) and $\boldsymbol{f}(\cdot)$ are smooth, Dulac’s criterion implies that there are no closed orbits in the positive quadrant. Therefore, A ₁,A ₂,A ₃ and A ₄ are asymptotically globally stable.

Proof of Proposition 2

The steady states B ₁ and B ₂ are locally stable which can be seen from Table 3. Please note that Θ turns out to be negative because of π _j  > π _i .

Table 3 Summary of the derived steady state conditions for system (8)

Applying Dulac’s criterion once again, we find with the steady state condition s _i  = 0:

$$ \label{eq:dulac4} \nabla\cdot(k\dot{\boldsymbol{x}})=-\frac{\xi N}{M}+\Theta<0 $$

(23)

which is clearly negative, since Θ < 0. Hence, the fixed-points B ₁ and B ₂ are asymptotically globally stable since the region N > 0 and s _j  = 1 is simply connected and k(·) and $\boldsymbol{f(\cdot)}$ fulfill the smoothness conditions implied by Dulac’s criterion.□

Proof of Proposition 3

Proposition 3 tells that $C_{i}\in(\underline{C}_{i};\overline{C}_{i})$, which implies π _i  = π _j . Further, the condition $C_{i}>\frac{1}{e_{i}}\left[p(e_{i}-e_{j})+e_{j}C_{j}\right]$ must hold. If π _i  = π _j , it follows immediately that Θ = 0. For Θ = 0, the Jacobian becomes singular. Thus, at least one Eigenvalue must be zero which can be seen from Table 4.

Table 4 Summary of the derived steady state conditions for system (8)

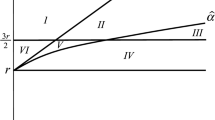
For this constellation, system (17) exhibits an entire line of fixed-points on g(N), whereas the Eigenvector associated with the Eigenvalue zero is the direction vector of the system. We know that one existence condition for D ₁ and D ₂ postulates that Θ = 0. Obviously, any marginally, infinitesimally change of Θ in the positive or negative direction drives the flow of system (17) completely out of these equilibria towards A ₁ or A ₂ ²⁸ or to B ₁ or B ₂.²⁹ In the case of Θ = 0, linearization of system (17) is not sufficient to deduce any information about the dynamic behaviour of the non-linear system (17), because Θ = 0 serves as a cut-off variable which separates saddle path equilibria and nodes.³⁰ Hence, Θ is defined as the so-called fold bifurcation point, because one stable node and one saddle collide and both disappear right at Θ = 0. A necessary condition for fold bifurcation is that det(Jac) = 0 or χ ₁ = 0, which obviously holds for system (17). Because of the fact that tr(Jac) ≠ 0, we call the fold bifurcation equilibrium a degenerate equilibrium.³¹

The center subspace E ^C is the space spanned by the Eigenvector of the linearization with Eigenvalue χ ₁ = 0. Based on the center manifold theorem we know, that this guarantees the existence of a manifold W ^C tangent to E ^C at the origin. In this case, χ ₁ passes through zero at Θ = 0, and given χ ₂ < 0, then W ^C is of dimension one and the evolution on W ^C is $\dot{x}=f(x,\Theta)$. We can conclude, that the fixed points D ₁ and D ₂ are locally saddle-node stable, because χ ₁ = 0, which guarantees a steady-state bifurcation.

If we could further exclude the possibility of closed orbits, obviously no limit circle bifurcation can occur globally. To show this, we use the Poincaré map, together with Dulac’s criterion. As shown before, the steady states A ₁ to A ₄ and B ₁ and B ₂ are globally asymptotically stable. With respect to a Poincaré mapping, this implies that every trajectory from a given starting point converges to the steady state und thus no closed orbits may occur. Referring to Eq. 21 and considering the fact that Θ = 0, we obtain:

$$ \label{eq:dulac3}\nabla\cdot(k\dot{\boldsymbol{x}})=-\frac{\xi N}{M}<0 $$

(24)

which is clearly negative due to the fact that N > 0. Since the region N > 0 and s _i  ∈ (0,1) is simply connected and k(·) and $\boldsymbol{f(\cdot)}$ again fulfill the smoothness conditions implied by Dulac’s criterion, the existence of limit circles can be ruled out. Hence, no limit circle bifurcation can occur globally and thus the steady states D ₁ and D ₂ are saddle-node-stable.□

Time series for s _h , π _h , $\bar{\pi}$ and N