Gravity field error analysis for pendulum formations by a semi-analytical approach

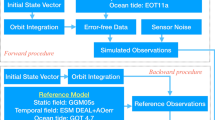
Abstract

Many geoscience disciplines push for ever higher requirements on accuracy, homogeneity and time- and space-resolution of the Earth’s gravity field. Apart from better instruments or new observables, alternative satellite formations could improve the signal and error structure compared to Grace. One possibility to increase the sensitivity and isotropy by adding cross-track information is a pair of satellites flying in a pendulum formation. This formation contains two satellites which have different ascending nodes and arguments of latitude, but have the same orbital height and inclination. In this study, the semi-analytical approach for efficient pre-mission error assessment is presented, and the transfer coefficients of range, range-rate and range-acceleration gravitational perturbations are derived analytically for the pendulum formation considering a set of opening angles. The new challenge is the time variations of the opening angle and the range, leading to temporally variable transfer coefficients. This is solved by Fourier expansion of the sine/cosine of the opening angle and the central angle. The transfer coefficients are further applied to assess the error patterns which are caused by different orbital parameters. The simulation results indicate that a significant improvement in accuracy and isotropy is obtained for small and medium initial opening angles of single polar pendulums, compared to Grace. The optimal initial opening angles are $45^\circ$ and $15^\circ$ for accuracy and isotropy, respectively. For a Bender configuration, which is constituted by a polar Grace and an inclined pendulum in this paper, the behaviour of results is dependent on the inclination (prograde vs. retrograde) and on the relative baseline orientation (left or right leading). The simulation for a sun-synchronous orbit shows better results for the left leading case.

Appendix: Fourier coefficients

The Fourier coefficients of the time-variable opening and central angle can be found numerically (see formula (21)). As an alternative, closed formulas are derived, which enable the determination of the coefficients via complete elliptic integrals based on the distances $\rho _{x,0}$ and $\rho _{y,0}$.

The distance between the two satellites is given by $\rho (t) = \sqrt{\rho _{x}^2+\rho _{y}^2+\rho _z^2}$, where $\rho _z$ is very small compared to the other components. In the planar approximation (see Sect. 3), the distance simplifies to

$$\begin{aligned} \rho = \sqrt{\rho _{x}^2+\rho _{y}^2} = \sqrt{\rho _{x,0}^2+\rho _{y,0}^2\cos ^2 \dot{u} t}, \end{aligned}$$

and the sine and cosine of the opening angle $\delta (t)$ become:

$$\begin{aligned}&\cos \delta (t) := \frac{\rho _{x,0}}{\sqrt{\rho _{x,0}^2+\rho _{y,0}^2\cos ^2 \dot{u}t}} = \sum \limits _{p=-\infty }^{\infty }A_{p} \text {e}^{\mathrm{i} p \dot{u} t}\end{aligned}$$

(28)

$$\begin{aligned}&\sin \delta (t) := \frac{\rho _{y,0}\cos \dot{u} t}{\sqrt{\rho _{x,0}^2+\rho _{y,0}^2\cos ^2 \dot{u}t}} = \sum \limits _{p = -\infty }^{\infty }B_{p} \text {e}^{\mathrm{i} p \dot{u} t}, \end{aligned}$$

(29)

where $\rho _{x,0}$ and $\rho _{y,0}$ are the maximal distortions.

A close inspection leads to the following conclusions:

• All components in the second column are even functions, and so the coefficients can be found by cosine transformation:

  $$\begin{aligned} f&\approx A_0 + \sum \limits _{p=1}^{\infty } \Big (A_{p} \cos p\dot{u}t+A_{-p}\cos (-p\dot{u}t)\Big ).\\ \Rightarrow&A_{p}=\frac{1}{2}\cdot \frac{2}{T}\int \limits _{0}^{T} f(t) \cos p\dot{u} t \mathrm {d}t\quad \quad \text {with } A_{p} = A_{-p}, \end{aligned}$$

  and analogous for $B_{p}$. Compared to standard notation, the coefficients contain the factor $\frac{1}{2}$, to consider positive and negative indexes.

• One revolution of the satellites is equal to a period $T = \frac{2\pi }{\dot{u}}$ for the integration.

• Due to the $\cos ^2$-term in the denominator, the series of $\cos \delta (t)$ is also symmetric for half of the period; hence, it contains only even indexes $p = \{0,\pm 2,\pm 4,\ldots \}$.

• Due to the $\cos$-factor in the numerator, the series of $\sin \delta (t)$ collects only odd indexes $p = \{\pm 1,\pm 3,\pm 5, \ldots \}$.

The Fourier coefficients are derived by the following steps:

• 1. consider the symmetries to reduce the period of integration by a factor of 4; 

• 2. replace $\cos ^2 \dot{u} t$ by $(1-\sin ^2 \dot{u} t);$

• 3. express $\cos (p\dot{u}t)$-terms in powers of $\sin {\dot{u}t};$

• 4. introduce the variable $K = \frac{\rho _{y,0}}{\sqrt{\rho _{x,0}^2+\rho _{y,0}^2}}$, which will be later identified with the module of the elliptic integrals;

• 5. use substitution $\xi = \sin \dot{u} t$ and $\mathrm {d}\xi = \dot{u} \cos \dot{u} t \mathrm {d}t$ to achieve the elliptic integrals in the representation of Jacobi:

  $$\begin{aligned} \mathcal {F}(\phi ,K)&:= \int \limits _{0}^{\sin \phi } \frac{1}{\sqrt{1-K^2\xi ^2}\sqrt{1-\xi ^2}}\mathrm {d}\xi \end{aligned}$$

  (30)
  $$\begin{aligned} \mathcal {E}(\phi ,K)&:= \int \limits _{0}^{\sin \phi } \frac{\sqrt{1-K^2\xi ^2}}{\sqrt{1-\xi ^2}}\mathrm {d}\xi , \end{aligned}$$

  (31)

  which leads to the complete elliptic integrals for $\phi = \frac{\pi }{2}$ (Morse et al. 1972)[pp. 587–626].

The first coefficients of the Fourier series of $\cos {\delta }(t)$ can be calculated by

The subsequent coefficients are calculated by

$$\begin{aligned} A_{2s} = \frac{\dot{u}}{2\pi }\int \limits _{0}^{\frac{2\pi }{\dot{u}}}\frac{\cos (2s\dot{u}t)}{\sqrt{1+\left( \frac{\rho _{y,0}}{\rho _{x,0}}\right) ^2\cos ^2\dot{u}t}} \mathrm {d}t,\quad p = 2s, s\in \mathbb {N}. \end{aligned}$$

To apply the previous steps, in particular the last substitution, it is convenient to express the numerator in polynomials of $\xi = \sin \dot{u} t$. Based on the identity

$$\begin{aligned} \cos nx + \text {i}\sin nx = (\cos x+{\text {i}}\sin x)^n \end{aligned}$$

of de Moivre and the binomial series (Morse et al. 1972 p. 74, p. 10), we find

$$\begin{aligned} \cos (2s\dot{u}t)&=\sum \limits _{j=0}^{s} (-1)^j\left( {\begin{array}{c}2s\\ 2j\end{array}}\right) \sin ^{2j}(\dot{u}t) \overbrace{\cos ^{2s-2j}(\dot{u}t)}^{(1-\sin ^2 \dot{u}t)^{s-j}} \nonumber \\&=\sum \limits _{j=0}^{s}\sum \limits _{h=0}^{s-j} (-1)^{j+h}\left( {\begin{array}{c}2s\\ 2j\end{array}}\right) \left( {\begin{array}{c}s-j\\ h\end{array}}\right) \sin ^{2j+2h}(\dot{u}t). \end{aligned}$$

(32)

With the abbreviation

$$\begin{aligned} R(\xi )&:= \sqrt{1-\xi ^2}\sqrt{1-K^2\xi ^2}, \end{aligned}$$

(33)

the Fourier coefficients are calculated by

$$\begin{aligned} A_{2s}=\frac{2 K}{\pi }\! \frac{\rho _{x,0}}{\rho _{y,0}}\sum \limits _{j=0}^{s}\sum \limits _{h=0}^{s-j} (-1)^{j+h}\left( {\begin{array}{c}2s\\ 2j\end{array}}\right) \left( {\begin{array}{c}s-j\\ h\end{array}}\right) \! \int \limits _{0}^{1} \frac{ \xi ^{2(j+h)} }{R(\xi )} \mathrm {d}\xi .\nonumber \\ \end{aligned}$$

(34)

Fig. 18

Coefficients and Fourier synthesis for an initial opening angle of $\delta _0 = 45^\circ$, inclination of $I = 90^\circ$ and orbital height of $h = 335\,\mathrm{km}$. The left column is the Fourier coefficients for time series $\cos \delta (t)$, $\sin \delta (t)$ and $\sin \eta (t)$. The middle column is the reconstructions with different orders for $\cos \delta (t)$, $\sin \delta (t)$ and $\sin \eta (t)$ in one revolution. The right column is the reconstruction errors

The remaining integral can be expressed by a combination of complete elliptic integrals and polynomials. To find a recursion formula, the auxiliary function $g(\xi ):=\xi ^{2n-1}R(\xi )$ with $n\ge 1$ is differentiated:

$$\begin{aligned}&\frac{\mathrm {d}\{\xi ^{2n-1}\sqrt{1-\xi ^2}\sqrt{1-K^2\xi ^2}\}}{\mathrm {d}\xi } \\&= (2n-1)\xi ^{2n-2}\sqrt{1-\xi ^2}\sqrt{1-K^2\xi ^2}\\&\quad - \frac{\xi ^{2n}\sqrt{1-K^2\xi ^2}}{\sqrt{1-\xi ^2}} - \sqrt{1-\xi ^2}\frac{{K^2 \xi ^{2n}}}{\sqrt{1-K^2\xi ^2}}\\&= \frac{(2n-1)\xi ^{2n-2}(1-K^2\xi ^2-\xi ^2+K^2\xi ^4)}{\sqrt{1-\xi ^2}\sqrt{1-K^2\xi ^2}} \\&\quad -\frac{ \xi ^{2n}(1-K^2\xi ^2) + (1-\xi ^2){K^2 \xi ^{2n}}}{\sqrt{1-\xi ^2}\sqrt{1-K^2\xi ^2}}\\&= \frac{[(2n+1)K^2]\xi ^{2n+2}+[2n(-K^2-1)]\xi ^{2n}}{R(\xi )} \\&\quad +\frac{\xi ^{2n-2}[(2n-1)]}{R(\xi )}\\&=\underbrace{(2n+1)K^2}_{a_0(K)}\frac{\xi ^{2n+2}}{R(\xi )}+\underbrace{[2n(-K^2-1)]}_{a_1(K)}\frac{\xi ^{2n}}{R(\xi )}\\&\quad +\underbrace{[(2n-1)]}_{_{a_2(K)}}\frac{\xi ^{2n-2}}{R(\xi )}. \end{aligned}$$

Dividing by $a_0(K)$ and considering $\sin \frac{\pi }{2} = 1$ leads to

$$\begin{aligned} \left[ \frac{\xi ^{2n-1}{R(\xi )}}{a_0}\right] _0^{1}= & {} \int \limits _{0}^{1} \frac{ \xi ^{2n+2} }{R(\xi )} \mathrm {d}\xi +\frac{a_1(K)}{a_0(K)}\int \limits _{0}^{1} \frac{ \xi ^{2n} }{R(\xi )} \mathrm {d}\xi \nonumber \\&+\frac{a_2(K)}{a_0(K)}\int \limits _{0}^{1} \frac{ \xi ^{2n-2} }{R(\xi )} \mathrm {d}\xi , \end{aligned}$$

(35)

where the left-hand side is zero for the given limits.

In other words, we find a three-term recursion

$$\begin{aligned} \int \limits _{0}^{1} \frac{ \xi ^{2n+2} }{R(\xi )} \mathrm {d}\xi= & {} \frac{2n(K^2+1)}{(2n+1)K^2}\int \limits _{0}^{1} \frac{ \xi ^{2n} }{R(\xi )} \mathrm {d}\xi \nonumber \\&- \frac{2n-1}{(2n+1)K^2}\int \limits _{0}^{1} \frac{ \xi ^{2n-2} }{R(\xi )} \mathrm {d}\xi \end{aligned}$$

(36)

with the initial values

$$\begin{aligned} \int \limits _{0}^{1} \frac{ \xi ^{0} }{R(\xi )} \mathrm {d}\xi = \int \limits _{0}^{\sin \frac{\pi }{2}} \frac{1}{\sqrt{1-K^2\xi ^2}\sqrt{1-\xi ^2}}\mathrm {d}\xi = \mathcal {F}\left( \frac{\pi }{2},K\right) \end{aligned}$$

(37)

and

$$\begin{aligned} \int \limits _{0}^{1} \frac{\xi ^{2}}{R(\xi )} \mathrm {d}\xi = \frac{1}{K^2}\left[ \mathcal {F}\left( \frac{\pi }{2},K\right) - \mathcal {E}\left( \frac{\pi }{2},K\right) \right] . \end{aligned}$$

(38)

The coefficients of $\sin \delta (t)$ are found by the integrals

$$\begin{aligned} B_{2s+1} = \frac{\dot{u}}{2\pi }\left( \frac{\rho _{y,0}}{\rho _{x,0}}\right) \int \limits _{0}^{\frac{2\pi }{\dot{u}}}\frac{\cos \dot{u}t\cos ((2s+1)\dot{u}t)}{\sqrt{1+\left( \frac{\rho _{y,0}}{\rho _{x,0}}\right) ^2\cos ^2\dot{u}t}} \mathrm {d}t, \end{aligned}$$

with $p = 2s+1,s\in \mathbb {N}$.

Appling again the identities of de Moivre and the binomial series leads to

$$\begin{aligned} \cos \dot{u}t \cdot \cos ((2s+1)\dot{u}t)= & {} \sum \limits _{j=0}^{s}\sum \limits _{h=0}^{s-j+1} (-1)^{j+h}\left( {\begin{array}{c}2s+1\\ 2j\end{array}}\right) \\&\cdot \left( {\begin{array}{c}s-j+1\\ h\end{array}}\right) \sin ^{2j+2h}(\dot{u}t) \end{aligned}$$

and the coefficients

$$\begin{aligned} B_{2s+1}= & {} \frac{2 K}{\pi }\sum \limits _{j=0}^{s}\sum \limits _{h=0}^{s-j+1} (-1)^{j+h}\left( {\begin{array}{c}2s+1\\ 2j\end{array}}\right) \left( {\begin{array}{c}s-j+1\\ h\end{array}}\right) \\&\cdot \left\{ \int \limits _0^{1}\frac{\xi ^{2(j+h)}}{R(\xi )} \mathrm {d}\xi \right\} , \end{aligned}$$

where the remaining integral is solved with the same 3-term-recursion (36).

Analogously, the closed formula for the Fourier coefficients for the sine of half of the central angle $\eta (t)$ can be derived.

From Fig. 4, the relation between the central angle and the inter-satellite distance $\rho = \sqrt{\rho _x^2+\rho _y^2}$ can be gathered and the relevant projection $\sin \eta (t)$ can be developed in a Fourier series

$$\begin{aligned} \sin \eta (t) = \frac{1}{2r}\sqrt{\rho _{x,0}^2+\rho _{y,0}^2\cos ^2 \dot{u} t} = \sum \limits _{p = -\infty }^{\infty } C_{p} \text {e}^{\mathrm{i} p \dot{u} t}. \end{aligned}$$

The term $\sin \eta$ is also an even function, such that the coefficients can be found by the cosine transformation and only even indexes remain. Now, the same strategy can be applied to derive the closed forms of the Fourier coefficients

The subsequent coefficients are calculated by

$$\begin{aligned} C_{2s} = \frac{\dot{u}}{2\pi } \int \limits _{0}^{\frac{2\pi }{\dot{u}}} \frac{1}{2r} \sqrt{\rho _{x,0}^2+\rho _{y,0}^2\cos ^2 \dot{u} t} \cos 2s\dot{u} t\mathrm {d}t, \end{aligned}$$

with $p =2s, s\in \mathbb {N}$.

Table 2 Amount of coefficients for a threshold level of $1\,\%$

Applying the means of the previous substitution and the equation (32), we obtain finally

$$\begin{aligned} C_{2s}&= \frac{\rho _{y,0}}{\pi Kr} \sum \limits _{j=0}^{s}\sum \limits _{h=0}^{s-j}(-1)^{j+h}\left( {\begin{array}{c}2s\\ 2j\end{array}}\right) \left( {\begin{array}{c}s-j\\ h\end{array}}\right) \nonumber \\&\quad \cdot \left\{ \int \limits _{0}^{1} \frac{ \xi ^{2(j+h)}}{R(\xi )}\mathrm {d}\xi - k^2 \int \limits _{0}^{1}\frac{ \xi ^{2(j+h+1)}}{R(\xi )}\mathrm {d}\xi \right\} . \end{aligned}$$

The remaining integrals can again be solved by the three-term recursion (36) in terms of the complete elliptic integrals.

The Fourier coefficients $A_{2s}$, $B_{2s+1}$ and $C_{2s}$, the reconstruction of different truncation levels $P=2s_{\max }$ ($P=2s_{\max }+1$) for the time series $\cos \delta (t)$, $\sin \delta (t)$ and $\sin \eta (t)$ and the reconstruction errors are displayed in Fig. 18 for the pendulum with the initial opening angle $\delta _0=45^\circ$.

The amounts of elements for the series expansions up to a threshold level of $1 \%$ are summarized in Table 2. As it can be seen, the convergence of the Fourier series expansion is quite fast, but decreases for larger initial opening angles.