EVA: GPS-based extended velocity and acceleration determination

Abstract

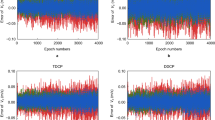
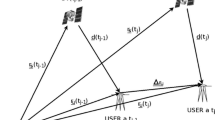
In this work, a new GPS carrier phase-based velocity and acceleration determination method is presented that extends the effective range of previous techniques. The method is named ‘EVA’, and may find applications in fields such as airborne gravimetry when rough terrain or water bodies make difficult or impractical to set up nearby GPS reference receivers. The EVA method is similar to methods such as Kennedy (Precise acceleration determination from carrier phase measurements. In: Proceedings of the 15th international technical meeting of the satellite division of the Institute of Navigation. ION GPS 2002, Portland pp 962–972, 2002b) since it uses L1 carrier phase observables for velocity and acceleration determination. However, it introduces a wide network of stations and it is independent of precise clock information because it estimates satellite clock drifts and drift rates ‘on-the-fly’, requiring only orbit data of sufficient quality. Moreover, with EVA the solution rate is only limited by data rate, and not by the available precise satellite clocks data rate. The results obtained are more robust for long baselines than the results obtained with the reference Kennedy method. An advantage of being independent of precise clock information is that, beside IGS Final products, also the Rapid, Ultra-Rapid (observed) and Ultra-Rapid (predicted) products may be used. Moreover, the EVA technique may also use the undifferenced ionosphere-free carrier phase combination (LC), overcoming baseline limitations in cases where ionosphere gradients may be an issue and very low biases are required. During the development of this work, some problems were found in the velocity estimation process of the Kennedy method. The sources of the problems were identified, and an improved version of the Kennedy method was used for this research work. An experiment was performed using a light aircraft flying over the Pyrenees, showing that both EVA and the improved Kennedy methods are able to cope with the dynamics of mountainous flight. A RTK-derived solution was also generated, and when comparing the three methods to a known zero-velocity reference the results yielded similar performance. The EVA and the improved-Kennedy methods outperformed the RTK solutions, and the EVA method provided the best results in this experiment. Finally, both the improved version of the Kennedy method and the EVA method were applied to a network in equatorial South America with baselines of more than 1,770 km, and during local noon. Under this tough scenario, the EVA method showed a clear advantage for all components of velocity and acceleration, yielding better and more robust results.