Diamond turning of freeform surfaces using non-zero rake angle tools

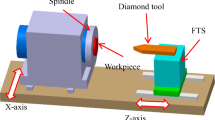
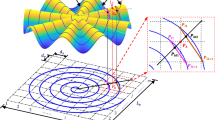
Abstract

Fast tool servo and slow slide servo-based diamond turning are very important and widely used machining methods for freeform surfaces. In diamond turning of freeform surfaces, the research on tool path generation and machined surface topography prediction is generally for diamond turning processes using zero rake angle tools. But diamond turning with non-zero rake angle tools is also of great importance, for which very little research has been done on tool path planning and surface topography modeling. Therefore, based on coordinate transformations, this paper develops a new tool path generation method and discusses the tool geometry parameter selection for diamond turning utilizing non-zero rake angle tools. In addition, a new surface topography prediction model based on the actual position and orientation of the tool cutting edge is constructed for the machined surface. The machining experiments of a typical freeform surface are conducted based on the calculated tool paths and necessary critical tool geometry parameters. The measured results validate the developed tool path generation and tool geometry selection methods, and the constructed surface topography model.

Appendices

Appendix 1

In the following transformation processes, the tool and the workpiece shown in Fig. 2 are taken as a whole rigid body. Rotate the freeform surface and the tool by −φ_t (t) around the Z direction, and the rotation matrix can be expressed as below:

$$ {R}_{\mathrm{z}}\left(-{\varphi}_{\mathrm{t}}(t)\right)=\left[\begin{array}{cccc}\cos \left({\varphi}_{\mathrm{t}}(t)\right)& -\sin \left({\varphi}_{\mathrm{t}}(t)\right)& 0& 0\\ {}\sin \left({\varphi}_{\mathrm{t}}(t)\right)& \cos \left({\varphi}_{\mathrm{t}}(t)\right)& 0& 0\\ {}0& 0& 1& 0\\ {}0& 0& 0& 1\end{array}\right] $$

(34)

Based on Eq. (1), the coordinates of point P_t can be written as (x_t (t), y_t (t), z_c (t)-R_t cos(γ)). Translate the surface and the tool from point P_t to the XOY plane along the negative Z direction, and the translation matrix can be expressed as below:

$$ {T}_{\mathrm{z}}\left(-\left({z}_{\mathrm{c}}(t)-{R}_{\mathrm{t}}\cos \left(\gamma \right)\right)\right)=\left[\begin{array}{cccc}1& 0& 0& 0\\ {}0& 1& 0& 0\\ {}0& 0& 1& 0\\ {}0& 0& -\left({z}_{\mathrm{c}}(t)-{R}_{\mathrm{t}}\cos \left(\gamma \right)\right)& 1\end{array}\right]. $$

(35)

The transformed surface and tool are shown in Fig. 18a. Rotate the freeform surface and the tool by −γ around the X direction, and the rotation matrix can be expressed as below:

$$ {R}_{\mathrm{x}}\left(-\gamma \right)=\left[\begin{array}{cccc}1& 0& 0& 0\\ {}0& \cos \left(\gamma \right)& -\sin \left(\gamma \right)& 0\\ {}0& \sin \left(\gamma \right)& \cos \left(\gamma \right)& 0\\ {}0& 0& 0& 1\end{array}\right]. $$

(36)

The transformed surface and tool are shown in Fig. 18b. Translate the surface and the tool from the XOY plane to the height of the original point P_t along the Z direction, and the translation matrix can be expressed as below:

$$ {T}_{\mathrm{z}}\left({z}_{\mathrm{c}}(t)-{R}_{\mathrm{t}}\cos \left(\gamma \right)\right)=\left[\begin{array}{cccc}1& 0& 0& 0\\ {}0& 1& 0& 0\\ {}0& 0& 1& 0\\ {}0& 0& \left({z}_{\mathrm{c}}(t)-{R}_{\mathrm{t}}\cos \left(\gamma \right)\right)& 1\end{array}\right]. $$

(37)

Fig. 18

Tool and workpiece positions after the transformations

The surface and the tool after the transformations are shown in Fig. 19, in which the tool rake plane is perpendicular to the XOY plane. Based on the geometric relationship between point P_t, point P_tan, and point P_c, the Z coordinates of point P_t can be obtained as

$$ {z}_{\mathrm{t}}(t)={z}_{\mathrm{t}\mathrm{an}}+{R}_t\cos \left({\alpha}_{\mathrm{t}\mathrm{an}}\right)-{R}_t $$

(38)

where z_tan is the Z coordinates of point P_tan, and α_tan is the angle between the X direction and the tangent line between the tool cutting edge and the intersection curve at point P_tan.

Fig. 19

Final positions of the tool and the surface

The coordinates of the transformed freeform surface can be calculated through a series of homogeneous coordinate transformations:

$$ \left[{x}_1\kern0.5em {y}_1\kern0.5em {z}_1\kern0.5em 1\right]=\left[\begin{array}{cccc}x& y& z& 1\end{array}\right]\cdot {R}_{\mathrm{z}}\left(-{\varphi}_{\mathrm{t}}(t)\right)\cdot {T}_{\mathrm{z}}\left(-\left({z}_{\mathrm{c}}(t)-{R}_{\mathrm{t}}\cos \left(\gamma \right)\right)\right)\cdot {R}_{\mathrm{x}}\left(-\gamma \right)\cdot {T}_{\mathrm{z}}\left(\left({z}_{\mathrm{c}}(t)-{R}_{\mathrm{t}}\cos \left(\gamma \right)\right)\right) $$

(39)

where (x, y, z) and (x₁, y₁, z₁) are the freeform surface coordinates before and after the coordinate transformations, respectively.

The transformed freeform surface can be expressed as

$$ F\left(\begin{array}{c}\cos \left({\varphi}_{\mathrm{t}}(t)\right)x-\cos \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)y+\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)z-\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)\left({z}_{\mathrm{c}}-{R}_t\cos \gamma \right),\\ {}\sin \left({\varphi}_{\mathrm{t}}(t)\right)x+\cos \left(\gamma \right)\cos \left({\varphi}_{\mathrm{t}}(t)\right)y-\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right)z+\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right)\left({z}_{\mathrm{c}}-{R}_t\cos \gamma \right),\\ {}\sin \left(\gamma \right)y+\cos \left(\gamma \right)z+\left({z}_{\mathrm{c}}-{R}_{\mathrm{t}}\cos \gamma \right)-\cos \left(\gamma \right)\left({z}_{\mathrm{c}}-{R}_{\mathrm{t}}\cos \gamma \right)\end{array}\right)=0. $$

(40)

The equation above can be further transformed to the cylindrical coordinates:

$$ F\left(\begin{array}{c}\cos \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \cos \left(\theta \right)\right)-\cos \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \sin \left(\theta \right)\right)+\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)z-\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)\left({z}_{\mathrm{c}}-{R}_{\mathrm{t}}\cos \gamma \right),\\ {}\sin \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \cos \left(\theta \right)\right)+\cos \left(\gamma \right)\cos \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \sin \left(\theta \right)\right)-\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right)z+\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right)\left({z}_{\mathrm{c}}-{R}_{\mathrm{t}}\cos \gamma \right),\\ {}\sin \left(\gamma \right)\left(\rho \sin \left(\theta \right)\right)+\cos \left(\gamma \right)z+\left({z}_{\mathrm{c}}-{R}_{\mathrm{t}}\cos \gamma \right)-\cos \left(\gamma \right)\left({z}_{\mathrm{c}}-{R}_{\mathrm{t}}\cos \gamma \right)\end{array}\right)=0. $$

(41)

The surface can be written as F (ρ, θ, z) = 0, and the intersection curve generated by the tool rake plane and the freeform surface at time t can be obtained as

$$ {\left.F\left(\rho, \theta, z\right)\right|}_{\theta =0}=0. $$

(42)

The first derivative of the intersection curve can be calculated as

$$ {z}^{\prime }=\frac{\mathrm{d}z}{\mathrm{d}\rho }=-\frac{{\left.{F}_{\uprho}\left(\rho, \theta, z\right)\right|}_{\theta =0}}{{\left.{F}_{\mathrm{z}}\left(\rho, \theta, z\right)\right|}_{\theta =0}}. $$

(43)

Appendix 2

Rotate the freeform surface shown in Fig. 3 by −φ_t (t) around the Z direction, and the rotation matrix can be expressed as below:

$$ {R}_{\mathrm{z}}\left(-{\varphi}_{\mathrm{t}}(t)\right)=\left[\begin{array}{cccc}\cos \left({\varphi}_{\mathrm{t}}(t)\right)& -\sin \left({\varphi}_{\mathrm{t}}(t)\right)& 0& 0\\ {}\sin \left({\varphi}_{\mathrm{t}}(t)\right)& \cos \left({\varphi}_{\mathrm{t}}(t)\right)& 0& 0\\ {}0& 0& 1& 0\\ {}0& 0& 0& 1\end{array}\right]. $$

(44)

Translate the surface from the surface point P_sur to the XOY plane along the negative Z direction, and the translation matrix can be expressed as below:

$$ {T}_{\mathrm{z}}\left(-{z}_{\mathrm{sur}}(t)\right)=\left[\begin{array}{cccc}1& 0& 0& 0\\ {}0& 1& 0& 0\\ {}0& 0& 1& 0\\ {}0& 0& -{z}_{\mathrm{sur}}(t)& 1\end{array}\right]. $$

(45)

Rotate the freeform surface by −γ around the X direction, and the rotation matrix can be expressed as below:

$$ {R}_{\mathrm{x}}\left(-\gamma \right)=\left[\begin{array}{cccc}1& 0& 0& 0\\ {}0& \cos \left(\gamma \right)& -\sin \left(\gamma \right)& 0\\ {}0& \sin \left(\gamma \right)& \cos \left(\gamma \right)& 0\\ {}0& 0& 0& 1\end{array}\right]. $$

(46)

Translate the surface from the XOY plane to point P_sur along the Z direction, and the translation matrix can be expressed as below:

$$ {T}_{\mathrm{z}}\left({z}_{\mathrm{sur}}(t)\right)=\left[\begin{array}{cccc}1& 0& 0& 0\\ {}0& 1& 0& 0\\ {}0& 0& 1& 0\\ {}0& 0& {z}_{\mathrm{sur}}(t)& 1\end{array}\right]. $$

(47)

The surface after the transformations is shown in Fig. 20. The coordinates of the transformed freeform surface can be calculated through a series of homogeneous coordinate transformations:

$$ \left[{x}_1\kern0.5em {y}_1\kern0.5em {z}_1\kern0.5em 1\right]=\left[\begin{array}{cccc}x& y& z& 1\end{array}\right]\cdot {R}_{\mathrm{z}}\left(-{\varphi}_{\mathrm{t}}(t)\right)\cdot {T}_{\mathrm{z}}\left(-{z}_{\mathrm{sur}}(t)\right)\cdot {R}_{\mathrm{x}}\left(-\gamma \right)\cdot {T}_{\mathrm{z}}\left({z}_{\mathrm{sur}}(t)\right) $$

(48)

where (x, y, z) and (x₁, y₁, z₁) are the freeform surface coordinates before and after the coordinate transformations, respectively.

Fig. 20

Final position of the transformed surface

The transformed freeform surface can be expressed as

$$ F\left(\begin{array}{c}\cos \left({\varphi}_{\mathrm{t}}(t)\right)x-\cos \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)y+\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)z-\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right){z}_{\mathrm{sur}}(t),\\ {}\sin \left({\varphi}_{\mathrm{t}}(t)\right)x+\cos \left(\gamma \right)\cos \left({\varphi}_{\mathrm{t}}(t)\right)y-\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right)z+\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right){z}_{\mathrm{sur}}(t),\\ {}\sin \left(\gamma \right)y+\cos \left(\gamma \right)z+{z}_{\mathrm{sur}}(t)-\cos \left(\gamma \right){z}_{\mathrm{sur}}(t)\end{array}\right)=0. $$

(49)

The equation above can be further written in cylindrical coordinates:

$$ F\left(\begin{array}{c}\cos \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \cos \left(\theta \right)\right)-\cos \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \sin \left(\theta \right)\right)+\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right)z-\sin \left(\gamma \right)\sin \left({\varphi}_{\mathrm{t}}(t)\right){z}_{\mathrm{sur}}(t),\\ {}\sin \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \cos \left(\theta \right)\right)+\cos \left(\gamma \right)\cos \left({\varphi}_{\mathrm{t}}(t)\right)\left(\rho \sin \left(\theta \right)\right)-\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right)z+\cos \left({\varphi}_{\mathrm{t}}(t)\right)\sin \left(\gamma \right){z}_{\mathrm{sur}}(t),\\ {}\sin \left(\gamma \right)\left(\rho \sin \left(\theta \right)\right)+\cos \left(\gamma \right)z+{z}_{\mathrm{sur}}(t)-\cos \left(\gamma \right){z}_{\mathrm{sur}}(t)\end{array}\right)=0. $$

(50)

The surface can be written as F (ρ, θ, z) = 0, and the intersection curve generated by the XOZ plane and the freeform surface at time t shown in Fig. 20b can be calculated as

$$ {\left.F\left(\rho, \theta, z\right)\right|}_{\theta =0}=0. $$

(51)

The first derivative of the intersection curve can be calculated as

$$ {z}^{\prime }=\frac{\mathrm{d}z}{\mathrm{d}\rho }=-\frac{{\left.{F}_{\uprho}\left(\rho, \theta, z\right)\right|}_{\theta =0}}{{\left.{F}_{\mathrm{z}}\left(\rho, \theta, z\right)\right|}_{\theta =0}}=G\left(\rho, z\right). $$

(52)

The second derivative of the intersection curve can be calculated as

$$ {z}^{\prime \prime }=\frac{{\mathrm{d}}^2z}{\mathrm{d}{\rho}^2}={G}_{\rho}\left(\rho, z\right)+{G}_z\left(\rho, z\right)G\left(\rho, z\right). $$

(53)