Algorithms for no-wait flowshops with total completion time subject to makespan

Abstract

The m-machine no-wait flowshop scheduling problem, with the objective of minimizing total completion time subject to the constraint that makespan has to be less than or equal to a certain value, is addressed in this paper. First, an algorithm is proposed to find an upper bound on the makespan in case the upper bound is not given or unknown. Given the upper bound on makespan, a proposed algorithm (PAL) with five versions L (1, 5, 10, 15, and 20) and a genetic algorithm (GA) are utilized for solving the problem. Furthermore, a dominance relation is established for the case of four machines. The five versions of PAL and GA are evaluated on randomly generated problems with different number of jobs and number of machines. Computational experiments show that the errors of PA1 0, PA15, and PA20 are much smaller than that of GA while the CPU times of PA10, PA15, and PA20 are significantly smaller than that of GA. Therefore, the algorithms PA10, PA15, and PA20 are superior to the GA algorithm.

Appendix (Proof of the Theorem)

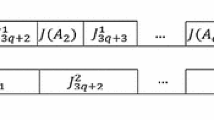
Let

t _k,m : processing time of job k on machine m(m = 1, 2, 3, and 4)

t _[k,m]: processing time of the job in position k on machine m

C _[k]: completion time of the job in position k on the fourth machine

We assume that every job requires processing on all machines, i.e., t _j,k  > 0. Such a four-machine no-wait flowshop is necessarily a permutation flow shop. In other words, the order of jobs on all machines must be the same.

It can be shown that for a job in position k,

$$ {C_{{\left[ k \right]}}}=\sum\limits_{r=1}^k { \max \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}+\sum\limits_{r=1}^k {{t_{{\left[ {r,4} \right]}}}} } $$

(1)

where, ${t_{{\left[ {0,2} \right]}}}={t_{{\left[ {0,3} \right]}}}={t_{{\left[ {0,4} \right]}}}=0$.

Since the total completion time, TCT, is

$$ \mathrm{TCT}=\sum\limits_{i=1}^n {{C_{{\left[ i \right]}}}} $$

It follows from Eq. 1 that

$$ \mathrm{TCT}=\sum\limits_{j=1}^n {\sum\limits_{i=1}^j {\left( { \max \left\{ {0;{t_{{\left[ {i,3} \right]}}}-{t_{{\left[ {i-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {i,1} \right]}}}+{t_{{\left[ {i,2} \right]}}}-{t_{{\left[ {i-1,2} \right]}}}-{t_{{\left[ {i-1,3} \right]}}};{t_{{\left[ {i,2} \right]}}}-{t_{{\left[ {i-1,3} \right]}}}} \right)} \right\}+{t_{{\left[ {i,4} \right]}}}} \right)} } $$

(2)

Consider two sequences σ ₁ and σ ₂ such that σ ₁ has job i in an arbitrary position τ and job j in position τ + 1. The sequence σ ₂ is exactly the same as σ ₁ except that job j is in position τ and job i in position τ + 1.

Since the two sequences have the same job in positions up to and including τ − 1,

$$ {C_{{\left[ r \right]}}}\left( {{\sigma_1}} \right)={C_{{\left[ r \right]}}}\left( {{\sigma_2}} \right)\mathrm{for}\ r=1,2,\ldots,\tau -1 $$

(3)

Then, we have the following equations for the two sequences,

$$ \begin{array}{*{20}c} {{C_{{\left[ r \right]}}}\left( {{\sigma_1}} \right)=\sum\limits_{r=1}^{{\tau -1}} { \max } \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} \hfill \\ {+\sum\limits_{r=1}^{{\tau -1}} {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ {+ \max \left\{ {0;{t_{i,3 }}-{t_{{\left[ {\tau, 1,4} \right]}}}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{i,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ {+{t_{i,4 }}} \hfill \\ \end{array} $$

(4)

$$ \begin{array}{*{20}c} {{C_{{\left[ \tau \right]}}}\left( {{\sigma_2}} \right)=\sum\limits_{r=1}^{{\tau -1}} { \max \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} } \hfill \\ {+\sum\limits_{r=1}^{{\tau -1}} {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ { + \max \left\{ {0;{t_{j,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{j,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ {+{t_{j,4 }}} \hfill \\ \end{array} $$

(5)

$$ \begin{array}{*{20}c} {{C_{{\left[ {\tau +1} \right]}}}\left( {{\sigma_1}} \right)=\sum\limits_{r=1}^{{\tau -1}} { \max } \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} \hfill \\ {+\sum\limits_{r=1}^{{\tau -1}} {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ { + \max \left\{ {0;{t_{i,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{i,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ {+{t_{i,4 }}} \hfill \\ { + \max \left\{ {0;{t_{j,3 }}-{t_{i,4 }}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{i,2 }}-{t_{i,3 }};{t_{j,2 }}-{t_{i,3 }}} \right)} \right\}} \hfill \\ {+{t_{j,4 }}} \hfill \\ \end{array} $$

(6)

$$ \begin{array}{*{20}c} {{C_{{\left[ {\tau +1} \right]}}}\left( {{\sigma_2}} \right)=\sum\limits_{r=1}^{{\tau -1}} { \max \left\{ {0;{t_{r,3 }}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} } \hfill \\ {+\sum\limits_{r=1}^{{\tau -1}} {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ { + \max \left\{ {0;{t_{j,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{j,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ {+{t_{j,4 }}} \hfill \\ { + \max \left\{ {0;{t_{i,3 }}-{t_{j,4 }}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{j,2 }}-{t_{j,3 }};{t_{i,2 }}-{t_{j,3 }}} \right)} \right\}} \hfill \\ {+{t_{i,4 }}} \hfill \\ \end{array} $$

(7)

From equations (3–7),

$$ \begin{array}{*{20}c} {\left[ {{C_{{\left[ \tau \right]}}}\left( {{\sigma_1}} \right)+{C_{{\left[ {\tau +1} \right]}}}\left( {{\sigma_1}} \right)} \right]-\left[ {{C_{{\left[ \tau \right]}}}\left( {{\sigma_2}} \right)+{C_{{\left[ {\tau +1} \right]}}}\left( {{\sigma_2}} \right)} \right]} \\ {=2 \max \left\{ {0;{t_{i,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{i,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \\ {+ \max \left\{ {0;{t_{j,3 }}-{t_{i,4 }}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{i,2 }}-{t_{i,3 }};{t_{j,2 }}-{t_{i,3 }}} \right)} \right\}} \\ {-2 \max \left\{ {0;{t_{j,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{j,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \\ {- \max \left\{ {0;{t_{i,3 }}-{t_{j,4 }}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{j,2 }}-{t_{j,3 }};{t_{i,2 }}-{t_{j,3 }}} \right)} \right\}} \\ {+{t_{i,4 }}-{t_{j,4 }}} \\ \end{array} $$

(8)

It can be easily shown that if the conditions given in the hypothesis are satisfied, then Eq. 8 reduces to

$$ \left[ {{C_{{\left[ \tau \right]}}}\left( {{\sigma_1}} \right)+{C_{{\left[ {\tau +1} \right]}}}\left( {{\sigma_1}} \right)} \right]\leq \left[ {{C_{{\left[ \tau \right]}}}\left( {{\sigma_2}} \right)+{C_{{\left[ {\tau +1} \right]}}}\left( {{\sigma_2}} \right)} \right] $$

(9)

Now, for C _[k] where k = τ + 2, … , n,

$$ \begin{array}{*{20}c} {{C_{{\left[ k \right]}}}\left( {{\sigma_1}} \right)=\sum\limits_{r=1}^{{\tau -1}} { \max \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} } \hfill \\ {+\sum\limits_{r=1}^{{\tau -1}} {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ { + \max \left\{ {0;{t_{i,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{i,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ {+{t_{i,4 }}} \hfill \\ { + \max \left\{ {0;{t_{j,3 }}-{t_{i,4 }}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{i,2 }}-{t_{i,3 }};{t_{j,2 }}-{t_{i,3 }}} \right)} \right\}} \hfill \\ {+{t_{j,4 }}} \hfill \\ { + \max \left\{ {0;{t_{{\left[ {\tau +2,3} \right]}}}-{t_{j,4 }}+ \max \left( {0;{t_{{\left[ {\tau +2,1} \right]}}}+{t_{{\left[ {\tau +2,2} \right]}}}-{t_{j,2 }}-{t_{j,3 }};{t_{{\left[ {\tau +2,2} \right]}}}-{t_{j,3 }}} \right)} \right\}} \hfill \\ {+{t_{{\left[ {\tau +2,3} \right]}}}} \hfill \\ {+\sum\limits_{{r=\tau +3}}^k { \max \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} } \hfill \\ {+\sum\limits_{{r=\tau +3}}^k {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ \end{array} $$

(10)

$$ \begin{array}{*{20}c} {{C_{{\left[ k \right]}}}\left( {{\sigma_2}} \right)=\sum\limits_{r=1}^{{\tau -1}} { \max \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} } \hfill \\ {+\sum\limits_{r=1}^{{\tau -1}} {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ { + \max \left\{ {0;{t_{j,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{j,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ {+{t_{j,4 }}} \hfill \\ { + \max \left\{ {0;{t_{i,3 }}-{t_{j,4 }}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{j,2 }}-{t_{j,3 }};{t_{i,2 }}-{t_{j,3 }}} \right)} \right\}} \hfill \\ {+{t_{i,4 }}} \hfill \\ { + \max \left\{ {0;{t_{{\left[ {\tau +2,3} \right]}}}-{t_{i,4 }}+ \max \left( {0;{t_{{\left[ {\tau +2,1} \right]}}}+{t_{{\left[ {\tau +2,2} \right]}}}-{t_{i,2 }}-{t_{i,3 }};{t_{{\left[ {\tau +2,2} \right]}}}-{t_{i,3 }}} \right)} \right\}} \hfill \\ {+{t_{{\left[ {\tau +2,3} \right]}}}} \hfill \\ {+\sum\limits_{{r=\tau +3}}^k { \max \left\{ {0;{t_{{\left[ {r,3} \right]}}}-{t_{{\left[ {r-1,4} \right]}}}+ \max \left( {0;{t_{{\left[ {r,1} \right]}}}+{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}};{t_{{\left[ {r,2} \right]}}}-{t_{{\left[ {r-1,3} \right]}}}} \right)} \right\}} } \hfill \\ {+\sum\limits_{{r=\tau +3}}^k {{t_{{\left[ {r,4} \right]}}}} } \hfill \\ \end{array} $$

(11)

Where $\sum\limits_{{r=\tau +3}}^{{\tau +2}} {(.)=0}$.

It follows from Eqs. 10 and 11 that

$$ \begin{array}{*{20}c} {{C_{{\left[ r \right]}}}\left( {{\sigma_1}} \right)-{C_{{\left[ r \right]}}}\left( {{\sigma_2}} \right)= \max \left\{ {0;{t_{i,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{i,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ { + \max \left\{ {0;{t_{j,3 }}-{t_{i,4 }}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{i,2 }}-{t_{i,3 }};{t_{j,2 }}-{t_{i,3 }}} \right)} \right\}} \hfill \\ { + \max \left\{ {0;{t_{{\left[ {\tau +2,3} \right]}}}-{t_{j,4 }}+ \max \left( {0;{t_{{\left[ {\tau +2,1} \right]}}}+{t_{{\left[ {\tau +2,2} \right]}}}-{t_{j,2 }}-{t_{j,3 }};{t_{{\left[ {\tau +2,2} \right]}}}-{t_{j,3 }}} \right)} \right\}} \hfill \\ { - \max \left\{ {0;{t_{j,3 }}-{t_{{\left[ {\tau -1,4} \right]}}}+ \max \left( {0;{t_{j,1 }}+{t_{j,2 }}-{t_{{\left[ {\tau -1,2} \right]}}}-{t_{{\left[ {\tau -1,3} \right]}}};{t_{j,2 }}-{t_{{\left[ {\tau -1,3} \right]}}}} \right)} \right\}} \hfill \\ { - \max \left\{ {0;{t_{i,3 }}-{t_{{\left[ {j,4} \right]}}}+ \max \left( {0;{t_{i,1 }}+{t_{i,2 }}-{t_{j,2 }}-{t_{j,3 }};{t_{i,2 }}-{t_{j,3 }}} \right)} \right\}} \hfill \\ { - \max \left\{ {0;{t_{{\left[ {\tau +2,3} \right]}}}-{t_{{\left[ {i,4} \right]}}}+ \max \left( {0;{t_{{\left[ {\tau +2,1} \right]}}}+{t_{{\left[ {\tau +2,2} \right]}}}-{t_{i,2 }}-{t_{i,3 }};{t_{{\left[ {\tau +2,2} \right]}}}-{t_{i,3 }}} \right)} \right\}} \hfill \\ \end{array} $$

(12)

Given that the conditions specified in the theorem are satisfied, then Eq. 12 satisfies

$$ {C_{{\left[ r \right]}}}\left( {{\sigma_2}} \right)\leq {C_{{\left[ r \right]}}}\left( {{\sigma_1}} \right)\ \mathrm{for}\ r=\tau +2,\tau +3,\ldots,n $$

(13)

Hence, the inequality TCT(σ ₁) ≤ TCT(σ ₂) holds as a result of Eqs. 3, 9, and 13.