Statistical mechanics of rate-independent stick-slip on a corrugated surface composed of parabolic wells

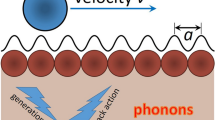
Abstract

The stick-slip phenomenon, at the basis of friction, is crucial for several applications ranging from nanotechnology and biophysics to mechanics and geology. Deep understanding of friction mechanisms and, in particular, the methodologies for its reduction must be sought in its nanoscopic nature, where atomic interactions and stick-slip processes play a crucial role. At this scale, thermal fluctuations clearly have a major effect on the physics of the problem. Hence, we develop here a theory for rate-independent stick-slip, based on equilibrium statistical mechanics. In particular, we introduce suitably modified Prandtl–Tomlinson and Frenkel–Kontorova models in order to study the system with one particle and the chain with N particles, respectively. The adopted corrugated substrate is composed of a sequence of quadratic wells. Interestingly, the calculation of corresponding partition functions shows a conceptual link with the theory of Jacobi and Riemann theta functions, allowing an efficient determination of the average static frictional force and other relevant quantities. We show some applications including the study of structural lubricity and thermolubricity.

Appendix A: reciprocal relation for Jacobi and Riemann theta functions

In Appendix, we briefly discuss the conceptual relationship between direct and reciprocal Bravais lattices, Dirac comb, Poisson summation formula, and reciprocal relation for the Riemann theta function and third Jacobi theta function. We consider a Bravais lattice composed of points $\vec {r}=n_1\vec {a}_1+...+n_N\vec {a}_N\in \mathbb {R}^N$ where $n_j\in \mathbb {Z}$ and $\vec {a}_j\in \mathbb {R}^N$ are the primitive vectors. We introduce a periodic sampling function $f(\vec {x})$ on this Bravais lattice constructed by delta functions (Dirac comb)

$$\begin{aligned} f(\vec {x})=\sum _{\vec {n}\in \mathbb {Z}^N}\delta \left( \vec {x}-n_1\vec {a}_1...-n_N\vec {a}_N\right) . \end{aligned}$$

(A1)

To simplify the notation, we define a matrix $\mathcal {C}\in \mathcal {M}_{N,N}(\mathbb {R})$ where the columns represent the vectors $\vec {a}_j$. It means that $\mathcal {C}=[\vec {a}_1\vert ...\vert \vec {a}_N]$ and we can write

$$\begin{aligned} f(\vec {x})=\sum _{\vec {n}\in \mathbb {Z}^N}\delta \left( \vec {x}-\mathcal {C}\vec {n}\right) , \end{aligned}$$

(A2)

with $\vec {n}=(n_1,...,n_N)^T$. We assume that $\mathcal {C}$ is not singular. Then, if we define $\vec {\eta }$ such that $\vec {x}=\mathcal {C}\vec {\eta }$, we have

$$\begin{aligned} f(\vec {\eta })=\sum _{\vec {n}\in \mathbb {Z}^N}\delta \left( \mathcal {C}(\vec {\eta }-\vec {n})\right) . \end{aligned}$$

(A3)

Now, the function $f(\vec {\eta })$ is multi-periodic with period one along all directions $\eta _1,...,\eta _N$. Hence, it can be developed in Fourier series

$$\begin{aligned} f(\vec {\eta })=\sum _{\vec {m}\in \mathbb {Z}^N}c_{\vec {m}}e^{2\pi i \vec {m}\cdot \vec {\eta }}, \end{aligned}$$

(A4)

where the coefficients are given by

$$\begin{aligned} c_{\vec {m}}=\int _{[0,1]^N}f(\vec {\eta })e^{-2\pi i \vec {m}\cdot \vec {\eta }}\mathrm {d}\vec {\eta }. \end{aligned}$$

(A5)

In the set $[0,1]^N$, we have $f(\vec {\eta })=\delta \left( \mathcal {C}\vec {\eta }\right)$, and therefore, we get

$$\begin{aligned} c_{\vec {m}}=\int _{[0,1]^N}\delta \left( \mathcal {C}\vec {\eta }\right) e^{-2\pi i \vec {m}\cdot \vec {\eta }}\mathrm {d}\vec {\eta }=\frac{1}{\det \mathcal {C}}, \end{aligned}$$

(A6)

as we can easily prove by applying the substitution $\vec {y}=\mathcal {C}\vec {\eta }$. Coming back to the variable $\vec {x}$, we finally obtain

$$\begin{aligned} f(\vec {x})=\frac{1}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}e^{2\pi i \vec {m}\cdot \mathcal {C}^{-1} \vec {x}}, \end{aligned}$$

(A7)

which is the Fourier series of the Dirac comb defined in Eq. (A1) or (A2). We can also determine the Fourier transform of the same function

$$\begin{aligned} F(\vec {k})=\int _{\mathbb {R}^N}f(\vec {x})e^{- i \vec {k}\cdot \vec {x}}\mathrm {d}\vec {x}=\frac{1}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}\int _{\mathbb {R}^N}e^{2\pi i \vec {m}\cdot \mathcal {C}^{-1} \vec {x}}e^{- i \vec {k}\cdot \vec {x}}\mathrm {d}\vec {x}. \end{aligned}$$

(A8)

Since $\delta (\vec {q})=\int _{\mathbb {R}^N}e^{i\vec {q}\cdot \vec {x}}\mathrm {d}\vec {x}/(2\pi )^N$, we easily get

$$\begin{aligned} F(\vec {k})=\frac{(2\pi )^N}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}\delta \left( \vec {k}-2\pi \mathcal {C}^{-T}\vec {m} \right) . \end{aligned}$$

(A9)

It means that if $\vec {r}=\mathcal {C}\vec {n}$ $\forall \vec {n}\in \mathbb {Z}^N$ is the direct Bravais lattice, then $\vec {k}=2\pi \mathcal {C}^{-T}\vec {m}$ $\forall \vec {m}\in \mathbb {Z}^N$ is the reciprocal Bravais lattice. In other words, the Dirac comb on the direct lattice is Fourier transformed into a Dirac comb on the reciprocal lattice. This property can be applied to find the so-called N-dimensional Poisson summation formula. We consider an arbitrary function $\phi (\vec {x})$ for which the Fourier transform $\Phi (\vec {k})$ exists. We define the replication or periodic summation

$$\begin{aligned} g(\vec {x})=\sum _{\vec {n}\in \mathbb {Z}^N}\phi (\vec {x}-\mathcal {C}\vec {n})=\phi *f, \end{aligned}$$

(A10)

where f is defined in Eq. (A2) and $*$ means convolution. Since the Fourier transform of the convolution is the product of the two Fourier transforms (convolution theorem), we have

$$\begin{aligned} G(\vec {k})= & {} \frac{(2\pi )^N}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}\Phi (\vec {k})\delta \left( \vec {k}-2\pi \mathcal {C}^{-T}\vec {m} \right) \nonumber \\= & {} \frac{(2\pi )^N}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}\Phi (2\pi \mathcal {C}^{-T}\vec {m})\delta \left( \vec {k}-2\pi \mathcal {C}^{-T}\vec {m} \right) . \end{aligned}$$

(A11)

Now, we can remember that the Fourier transform of $e^{i\vec {x}\cdot \vec {v}}$ is given by $(2\pi )^N\delta (\vec {k}-\vec {v})$, and then from Eq. (A11) we come back to the original function $g(\vec {x})$, eventually obtaining

$$\begin{aligned} \sum _{\vec {n}\in \mathbb {Z}^N}\phi (\vec {x}-\mathcal {C}\vec {n})=\frac{1}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}\Phi (2\pi \mathcal {C}^{-T}\vec {m})e^{2\pi i \vec {x}\cdot \mathcal {C}^{-T} \vec {m}}, \end{aligned}$$

(A12)

which is the Fourier series of the periodic summation. For $\vec {x}=0$, this result delivers the Poisson summation formula

$$\begin{aligned} \sum _{\vec {n}\in \mathbb {Z}^N}\phi (\mathcal {C}\vec {n})=\frac{1}{\det \mathcal {C}}\sum _{\vec {m}\in \mathbb {Z}^N}\Phi (2\pi \mathcal {C}^{-T}\vec {m}). \end{aligned}$$

(A13)

We take now into account the following particular function $\phi (\vec {x})$ with its Fourier transform $\Phi (\vec {k})$

$$\begin{aligned} \phi (\vec {x})= & {} e^{2\pi i \left( \frac{1}{2}\vec {x}\cdot \mathcal {T}\vec {x}+\vec {x}\cdot \vec {\sigma } \right) },\end{aligned}$$

(A14)

$$\begin{aligned} \Phi (\vec {k})= & {} \frac{1}{\sqrt{\det (-i\mathcal {T})}}e^{-\pi i \left( \vec {\sigma }-\frac{\vec {k}}{2\pi }\right) \cdot \mathcal {T}^{-1}\left( \vec {\sigma }-\frac{\vec {k}}{2\pi }\right) }, \end{aligned}$$

(A15)

where $\mathcal {T}\in \mathcal {M}_{N,N}(\mathbb {C})$ with $\mathcal {T}=\mathcal {T}^T$, $\Im \text{ m }(\mathcal {T})>0$ and $\vec {\sigma }\in \mathbb {C}^N$. To conclude, we substitute Eqs. (A14) and (A15) in the Poisson summation formula given in Eq. (A13) and we get, after straightforward calculations, the N-dimensional Jacobi reciprocal relation for the Riemann theta function

$$\begin{aligned} \Theta (\vec {z}\vert \Omega )=\sqrt{\frac{1}{\det \left( -i\Omega \right) }}e^{-\pi i \vec {z}\cdot \Omega ^{-1}\vec {z}}\Theta (\Omega ^{-1}\vec {z}\vert -\Omega ^{-1}),\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \end{aligned}$$

(A16)

where we have identified $\Omega =\mathcal {C}^T\mathcal {T}\mathcal {C}$ and $\vec {z}=\mathcal {C}^T\vec {\sigma }$. This proves Eq. (47) of the main text. Other more refined properties of $\Theta (\vec {z}\vert \Omega )$ can be found in the literature [112, 113]. The result given in Eq. (A16) can be specialized to the case with $N=1$ by obtaining the original Jacobi identity

$$\begin{aligned} \vartheta _3\left( z,\tau \right) =\frac{1}{\sqrt{-i\tau }}e^{\frac{z^2}{\pi i \tau }}\vartheta _3\left( \frac{z}{\tau },-\frac{1}{\tau }\right) , \end{aligned}$$

(A17)

where we have conveniently compared the definitions of $\vartheta _3\left( z,\tau \right)$ and $\Theta (\vec {z}\vert \Omega )$ with $N=1$ [112, 113]. This finally proves Eq. (17) of the main text as well.