Impact of damage on the effective properties of network materials and on bulk and surface wave propagation characteristics

Abstract

We analyze in this contribution the propagation of bulk and Rayleigh surface waves in periodic architectured materials undergoing internal damage. An elastic damageable continuum-based model is developed in the framework of the thermodynamics of irreversible processes, whereby the displacement experiences a jump across the faces of the propagating crack. The crack propagation involves an enhancement of the displacement field associated with embedded discontinuities, which remain localized within each (triangular type) finite element. The displacement discontinuity is regulated by the traction separation behavior described by an exponential cohesive model with damage hardening followed by softening. The effective mechanical properties of the overall network are evaluated versus the increasing damage. The phase velocities for the longitudinal and transverse modes are then computed continuously versus the amount of damage, considering successively the situations of symmetrically and unsymmetrically distributed damage occurrence and propagation. Simulation results show that although the crack pattern is different in these two situations, it has no impact on the evolution of the effective moduli versus global damage. The phase velocities computed based on the effective moduli decrease as damage propagates within the network; thus, it is an indicator of the amount of global damage.

Appendices

Appendix 1: Computation of the effective properties

In general, the computation of the effective moduli of a Y-shape unit cell (with the dimensions as in Fig. 24), which is in turn subjected to the homogeneous displacement-controlled tension $({}^{{u}_{xx}}/ 2)$ along the X-direction, the homogeneous displacement-controlled tension $\left( u_{yy} \right)$ in the Y-direction, and the homogeneous displacement-controlled shearing $\left( u_{yx} \right)$ case shown in Fig. 9, is separately treated as follows:

Fig. 24

Loading conditions for the computation of the effective moduli

• In case the sample is loaded in tension by homogeneous displacements $({}^{{u}_{xx}}/2)$, the effective modulus is computed as: $E_{xx}=\frac{\sigma _{xx}}{\varepsilon _{xx}}$ with $\sigma _{xx}=\frac{\left| R_{x,2} \right| }{h}$, and $\varepsilon _{xx}=\frac{u_{xx}}{L}$

  The corresponding Poisson’s ratio can be easily defined as: $\upsilon _{xy}=-\frac{\frac{u_{y,\mathrm{node} \,{{\varvec{c}}}}}{H}}{\varepsilon _{xx}} =-\frac{u_{y,\mathrm{node}\,{{\varvec{c}}}}}{H\varepsilon _{xx}}$

  where $\sigma _{xx}$ is the stress, $\varepsilon _{xx}$ refers to the strain, $R_{x,2}$ denotes the total reaction force at nodes on the right edge of the unit cell where displacements ${}^{u_{xx}}/ 2$ are imposed, and $u_{y,\mathrm{node}\,{{\varvec{c}}}}$ stands for the measured displacement in the Y-direction at node c.

• Similarly, when homogeneous displacements $\left( u_{yy} \right)$ are applied to the specimen the value of the effective modulus is $E_{yy}=\frac{\sigma _{yy}}{\varepsilon _{yy}}$ with $\sigma _{yy}=\frac{\left| R_{y} \right| }{2l}$ and $\varepsilon _{yy}=\frac{u_{yy}}{H}$.

  Meanwhile, $\upsilon {}_{yx}=-\frac{\frac{u_{x,\mathrm{node}\,{{\varvec{a}}}}-u_{x,\mathrm{node}\,{{\varvec{b}}}}}{L}}{\varepsilon _{yy}}=-\frac{u_{x,\mathrm{node}\,{{\varvec{a}}}}-u_{x,\mathrm{node}\,{{\varvec{b}}}}}{L\varepsilon _{yy}}$ is the expression of the Poisson’s ratio in this case, wherein $\sigma _{yy}$ is the stress, $\varepsilon _{yy}$ is the strain, $R_{y}$ is referred to the sum of reaction forces in the Y-direction at the support points, and $u_{x,\mathrm{node}\,{{\varvec{a}}}},u_{x,\mathrm{node}\,{{\varvec{b}}}}$ represent the measured displacement in the X-direction at node a and b, respectively.

• In the last case in which the imposed loads are the homogeneous displacement-controlled shearing $\left( u_{yx} \right)$, the effective shear modulus can be written as: $G_{yx}=\frac{\tau _{yx}}{\varepsilon _{yx}}$ with $\tau _{yx}=\frac{\left| R_{x,1} \right| }{2l}$, $\varepsilon _{yx}=\frac{u_{yx}}{H}$, wherein $\tau _{yx}$ is the shear stress, $\varepsilon _{yx}$ is the shear strain, and $R_{x,1}$ is the sum of reaction forces in the X-direction at the support points.

Finally, we obtain the full rigidity matrix as follows:

$$\begin{aligned} \left[ {\begin{array}{*{20}c} \varepsilon _{xx}\\ \varepsilon _{yy}\\ 2\varepsilon _{xy}\\ \end{array} } \right] =\left[ {\begin{array}{*{20}c} \frac{1}{E_{xx}} &{} -\frac{\nu _{yx}}{E_{yy}} &{} \frac{\eta _{xy}}{G_{xy}}\\ -\frac{\nu _{xy}}{E_{xx}} &{} \frac{1}{E_{yy}} &{} \frac{\mu _{xy}}{G_{xy}}\\ \frac{\eta _{xy}}{G_{xy}} &{} \frac{\mu _{xy}}{G_{xy}} &{} \frac{1}{G_{xy}}\\ \end{array} } \right] \left[ {\begin{array}{*{20}c} \sigma _{xx}\\ \sigma _{yy}\\ \tau _{xy}\\ \end{array} } \right] . \end{aligned}$$

(72)

Appendix 2: Implementation of periodicity conditions

The finite element model is capable of simulating elementary cells under combined loading conditions. Based upon the relation between the strain energy of the microstructure and that of the homogenized equivalent model under specific periodic boundary conditions, a strain energy-based method is developed. This method is then used to compute the effective elastic properties. The heterogeneity within the unit cell of the repetitive architectured material is taken into account through the homogenized material properties.

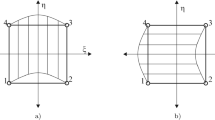
At the meso-level, the finite element modeling procedure accounts for a single unit cell instead of the entire structure. By imposing a specific type of geometric constraints known as “periodic boundary conditions” on the unit cells, the boundary effects from the adjacent cells are taken into account. It is noteworthy that the boundary surfaces of the unit cell always appear in parallel pairs. The periodicity vectors along with the periodic boundary conditions are shown in Fig. 25.

Fig. 25

Periodicity vectors $\hbox {Y}_{1}$, $\hbox {Y}_{2}$ and related nodes for the implementation of periodicity conditions

On a pair of parallel opposite boundary surfaces, the displacements can be written as

$$\begin{aligned} u_{i}^{k^+}={\tilde{\varepsilon }}_{ij}x_{j}^{k^+}+u_{i}^{*}, u_{i}^{k^-}={\tilde{\varepsilon }}_{ij}x_{j}^{k^-}+u_{i}^{*} \end{aligned}$$

(73)

in which the kth pair of two parallel opposite boundary surfaces of a repeated unit cell is identified by indices “$k^+$” and “$k^-$”. It is important to note that at the two parallel boundaries (periodicity) $u_{i}^{*}$ is exactly the same; thus, the difference between $u_{i}^{{k}^+}$ and $u_{i}^{{k}^-}$ in the above two equations yields

$$\begin{aligned} u_{i}^{k^+}-u_{i}^{k^-}={\tilde{\varepsilon }}_{ij}(x_{j}^{k^+} -x_{j}^{k^-}){\tilde{\varepsilon }}_{ij}\Delta x_{j}^{k} \end{aligned}$$

(74)

with ${\tilde{\varepsilon }}_{ij}$ being the macroscopic (average) strains of the unit cell. For a specified macro-strain ${\tilde{\varepsilon }}_{ij}$, the right-hand side of (74) becomes constant owing to constant quantities $\Delta x_{j}^{k}$ for each pair of the parallel boundary surfaces. Instead of giving Eq. (73) directly as boundary conditions, the constraint equations are imposed as nodal displacement constraint ones.

In order to estimate effective elastic properties with specific boundary conditions imposed on the microstructure, a strain energy-based method is exploited. The total strain energy stored in the unit cell is equal to the energy of an equivalent homogeneous continuum achieved through the prescribed strain/stress fields. In the elastic phase, the macroscopic behaviors of a unit cell can be represented by the effective strain tensor $E_{ij}$ and stress tensor $\sum _{ij}$ over the homogeneous equivalent model. The relation between $\sum _{ij}$ and $E_{ij}$ can then be written as:

$$\begin{aligned} \sum \nolimits _{ij}=K^\mathrm{hom}E_{ij} \end{aligned}$$

(75)

where $K^\mathrm{hom}$ is the effective (homogenized) stiffness matrix, $\sum _{ij}=\frac{1}{V_{u}}\int _{V_{u}} \sigma _{ij}\hbox {d}V_{u}$ and $E_{ij}=\frac{1}{V_{u}}\int _{V_{u}} {\varepsilon _{ij}\hbox {d}V_{u}}$ stand for the volume averaging of the microscopic stress and strain tensors, $\sigma _{ij}$ and $\varepsilon _{ij}$, respectively, and $V_{u}$ refers to the volume of the unit cell.

In a 2D state, Eq. (75) can then be rewritten as follows:

$$\begin{aligned} \left[ {\begin{array}{*{20}c} {\sum }_{xx}\\ {\sum }_{yy}\\ {\sum }_{xy}\\ \end{array} } \right] =\left[ {\begin{array}{*{20}c} K_{11}^\mathrm{hom} &{} K_{12}^\mathrm{hom} &{} K_{16}^\mathrm{hom}\\ K_{12}^\mathrm{hom} &{} K_{22}^\mathrm{hom} &{} K_{26}^\mathrm{hom}\\ K_{16}^\mathrm{hom} &{} K_{26}^\mathrm{hom} &{} K_{66}^\mathrm{hom}\\ \end{array} } \right] \left[ {\begin{array}{*{20}c} E_{xx}\\ E_{yy}\\ E_{xy}\\ \end{array} } \right] . \end{aligned}$$

(76)

The strain energy density over the unit cell $U_\mathrm{cell}$ can be obtained from the following expression:

$$\begin{aligned} U_\mathrm{cell}=\frac{1}{2}\left[ {\begin{array}{*{20}c} \sum \nolimits _{xx} &{} \sum \nolimits _{yy} &{} 2\sum \nolimits _{xy}\\ \end{array} } \right] \left[ {\begin{array}{*{20}c} E_{xx}\\ E_{yy}\\ E_{xy}\\ \end{array} } \right] =\frac{1}{2}\left( \sum \nolimits _{xx}E_{xx}+\sum \nolimits _{yy}E_{yy} +2\sum \nolimits _{xy}E_{xy}\right) . \end{aligned}$$

(77)

The components of the stiffness tensor for the unit cell can then be computed through the periodic boundary conditions imposed over a unit cell of domain $\Omega$ with boundary $\partial \Omega$. From there, the following six elementary tests are considered:

Load case 1: Prescribed unit strain state in the x-direction, $E_{xx}=1,{E_{yy}=E}_{xy}=0$.

The corresponding displacement boundary conditions: $u=x, v=0$, on $\partial \Omega$, yielding:

$$\begin{aligned} K_{11}^\mathrm{hom}={2U_\mathrm{cell}} / V_{u}. \end{aligned}$$

(78)

Load case 2: Prescribed unit strain state in the y-direction, $E_{xx}=E_{xy}=0,{E}_{yy}=1$.

The corresponding kinematic boundary conditions: $u=0, v=y$, on $\partial \Omega$, leading to:

$$\begin{aligned} K_{22}^\mathrm{hom}={2U_\mathrm{cell}} / V_{u}. \end{aligned}$$

(79)

Load case 3: Prescribed biaxial strain state, $E_{xx}=E_{yy}=1,{E}_{xy}=0$.

The corresponding boundary conditions: $u=x, v=y$, on $\partial \Omega$, resulting in:

$$\begin{aligned} K_{12}^\mathrm{hom}=\left( {2U_\mathrm{cell}} / V_{u}-K_{11}^\mathrm{hom}-K_{22}^\mathrm{hom} \right) / 2. \end{aligned}$$

(80)

Load case 4: Prescribed shear strain state, $E_{xx}=E_{yy}=0,{E}_{xy}=1$.

The corresponding kinematic boundary conditions: $u=y/2, v=x/2$, on $\partial \Omega$, from which we obtain:

$$\begin{aligned} K_{66}^\mathrm{hom}={2U_\mathrm{cell}} / V_{u}. \end{aligned}$$

(81)

Load case 5: Prescribed coupling strain state, $E_{xx}=E_{xy}=1,{E}_{yy}=0$.

The kinematic boundary conditions in this case: $u=x+y/2, v=x/2$, on $\partial \Omega$, from which we get:

$$\begin{aligned}&\left[ {\begin{array}{*{20}l} {\sum }_{xx}\\ {\sum }_{yy}\\ {\sum }_{xy}\\ \end{array} } \right] =\left[ {\begin{array}{*{20}c} K_{11}^\mathrm{hom} &{} K_{12}^\mathrm{hom} &{} K_{16}^\mathrm{hom}\\ K_{12}^\mathrm{hom} &{} K_{22}^\mathrm{hom} &{} K_{26}^\mathrm{hom}\\ K_{16}^\mathrm{hom} &{} K_{26}^\mathrm{hom} &{} K_{66}^\mathrm{hom}\\ \end{array} } \right] \left[ {\begin{array}{*{20}l} 1\\ 0\\ 1\\ \end{array} } \right] =\left[ {\begin{array}{*{20}l} K_{11}^\mathrm{hom}+K_{16}^\mathrm{hom}\\ K_{12}^\mathrm{hom}+K_{26}^\mathrm{hom}\\ K_{16}^\mathrm{hom}+K_{66}^\mathrm{hom}\\ \end{array} } \right] \nonumber \\&\quad \Longrightarrow K_{16}^\mathrm{hom}=\left( {2U_\mathrm{cell}} / V_{u}-K_{11}^\mathrm{hom}-2K_{66}^\mathrm{hom} \right) . \end{aligned}$$

(82)

Load case 6: Prescribed coupling strain state, $E_{xx}=0,{E}_{yy}=E_{xy}=1$.

The boundary conditions in this situation: write $u=y/2, v=x/2+y$, on $\partial \Omega$, allowing to achieve:

$$\begin{aligned}&.\left[ {\begin{array}{*{20}l} {\sum }_{xx}\\ {\sum }_{yy}\\ {\sum }_{xy}\\ \end{array} } \right] =\left[ {\begin{array}{*{20}c} K_{11}^\mathrm{hom} &{} K_{12}^\mathrm{hom} &{} K_{16}^\mathrm{hom}\\ K_{12}^\mathrm{hom} &{} K_{22}^\mathrm{hom} &{} K_{26}^\mathrm{hom}\\ K_{16}^\mathrm{hom} &{} K_{26}^\mathrm{hom} &{} K_{66}^\mathrm{hom}\\ \end{array} } \right] \left[ {\begin{array}{*{20}c} 0\\ 1\\ 1\\ \end{array} } \right] =\left[ {\begin{array}{*{20}c} K_{12}^\mathrm{hom}+K_{16}^\mathrm{hom}\\ K_{22}^\mathrm{hom}+K_{26}^\mathrm{hom}\\ K_{26}^\mathrm{hom}+K_{66}^\mathrm{hom}\\ \end{array} } \right] \nonumber \\&\quad \Longrightarrow 2K_{26}^\mathrm{hom}=\left( {2U_\mathrm{cell}} / V_{u}-K_{22}^\mathrm{hom}-3K_{66}^\mathrm{hom} \right) . \end{aligned}$$

(83)

The components of the effective stiffness matrix, quantities $K_{11}^\mathrm{hom},K_{22}^\mathrm{hom},K_{12}^\mathrm{hom},K_{66}^\mathrm{hom},K_{16}^\mathrm{hom},K_{26}^\mathrm{hom}$, as well as the equivalent moduli, coupling coefficients in the constitutive law, Eq. (72) and Poisson’s ratio are computed from the above numerical analyses.