The chips are down: the influence of family on children’s trust formation

Abstract

Understanding the formation of trust is a key issue because of the impact of trust on economic performance. Earlier attempts to measure the strength of intergenerational transmission of trust relied on the cross-sectional regression of children’s trust on the contemporaneous trust of parents. In this paper, we take an original approach to the analysis of the transmission process by introducing the distinction between permanent trust (the long-lasting belief on whether one trusts people) and transient trust (capturing, e.g., random errors in the reported trust), and argue that only permanent trust is relevant for the transmission process. Using data from the German Socio-Economic Panel, we show that 2/3 of the observed variability in children’s trust is due to the transient component. The remaining variability due to the permanent component is only moderately determined by the permanent trust of the parents, with mothers being much more relevant than fathers. Focusing on the subsample of families with more than one child, we show that most of the variability in children’s permanent trust is due to unobservable family-specific features of the environment shared by siblings. We conclude that while the family environment in which children grew up determines most of their permanent trust, the direct role of intergenerational transmission is small.

1 Introduction

The role of culture on economic choices and its effect on economic development have been the subject of a lively debate in recent research. Trust towards others is one of the cultural traits most studied by social scientists (see Alesina and Giuliano (2015), for a review). Following the seminal contributions of Banfield (1958), Coleman (1988), and Coleman (1990) and Putnam (1993) and Putnam (2000), trust has been found to affect economic development (Knack and Keefer 1997), innovation (Fukuyama 1995), individual performance (Butler et al. 2016), financial development and trade (see Guiso et al. 2004, 2008b, 2009), and firm productivity (Bloom et al. 2012; La Porta et al. 1997).¹

Considering the important influence of trust on economic outcomes, the process of its formation is of paramount interest. The economic literature has long been interested in studying the evolution of trust over time and its long-term economic impacts (e.g., Guiso et al. 2006; Guiso et al. 2016; Tabellini 2010; Nunn and Wantchekon 2011; Becker et al. 2016). One of the common findings of these studies is that trust and other values possess a persistent component. However, what exactly drives such persistence is an unanswered question. One hypothesis is that intergenerational transmission of trust is one of the main mechanisms behind it. In this context, the theoretical work of Bisin and Verdier (2001) has highlighted the role of intergenerational transmission of values such as trust in explaining the persistence of ethnic differences.

Recent studies have provided empirical content to the intergenerational transmission of values. Notably, Dohmen et al. (2012), using data from the German Socio-Economic Panel (SOEP), analyze the transmission of trust and risk attitudes from parents to children within a regression framework whereby children’s attitudes are modelled as a function of those of parents. Their results suggest the presence of a positive intergenerational correlation.²

The aim of this paper is to build upon the existing literature on intergenerational transmission of trust by taking an original approach to how the transmission process is empirically analyzed. In particular, our main contribution is to introduce the distinction between the permanent and transient components of trust. We develop an analytical framework within which we postulate that only permanent trust matters for intergenerational transmission, while the transient component is irrelevant to intergenerational transmission because it captures unpredictable shocks that do not influence parents’ long-lasting level of trust (e.g., events happening on the day of the interview that affect just the reported level of trust or random errors in the reported trust). We subsequently introduce this innovation into the standard model for intergenerational transmission of trust (e.g., used by Dohmen et al. (2012)), pinning down the econometric implications of the distinction between permanent and transient trust. Our empirical exercise revolves around the standard notion of explained variability, i.e., how much of the variability of children’s permanent trust is explained by their parents’ permanent trust. The availability of longitudinal data is crucial to disentangle the two components of trust. We exploit three waves of the German Socio-Economic Panel, which allows us to model the dynamics of individual trust over a decade. One of the challenges brought by our framework is that permanent trust is unobservable to the econometrician. To circumvent this hurdle, we show that a measure of the explained variance of permanent trust can be obtained by using the lagged trust of parents as an instrumental variable for current parental trust.

The most remarkable finding of our analysis is that after accounting for the large fraction of the variance of observed trust due to the transient component, the role of parents’ permanent trust in the intergenerational transmission process is moderate. In line with previous work, but with a stronger effect, we also find that it is the mother that has a substantial role in the transmission of permanent trust to children. The correlation between the permanent trust of fathers and of children is instead spuriously attributable to the strong correlation between the permanent trust of the two parents. Ultimately, our empirical exercise demonstrates that parents’ permanent component of trust only explains a small fraction of the variance of children’s (permanent) trust and this is too low a value to drive the long-term persistence of trust observed at the aggregate level and reported in the literature.

Motivated by this finding, and to further investigate what explains the variability in children’s permanent trust, we focus our attention on the subsample of families with more than one child. This allows us to disentangle the role of the direct transmission of trust from parents to children from that played by other factors of the environment shared by siblings. Remarkably, our analysis reveals that approximately 60% of the variance of children’s permanent trust is attributable to a family-specific effect, pointing to the existence of environmental factors shared by siblings that are independent of their parents’ trust but relevant to the formation of their own trust. This result demonstrates that the direct role of intergenerational transmission of (permanent) trust is rather exiguous compared to the impact of the overall family environment in which children grew up.

Our paper adds to the existing literature on the intergenerational transmission of trust along an additional dimension. As an instrumental part of our analysis, we test a crucial assumption implicit in the literature, namely that individual trust is invariant over time (at least from late adolescence/early adulthood). This conjecture has been the important but somewhat controversial argument on which the existing empirical literature hinges. It is crucial because parents’ and children’s trust used in regression analyses are contemporaneously measured at the time of the interviews, whereas ideally they should be gauged at the time the transmission took place. These two measurements are equivalent only if trust is invariant over time. That is a controversial hypothesis too since, for instance, in their US longitudinal study, Poulin and Haase (2015) find that generalized trust changes with age. Using the three-wave structure of our panel dataset, we test for the invariance of the permanent component of trust and find no evidence to reject it, even if only within the 10-year window available in our longitudinal sample.

The remainder of the paper is organized as follows. Section 2 provides a brief review of the literature on cultural transmission. Section 3 outlines a framework where we introduce the distinction between permanent and transient trust and clarifies a necessary condition required to attach a structural interpretation to the regression parameters. Section 4 describes the data and the econometric model. Section 5 presents the main results of our analyses. Section 6 follows with a discussion of our results and of their implication for the literature on long term persistence. Section 7 concludes.

2 Theoretical background

To better understand the transmission of trust within the family, we briefly review the literature about cultural transmission. The first theoretical frameworks for the study of cultural transmission are due to Cavalli-Sforza and Feldman (1981) and Boyd and Richerson (1988), who apply models of evolutionary biology to the transmission of beliefs, preferences and norms. These works show how cultural traits can be acquired through learning and other forms of social interactions. Cultural transmission is seen as the result of the direct vertical socialization (the role played by parents), and the horizontal and oblique socializations (taking place in the society). Horizontal and oblique socializations can be described as imitation and learning behaviors, and refer mainly to the interactions with peers and the environment outside the family. Cultural transmission is different from genetic evolution, although the two can interact. The distinct effects of the cultural, environmental, and genetic factors on cognitive and non-cognitive skills of an individual is at the core of a lively debate on “nature” versus “nurture”, which is the object of study of several disciplines, from behavioral genetics to social sciences (for a survey, see Sacerdote (2011)).

With the growing evidence of the persistence of ethnic and religious traits across generations, cultural transmission has recently gained new emphasis in the theoretical and empirical literature. It has been documented how migrants generally struggle to maintain specific traits of the culture of the country of origin. The cultural renaissance of several ethnic and religious communities in the USA apparently endangered (Orthodox Jews, for example) is a significant case. Similarly, Africa has witnessed the persistence of tribal distinctions even after the emergence of national institutions.³ Bisin and Verdier (2000) and Bisin and Verdier (2001) have significantly extended existing models. In particular, they introduced the parental socialization choice, which is motivated by what they call imperfect empathy. In their framework, parents are altruistic and care about children’s choices, which are however evaluated using the parents’ preferences. Children acquire traits through their parents’ socialization choices and by learning from the social environment in which they grow up. Parents choose the optimal socialization effort taking into consideration also the environment, with their choices depending on the distribution of the population with respect to the relevant trait. Bisin et al. (2009) extend this model by analyzing multi-trait populations.

In the following sections, we endeavor to reconcile vertical and horizontal socialization within the family by quantitatively estimating and distinguishing the roles of the intergenerational correlation (deemed to capture vertical socialization) and the residual sibling correlation (which is thought to embody horizontal socialization).

3 Analytical framework

3.1 Permanent vs transient trust

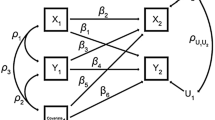
We develop a framework that brings in the novel distinction between permanent and transient trust within the classic econometric model for studying the intergenerational transmission process. As a starting point, we postulate that the observable level of trust of individual i at time t is:

$$\begin{array}{@{}rcl@{}} Tk_{it}=Tk^{p}_{it}+vk_{it}; \quad k \in(c, m, f) \end{array}$$

(1)

where c represent the child, m the mother and f the father. Here, $Tk^{p}_{it}$ is the permanent level of trust at time t and vk_it is a zero mean transient shock uncorrelated over time and unrelated to past, current and future values of the permanent trust. In other words, observed trust is a combination of permanent trust and a transitory shock, where the latter—econometrically speaking—is essentially measurement error.

To fix ideas, let the evolution of the permanent level of trust over time be driven by the following model:

$$\begin{array}{@{}rcl@{}} Tk^{p}_{it}=\rho Tk^{p}_{it-1}+(1-\rho) uk_{it}; \quad k \in(c, m, f) \end{array}$$

(2)

where uk_it is a permanent shock hitting $Tk^{p}_{it}$ at time t. The permanent shock is uncorrelated over time and uncorrelated to past values of the permanent trust.

The intuition motivating this model is as follows. $Tk^{p}_{it-1}$ is the level of permanent trust of individual i at time t − 1 summarizing events up to time t − 1 relevant to their lasting belief on whether one can trust people. At time t the individual experiences the unpredictable shocks (uk_it,vk_it). The component uk_it brings in news that are relevant to the lasting belief of the individual, who will therefore update his/her permanent trust according to Eq. (2). The component vk_it affects the current level of observable trust but does not bring any news relevant to the individual’s lasting belief. This component might include events happening on the day of the interview and affecting just the reported level of trust on that day, or random errors in the reported trust. Consequently, vk_it does not leave any trace on the individual’s future belief.

This simple framework has an important implication for measuring trust, namely that observable and permanent trust are different. Previous studies do not contemplate a transient component of trust, implicitly assuming that the transient shock vk_it is negligible. Presumably, however, only permanent trust is relevant for the intergenerational transmission, in that transient shocks—being uninformative about the updating process of individuals—are unlikely to be passed to the child. In the next section, we will show that the latter is actually a testable implication of the model (see Sections 4.2 and 5.2). Since the individual’s permanent trust is observable only up to vk_it, the presence of a transient shock raises the classic measurement error problem to the purpose of the econometric identification of the intergenerational transmission.

Our model has a straightforward but fundamental consequence on how the standard equation relating the permanent trust of children to the contemporaneus permanent trust of their parents should be written:

$$\begin{array}{@{}rcl@{}} Tc^{p}_{it}={\upbeta}_{0}+{\upbeta}_{1} Tf^{p}_{it}+{\upbeta}_{2} Tm^{p}_{it} + \epsilon_{it} \end{array}$$

(3)

where the subscript t refers to the time of the interview. Our equation is similar to the one adopted in the existing literature on intergenerational transmission of trust (e.g., Dohmen et al. 2012), but the important novelty is that in our framework we emphazise that it is the permanent trust which is passed on from parents to their children.

Equation (3) cannot be directly estimated, because permanent trust is unobserved. We can however obtain a feasible version of the transmission equation by replacing the unobservable permanent trust of children and of their parents by their error-ridden observable counterparts (i.e., combining Eqs. (1), (2), and (3)):

$$\begin{array}{@{}rcl@{}} Tc_{it}={\upbeta}_{0}+{\upbeta}_{1} Tf_{it}+{\upbeta}_{2} Tm_{it} + \epsilon_{it} + vc_{it} - {\upbeta}_{1} vf_{it} - {\upbeta}_{2} vm_{it} \end{array}$$

(4)

This raises the problem of how to estimate this feasible equation taking into account the endogeneity induced by the measurement errors in the observable trust of parents (as well as by the possible correlation between the measurement errors of parents and of their children). Our identification strategy outlined in Section 4 will tackle all these issues.

3.2 Aspects about Identification

Following the literature (e.g., Dohmen et al. 2012), to estimate our model, we relate children’s trust as reported at a specific point in time to their parents’ trust as reported at the same point in time. The feasible regression looks like Eq. (4). In our case, we use trust observed in 2013 for individuals—children and their parents—that are at least 17 years old (see below Section 4.1). There are several issues that one needs to carefully take into account in order to attach a meaningful interpretation to the results of this exercise.

To begin with, note that with the type of data we—as well as previous studies—use, it is not possible to model the way in which transmission from parents to children took place from early childhood to late adolescence. A crucial precondition to do this would be to observe trust of children during childhood and adolescence as well as their parents’ trust during the same span of time.⁴ A feasible and interesting alternative is to model the link between the level of permanent trust of children at the age when the intergenerational transmission is presumably completed and the trust that parents put in the process up to that time. This is a kind of reduced form model that links the inputs—trust of parents—to the output—trust of children—skipping over the circumstances inside the black box of the transmission process.

Even recasting the problem this way, several issues persist. First, the trust that parents input in the transmission process is their trust when transmission took place, not the one we observe at the time of the interview. Similarly, one should use the trust of children by when the transmission process was completed (say by age of 17), not the level of trust at the time of the interview. One way to bypass this problem is to assume that the level of trust of parents and children did not change from when transmission was completed to the time of the interview. This seems to be the implicit assumption researchers typically have in mind when they regress the trust of children observed at time t on the trust of parents observed at the same time t: by invoking time invariance of trust what they observe at time t is a reasonable proxy of what they would like to observe.

The assumption of time invariance of trust can be easily recast in our framework with reference to the permanent component of trust by setting ρ = 1 in Eq. (2), i.e., ruling out the existence of permanent shocks to trust. In Section 4, we show that this invariance assumption implies testable restrictions as long as one can observe trust on a sample of individuals at least in three points in time. Of course, by not rejecting the null hypothesis one can only say that the evidence available from the specific time window available in the longitudinal dataset is consistent with the hypothesis of time invariance of trust. Still, by not rejecting the null hypothesis one has available a firmer basis to claim that the regression of children’s (older than 17) trust on their parents’ trust identifies the amount of trust passed on from parents to children by the end of the transmission process.

4 Econometrics

4.1 Data

Our sample of parents and children is drawn from the German Socio-Economic Panel (SOEP). The SOEP is a large longitudinal survey extensively used by economists and that has been the basis for intergenerational studies (see, e.g., Dohmen et al. (2012)). The survey was introduced in West Germany in 1984 and collected data on 12,000 households; in 1990, it was extended to include about 2,000 households from East Germany.⁵ SOEP collects information on trust on all family members aged 17 and above. Two features of SOEP are key to our study. First, the survey “tracks” individuals, which means that those who move internally in Germany can still be followed over time, thereby reducing attrition. Second, it provides identifiers to match children with their biological parents. This feature is essential in order to construct families and observe them over time. A family is defined as the parental couple (mother and father) and their biological child(ren). Given the structure of SOEP, it is not necessary for the family members to live in the same household in order to be observed in the panel.⁶

We include in the sample all couples who took part into the survey in waves 2003, 2008, and 2013 with at least one child of age 17 or older in 2013. Crucial to our analysis, this sample selection implies that we observe the trust of both parents in three time periods. Defining our working sample this way, the trust of children included in the sample is observed at least in 2013. For a subset of children, trust is also observable in either or both the previous waves (2003 and 2008) provided they were at least 17 and present during the survey. The resulting panel comprises 1,627 children within 1,109 families.

As in Dohmen et al. (2012), the key variable of our analysis is trust, measured as the first principal component obtained from the principal component analysis of the three questions on trust contained in the SOEP. These are as follows: general trust (“On the whole, one can trust people”); reliance on others (“These days you cannot rely on anybody else”); caution with strangers “When dealing with strangers, it is better to be careful before you trust them”). All variables are measured on a four-point scale. We reversed the scale of the responses for the variables “Reliance on others” and “Caution with strangers”, so that larger values reflect higher values of trust. We then extract the principal components for children and their parents. Table A1 in the Appendix reports the correlation of the three trust measures with the first component, as well as the variance explained by the first component. From the SOEP, we derive additional variables, including gender, age, number of siblings, nationality, education and information on the place of residence when aged 15. We additionally include the average level of trust in the region, following the argument of Dohmen et al. (2012) that trust in the area of residence might affect children’s trust or the transmission process.⁷ The age distribution of fathers, mothers, and children in 2013 is set in Fig. 1.

In Table 1, we report the distribution of families by number of children. The left panel reports the distribution of families by the number of children included in our sample in 2013, while the right panel reports the distribution of families by the total number of children (as reported in 2013). This total includes also children who are outside the sample (e.g., because they are still younger than 17 or because they were not originally sampled). An obvious question is whether missing (i.e., not in sample) children are an ignorable issue. To provide an indirect evidence about this, we first split the families into two subgroups: those with all children in the sample and those with at least one child out of the sample. Then, we compare the average level of trust of parents and of children included in the sample across the two subgroups. Results are in Table 2: differences are small and statistically insignificant at the conventional level. Based on this evidence, from now on, we proceed assuming that having missing children is an issue that can be ignored.

A remarkable aspect that emerges from a deeper inspection of the raw data—and not detectable with cross-sectional studies—is the variability of observed trust over time. Figure A1 in the Appendix shows the graphs of the difference in the level of trust for two consecutive waves, for both fathers and mothers. The graphs reveal the existence of substantial variability of trust between periods.

Additional evidence about this aspect comes from Table 3, where we report autocovariance matrices of trust for the three waves forming our sample. The results are reported separately for fathers, mothers, and children and for whether we include or not additional covariates in the computation of the covariances.⁸ A cursory inspection of these matrices immediately reveals that the observable trust is far from stable over time, complementing what observed in Figure A1 in the Appendix. The autocorrelation of order one is in the range 0.57–0.67 when we add control variables. This is in stark contrast with the assumption—implicit in the existing empirical literature—that trust is stable over time. We argue that the low degree of persistence observed in our data is due to the transient component of trust, as defined in Eq. (1). This implies that we need to establish whether the permanent component of trust—i.e., the one relevant for the intergenerational transmission according to our hypothesis—is invariant over time. The evidence in Table 3 will be the basis for our test for the invariance of permanent trust over time developed in the next section.

4.2 Specification testing and estimation

The testable implication of the invariance condition is:

$$cov\{Tk_{i 2003}, Tk_{i 2008}\}=cov\{Tk_{i 2003}, Tk_{i 2013}\}=cov\{Tk_{i 2008}, Tk_{i 2013}\} \quad k\in{c,f,m} .$$

(5)

In words, if the permanent trust $Tk^{p}_{it}$ does not vary over time and the variation over time of the observable trust Tk_it is only due to random shocks uncorrelated over time, then the covariance between the observable trust at time t and at time s equals the variance of the permanent trust for any choice of (t,s). That is, if the permanent trust is invariant over time, the three covariances in each panel of Table 3 should be equal (up to sampling variability).

Condition (5) could be violated due to different reasons. Particularly relevant to our case, it would not hold if the transient shocks were correlated at lag 1. It would also be violated if the equation driving the dynamics of Tk^p were as in Eq. (2). In both cases the covariance between observable trust in (2013, 2008) would be different from the corresponding covariance in (2013, 2003).

To implement the test, note that (5) is equivalent to:

$$cov\{Tk_{i 2003}, Tk_{i 2008}-Tk_{i 2013}\}=cov\{Tk_{i 2008}, Tk_{i 2003}-Tk_{i 2013}\}=cov\{Tk_{i 2013}, Tk_{i 2003}-Tk_{i 2008}\} = 0.$$

(6)

To test the first condition, it is sufficient to perform the regression of Tk_i2008 − Tk_i2013 on Tk_i2003 (or the other way around) and check whether the regression coefficient is zero. The same applies to the remaining two conditions. Clearly, at least three waves of trust data for the same individuals are needed to perform the test.

On accepting the invariance condition (5), the decomposition of the variance of the observable trust into its components due to the permanent trust and to the transient shock, respectively, proceeds in the following way:

$$\begin{array}{@{}rcl@{}} var\{vk_{it}\}=var\{Tk_{it}\}-var\{T{k^{p}_{i}}\} \end{array}$$

(7)

with $T{k^{p}_{i}}$ identified using Eq. (5). Finally, to estimate the parameters of the feasible transmission Eq. (4), note that Tf_it− 1 and Tm_it− 1 are valid instrumental variables for Tf_it and Tm_it provided that the transient shock is not correlated over time. Also, note that with a panel of length three the model is overidentified since Tf_it− 2 and Tm_it− 2 are valid instruments as well. To sum up, failing to reject restriction (5), we conclude that the evidence we have from the specific time window covered by our our three-wave panel is that permanent trust is invariant and transient shocks are serially uncorrelated. Consequently, trust of mother and father in 2003 and 2008 are valid instrumental variables for the regression of children’s trust on their parents’ trust in 2013.⁹

Also, note that this setting provides the basis for an additional test of the hypothesis of no autocorrelation of the transient shock. Under the alternative hypothesis of autocorrelated shocks, the IV at time t − 1 is plausibly more correlated to the disturbance term in Eq. (4) than the IV at time t − 2. Therefore, the Sargan overidentification test should detect a violation of the null hypothesis. The same test is in principle useful also to detect a violation of our conjecture that transient shocks of parents’ trust are irrelevant for the transmission process. If these shocks were otherwise relevant, the exclusion restriction on our candidate IV would not hold since past values of parents’ observable trust would matter for current values of children’s observable trust, even conditional on the current values of parents’ permanent trust. Since the degree of violation of the exclusion restriction is likely to vary with the lag of the instrument, the Sargan overidentification test should detect whether the null hypothesis does not hold.

Last but not least, key to the identification of the structural parameters in Eq. (3) is controlling for confounders which could be correlated to the trust of parents and children. To deal with this issue, we check the sensitivity of the estimates of (4) to the inclusion of several observables.

To quantify the strength of the transmission process, we follow the standard practice in the literature on intergenerational transmission and consider the fraction of the variance of Tc^p explained by (Tf^p,Tm^p), i.e., the R² of regression (3). This depends on both the size of the coefficients β₁ and β₂ and the degree of correlation between the permanent trust of parents:

$$\begin{array}{@{}rcl@{}} {{\upbeta}_{1}^{2}} var\{Tf^{p}_{it}\} + {{\upbeta}_{2}^{2}} var\{Tm^{p}_{it}\} + 2 {\upbeta}_{1} {\upbeta}_{2} cov\{Tf^{p}_{it}, Tm^{p}_{it}\}. \end{array}$$

(8)

Distinguishing between observable and permanent trust is crucial to properly assess the extent to which children inherit trust from their parents since it is clear that, even leaving aside the issue of how to estimate the coefficients β₁ and β₂, the relevant R² should be evaluated with respect to the variance of Tc^p and not of Tc. Whether this distinction is important is an empirical issue that we will tackle in Section 5.1, where we provide an estimate of the variance of the two components.

The variance of Tc^p explained by the regression can be calculated according to expression (8). The variance of $Tc^{p}_{it}$, $Tf^{p}_{it}$, and $Tm^{p}_{it}$ are derived as a corollary of the invariance condition in Eq. (5). A convenient way to recover the covariance between the permanent trust of parents is to perform a regression of Tf_it on Tm_it using Tm_it− 1 and Tm_it− 2 as an IV to eliminate the bias due to the measurement error. This is a consistent estimate of the regression coefficient of $Tf^{p}_{it}$ on $Tm^{p}_{it}$. The next step is to rescale the estimated coefficient by $var\{Tm^{p}_{it}\}$ to obtain the covariance between the trust of parents.

4.3 Sibling correlation in trust

To investigate further the role of the family environment in the transmission process, we use families with more that one child in the sample—which are about 38% of our sample (see Table 1). The availability of siblings in the data allows to estimate a transmission equation which includes a family specific unobservable effect. This can be achieved by estimating a modified version of Eq. (3):

$$\begin{array}{@{}rcl@{}} Tc^{p}_{ij}={\upbeta}_{0}+{\upbeta}_{1} T{f^{p}_{j}}+{\upbeta}_{2} T{m^{p}_{j}} + \alpha_{j}+\epsilon_{ij} \end{array}$$

(9)

The subscript ij refers to children belonging to the same family j (we drop the time suffix for ease of exposition).

According to how our econometric model is specified, the family specific effect a_j is uncorrelated to the parental permanent trust. It accounts for what is left of parental influences after accounting for their permanent trust, as well as for other environmental factors shared by siblings and not accounted for by parents’ trust. Schools, friendship networks, and other circumstances operating at the community level are examples of this family-specific component shared by siblings. Similar to Bingley and Cappellari (2019), while we are able to measure the direct transmission of trust from parents to their children, with the available data we are able to measure the relevance of these other channels of intergenerational transmission of trust but we cannot identify them. Also, note that a possible interpretation for a_j is that it captures (also) the heterogeneity of the transmission parameters across families.

There are two important remarks about the identification of var{α_j} and its interpretation. First, since the identification of the variance is based on the between-sibling covariance of the residuals from the feasible IV regression of Tc on Tf and Tm, var{α_j} could partially capture the correlation between the transient shocks of siblings. However, the null hypothesis of no correlation between the transient shocks of siblings is testable. Under this null hypothesis, the covariance between the trust of one sibling in 2013 and the trust of another sibling at, say, time t, does not depend on t since it is equal to the covariance between the permanent trust of the two siblings. We implement this test in the same way as in Eq. (6).

Second, var{α_j} strictly refers to families with at least two children in the sample. Note, however, that the overall (i.e., including out of sample) number of siblings—and thus of families with more than one child—is much larger. The second panel of Table 1 shows that nearly 85% of the families in our sample have more than one child, meaning that the estimate of var{α_j} is virtually representative of the majority of our sample (provided that the abovementioned assumption of ignorability of the missing children holds).

5 Results

5.1 Testing for invariance of permanent trust

Table 4 presents the results of the test for invariance of permanent trust separately for fathers, mothers, and children. After controlling for observables (columns 4 to 6), only in one case out of nine the null hypothesis is weakly rejected. This provides clear evidence that observable trust is equal to a time invariant component plus a random shock.

The two most important consequences of the tests in Table 4 for the identification of the transmission parameters is that by age 17 (and above)—i.e., the age at which the transmission of trust is presumably completed—the permanent trust of parents and children is not affected by permanent shocks, and the transient shocks are not serially correlated (at least over the time span 2003 to 2013).

Table 5 presents the decomposition of the variance of observable trust into the permanent trust and transient shock components. The main result here is that for mothers and fathers—after controlling for observables—the variance of permanent trust is approximately just less half of the total variance. This fraction is slightly smaller in the case of children.

5.2 Estimating the transmission parameters

Table 6 presents the results of the estimation of the feasible transmission Eq. (4) using observations on trust for t= 2013. We report both OLS and IV estimates with standard errors clustered at the family level. We also report the IV estimate including a family random effect to estimate the sibling correlation. Finally, we report the results both including and not including a set of controls. The instruments used are the first and second lag of trust for both fathers and mothers, i.e., trust observed in t= 2008 and t= 2003. The validity of our instruments is supported by the absence of autocorrelation of the transient shocks, for which we provided evidence in the previous section (see Table 4). Not surprisingly, given the size of the measurement error, the Durbin-Wu-Hausman test strongly rejects the hypothesis of exogeneity of Tf and Tm.

Given the result of the test on the autocovariance matrix of parents’ trust, it is not surprising that the Sargan overidentification test does not reject the validity of our IV. On the other hand, the Sargan overidentication test does not reject the null hypothesis adding further evidence in favor of the validity of our assumption of no autocorrelation for the transient shock.¹⁰ As pointed out in Section 4.2, this result provides support also to our conjecture that transient shocks of parents’ trust are irrelevant for the transmission process.

Focusing on the results for the regressions with controls and comparing the estimates in columns 4 and 6 of the table, one notices that despite the usual loss of precision, the IV estimate for the coefficient of mothers is strongly significant (0.335, s.e. 0.067) and twice as large as the OLS estimate (0.162, s.e. 0.030). On the other hand, the IV estimate for the coefficient of father is much closer to the OLS (0.129, s.e. 0.065 for the IV and 0.106, s.e. 0.031 for the OLS). Note that the same pattern of results holds for the regressions without controls in columns 1 and 3.

The evidence that accounting for measurement errors makes a major difference for the estimated coefficient for mothers while it does not matter at all for the estimated coefficient for fathers might seem puzzling in light of the textbook notion that measurement errors on the explanatory variable imply an attenuation bias. To provide an explanation, we make use of an approximation to the OLS bias due to measurement error proposed by Theil (1961), who shows that when there are two regressors both affected by measurement errors the approximate OLS bias is:

$$\begin{array}{@{}rcl@{}} bias({\upbeta}_{1})=-\frac{{\upbeta}_{1}\lambda_{1}}{1-\rho^{2}}+\frac{{\upbeta}_{2}\lambda_{2}\rho}{1-\rho^{2}} \end{array}$$

(10)

$$\begin{array}{@{}rcl@{}} bias({\upbeta}_{2})=-\frac{{\upbeta}_{2}\lambda_{2}}{1-\rho^{2}}+\frac{{\upbeta}_{1}\lambda_{1}\rho}{1-\rho^{2}} \end{array}$$

(11)

where ρ is the correlation coefficient between the true regressors and λ_j,j ∈{1,2} is the ratio of the variance of the measurement error to the variance of the respective observable regressor (i.e., the sum of the variances of the measurement error and of the true regressor). If ρ were equal to zero, the bias would collapse to the standard attenuation bias for both coefficients. In this instance, the correlation between the two explanatory variables is large (ρ is 0.55 for the model with controls); hence, the second component on the right-hand side of the equations has a positive sign, counterbalancing the standard attenuation bias, since both β₁ and β₂ are positive in our case. Deriving the values of λ_j and β_j from Tables 5 and 6 and plugging them in Eqs. (10) and (11), we obtain a bias for the coefficient of fathers of 0.04 in the model with controls, while the bias for the coefficient of mothers is -0.19. This is in line with the difference we observe between the OLS and the IV estimates in Table 6, also taking into account sampling variability.

We also replicated the main analysis by splitting the sample by gender of the child (Table A3 in the Appendix). Results are qualitatively similar to the baseline, although the effect of mother’s trust is stronger for female children.

The key result of this analysis is that a clear hierarchy emerges in the roles of mothers and fathers with the formers being more influential in the transmission process. The pattern of our estimates is consistent with Dohmen et al. (2012), although our results show a sharper difference between the coefficients of mothers’ and fathers’ trust. It is important to emphasize, though, that differently from Dohmen et al. (2012) who use observed trust in their analysis, the parameter estimates of our model refer to the intergenerational transmission of permanent trust.

As for the strength of the intergenerational transmission, we summarize it as the fraction of the variance of permanent trust of children explained by the permanent trust of parents. As a first step, we estimate the strength of the correlation between the permanent trust of fathers and mothers as outlined at the end of the previous section. The IV estimate of the regression of Tf^p on Tm^p is approximately equal to the correlation coefficient between the two variables and is 0.537 (s.e. 0.068) for the model with controls.¹¹ The R² pertinent to the transmission process is about 0.24 in the model with controls. In words, this means that a large fraction of the variability of the permanent trust of children is not attributable to the parents’ permanent trust. Note that failing to distinguish between permanent trust and transient shocks would result in a severe underestimation of the strength of the transmission process.

Turning to the results of the random effect specifications, we notice that the pattern of estimates are similar to the IV model estimated without considering sibling correlations. The striking result, however, lies in the estimated contribution of the family-specific unobservable a_j component to the variance of Tc^p. The ratio of var{α_j} to var{Tc^p} is 2.4 times larger than the contribution of the parents’ permanent trust when controls are included.¹² Taken together, family-specific characteristics—whether observable (permanent trust of parents) or unobservable (α_j)—account for more than 80% of the variance of children’s permanent trust.

Table A5 in the Appendix shows that the sibling correlation estimate is not biased by the correlation between transient shocks of siblings, with only few tests rejecting the null hypothesis.

6 Discussion

In Table 7, we present the decomposition of the variance of the trust of children in 2013. Two striking facts emerge. First, the observed variability of the children’s trust is dominated by random shocks—nearly 2/3 of the total variance in the model with controls—with permanent trust accounting for the remaining 1/3. As explained in Section 3, we identify the size of these components by exploiting the longitudinal variation of trust.

Second, less than one-fourth of the variance of children’s permanent trust is attributable to the direct transmission of permanent trust from parents. We identify the size of this component by exploiting the correlation between children’s and parents’ trust (accounting for the attenuation bias due to transient shocks).

Then, approximately 60% of the variance of children’s permanent trust is attributable to the family specific effect a_j. It captures characteristics of the environment—within or outside the family—which are shared by siblings and are uncorrelated to parental permanent trust. As explained in Section 4.3, in principle also this component might include intergenerationally transmitted trust through channels that work independently from parents’ trust. Furthermore, residual sibling correlations could also be due to the heterogeneity of the transmission parameters across families.

Overall, direct transmission of trust from parents together with family-specific effects account for more than 80% of the variance of the permanent trust of children. Even if the evidence we provide emphasizes the major role played by the family environment in shaping children’s trust, it is clear that the direct transmission from parents plays a minor role in the persistence of trust over generations.

One challenge is how to reconcile our evidence with some results coming from the literature on long term persistence of trust. For example, Guiso et al. (2016) show that the establishment of free cities in Center-North Italy during the medieval period generated a positive shock in the accumulation of social capital in the affected municipalities which is perceivable even nowadays. In a companion paper, the authors develop a theoretical model to show how the intergenerational transmission of trust is compatible with their empirical evidence (Guiso et al. 2008a).

A possible argument to reconcile our evidence of a weak “short run” intergenerational transmission effect with the results by Guiso et al. (2016) comes from the literature on intergenerational mobility of income and wealth. Building on Güell et al. (2015), Barone and Mocetti (2020) argue that intergenerational mobility of earnings up to the end of the nineteenth century in Florence might have been much lower than what observed today. The authors put forward the idea that in less mobile societies like those prevailing in the pre-industrial era, intergenerational transmission took place thanks to a variety of social institutions and not only through the direct parent-child transmission. Additional arguments postulating the environment as a driver of the long term persistence of trust come from simple models of cultural transmission (see the review in Bisin and Verdier (2011)). In these models, if trust is not vertically transmitted, the child draws it at random from the population. Our results suggest a possible “amendment” to these frameworks: the random draw from the population is sibling-specific rather than being individual-specific, i.e., it affects in the same manner the trust of children who grew up in the same family environment.

7 Summary and conclusion

We study the intergenerational transmission of trust using a sample of parents and children drawn from the German Socio-Economic Panel. Our key asset is the availability of longitudinal information, which is crucial to disentangle the two components of observable trust, namely the permanent trust and the transient shock. This distinction is vital because it is plausible—as well as consistent with the evidence we provide in this paper—that parents transmit to their children only their permanent trust, i.e., their lasting belief. On the other hand, the transient shock—being temporary by construction (attributable to, e.g., random errors in the reported trust)—is unlikely to be passed to the children. Our argument is akin to the point made by Solon et al. (1991) in their analysis of intergenerational transmission of economic status. We show that parents’ permanent trust only accounts for one-third of the observed cross-sectional variability of their children’s permanent trust. To the purpose of the econometric identification of the transmission parameters, the remaining part of the variability rises the classic measurement error problem.

Next, with our panel data, we can test the invariance of trust over time—an important assumption which is implicitly maintained in the previous literature but that has not been proven empirically before. In particular, we show that—within the 10-year window of our longitudinal sample—we do not reject the hypothesis of invariance in our data.

Based on this evidence, we model the relationship between the permanent trust of children and the contemporaneous permanent trust of their parents. The structural interpretation that we give to the parameters of this equation is that they capture the link between the trust that parents input in the transmission process (up to when their children are 17 years old) and the level of permanent trust of their children at the time the transmission is completed. The estimation of these structural parameters requires replacing the unobservable permanent trust of children and of their parents by their error-ridden observable counterpart. The importance of having longitudinal information is once again evident since we can use the lagged trust of parents as a valid instrumental variable to solve the measurement error problem. The remarkable result that transpires is that mothers play a much stronger role than fathers in the transmission process. This result is in line with previous findings (see, for instance, Dohmen et al. (2012)), but the difference we find in the parental roles is stronger.

Finally, exploiting the availability of families with more than one child in our sample, we estimate the variance of the unobservable family-specific environment shared by siblings, such as parental influences not captured by the direct transmission of trust, as well as other local effects shared by siblings, independent of the parents (e.g., schools, friendship networks or other factors operating at the community level) and relevant to their permanent trust.

The variance explained by this component is much larger than the variance explained by the permanent trust of parents. Taken together, the intergenerational correlation and the family-specific effect account for approximately 80% of the variance of the permanent trust of children. In conclusion, while the family environment in which children grew up determines most of their permanent trust, the direct role of intergenerational transmission is on average rather exiguous.

By distinguishing between the permanent and transient components of trust our framework contributes to a better understanding about how the intergenerational transmission process of trust works. We hope that our approach will be useful for future research on the intergenerational transmission of trust, particularly to inform research questions around the roles of nature vs nurture in the determination of trust and possibly to better understand intergenerational transmission mechanisms of other values and norms.

Fig. 1

Age distribution in 2013. Source: SOEP wave 2013. Sample is composed of families with fathers and mothers for whom trust is observed in all three waves and with children for whom trust is observed at least in wave 2013

Table 1 Distribution of families by number of children

Table 2 Average trust in families with and without missing children

Table 3 Autocovariance matrices for trust

Table 4 Testing the invariance of permanent trust

Table 5 Variances of permanent trust and transient shock

Table 6 Intergenerational transmission

Table 7 Decomposition of observed variance of children in 2013