Insights into the Filtered-x LMS Algorithm in the Presence of Frequency Mismatch

Abstract

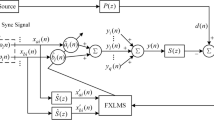
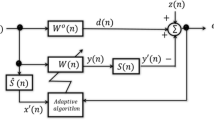
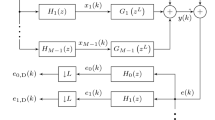
The performance of a narrowband active noise control (NANC) system can be significantly degraded due to the frequency mismatch (FM). In this paper, the statistical performance of a typical FxLMS-based NANC system in the presence of FM is analyzed in detail. Difference equations governing the system dynamics and closed-form steady-state mean-square error expressions are derived and discussed. The stochastic analysis results reveal that the FM introduces small troublesome sinusoids into the NANC system. The controller has to track these sinusoids to minimize the residual noise, which leads to a serious performance deterioration. As a by-product, the optimal step sizes that minimize the effect of a relatively small FM are also derived. The findings significantly enrich our understanding of the stochastic behavior of the FxLMS algorithm in the presence of FM and also provide some useful information for NANC system design. Extensive simulations are conducted to confirm the validity of the analytical findings.

Appendices

Appendix A: Derivation of (18)

Through $\textbf{D}_i$ defined by (13) in [17], one easily has

$$\begin{aligned} \textbf{D}_i^{-1} = \frac{1}{\alpha _i^2+\beta _i^2} \begin{bmatrix} \alpha _i &{} \quad \beta _i\\ -\beta _i &{} \quad \alpha _i \end{bmatrix} \end{aligned}$$

and from the third step of $\Delta \textbf{G}_i(n)$ given by (32) in [17] without applying Taylor series expansions, we have

$$\begin{aligned} \Delta&g_{a_i}(n) = c_{1,i}(\Delta \omega _i)\cos (\Delta \omega _i n) +c_{2,i}(\Delta \omega _i)\sin (\Delta \omega _i n) \end{aligned}$$

(A1)

$$\begin{aligned} \Delta&g_{b_i}(n) = c_{2,i}(\Delta \omega _i)\cos (\Delta \omega _i n) -c_{1,i}(\Delta \omega _i)\sin (\Delta \omega _i n) \end{aligned}$$

(A2)

where

$$\begin{aligned} c_{1,i}(\Delta \omega _i)&= [(\alpha _i a_{p,i}+\beta _i b_{p,i})(1-\cos \Delta \omega _i)\\&\quad -(\alpha _i b_{p,i}-\beta _i a_{p,i})\sin \Delta \omega _i] \left( \alpha _i^2+\beta _i^2 \right) ^{-1}\\ c_{2,i}(\Delta \omega _i)&= [(\alpha _i b_{p,i}-\beta _i a_{p,i})(1-\cos \Delta \omega _i)\\&\quad +(\alpha _i a_{p,i}+\beta _i b_{p,i})\sin \Delta \omega _i] \left( \alpha _i^2+\beta _i^2 \right) ^{-1}. \end{aligned}$$

Then, substituting $\textbf{P}_i(n)$ and $\textbf{Q}_i(n)$, respectively, defined by (29) and (30) in [17], into (15) yields (18).

Appendix B: Some Terms Used in Deriving Difference Eqs. (23) and (24)

$$\begin{aligned} \varepsilon _i&= \alpha _i^2+\beta _i^2,\quad \hat{\varepsilon }_i = \hat{\alpha }_i^2+\hat{\beta }_i^2 \end{aligned}$$

(B3)

$$\begin{aligned} \zeta _{1,i}&= \frac{3}{8} \big (\alpha _i^2\hat{\alpha }_i^2+\beta _i^2\hat{\beta }_i^2 \big ) +\frac{1}{8} \big (\alpha _i^2\hat{\beta }_i^2+\hat{\alpha }_i^2\beta _i^2 \big ) +\frac{1}{2}\alpha _i\beta _i\hat{\alpha }_i\hat{\beta }_i \end{aligned}$$

(B4)

$$\begin{aligned} \zeta _{2,i}&= \frac{1}{8} \big (\alpha _i^2\hat{\alpha }_i^2+\beta _i^2\hat{\beta }_i^2 \big ) +\frac{3}{8} \big (\alpha _i^2\hat{\beta }_i^2+\hat{\alpha }_i^2\beta _i^2 \big ) -\frac{1}{2}\alpha _i\beta _i\hat{\alpha }_i\hat{\beta }_i \end{aligned}$$

(B5)

$$\begin{aligned} \zeta _{3,i}&= \frac{1}{2} \big [\alpha _i\beta _i \big (\hat{\alpha }_i^2-\hat{\beta }_i^2 \big ) -\hat{\alpha }_i\hat{\beta }_i \big (\alpha _i^2-\beta _i^2 \big ) \big ] \end{aligned}$$

(B6)

$$\begin{aligned} T_{1,i}&= \frac{1}{8} \big (\hat{\alpha }_i^2-\hat{\beta }_i^2 \big ) \sum _{k_1=1}^q\sum _{k_2=1,k_2\ne k_1}^q \Bigm \{ \alpha _{k_1}\alpha _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]\nonumber \\&\qquad \times E \big [\delta _{\hat{a}_{k_2}}(n) \big ]-\alpha _{k_1}\beta _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ]\nonumber \\&\qquad -\beta _{k_1}\alpha _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{a}_{k_2}}(n) \big ]\nonumber \\&\qquad +\beta _{k_1}\beta _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ]\Bigm \}\nonumber \\&\quad \times \big [\delta \big (\omega _{k_1}+\omega _{k_2}-2\omega _i \big ) +\delta \big (|\omega _{k_1}-\omega _{k_2}|-2\omega _i \big ) \big ] \end{aligned}$$

(B7)

$$\begin{aligned} T_{2,i}&= \frac{1}{8} \big (\hat{\alpha }_i^2-\hat{\beta }_i^2 \big ) \sum _{k_1=1}^q\sum _{k_2=1,k_2\ne k_1}^q \Bigm \{\beta _{k_1}\beta _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]\nonumber \\&\qquad \times E \big [\delta _{\hat{a}_{k_2}}(n) \big ]+\beta _{k_1}\alpha _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ]\nonumber \\&\qquad +\alpha _{k_1}\beta _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{a}_{k_2}}(n) \big ]\nonumber \\&\qquad +\alpha _{k_1}\alpha _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ] \Bigm \}\nonumber \\&\quad \times \big [\delta \big (\omega _{k_1}+\omega _{k_2}-2\omega _i \big )-\delta \big (|\omega _{k_1}-\omega _{k_2}|-2\omega _i \big ) \big ] \end{aligned}$$

(B8)

$$\begin{aligned} T_{3,i}&= \frac{1}{4}\hat{\alpha }_i\hat{\beta }_i \sum _{k_1=1}^q\sum _{k_2=1,k_2\ne k_1}^q \Bigm \{\alpha _{k_1}\beta _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]\nonumber \\&\qquad \times E \big [\delta _{\hat{a}_{k_2}}(n) \big ]+\alpha _{k_1}\alpha _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ] \nonumber \\&\qquad -\beta _{k_1}\beta _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{a}_{k_2}}(n) \big ]\nonumber \\&\qquad -\beta _{k_1}\alpha _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ]\Bigm \}\nonumber \\&\quad \times \big [\delta \big (\omega _{k_1}+\omega _{k_2}-2\omega _i \big )-\textrm{sgn} \big (\omega _{k_1}-\omega _{k_2} \big )\nonumber \\&\qquad \times \delta \big (|\omega _{k_1}-\omega _{k_2}|-2\omega _i \big ) \big ] \end{aligned}$$

(B9)

$$\begin{aligned} T_{4,i}&= \frac{1}{4}\hat{\alpha }_i\hat{\beta }_i \sum _{k_1=1}^q\sum _{k_2=1,k_2\ne k_1}^q \Bigm \{\beta _{k_1}\alpha _{k_2}E \big [\delta _{\hat{a}_{k_1}}(n) \big ]\nonumber \\&\qquad \times E \big [\delta _{\hat{a}_{k_2}}(n) \big ]-\beta _{k_1}\beta _{k_2} E \big [\delta _{\hat{a}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ] \nonumber \\&\qquad +\alpha _{k_1}\alpha _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{a}_{k_2}}(n) \big ]\nonumber \\&\qquad -\alpha _{k_1}\beta _{k_2}E \big [\delta _{\hat{b}_{k_1}}(n) \big ]E \big [\delta _{\hat{b}_{k_2}}(n) \big ]\Bigm \}\nonumber \\&\quad \times \big [\delta \big (\omega _{k_1}+\omega _{k_2}-2\omega _i \big )+\textrm{sgn} \big (\omega _{k_1}-\omega _{k_2} \big )\nonumber \\&\qquad \times \delta \big (\big |\omega _{k_1}-\omega _{k_2}\big |-2\omega _i \big ) \big ]. \end{aligned}$$

(B10)

Here, $\textrm{sgn}(\cdot )$ and $\delta (\cdot )$ are signum and Dirac delta functions, respectively.

Appendix C: Derivations of the Steady-State Time-Averaged MSEs (30) and (31)

Signals $\Delta g_{a_i}(n)$, $\Delta g_{b_i}(n)$ given in (A1) and (A2) can be recursively calculated by

$$\begin{aligned} \Delta&g_{a_i}(n+1) = \cos \Delta \omega _i\cdot \Delta g_{a_i}(n)+\sin \Delta \omega _i\cdot \Delta g_{b_i}(n) \end{aligned}$$

(C11)

$$\begin{aligned} \Delta&g_{b_i}(n+1) = \cos \Delta \omega _i\cdot \Delta g_{b_i}(n)-\sin \Delta \omega _i\cdot \Delta g_{a_i}(n). \end{aligned}$$

(C12)

Cross multiplying (21) and (22) by (C11) and (C12), one can obtain the following recursive equations:

$$\begin{aligned} \Delta g_{a_i}(n+1)E \left[ \delta _{\hat{a}_i}(n+1) \right]&= \phi _i \cos \Delta \omega _i\cdot \Delta g_{a_i}(n)E \left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad -\psi _i \cos \Delta \omega _i\cdot \Delta g_{a_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad +\phi _i \sin \Delta \omega _i\cdot \Delta g_{b_i}(n)E\left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad -\psi _i \sin \Delta \omega _i\cdot \Delta g_{b_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad +\cos \Delta \omega _i\cdot \Delta g_{a_i}^2(n)\nonumber \\&\quad +\sin \Delta \omega _i\cdot \Delta g_{a_i}(n)\Delta g_{b_i}(n) \end{aligned}$$

(C13)

$$\begin{aligned} \Delta g_{a_i}(n+1)E[\delta _{\hat{b}_i}(n+1)]&= \phi _i \cos \Delta \omega _i\cdot \Delta g_{a_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad +\psi _i \cos \Delta \omega _i\cdot \Delta g_{a_i}(n)E\left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad +\phi _i \sin \Delta \omega _i\cdot \Delta g_{b_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad +\psi _i \sin \Delta \omega _i\cdot \Delta g_{b_i}(n)E\left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad +\cos \Delta \omega _i\cdot \Delta g_{a_i}(n)\Delta g_{b_i}(n)\nonumber \\&\quad +\sin \Delta \omega _i\cdot \Delta g_{b_i}^2(n) \end{aligned}$$

(C14)

$$\begin{aligned} \Delta g_{b_i}(n+1)E[\delta _{\hat{a}_i}(n+1)]&= \phi _i \cos \Delta \omega _i\cdot \Delta g_{b_i}(n)E\left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad -\psi _i \cos \Delta \omega _i\cdot \Delta g_{b_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad -\phi _i \sin \Delta \omega _i\cdot \Delta g_{a_i}(n)E\left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad +\psi _i \sin \Delta \omega _i\cdot \Delta g_{a_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad +\cos \Delta \omega _i\cdot \Delta g_{a_i}(n)\Delta g_{b_i}(n)\nonumber \\&\quad -\sin \Delta \omega _i\cdot \Delta g_{a_i}^2(n) \end{aligned}$$

(C15)

$$\begin{aligned} \Delta g_{b_i}(n+1)E[\delta _{\hat{b}_i}(n+1)]&= \phi _i \cos \Delta \omega _i\cdot \Delta g_{b_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad +\psi _i \cos \Delta \omega _i\cdot \Delta g_{b_i}(n)E \left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad -\phi _i \sin \Delta \omega _i\cdot \Delta g_{a_i}(n)E \left[ \delta _{\hat{b}_i}(n) \right] \nonumber \\&\quad -\psi _i \sin \Delta \omega _i\cdot \Delta g_{a_i}(n)E\left[ \delta _{\hat{a}_i}(n) \right] \nonumber \\&\quad +\cos \Delta \omega _i\cdot \Delta g_{b_i}^2(n)\nonumber \\&\quad -\sin \Delta \omega _i\cdot \Delta g_{a_i}(n)\Delta g_{b_i}(n) \end{aligned}$$

(C16)

where

$$\begin{aligned} \phi _i = 1-\frac{1}{2}\mu _i\kappa _i,\quad \psi _i = \frac{1}{2}\mu _i\tau _i. \end{aligned}$$

From (A1), (A2), and (C13)–(C16), considering the time averaging manipulations in the steady state, one readily yields

$$\begin{aligned} E_T \left[ \Delta g_{a_i}^2(n) \right] |_{n\rightarrow \infty }&= E_T[\Delta g_{b_i}^2(n)]|_{n\rightarrow \infty }\nonumber \\&= \frac{1}{2}[c_{1,i}^2(\Delta \omega _i)+c_{2,i}^2(\Delta \omega _i)]\nonumber \\&= \frac{A_{p,i}^2}{\varepsilon _i}(1-\cos \Delta \omega _i) \end{aligned}$$

(C17)

$$\begin{aligned} E_T[\Delta g_{a_i}(n)\Delta g_{b_i}(n)]|_{n\rightarrow \infty } = 0 \end{aligned}$$

(C18)

$$\begin{aligned} E_T[\Delta g_{a_i}(n+1)E[\delta _{\hat{a}_i}(n+1)]]|_{n\rightarrow \infty }&= E_T[\Delta g_{a_i}(n)E[\delta _{\hat{a}_i}(n)]]|_{n\rightarrow \infty } \nonumber \\&:= F_i^{(aa)}(\mu _i,\Delta \omega _i) \end{aligned}$$

(C19)

$$\begin{aligned} E_T[\Delta g_{a_i}(n+1)E[\delta _{\hat{b}_i}(n+1)]]|_{n\rightarrow \infty }&= E_T[\Delta g_{a_i}(n)E[\delta _{\hat{b}_i}(n)]]|_{n\rightarrow \infty } \nonumber \\&:= F_i^{(ab)}(\mu _i,\Delta \omega _i) \end{aligned}$$

(C20)

$$\begin{aligned} E_T[\Delta g_{b_i}(n+1)E[\delta _{\hat{a}_i}(n+1)]]|_{n\rightarrow \infty }&= E_T[\Delta g_{b_i}(n)E[\delta _{\hat{a}_i}(n)]]|_{n\rightarrow \infty } \nonumber \\&:= F_i^{(ba)}(\mu _i,\Delta \omega _i) \end{aligned}$$

(C21)

$$\begin{aligned} E_T[\Delta g_{b_i}(n+1)E[\delta _{\hat{b}_i}(n+1)]]|_{n\rightarrow \infty }&= E_T[\Delta g_{b_i}(n)E[\delta _{\hat{b}_i}(n)]]|_{n\rightarrow \infty } \nonumber \\&:= F_i^{(bb)}(\mu _i,\Delta \omega _i) \end{aligned}$$

(C22)

where

$$\begin{aligned} F_i^{(aa)}(\mu _i,\Delta \omega _i)&= \phi _i\cos \Delta \omega _i\cdot F_i^{(aa)}(\mu _i,\Delta \omega _i)\\&\quad -\psi _i\cos \Delta \omega _i\cdot F_i^{(ab)}(\mu _i,\Delta \omega _i)\\&\quad +\phi _i\sin \Delta \omega _i\cdot F_i^{(ba)}(\mu _i,\Delta \omega _i)\\&\quad -\psi _i\sin \Delta \omega _i\cdot F_i^{(bb)}(\mu _i,\Delta \omega _i)\\&\quad +\frac{A_{p,i}^2}{\varepsilon _i}(1-\cos \Delta \omega _i)\cos \Delta \omega _i\\ F_i^{(ab)}(\mu _i,\Delta \omega _i)&= \phi _i\cos \Delta \omega _i\cdot F_i^{(ab)}(\mu _i,\Delta \omega _i)\\&\quad +\psi _i\cos \Delta \omega _i\cdot F_i^{(aa)}(\mu _i,\Delta \omega _i)\\&\quad +\phi _i\sin \Delta \omega _i\cdot F_i^{(bb)}(\mu _i,\Delta \omega _i)\\&\quad +\psi _i\sin \Delta \omega _i\cdot F_i^{(ba)}(\mu _i,\Delta \omega _i)\\&\quad +\frac{A_{p,i}^2}{\varepsilon _i}(1-\cos \Delta \omega _i)\sin \Delta \omega _i\\ F_i^{(ba)}(\mu _i,\Delta \omega _i)&= \phi _i\cos \Delta \omega _i\cdot F_i^{(ba)}(\mu _i,\Delta \omega _i)\\&\quad -\psi _i\cos \Delta \omega _i\cdot F_i^{(bb)}(\mu _i,\Delta \omega _i)\\&\quad -\phi _i\sin \Delta \omega _i\cdot F_i^{(aa)}(\mu _i,\Delta \omega _i)\\&\quad +\psi _i\sin \Delta \omega _i\cdot F_i^{(ab)}(\mu _i,\Delta \omega _i)\\&\quad -\frac{A_{p,i}^2}{\varepsilon _i}(1-\cos \Delta \omega _i)\sin \Delta \omega _i\\ F_i^{(bb)}(\mu _i,\Delta \omega _i)&= \phi _i\cos \Delta \omega _i\cdot F_i^{(bb)}(\mu _i,\Delta \omega _i)\\&\quad +\psi _i\cos \Delta \omega _i\cdot F_i^{(ba)}(\mu _i,\Delta \omega _i)\\&\quad -\phi _i\sin \Delta \omega _i\cdot F_i^{(ab)}(\mu _i,\Delta \omega _i)\\&\quad -\psi _i\sin \Delta \omega _i\cdot F_i^{(aa)}(\mu _i,\Delta \omega _i)\\&\quad +\frac{A_{p,i}^2}{\varepsilon _i}(1-\cos \Delta \omega _i)\cos \Delta \omega _i. \end{aligned}$$

Solving simultaneous equations composed of the above four equations, one easily reaches

$$\begin{aligned} F_i^{(aa)}(\mu _i,\Delta \omega _i)&= F_i^{(bb)}(\mu _i,\Delta \omega _i)\nonumber \\&= \frac{A_{p,i}^2}{\varepsilon _i}\frac{(\cos \Delta \omega _i-\phi _i)(1-\cos \Delta \omega _i)}{1+\phi _i^2+\psi _i^2-2\phi _i\cos \Delta \omega _i+2\psi _i\sin \Delta \omega _i}\nonumber \\&:= F_{i,1}(\mu _i,\Delta \omega _i) \end{aligned}$$

(C23)

$$\begin{aligned} F_i^{(ab)}(\mu _i,\Delta \omega _i)&= \frac{A_{p,i}^2}{\varepsilon _i}\frac{(\sin \Delta \omega _i+\psi _i)(1-\cos \Delta \omega _i)}{1+\phi _i^2+\psi _i^2-2\phi _i\cos \Delta \omega _i+2\psi _i\sin \Delta \omega _i}\nonumber \\&:= F_{i,2}(\mu _i,\Delta \omega _i) \end{aligned}$$

(C24)

$$\begin{aligned} F_i^{(ba)}(\mu _i,\Delta \omega _i)&= -F_i^{(ab)}(\mu _i,\Delta \omega _i) = -F_{i,2}(\mu _i,\Delta \omega _i). \end{aligned}$$

(C25)

Now, using the relations obtained above after taking the time averaging of (23) and (24), we have (30) and (31).