Abstract
Critical velocities of a two-layer composite tube subjected to a uniform internal pressure moving at a constant velocity are analytically derived by using a first-order shear deformation shell theory incorporating the transverse shear, rotary inertia and material anisotropy. The composite tube consists of two perfectly bonded axisymmetric circular cylindrical layers of dissimilar materials, which can be orthotropic, transversely isotropic, cubic or isotropic. Closed-form expressions for four critical velocities are first derived for the general case by including the effects of transverse shear, rotary inertia, material orthotropy and radial stress. The formulas for composite tubes without the transverse shear, rotary inertia or radial stress effect and with simpler anisotropy are then obtained as special cases. In addition, it is shown that the model for a single-layer, homogeneous tube is included in the current model as a special case. To illustrate the newly derived closed-form formulas, a composite tube with an isotropic inner layer and an orthotropic outer layer is analyzed as an example. The numerical values of the lowest critical velocity of the two-layer composite tube predicted by the new formulas compare well with existing data.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
Dynamic strains in a single-layer tube subjected to a moving internal pressure can exceed three times of their counterpart static values when the pressure velocity approaches the lowest critical velocity of the tube (e.g., [1,2,3,4]). Hence, knowing critical velocities of such tubes is essential. Critical velocities are also important for safe designs of other structures under moving loads (e.g., [5,6,7,8,9]).
For single-layer, homogeneous tubes, continuing efforts have been made in the last 60 years to find analytical formulas for computing their critical velocities (e.g., [2, 4, 10,11,12,13,14]). However, for composite tubes with two or more layers of dissimilar materials (e.g., [15,16,17]), very few studies have been conducted to analytically determine their critical velocities. Jones and Whittier [18] analyzed axisymmetric wave propagation in a two-layered cylindrical shell based on a Timoshenko-type shell theory, but they did not discuss critical velocities of the composite cylinder. Chonan [19] investigated the dynamic response of a two-layered cylindrical shell to a moving ring load and computed the lowest critical velocity of the composite shell with imperfect or perfect interfacial bonding using the shell theory of Herrmann and Mirsky [20]. Simkins [15] determined the critical velocity of an isotropic tube wrapped with an orthotropic layer by employing the model for laminated orthotropic cylindrical shells presented in Dong [21]. However, no closed-form formula has been reported in these and other existing shell theory-based studies for critical velocities of composite tubes with two or more layers of dissimilar materials.
In the current work, closed-form formulas for four critical velocities of a composite tube consisting of two layers of dissimilar materials under a uniform internal pressure moving at a constant velocity are derived by using a first-order shear deformation shell theory (e.g., [22, 23]) that incorporates the transverse shear, rotary inertia and material anisotropy. Being based on the general 3-D constitutive relations for orthotropic elastic materials, the current formulation provides a unified treatment of composite tubes containing two layers of dissimilar materials, each of which can have a different type of material symmetry, including orthotropic, transversely isotropic, cubic or isotropic. When the transverse shear effect is suppressed, the current model reduces to that for axisymmetric circular cylindrical Love–Kirchhoff thin shells with the rotary inertia effect [24]. In addition, the newly derived formulas for two-layer composite tubes recover those for single-layer, homogeneous tubes [4, 14] as special cases.
The rest of this paper is organized as follows. In Sect. 2, the first-order shear deformation (FSD) shell model for axisymmetric orthotropic cylindrical shells with the transverse shear and rotary inertia effects formulated in Gao [14] is briefly reviewed. In Sect. 3, closed-form formulas for four critical velocities of a composite tube consisting of two layers of dissimilar materials under a moving internal pressure are derived by using the FSD shell model reviewed in Sect. 2. In Sect. 4, the general formulas obtained in Sect. 3 are reduced to simplified forms for special cases without the transverse shear effect or with simpler anisotropy. A numerical example is provided in Sect. 5 for a composite tube consisting of an isotropic inner layer and an orthotropic outer layer by directly applying the new formulas derived in Sects. 3 and 4. The critical velocity values predicted by three sets of the newly derived analytical formulas are compared to each other and with that reported in Simkins [15]. The paper concludes in Sect. 6 with a summary.
2 FSD shell model incorporating the transverse shear and rotary inertia effects: review
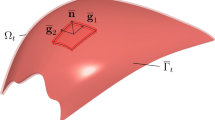
According to the first-order shear deformation (FSD) shell theory, the displacement field in an axisymmetric circular cylindrical shell of uniform thickness h and mean radius R in the coordinate system \(\{x, \theta , z\}\) shown in Fig. 1 can be written as (e.g., [23, 25])
where \(u_{x}\), \(u_{\theta }\) and \(u_{z} \) are, respectively, the x-, \(\theta \)- and z-components of the displacement vector u of a point (x, \(\theta \), z) in the shell at time t, u and w are, respectively, the x- and z-displacement components of the corresponding point (\(x, \theta , \) 0) on the shell middle surface at time t, and \(\psi _{x}\) is the rotation angle of a transverse normal about the \(\theta \)-direction.
From Eq. (1), the components of the infinitesimal strain tensor in the axisymmetric circular cylindrical shell can be obtained as (e.g., [14]), with \(r \approx R\),
The constitutive equations for an orthotropic linear elastic material in the cylindrical coordinate system {x, \(\theta \), z} have the general expressions (e.g., [26,27,28,29]):
where \({\sigma }_{\textit{ij}}\) (\(i,j\in \){x, \(\uptheta \), z}) are the components of the Cauchy stress tensor (with \({\sigma }_{ij} \,{=}\, {\sigma } _{ji})\) and \( C_{ij}(i, j\in \){1, 2, 3, 4, 5, 6}) are the components of the elastic stiffness matrix (with C\(_{ij} \,{=}\) C\(_{ji})\), which contains nine independent components for the orthotropic material, as indicated in Eq. (3).
Using Eq. (2) in Eq. (3) gives the stress components in terms of the kinematic variables u(x, t), w(x, t) and \(\psi _{x}(x, t)\) as
The equations of motion in terms of u, w and \(\psi _{x}\) for the axisymmetric circular cylindrical FSD shell satisfying Eqs. (1), (2) and (4) are given by [14]
where \(f_{x}\) and \(f_{z}\) are, respectively, the x- and z-components of the body force resultant (force per unit area) through the shell thickness acting on the mid-surface, and \(k_{s} \) is a shear correction factor accounting for the non-uniform distribution of \({\varvec{\varepsilon }}_{xz}\) over the shell thickness.
Note that the two second-order equations in Eqs. (5b) and (5c) can be combined to obtain the following fourth-order equation [14]:
Solving Eqs. (5a) and (6) will give u(x, t) and w(x, t). The rotation angle \(\psi _{x}(x\), t) can then be readily determined from Eq. (5b). Equation (6) shows that in this model for axisymmetric cylindrical shells the rotary inertia effect is incorporated through the term “\(\textstyle {{\rho h^{3}} \over {12}}\textstyle {{\partial ^{4}w} \over {\partial x^{2}\partial t^{2}}}\)” (e.g., [30, 31]), while the transverse shear effect is included via the terms containing \(k_{s}C_{55}\) (e.g., [32,33,34]).
3 Critical velocities of a two-layer composite tube under a moving pressure
The critical velocities of a two-layer composite tube subjected to an internal pressure moving at a constant velocity are derived herein using the axisymmetric circular cylindrical FSD shell model reviewed in Sect. 2, which incorporates the transverse shear and rotary inertia effects and accounts for the anisotropy of the shell material.
Consider an axisymmetric two-layer composite tube consisting of an inner cylindrical layer of mean radius \(R_{\textrm{1}}\) and thickness \(h_{\textrm{1}}\) and an outer cylindrical layer of mean radius \(R_{\textrm{2}}\) and thickness \(h_{\textrm{2}}\). The two layers of dissimilar materials are perfectly bonded at the interface \(r = R_{\textrm{1}} +\) \(h_{\textrm{1}}\)/2 \(= R_{\textrm{2}} - h_{\textrm{2}}\)/2, and the composite tube is under a uniform internal pressure \(p_{\textrm{0}}\) moving at a constant velocity V, as shown in Fig. 2. The moving pressure can be represented by
where \(p_{\textrm{0}}\) is the magnitude of the pressure and \(H(\cdot )\) is the Heaviside step function.
Each layer is taken to be an orthotropic elastic material that satisfies the governing equations in Eqs. (5a) and (6) for an axisymmetric circular cylindrical FSD shell. Then, applying Eqs. (5a) and (6) to the inner layer yields, with \(f_{x}^{(1)} =\) 0,
where the superscript “(1)” denotes the inner layer (i.e., material 1) and use has been made of Eq. (7).
Similarly, using Eqs. (5a) and (6) for the outer cylindrical layer gives, with \(f_{x}^{(2)} =\) 0,
where the superscript “(2)” stands for the outer layer (i.e., material 2)
The continuity conditions at the perfectly bonded interface \(r =R_{\textrm{1}}+ h_{\textrm{1}}/2=R_{\textrm{2}}-h_{\textrm{2}}/2\) of the axisymmetric composite tube read
Substituting Eq. (1) into Eqs. (10a) and (10b) leads to
where use has been made of the approximations \(\psi _{x}^{(1)} (x,t)h_{1} /2\ll u^{(1)}(x,t)\) and \(\psi _{x}^{(2)} (x,t)h_{2} /2\ll u^{(2)}(x,t)\) in reaching Eq. (11a). Note that Eqs. (11a) and (11b) are the same as those obtained in [18] by setting \(z=0\) at the interface.
From Eqs. (8a), (8b), (9a), (9b), (10c), (11a) and (11b), it follows that
These are the equations of motion for the composite tube to be solved to obtain u(x, t) and w(x, t). From Eq. (12b), it is clear that for the current composite tube the rotary inertia effect is included through the term “\(\left[ {\frac{\rho ^{(1)}h_{1}^{3} }{12}+\frac{\rho ^{(2)}h_{2}^{3} }{12}} \right] \frac{\partial ^{4}w}{\partial x^{2}\partial t^{2}}\)”, while the transverse shear effect is incorporated via the terms containing \(k_{s}C_{\textrm{55}}\) If the transverse shear effect is suppressed by dropping all of the \(k_{s}C_{\textrm{55}}\) terms, then Eqs. (12a) and (12b) will reduce to the governing equations for a two-layer composite tube derived in [24] using the Love–Kirchhoff thin shell theory (e.g., [35, 36]).
When the tube is homogeneous with \(C_{ij}^{(1)} =C_{ij}^{(2)} =C_{ij},\rho ^{(1)}=\rho ^{(2)}=\rho , R_{1} \approx R\approx R_{2},h_{1} =h\) and \(h_{2} =0\), Eqs. (12a) and (12b) reduce to
which are identical to the governing equations for a single-layer axisymmetric orthotropic tube under a uniform internal pressure \(p_{0}\) moving at a constant velocity V derived in [14] by applying the FSD shell theory.
To obtain the steady-state solution of Eqs. (12a) and (12b), consider the following transformation (e.g., [2, 11]):
where \(\xi \) is a new variable. In terms of \(\xi \), Eqs. (12a) and (12b) become
where all derivatives are with respect to the new variable \(\xi \).
When \(C_{11}^{(1)} +C_{11}^{(2)} -\left[ {\rho ^{(1)}+\rho ^{(2)}} \right] V^{2}=0\), Eq. (15a) shows that w is a constant for any value of u. This defines one critical velocity of the composite tube given by
which is the dilatational wave velocity of the two-layer shell.
When \(C_{11}^{(1)} +C_{11}^{(2)} -\left[ {\rho ^{(1)}+\rho ^{(2)}} \right] V^{2}\ne 0\), Eq. (15a) can be integrated once with respect to \(\xi \) to obtain
where \(\bar{{c}}\) is an integration constant. Substituting Eq. (16b) into Eq. (15b) then results in
where c (\(\equiv -\left[ {\frac{C_{12}^{(1)} h_{1} }{R_{1} }+\frac{C_{12}^{(2)} h_{2} }{R_{2} }} \right] \bar{{c}})\) is a constant. The solution of Eq. (17) can be written as
where \(w_{p}\) is a particular solution and \(w_{h}\) is the general solution of the homogeneous part of Eq. (17) given by
The solution of Eq. (19) has the form:
where W and \(\alpha \) are constants. Using Eq. (20) in Eq. (19) yields the characteristic equation as
where
The roots of Eq. (21), a quadratic equation in \(\alpha ^{\textrm{2}}\), can be readily obtained as, with \(A \ne \) 0,
Equations (22a)–(22c) and (23) show that the four values of \(\alpha \) can be real, complex or purely imaginary, depending on the value of V for given material constants (i.e., \(\rho ^{(1)}\), \(\rho ^{(2)}\), \(C_{11}^{(1)}\), \(C_{22}^{(1)}\), \(C_{12}^{(1)}\), \(C_{55}^{(1)}\), \(C_{11}^{(2)}\), \(C_{22}^{(2)}\), \(C_{12}^{(2)}\) and \(C_{55}^{(2)}\)) and geometrical parameters (i.e., \(R_{\textrm{1}}\), \(h_{\textrm{1}}\), \(R_{\textrm{2}}\), \(h_{\textrm{2}},k_{s}^{(1)}\) and \(k_{s}^{(2)}\)).
When \(A =\) 0, Eq. (22a) gives
which, as a quadratic equation in \(V^{\textrm{2}}\), can be solved to obtain a critical velocity as
which is the smallest positive real root of Eq. (24).
When \(C =\) 0, Eq. (22c) leads to
which is another critical velocity. This is the same as that obtained in [24] without including the transverse shear effect, which shows that \(V_{{cr}_{\textrm{2}}}\) is not affected by the transverse shear
Finally, when the discriminant of Eq. (21) vanishes, one more critical velocity can be obtained. That is, from Eqs. (22a)–(22c) and (23), it follows that
which can be rewritten as
where
Equation (28) is a quartic equation in \(V^{\textrm{2}}\) of the standard form, which can be solved to obtain its four roots as (e.g., [37, 38])
where \(\lambda \) is a solution of the following cubic equation:
and
with the parameters a, b, c, d and e defined in Eqs. (29a)–(29e).
Note that Eq. (31) can be changed to the depressed cubic equation:
where
The discriminant of Eq. (33) is
When \(\Delta _{d }>0\), Eq. (33) has three distinct real roots that can be determined from Viète’s trigonometric solution (e.g., [39, 40]), which lead to the three distinct real roots of Eq. (31) as, with the help of Eq. (34a),
Any of these three values of \(\lambda \) can be used in Eq. (30) to obtain \(V_{j}^{2}\, (j = 1, 2, 3, 4)\). The smallest positive real value among \(V_{\textrm{1}}\), \(V_{\textrm{2}}\), \(V_{\textrm{3}}\) and \(V_{\textrm{4}}\) computed from Eq. (30) gives the critical velocity \(V_{{cr}_{\textrm{0}}}\) in this case.
When \(\Delta _{d}<0\), Eq. (33) has one real root and two conjugated complex roots that can be obtained using the Cardano formula (e.g., [41]), which give the three roots of Eq. (31) as, upon using Eq. (34a),
where \(\lambda _{1} \) in Eq. (34a) is the real root, which will be used in Eq. (30) to determine \(V_{j}^{2}\, (j = 1, 2,3, 4)\) and thus the critical velocity \(V_{{cr}_{0}}\) in this case as the smallest positive real value among \(V_{\textrm{1}}\), \(V_{\textrm{2}}\), \(V_{\textrm{3}}\) and \(V_{\textrm{4}}.\)
When \(\Delta _{d }= 0\), Eq. (33) has a triple root of 0 if \(\varGamma = 0\) or a single root of \(\omega _{1} =\frac{3\varOmega }{\varGamma }\) and a double root of \(\omega _{2} =\omega _{3} =-\frac{3\varOmega }{2\varGamma }\) if \(\varGamma \ne 0\) (e.g., [40]). Then, it follows from these results and Eq. (34a) that the roots of Eq. (31) in this case are given by
Using any of the three values of \(\lambda \) listed in Eq. (34a) or (34b) in Eq. (30) will yield \(V_{\textrm{1}}\), \(V_{\textrm{2}}\), \(V_{\textrm{3}}\) and \(V_{\textrm{4}}\) in each case, the smallest positive value of which will be \(V_{cr}\)0.
4 Special cases
4.1 Composite tube without the transverse shear effect
When the transverse shear effect is suppressed, the characteristic equation in Eq. (21) reduces to
which is the same as that first derived in [24] without considering the transverse shear effect.
By setting the coefficient of the fourth-polynomial term in Eq. (39) to zero, the critical velocity \(V_{{cr}_{\textrm{1}}}\) can be readily obtained as
which was initially obtained in [24] based on the Love–Kirchhoff thin shell theory.
Similarly, the critical velocity \(V_{{cr}_{\textrm{2}}}\)can be determined by letting the zeroth-polynomial term in Eq. (39) vanish, which yields the formula identical to that listed in Eq. (26) for \(V_{{cr}_{\textrm{2}}}\)
The critical velocity \(V_{{cr}_{\textrm{3}}}\) remains the same as that obtained in Eq. (16a) as the dilatational wave velocity \(V_{d}\) of the two-layer shell.
The critical velocity \(V_{{cr}_{0}}\) is given by the condition of vanishing discriminant of Eq. (39), which yields
Solving this cubic equation in \(V^{\textrm{2}}\) will lead to the determination of \(V_{{cr}_{\textrm{0}}}\), which is discussed in detail in [24]
Note that Eq. (39) can be rewritten as, with the help of Eqs. (16a) and (40),
When \(V/V_{{cr}_{\textrm{1}}}\ll 1\) and \(V/V_{{cr}_{\textrm{3}}} \ll 1\), Eq. (42) reduces to
This is a quadratic equation in \(\alpha ^{\textrm{2}}\). The critical velocity \(V_{{cr}_{0}}\) can be found from the condition that the discriminant of Eq. (43) vanishes, which results in
This critical velocity formula was first derived in [24] for two-layer composite tubes without the transverse shear effect.
4.2 Composite tube without the transverse shear and rotary inertia effects
When both the transverse shear and rotary inertia effects are not considered, the characteristic equation in Eq. (39) further simplifies to
which was first obtained in [24] as a special case of the two-layer composite tube model that does not include the transverse shear effect.
From Eq. (45), \(V_{{cr}_{\textrm{2}}}\) in this case can be readily obtained by letting the coefficient of the zeroth polynomial term vanish, which gives the same formula as that listed in Eq. (26). The critical velocity \(V_{{cr}_{\textrm{3}}}\) remains the same as that derived in in Eq. (16a). However, the critical velocity \(V_{{cr}_{\textrm{1}}}\)is irrelevant in this case.
The critical velocity \(V_{{cr}_{\textrm{0}}}\) is reached when the discriminant of Eq. (45) vanishes, which gives
This cubic equation in \(V^{\textrm{2}}\) can be solved to determine \(V_{{cr}_{0}}\) by following a procedure similar to that used in solving Eq. (41), as reported in [24]
4.3 Composite tube without the radial stress effect
For thin orthotropic cylindrical shells with \(\sigma _{zz} \approx 0\) and under axisymmetric loading, the elastic stiffness constants are given by [4, 27, 42]
where \(E_{xx}\) and \(E_{\theta \theta }\) are, respectively, Young’s moduli in the x- and \(\theta \)-directions, \(\nu _{x\theta }\) and \(\nu _{x\theta }\) are Poisson’s ratios, and \(\mu _{xz}\) is the shear modulus in the xz-plane.
Applying Eq. (47) to each orthotropic layer and subsequently using Eq. (30) along with Eq. (36), (37a), (38a) or (38b) will lead to the determination of the critical velocity \(V_{{cr}_{\textrm{0}}}\) of a composite tube consisting of two dissimilar orthotropic thin layers without the radial stress effect
Substituting Eq. (47) into Eqs. (25), (26) and (16a), respectively, gives the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) as
for a composite tube made from two dissimilar orthotropic thin layers, which incorporate the transverse shear and rotary inertia effects (but exclude the radial stress effect) and have no restriction on the magnitude of the pressure velocity.
When V \(<<\) \(V_{{cr}_{\textrm{1}}}\) and V \(<<\) \(V_{{cr}_{\textrm{3}}}\), the substitution of Eq. (47) into Eq. (44) yields
as the critical velocity for a composite tube consisting of two dissimilar orthotropic thin layers under an internal pressure moving at a constant velocity V \(<<\) min(\(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{3}}})\), with the transverse shear, rotary inertia and radical stress effects all precluded. If the tube is homogeneous with \(E_{xx}^{(1)} =E_{xx}^{(2)} =E_{xx}, E_{\theta \theta }^{(1)} =E_{\theta \theta }^{(2)} =E_{\theta \theta }, \,\nu _{x\theta }^{(1)} =\nu _{x\theta }^{(2)} =\nu _{x\theta }, \,\nu _{\theta x}^{(1)} =\nu _{\theta x}^{(2)} =\nu _{\theta x}, \rho ^{(1)}=\rho ^{(2)}=\rho \), \(R_{1} \approx R\approx R_{2}, h_{1} =h\) and \(h_{2} =0\), then Eq. (49) reduces to
which is the same as that reported in [13] and derived in [4] for single-layer orthotropic thin tubes without including the transverse shear, rotary inertia and radial stress effects.
4.4 Composite tube with two layers exhibiting simpler anisotropy
The general case of a composite tube made from two dissimilar orthotropic layers with different \(C_{ij}\) and \(\rho \) has been discussed in detail in Sects. 3 and 4.1–4.3. The critical velocity formulas for tubes consisting of two dissimilar transversely isotropic, cubic or isotropic layers are obtained here as special cases of those for composite tubes with two dissimilar orthotropic layers.
4.4.1 Composite tube with two transversely isotropic layers
For transversely isotropic materials with five independent elastic constants, the stiffness components for orthotropic materials introduced in Eq. (3) reduce to (e.g., [43])
where \(E_{T}\) and \(E_{L}\) are, respectively, the Young’s moduli in the transverse (isotropic) plane and longitudinal direction, \(\nu _{T}\), \(\nu _{TL}\) and \(\nu _{LT}\) are Poisson’s ratios, and \(\mu _{LT}\) is the shear modulus in the longitudinal direction. Note that the relation \(\nu _{LT} /E_{L} =\nu _{TL} /E_{T} \) holds.
Applying Eq. (51) to each transversely isotropic layer and then using Eq. (30) along with Eq. (36), (37a),(38a) or (38b) will lead to the determination of the critical velocity \(V_{{cr}_{0}}\), and substituting Eq. (51) into Eqs. (25), (26) and (16a), respectively, yields the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) as
for a composite tube consisting of two dissimilar transversely isotropic layers, which account for the transverse shear, rotary inertia and radial stress effects and have no restriction on the magnitude of the pressure velocity.
Note that in Eqs. (52a)–(52c),
For thin transversely isotropic cylindrical shells with \(\sigma _{zz}\) \(\approx 0\) and under axisymmetric loading, the elastic stiffness constants are given by [42, 44]
Using Eq. (54) for each transversely isotropic layer and then applying Eq. (30) along with Eq. (36), (37a),(38a) or (38b) will give the critical velocity \(V_{{cr}_{0}}\), and substituting Eq. (54) into Eqs. (25), (26) and (16a), respectively, will yield the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) as
for a composite tube consisting of two dissimilar transversely isotropic thin layers, which account for the transverse shear and rotary inertia effects (but exclude the radial stress effect) and have no restriction on the magnitude of the pressure velocity.
When \(V\ll V_{{cr}_{\textrm{1}}}\) and \(V\ll V_{{cr}_{\textrm{3}}}\), substituting Eq. (54) into Eq. (44) gives
as the critical velocity \(V_{{cr}_{\textrm{0}}}\) for a composite tube consisting of two dissimilar transversely isotropic thin layers under an internal pressure moving at a constant velocity V \(<<\) min(\(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{3}}})\), which has precluded the transverse shear, rotary inertia and radial stress effects. If the tube is homogeneous with \(E_{L}^{(1)} =E_{L}^{(2)} =E_{L}, E_{T}^{(1)} =E_{T}^{(2)} =E_{T}, \nu _{LT}^{(1)} =\nu _{LT}^{(2)} =\nu _{LT}, \nu _{TL}^{(1)} =\nu _{TL}^{(2)} =\nu _{TL},\rho ^{(1)}=\rho ^{(2)}=\rho \), \(R_{1} \approx R\approx R_{2}, h_{1} =h\) and \(h_{2} =0\), then Eq. (56) simplifies to
which is the same as that first derived in [4] for single-layer transversely isotropic thin tubes without including the transverse shear, rotary inertia and radial stress effects
4.4.2 Composite tube with two cubic material layers
For cubic materials with three independent elastic constants E, \(\nu \) and \(\mu \), the stiffness components for orthotropic materials introduced in Eq. (3) simplify to (e.g., [42, 45])
Applying Eq. (58) to each cubic material layer and then using Eq. (30) along with Eq. (36), (37a),(38a) or (38b) will lead to the determination of the critical velocity \(V_{{cr}_{0}}\), and substituting Eq. (58) into Eqs. (25), (26) and (16a), respectively, will yield the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) as
for a composite tube consisting of two dissimilar cubic material layers, which incorporate the transverse shear, rotary inertia and radial stress effects and have no restriction on the magnitude of the pressure velocity.
When \(V \ll V_{{cr}_{\textrm{1}}}\) and \(V \ll V_{{cr}_{\textrm{3}}}\), using Eq. (58) in Eq. (44) leads to
as the critical velocity \(V_{{cr}_{\textrm{0}}}\)for a composite tube consisting of two dissimilar cubic material layers under an internal pressure moving at a constant velocity V \(<<\) min(\(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{3}}})\), which has precluded the transverse shear and rotary inertia effects but accounts for the radial stress effect. If the tube is homogeneous with \(E^{(1)}=E^{(2)}=E,\,\,\nu ^{(1)}=\nu ^{(2)}=\nu ,\rho ^{(1)}=\rho ^{(2)}=\rho , R_{1} \approx R\approx R_{2},h_{1} =h\) and \(h_{2} =0\), then Eq. (60) reduces to
which is the same as that first derived in [4] for single-layer cubic material tubes without including the transverse shear and rotary inertia effects
For thin cubic material cylindrical shells with \(\sigma _{zz} \approx 0\) and under axisymmetric loading, the elastic stiffness constants for orthotropic materials introduced in Eq. (3) reduce to [4, 42]
Using Eq. (62) for each cubic material thin layer and then applying Eq. (30) along with Eq. (36), (37a),(38a) or (38b) will result in the critical velocity \(V_{{cr}_{0}}\), and substituting Eq. (62) into Eqs. (25), (26) and (16a), respectively, will lead to the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) as
for a composite tube consisting of two dissimilar cubic material thin layers, which include the transverse shear and rotary inertia effects (but exclude the radial stress effect) and have no restriction on the magnitude of the pressure velocity.
When \(V\ll V_{{cr}_{\textrm{1}}}\) and \(V\ll V_{{cr}_{\textrm{3}}}\), the use of Eq. (62) in Eq. (44) yields
as the critical velocity \(V_{{cr}_{\textrm{0}}}\)for a composite tube consisting of two dissimilar cubic material thin layers under an internal pressure moving at a constant velocity V \(<<\) min(\(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{3}}})\), which has excluded the transverse shear, rotary inertia and radial stress effects. If the tube is homogeneous with \(E^{(1)}=E^{(2)}=E,\nu ^{(1)}=\nu ^{(2)}=\nu ,\rho ^{(1)}=\rho ^{(2)}=\rho , R_{1} \approx R\approx R_{2},h_{1} =h\) and \(h_{2} =0,\) then Eq. (64) simplifies to
which is the same as that first derived in [4] for single-layer cubic material thin tubes without including the transverse shear, rotary inertia and radial stress effects
4.4.3 Composite tube with two isotropic layers
For isotropic materials with two independent elastic constants E (Young’s modulus) and \(\nu \) (Poisson’s ratio), the stiffness components for orthotropic materials introduced in Eq. (3) simplify to (e.g., [27])
Using Eq. (66) for each isotropic layer and then applying Eq. (30) along with Eq. (36), (37a), (38a) or (38b) will give the critical velocity \(V_{{cr}_{0}}\), and substituting Eq. (66) into Eqs. (25), (26) and (16a), respectively, will yield the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) as
for a composite tube consisting of two dissimilar isotropic layers, which incorporate the transverse shear, rotary inertia and radial stress effects and have no restriction on the magnitude of the pressure velocity.
When V \(<<\) \(V_{{cr}_{\textrm{1}}}\) and V \(<<\) \(V_{{cr}_{\textrm{3}}}\), substituting Eq. (66) into Eq. (44) gives
as the critical velocity for a composite tube consisting of two dissimilar isotropic layers under an internal pressure moving at a constant velocity V \(<<\) min(\(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{3}}})\), which has precluded the transverse shear and rotary inertia effects but accounts for the radial stress effect. If the tube is homogeneous with \(E^{(1)}=E^{(2)}=E,\,\,\nu ^{(1)}=\nu ^{(2)}=\nu ,\rho ^{(1)}=\rho ^{(2)}=\rho ,R_{1} \approx R\approx R_{2},h_{1} =h\) and \(h_{2} =0\), then Eq. (68) reduces to
which is the same as that first derived in [4] for single-layer isotropic tubes without including the transverse shear and rotary inertia effects
For the composite tube with two dissimilar isotropic thin layers, \(\sigma _{zz} \approx 0\) and the expressions for the stiffness components \(C_{\textrm{11}}\), \(C_{\textrm{12}}\), \(C_{\textrm{22}}\) and \(C_{\textrm{55}}\) are the same as those for the cubic material thin layers listed in Eq. (62) except that \(\mu =\frac{E}{2(1+\nu )}\) in the current case.
Applying Eq. (62) to each isotropic thin layer and then using Eq. (30) along with Eq. (36), (37a), (38a) or (38b) will lead to the critical velocity \(V_{{cr}_{0}}\), and substituting Eq. (62) into Eqs. (25), (26) and (16a), respectively, will yield the critical velocities \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) and \(V_{{cr}_{\textrm{3}}}\) that are, respectively, the same as those listed in Eqs. (63a), (63b) and (63c) for the composite tube consisting of two dissimilar cubic material thin layers, which include the transverse shear and rotary inertia effects (but exclude the radial stress effect) and have no restriction on the magnitude of the pressure velocity.
When \(V\ll \min (V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{3}}})\), substituting Eq. (62) into Eq. (44) will yield the critical velocity formula \(V_{{cr}_{0}}\) for a composite tube consisting of two dissimilar isotropic thin layers under an internal pressure moving at a constant velocity \(V\ll \min (V_{{cr}_{\textrm{1}}}, V_{{cr}_{\textrm{3}}})\), which will be the same as that obtained in Eq. (64) for the composite tube with two dissimilar cubic material thin layers without the transverse shear, rotary inertia and radial stress effects except that \(\mu =\frac{E}{2(1+\nu )}\) here.
If the tube is homogeneous with \(E^{(1)}=E^{(2)}=E,\,\,\nu ^{(1)}=\nu ^{(2)}=\nu ,\rho ^{(1)}=\rho ^{(2)}=\rho , R_{1} \approx R\approx R_{2},h_{1} =h\) and \(h_{2} =0\), then the critical velocity formula for a single-layer isotropic thin tube under an internal pressure moving at a constant velocity V \(<<\) \(V_{{cr}_{\textrm{3}}}\) (\(=V_{{cr}_{\textrm{1}}}\)here) can be obtained from Eq. (64) as a special case, which will be the same as that derived in Eq. (65) for single-layer cubic material thin tubes.
The general formulas obtained in Sect. 3 can also be directly used for composite tubes containing two layers with a combination of any two of the four types of materials discussed in this section, including orthotropic, transversely isotropic, cubic and isotropic
5 Example
To quantitatively illustrate the new formulas derived in Sects. 3 and 4, a numerical example is provided herein.
Consider a composite tube consisting of an isotropic steel inner layer with \(R_{\textrm{1}} = 31.524\) mm, \(h_{\textrm{1}}=3.048\) mm, \(\rho ^{\mathrm {(1)}} = 7870.9755\) kg/m\(^{\textrm{3}}\), \(E^{\mathrm {(1)}}= 208.9111\) GPa and \(\nu ^{\mathrm {(1)}}=0.3\) and an orthotropic outer layer with \(R_{\textrm{2}} = 34.8768\) mm, \(h_{\textrm{2}} = 3.6576\) mm, \(\rho ^{\mathrm {(2)}} =2107.4764\) kg/m\(^{\textrm{3}}\), \(C_{11}^{(2)} = 22.1312\) GPa, \(C_{22}^{(2)} = 40.2654\) GPa, \(C_{12}^{(2)} = 9.6527\) GPa and \(C_{55}^{(2)} =2\mu _{xz}^{(2)} = 10.4345\) GPa Note that the value of \(C_{55}^{(2)}\) listed here is estimated using the computer program “PC-Laminate” that was originally employed to obtain the properties of the outer layer (as an 18-ply \({+}60^{\circ }\)/\(-60^{\circ }\) S glass-epoxy laminate) by Simkins [15] The geometrical and the other material constants for the inner and outer layers of the composite tube are directly taken from [15] for the case with predicted values. In [15], the critical velocity \(V_{{cr}_{0}}\) was numerically determined as the minimum phase velocity from a dispersion curve, while the other critical velocities \(V_{{cr}_{\textrm{1}}}-V_{{cr}_{\textrm{3}}}\) were not discussed due to the limitations of the approach adopted there
From the closed-form formulas given in Eqs. (16a), (25) and (26), the critical velocities \(V_{{cr}_{\textrm{1}}}-V_{{cr}_{\textrm{3}}}\) of this two-layer composite tube can be directly obtained as
The critical velocity \(V_{{cr}_{\textrm{0}}}\) can be determined as follows by using the general formulas derived in Sect. 3.
For the general case incorporating the transverse shear, rotary inertia and radial stress effects, it follows from Eqs. (29a)–(29e), (32a), (32b), (34b), (34c) and (35) that for the current two-layer composite tube,
With \(\Delta _{d}>\)0 from Eq. (71), it follows from Eq. (36) that
as the three distinct real roots of Eq. (31). Substituting \(\lambda _{\textrm{1}}\), \(\lambda _{\textrm{2}}\) and \(\lambda _{\textrm{3}}\) into Eq. (30), respectively, yields the following common set of values for \(V^{\textrm{2}}\) (as the four roots of the quartic equation in Eq. (28)):
where the last root does not lead to any real value of V. The smallest positive value of V from the first three roots in Eq. (73) gives the critical velocity \(V_{{cr}_{0}}\) for the two-layer composite tube as
When the transverse shear effect is neglected, the critical velocities \(V_{{cr}_{\textrm{1}}}-V_{{cr}_{\textrm{3}}}\) of the two-layer composite tube can be determined from Eqs. (16a), (26) and (40) as
and the critical velocity \(V_{{cr}_{\textrm{0}}}\) can be obtained from solving Eq. (41) as [24]
When both the transverse shear and rotary inertia effects are suppressed, the critical velocity \(V_{{cr}_{\textrm{0}}}\) for the two-layer composite tube can be found from solving Eq. (46) as [24]
The numerical values of \(V_{{cr}_{0}}\) of the two-layer composite tube determined above using the new formulas are listed in Table 1, where they are also compared to the value of \(V_{{cr}_{0}}\) obtained numerically in [15] by plotting the dispersion curve, which reads
It is clear from comparing the values of \(V_{{cr}_{\textrm{0}}}\) listed in Table 1 with those of \(V_{{cr}_{\textrm{1}}}- V_{{cr}_{\textrm{3}}}\) given in Eq. (70) or (75) for the two-layer composite tube with or without the transverse shear effect that \(V_{{cr}_{\textrm{0}}}\) is much smaller than \(V_{{cr}_{\textrm{1}}}\), \(V_{{cr}_{\textrm{2}}}\) or \(V_{{cr}_{\textrm{3}}}\) in each case. This agrees with what was observed for the single-layer isotropic steel tube [4, 14]
From Table 1, it is seen that the critical velocity \(V_{cr}\) obtained from the formulas without including the transverse shear effect is higher than that predicted by the general formulas incorporating the transverse shear and rotary inertia effects In addition, it is observed that the value of \(V_{{cr}_{0}}\) determined without considering the rotary inertia effect is larger than that with the rotary inertia effect. These indicate that the simplified formulas in the cases excluding the transverse shear effect and/or the rotary inertia effect over-predict the critical velocity \(V_{{cr}_{\textrm{0}}}\) of the composite tube and may lead to unsafe designs. This finding is consistent with what was revealed for single-layer homogeneous tubes [4, 14].
Moreover, Table 1 shows that the values of the lowest critical velocity \(V_{{cr}_{0}}\) predicted by the new formulas agree fairly well with that numerically determined by Simkins [15] from plotting the dispersion curve of traveling flexural waves using the laminated orthotropic shell model of Dong [21]. In particular, the value of \(V_{{cr}_{0}}\) given by the general formulas incorporating the transverse shear and rotary inertia effects (i.e., the one with no constraint) is closest to that of Simkins [15]. This provides a validation of the newly developed analytical model.
6 Summary
Closed-form formulas are obtained for four critical velocities of a composite tube consisting of two laminated cylindrical layers of dissimilar materials under a uniform internal pressure moving at a constant velocity. The formulation is based on a first-order shear deformation shell theory incorporating the effects of transverse shear, rotary inertia and material anisotropy, which leads to a unified treatment of composite tubes containing two layers of dissimilar materials with the orthotropic, transversely isotropic, cubic or isotropic symmetry.
The formulas for two-layer composite tubes derived for the general case include the transverse shear, rotary inertia and radial stress effects and consider the material anisotropy for each layer, which are reduced to those for the special cases without the transverse shear, rotary inertia or radial stress effect and with simpler material symmetry. It is shown that the current new model for two-layer composite tubes recovers the model for single-layer, homogeneous tubes as a special case.
The example for a composite tube with an isotropic inner layer and an orthotropic outer layer shows that the values of the lowest critical velocity predicted by the newly derived closed-form formulas are in good agreement with that numerically determined by Simkins [15]
References
Simkins, T.E., Pflegl, G.A., Stilson, E.G.: Dynamic strains in a 60 mm gun tube: an experimental study. J. Sound Vib. 168, 549–557 (1993)
Simkins, T.E.: Amplification of flexural waves in gun tubes. J. Sound Vib. 172, 145–154 (1994)
Simkins, T.E.: The influence of transient flexural waves on dynamic strains in cylinders. ASME J. Appl. Mech. 62, 262–265 (1995)
Gao, X.-L., Littlefield, A.G.: Critical velocities and displacements of anisotropic tubes under a moving pressure. Math. Mech. Solids 27, 2662–2688 (2022)
Hölzle, J.: Influence of the “critical velocity’’ phenomenon on chromium-plated gun barrels. ASME J. Pres. Ves. Tech. 125, 352–354 (2003)
Nechitailo, N.V., Lewis, K.B.: Critical velocity for rails in hypervelocity launchers. Int. J. Impact Eng. 33, 485–495 (2006)
Ruzzene, M., Baz, A.: Dynamic stability of periodic shells with moving loads. J. Sound Vib. 296, 830–844 (2006)
Sofiyev, A.H.: Dynamic response of an FGM cylindrical shell under moving loads. Compos. Struct. 93, 58–66 (2010)
Eipakchi, H., Nasrekani, F. M.: Vibrational behavior of composite cylindrical shells with auxetic honeycombs core layer subjected to a moving pressure. Compos. Struct. 254, 112847 (2020)
Prisekin, V.L.: The stability of a cylindrical shell subjected to a moving load. Mekhanika i Mashinostroenie 5, 133–134 (1961)
Jones, J.P., Bhuta, P.G.: Response of cylindrical shells to moving loads. ASME J. Appl. Mech. 31, 105–111 (1964)
Tang, S.-C.: Dynamic response of a tube under moving pressure. J .Eng. Mech. Div. 91(5), 97–122 (1965)
Tzeng, J.T., Hopkins, D.A.: Dynamic response of composite cylinders subjected to a moving internal pressure. J. Reinf. Plas. Compos. 15, 1088–1105 (1996)
Gao, X.-L.: Critical velocities of anisotropic tubes under a moving pressure incorporating transverse shear and rotary inertia effects. Acta Mech. 233, 3511–3534 (2022)
Simkins, T. E.: Dynamic strains in an orthotropically-wrapped gun tube. Part I—Theoretical. Technical Report ARCCB-TR-93026. U.S. Army Armament Research, Development and Engineering Center, Benét Laboratories, Watervliet, NY (1993)
Littlefield, A.G., Hyland, E.J., Andalora, A., Klein, N., Langone, R., Becker, R.: Carbon fiber/thermoplastic overwrapped gun tube. ASME J. Pres. Ves. Tech. 128, 257–262 (2006)
Littlefield, A. G., Hyland, E. J.: 120 mm prestressed carbon fiber/thermoplastic overwrapped gun tubes. ASME J. Pres. Ves. Tech. 134, 041008 (2012)
Jones, J.P., Whittier, J.S.: Axially symmetric motions of a two-layered Timoshenko-type cylindrical shell. ASME J. Appl. Mech. 33, 838–844 (1966)
Chonan, S.: Moving load on a two-layered cylindrical shell with imperfect bonding. J. Acoust. Soc. Am. 69, 1015–1020 (1981)
Herrmann, G., Mirsky, I.: Three-dimensional and shell-theory analysis of axially symmetric motions of cylinders. ASME J. Appl. Mech. 23, 563–568 (1956)
Dong, S.B.: Free vibration of laminated orthotropic cylindrical shells. J. Acoust. Soc. Am. 44, 1628–1635 (1968)
Bert, C.W., Birman, V.: Parametric instability of thick, orthotropic, circular cylindrical shells. Acta Mech. 71, 61–76 (1988)
Zhang, G.Y., Gao, X.-L.: A non-classical model for first-order shear deformation circular cylindrical thin shells incorporating microstructure and surface energy effects. Math. Mech. Solids 26, 1294–1319 (2021)
Gao, X.-L.: Critical velocities of a two-layer composite tube under a moving internal pressure. Acta Mech. 234, 2021–2043 (2023)
Leissa, A. W.: Vibration of shells. In: NASA SP-288. Scientific and Technical Information Office. National Aeronautics and Space Administration, Washington, DC (1973)
Mirsky, I.: Axisymmetric vibrations of orthotropic cylinders. J. Acous. Soc. Am. 36, 2106–2112 (1964)
Gao, X.-L.: Two displacement methods for in-plane deformations of orthotropic linear elastic materials. Z. Angew. Math. Phys. 52, 810–822 (2001)
Zhang, G.Y., Gao, X.-L., Guo, Z.Y.: A non-classical model for an orthotropic Kirchhoff plate embedded in a viscoelastic medium. Acta Mech. 228, 3811–3825 (2017)
Cao, R., Li, L., Li, X., Mi, C.: On the frictional receding contact between a graded layer and an orthotropic substrate indented by a rigid flat-ended stamp. Mech. Mater. 158, 103847 (2021)
Labuschagne, A., van Rensburg, N.F.J., van der Merwe, A.J.: Comparison of linear beam theories. Math. Comput. Modell. 49, 20–30 (2009)
Zhang, G.Y., Gao, X.-L., Bishop, J.E., Fang, H.E.: Band gaps for elastic wave propagation in a periodic composite beam structure incorporating microstructure and surface energy effects. Compos. Struct. 189, 263–272 (2018)
Ma, H.M., Gao, X.-L., Reddy, J.N.: A non-classical Mindlin plate model based on a modified couple stress theory. Acta Mech. 220, 217–235 (2011)
Gao, X.-L.: A new Timoshenko beam model incorporating microstructure and surface energy effects. Acta Mech. 226, 457–474 (2015)
Gao, X.-L., Zhang, G. Y.: A non-classical Mindlin plate model incorporating microstructure, surface energy and foundation effects. Proc. R. Soc. A 472, 20160275 (2016)
Steigmann, D.J.: On the relationship between the Cosserat and Kirchhoff–Love theories of elastic shells. Math. Mech. Solids 4, 275–288 (1999)
Zhang, G.Y., Gao, X.-L., Littlefield, A.G.: A non-classical model for circular cylindrical thin shells incorporating microstructure and surface energy effects. Acta Mech. 232, 2225–2248 (2021)
Auckly, D.: Solving the quartic with a pencil. Am. Math. Mon. 114, 29–39 (2007)
Okoli, O.C., Oraekie, P., Okeke, N.C.: Alternative method of solution to quartic equation. Coou J. Phys. Sci. 2(8), 13–19 (2019)
Nickalls, R.W.D.: Viète, Descartes and the cubic equation. Math. Gaz. 90(518), 203–208 (2006)
Okereke, O.E., Iwueze, I.S., Ohakwe, J.: Some contributions to the solution of cubic equations. Br. J. Math. Comput. Sci. 4, 2929–2941 (2014)
Okoli, O.C., Laisin, M., Nsiegbe, N.A., Eze, A.C.: Method of solution to cubic equation. Coou J. Phys. Sci. 3, 515–521 (2020)
Bower, A.F.: Applied Mechanics of Solids. CRC Press, Boca Raton, FL (2009)
Gao, X.-L., Mao, C. L.: Solution of the contact problem of a rigid conical frustum indenting a transversely isotropic elastic half-space. ASME J. Appl. Mech. 81, 041007 (2014)
Zhang, G. Y., Qu, Y. L., Gao, X.-L. Jin, F.: A transversely isotropic magneto-electro-elastic Timoshenko beam model incorporating microstructure and foundation effects. Mech. Mater. 149, 103412 (2020)
Ai, L. Gao, X.-L.: Micromechanical modeling of 3-D printable interpenetrating phase composites with tailorable effective elastic properties including negative Poisson’s ratio. J. Micromech. Mol. Phys. 2, 1750015 (2017)
Acknowledgements
The author would like to thank Professor David Steigmann for his encouragement and comments.
Funding
Open access funding provided by SCELC, Statewide California Electronic Library Consortium.
Author information
Authors and Affiliations
Contributions
X.-L. Gao conducted the research and wrote the paper.
Corresponding author
Ethics declarations
Conflict of interest
The author declares no competing interests.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Gao, XL. Critical velocities of a two-layer composite tube incorporating the effects of transverse shear, rotary inertia and material anisotropy. Z. Angew. Math. Phys. 74, 166 (2023). https://doi.org/10.1007/s00033-023-02023-8
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00033-023-02023-8
Keywords
- Critical velocity
- Composite tubes
- Moving pressure
- First-order shear deformation shell theory
- Transverse shear
- Rotary inertia
- Orthotropic
- Transversely isotropic
- Cubic or isotropic material