Abstract
In this paper, we consider a diffusive Lotka–Volterra predator–prey system with spatial heterogeneity and two delays. We first show that there exists a nonconstant positive steady state when the diffusion rates of prey and predator are large. Then, we obtain the stability of the steady state and show the existence of a Hopf bifurcation. Moreover, some numerical simulations are provided to illustrate our theoretical results.




Similar content being viewed by others
Data availability
All data generated or analyzed during this study are included in this published article.
References
An, Q., Wang, C., Wang, H.: Analysis of a spatial memory model with nonlocal maturation delay and hostile boundary condition. Discrete Contin. Dyn. Syst. 40(10), 5845–5868 (2020)
Blat, J., Brown, K.J.: Bifurcation of steady-state solutions in predator–prey and competition systems. Proc. Roy. Soc. Edinburgh Sect. A 97, 21–34 (1984)
Busenberg, S., Huang, W.: Stability and Hopf bifurcation for a population delay model with diffusion effects. J. Differ. Equ. 124(1), 80–107 (1996)
Chen, S., Lou, Y., Wei, J.: Hopf bifurcation in a delayed reaction–diffusion–advection population model. J. Differ. Equ. 264(8), 5333–5359 (2018)
Chen, S., Shi, J.: Stability and Hopf bifurcation in a diffusive logistic population model with nonlocal delay effect. J. Differ. Equ. 253(12), 3440–3470 (2012)
Chen, S., Yu, J.: Stability and bifurcations in a nonlocal delayed reaction–diffusion population model. J. Differ. Equ. 260(1), 218–240 (2016)
Chen, S., Shi, J.: Global dynamics of the diffusive Lotka–Volterra competition model with stage structure. Calc. Var. Part. Differ. Equ. 59(1), 33, 19 (2020)
Chen, S., Wei, J.: Stability and bifurcation in a diffusive logistic population model with multiple delays. Internat. J. Bifur. Chaos Appl. Sci. Eng. 25(8), 1550107, 9 (2015)
Dockery, J., Hutson, V., Mischaikow, K., Pernarowski, M.: The evolution of slow dispersal rates: a reaction diffusion model. J. Math. Biol. 37(1), 61–83 (1998)
Du, Y., Hsu, S.-B.: A diffusive predator–prey model in heterogeneous environment. J. Differ. Equ. 203(2), 331–364 (2004)
Du, Y., Niu, B., Guo, Y., Wei, J.: Double Hopf bifurcation in delayed reaction–diffusion systems. J. Dyn. Differ. Equ. 32(1), 313–358 (2020)
Du, Y., Shi, J.: Allee effect and bistability in a spatially heterogeneous predator–prey model. Trans. Am. Math. Soc. 359(9), 4557–4593 (2007)
Faria, T.: Stability and bifurcation for a delayed predator–prey model and the effect of diffusion. J. Math. Anal. Appl. 254(2), 433–463 (2001)
Guo, S.: Stability and bifurcation in a reaction–diffusion model with nonlocal delay effect. J. Differ. Equ. 259(4), 1409–1448 (2015)
Guo, S.: Patterns in a nonlocal time-delayed reaction–diffusion equation. Z. Angew. Math. Phys. 69(1), 1–31 (2018)
Guo, S., Yan, S.: Hopf bifurcation in a diffusive Lotka–Volterra type system with nonlocal delay effect. J. Differ. Equ. 260(1), 781–817 (2016)
He, X., Lam, K.-Y., Lou, Y., Ni, W.-M.: Dynamics of a consumer-resource reaction–diffusion model. J. Math. Biol. 78(6), 1605–1636 (2019)
He, X., Ni, W.-M.: The effects of diffusion and spatial variation in Lotka–Volterra competition-diffusion system I: Heterogeneity vs. homogeneity. J. Differ. Equ. 254(2), 528–546 (2013)
He, X., Ni, W.-M.: Global dynamics of the Lotka–Volterra competition-diffusion system: diffusion and spatial heterogeneity I. Commun. Pure Appl. Math. 69(5), 981–1014 (2016)
Hu, G.-P., Li, W.-T.: Hopf bifurcation analysis for a delayed predator–prey system with diffusion effects. Nonlinear Anal. Real World Appl. 11(2), 819–826 (2010)
Huang, D., Chen, S.: The stability and Hopf bifurcation of the diffusive Nicholson’s blowflies model in spatially heterogeneous environment. Z. Angew. Math. Phys. 72(1), 41, 24 (2021)
Huang, Y., Li, F., Shi, J.: Stability of synchronized steady state solution of diffusive Lotka–Volterra predator–prey model. Appl. Math. Lett. 105, 106331, 5 (2020)
Korman, P.: Dynamics of the Lotka–Volterra systems with diffusion. Appl. Anal. 44(3–4), 191–207 (1992)
Lam, K.-Y., Ni, W.-M.: Uniqueness and complete dynamics in heterogeneous competition-diffusion systems. SIAM J. Appl. Math. 72(6), 1695–1712 (2012)
Li, S., Wu, J.: Asymptotic behavior and stability of positive solutions to a spatially heterogeneous predator–prey system. J. Differ. Equ. 265(8), 3754–3791 (2018)
Li, Z., Dai, B.: Stability and Hopf bifurcation analysis in a Lotka–Volterra competition-diffusion-advection model with time delay effect. Nonlinearity 34(5), 3271–3313 (2021)
Li, Z., Dai, B., Han, R.: Hopf bifurcation in a reaction–diffusion–advection two species model with nonlocal delay effect. J. Dyn. Differ. Equ. 1–34 (2021)
Lou, Y.: On the effects of migration and spatial heterogeneity on single and multiple species. J. Differ. Equ. 223(2), 400–426 (2006)
Lou, Y., Wang, B.: Local dynamics of a diffusive predator–prey model in spatially heterogeneous environment. J. Fixed Point Theory Appl. 19(1), 755–772 (2017)
Ma, L., Feng, Z.: Stability and bifurcation in a two-species reaction–diffusion–advection competition model with time delay. Nonlinear Anal. Real World Appl. 61, 103327 (2021)
Ma, L., Guo, S.: Stability and bifurcation in a diffusive Lotka–Volterra system with delay. Comput. Math. Appl. 72(1), 147–177 (2016)
Ma, L., Xie, X.: Bifurcation analysis of coexistent state in a delayed two-species predator–prey model. Appl. Anal. 99(7), 1195–1217 (2020)
Ni, W.-M.: The Mathematics of Diffusion. CBMS-NSF Regional Conference Series in Applied Mathematics, vol. 82. Society for Industrial and Applied Mathematics (SIAM), Philadelphia (2011)
Ruan, S.: On nonlinear dynamics of predator–prey models with discrete delay. Math. Model. Nat. Phenom. 4(2), 140–188 (2009)
Shi, J., Wang, C., Wang, H.: Diffusive spatial movement with memory and maturation delays. Nonlinearity 32(9), 3188–3208 (2019)
Shi, Q., Shi, J., Song, Y.: Hopf bifurcation in a reaction–diffusion equation with distributed delay and Dirichlet boundary condition. J. Differ. Equ. 263(10), 6537–6575 (2017)
Song, Y., Wei, J.: Local Hopf bifurcation and global periodic solutions in a delayed predator–prey system. J. Math. Anal. Appl. 301(1), 1–21 (2005)
Su, Y., Wei, J., Shi, J.: Hopf bifurcations in a reaction–diffusion population model with delay effect. J. Differ. Equ. 247(4), 1156–1184 (2009)
Sun, X., Yuan, R.: Hopf bifurcation in a diffusive population system with nonlocal delay effect. Nonlinear Anal. 214(1), 112544, 21 (2022)
Sun, X., Yuan, R., Wang, L.: Bifurcations in a diffusive predator–prey model with Beddington–DeAngelis functional response and nonselective harvesting. J. Nonlinear Sci. 29(1), 287–318 (2019)
Wu, J.: Theory and Applications of Partial Functional-Differential Equations. Applied Mathematical Sciences, vol. 119. Springer, New York (1996)
Yan, X.-P., Li, W.-T.: Stability of bifurcating periodic solutions in a delayed reaction–diffusion population model. Nonlinearity 23(6), 1413–1431 (2010)
Yan, X.-P., Li, W.-T.: Stability and Hopf bifurcations for a delayed diffusion system in population dynamics. Discrete Contin. Dyn. Syst. Ser. B 17(1), 367–399 (2012)
Yang, R., Nie, C., Jin, D.: Spatiotemporal dynamics induced by nonlocal competition in a diffusive predator–prey system with habitat complexity. Nonlinear Dyn. 110, 879–900 (2022)
Zuo, W., Wei, J.: Stability and Hopf bifurcation in a diffusive predator–prey system with delay effect. Nonlinear Anal. Real World Appl. 12(4), 1998–2011 (2011)
Acknowledgements
The authors are grateful to two anonymous reviewers for their thoughtful and valuable comments which greatly improve the original manuscript. They also thank Professor Shanshan Chen for her helpful suggestions on the manuscript.
Funding
This research is supported by National Natural Science Foundation of China (Nos. 12171117 and 11771109).
Author information
Authors and Affiliations
Contributions
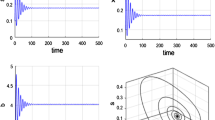
H. Zhang and J. Wei wrote the main manuscript text, and H. Zhang prepared figures 1–4. All authors reviewed the manuscript.
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Ethical approval
Not applicable.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
In this subsection, we study the stability change of the positive steady state \(U_{\lambda }\) when \(\tau \) and r are proportional. Without loss of generality, we let \(\tau =\delta r\), where \(\delta >0\) is a fixed positive constant. The method used is similar to that in Sect. 3. In this case, the infinitesimal generator of the solution semigroup of (3.4) can be defined by
with
and \(C_{\mathbb {C}}=C([-\max \{\delta r,r\},0],X^{2}_{\mathbb {C}})\), \(C_{\mathbb {C}}^{1}=C^{1}([-\max \{\delta r,r\},0],X^{2}_{\mathbb {C}})\). Then, the spectrum of \(\mathfrak {A}_{\lambda }(r)\) is
where
We first analyze whether \(\mathfrak {A}_{\lambda }(r)\) has purely imaginary eigenvalues when \(r>0\) and \(\bar{a}_{1}\bar{a}_{4}<\bar{a}_{2}\bar{a}_{3}\). Similar to the case where \(\tau \) is fixed and r changes, set \(\mu =\pm \textrm{i}\omega (\omega >0)\), \(\omega =\lambda h\), \(\theta =\omega r\), and ignore a scale factor, take \(\psi =(\psi _1,\psi _2)^T\), where
Then, (4.2) becomes
Define \(\check{G}:(X_{1})_{\mathbb {C}}^{2}\times \mathbb {R}^{6}\mapsto Y_{\mathbb {C}}^{2}\times \mathbb {R}\) as \(\check{G}=(\check{g}_{1},\check{g}_{2},\check{g}_{3})\). If
is solvable, then \(\mathfrak {A}_{\lambda }(r)\) has purely imaginary eigenvalues \(\mu =\textrm{i}\omega =\textrm{i} \lambda h\). Using similar arguments as in Lemma 3.2 and Theorem 3.3, we have the following result.
Theorem 4.1
Suppose that (2.2) holds, and \(\bar{a}_{1}\bar{a}_{4}<\bar{a}_{2}\bar{a}_{3}\). Then, there exists \(0<\check{\lambda }\ll 1\), and a continuously differential mapping \(\lambda \mapsto (\check{z}_{1\lambda }, \check{z}_{2\lambda },\check{s}_{\lambda },\check{R}_{1\lambda },\check{R}_{2\lambda }, \check{h}_{\lambda },\check{\theta }_{\lambda })\) from \([0,\check{\lambda }]\) to \((X_{1})^{2}_{\mathbb {C}}\times \mathbb {R}^{5}\), such that (4.4) has a unique solution \((\check{z}_{1\lambda }, \check{z}_{2\lambda },\check{s}_{\lambda },\check{R}_{1\lambda },\check{R}_{2\lambda }, \check{h}_{\lambda },\check{\theta }_{\lambda })\). Moreover, when \(\lambda =0\),
and \((\check{z}_{10},\check{z}_{20})\) uniquely solves the following system
As a consequence of Theorem 4.1, we obtain the existence of purely imaginary eigenvalues of \(\mathfrak {A}_{\lambda }(r)\) as follows.
Theorem 4.2
Suppose that (2.2) holds, and \(\bar{a}_{1}\bar{a}_{4}<\bar{a}_{2}\bar{a}_{3}\). Then for \(\lambda \in (0,\check{\lambda }]\) with \(0<\check{\lambda }\ll 1\), \(\Pi (\lambda ,\textrm{i}\omega ,r)\psi =0\) with \(\omega >0\), \(r>0\) and \(\psi \in X^2_\mathbb {C}\setminus \{\textbf{0}\}\) if and only if
where \(\check{a}\) is a nonzero constant. \(\check{z}_{i\lambda }\), \(\check{s}_{\lambda }\), \(\check{R}_{i\lambda }\), \(\check{h}_{\lambda }\), \(\check{\theta }_{\lambda }\), \(i=1,2\) are defined in Theorem 4.1.
Similarly in Theorems 3.7 and 3.8, we can obtain the simplicity of \(\textrm{i}\check{\omega }_{\lambda }\) and the transversality condition. From Lemma 3.10, we know that there exists \(0<\check{\lambda }\ll 1\) such that when \(\bar{a}_{1}\bar{a}_{4}\le \bar{a}_{2}\bar{a}_{3}\) and \(r=0\), all eigenvalues of \(\mathfrak {A}_{\lambda }(r)\) have negative real parts for \(\lambda \in (0,\check{\lambda }]\). Moreover, similar to Lemma 3.9, we can verify that when \(\bar{a}_{1}\bar{a}_{4}>\bar{a}_{2}\bar{a}_{3}\), all real parts of eigenvalues of \(\mathfrak {A}_{\lambda }(r)\) are negative for any \(r\ge 0\) and \(\lambda \in (0,\check{\lambda }]\) for sufficiently small \(\check{\lambda }\). Thus, we have the similar result to Theorem 3.11.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Zhang, H., Wei, J. Hopf bifurcation analysis in a diffusive predator–prey system with spatial heterogeneity and delays. Z. Angew. Math. Phys. 74, 98 (2023). https://doi.org/10.1007/s00033-023-01990-2
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00033-023-01990-2