Abstract
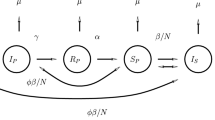
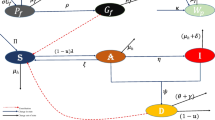
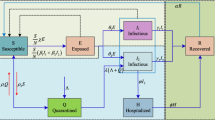
We investigate a susceptible-infected-susceptible (SIS) epidemic model based on the Caputo–Fabrizio operator. After performing an asymptotic analysis of the system, we study a related finite horizon optimal control problem with state constraints. We prove that the corresponding value function satisfies in the viscosity sense a dynamic programming equation. We then turn to the asymptotic behavior of the value function, proving its convergence to the solution of a stationary problem, as the planning horizon tends to infinity. Finally, we present some numerical simulations providing a qualitative description of the optimal dynamics and the value functions involved.






Similar content being viewed by others
References
Bardi, M., Da Lio, F.: On the bellman equation for some unbounded control problems. Nonlinear Differ. Equ. Appl. 4(4), 491–510 (1997)
Balzotti, C., D’Ovidio, M., Lai, A.C., Loreti, P.: Effects of fractional derivatives with different orders in sis epidemic models. Computation 9, 89 (2021)
Behncke, H.: Optimal control of deterministic epidemics. Optimal Control Appl. Methods 21(6), 269–285 (2000)
Capuzzo-Dolcetta, I., Lions, P.-L.: Hamilton-jacobi equations with state constraints. Trans. Am. Math. Soc. 318(2), 643–683 (1990)
Caputo, M., Fabrizio, M.: A new definition of fractional derivative without singular kernel. Progr. Fract. Differ. Appl 1(2), 1–13 (2015)
Chen, Y., Liu, F., Qiang, Yu., Li, T.: Review of fractional epidemic models. Appl. Math. Model. 97, 281–307 (2021)
Capasso, V., Serio, G.: A generalization of the Kermack–McKendrick deterministic epidemic model. Math. Biosci. 42(1–2), 43–61 (1978)
Falcone, M., Ferretti, R.: Semi-Lagrangian approximation schemes for linear and Hamilton–Jacobi equations. SIAM (2013)
Fujita, Y., Ishii, H., Loreti, P.: Asymptotic solutions of Hamilton–Jacobi equations in Euclidean n space. Indiana Univ. Math. J. 55(5), 1671–1700 (2006)
Kirschner, D., Panetta, J.C.: Modeling immunotherapy of the tumor-immune interaction. J. Math. Biol. 37(3), 235–252 (1998)
Kumar, D., Singh, J., Al Qurashi, M., Baleanu, D.: A new fractional SIRS-SI malaria disease model with application of vaccines, antimalarial drugs, and spraying. Adv. Differ. Equ. 2019(1), 278 (2019)
Lions, P.-L.: Generalized solutions of Hamilton–Jacobi equations, vol. 69. Pitman, London (1982)
Losada, J., Nieto, J.J.: Properties of a new fractional derivative without singular kernel. Progr. Fract. Differ. Appl. 1, 87–92 (2015)
Loreti, P.: Some properties of constrained viscosity solutions of Hamilton–Jacobi–Bellman equations. SIAM J. Control. Optim. 25(5), 1244–1252 (1987)
Li, T., Wang, Y., Liu, F., Turner, I.: Novel parameter estimation techniques for a multi-term fractional dynamical epidemic model of dengue fever. Numer. Algorithms 82(4), 1467–1495 (2019)
Piccoli, B.: Optimal syntheses for state constrained problems with application to optimization of cancer therapies. Math. Control Relat. Fields 2(4), 383 (2012)
Sethian, J.A.: Level Set Methods and Fast Marching Methods: Evolving Interfaces in Computational Geometry, Fluid Mechanics, Computer Vision, and Materials Science, vol. 3. Cambridge University Press, Cambridge (1999)
Soner, H.M.: Optimal control with state-space constraint i. SIAM J. Control. Optim. 24(3), 552–561 (1986)
Wang, Y., Yong, J.: A deterministic affine-quadratic optimal control problem. ESAIM Control Optim. Calc. Var. 20(3), 633–661 (2014)
Zhang, X., Liu, X.: Backward bifurcation of an epidemic model with saturated treatment function. J. Math. Anal. Appl. 348(1), 433–443 (2008)
Zhou, T., Zhang, W., Qiuying, L.: Bifurcation analysis of an SIS epidemic model with saturated incidence rate and saturated treatment function. Appl. Math. Comput. 226, 288–305 (2014)
Acknowledgements
The authors thank the anonymous reviewers for their helpful comments and suggestions.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflicts of interest
All authors declare that they have no conflicts of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Cacace, S., Lai, A.C. & Loreti, P. A dynamic programming approach for controlled fractional SIS models. Nonlinear Differ. Equ. Appl. 30, 20 (2023). https://doi.org/10.1007/s00030-022-00832-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00030-022-00832-w