Abstract
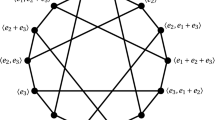
We use the Hopf fibration to explicitly compute generators of the second homotopy group of the flag manifolds of a compact Lie group. We show that these 2-spheres have nice geometrical properties such as being totally geodesic surfaces with respect to any invariant metric on the flag manifold, generalizing a result in Burstall and Rawnsley (Springer Lect. Notes Math. 2(84):1424, 1990). This illustrates how “rubber-band” topology can, in the presence of symmetry, single out very rigid objects. We characterize when these 2-spheres in the same homotopy class have the same geometry for all invariant metrics. This is done by exploring the action of Weyl group of the flag manifold, generalizing results of Patrão and San Martin (Indag. Math. 26:547–579, 2015) and de Siebenthal (Math. Helvetici 44(1):1–3, 1969). This illustrates how some aspects of “continuum” invariant geometry can, in the presence of symmetry, be reduced to the study of discrete objects. We remark that the topology singling out very rigid objects and the study of a continuum object being reduced to discrete ones is a characteristic of situations with a lot of symmetry and, thus, are recurring themes in Lie theory.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Besse, A.L.: Einstein Manifolds. Springer, Berlin (2008)
Black, M.: Harmonic maps into homogeneous spaces, Pitman Research Notes in Mathematics Series, 255, (1991)
Borel, A.: Kählerian coset spaces of semisimple Lie groups. Proc. Nat. Acad. Sci. USA 40, 1147–1151 (1954)
Brion, M.: Lectures on the geometry of flag varieties. arXiv:math/0410240
Burstall, F.E., Rawnsley, J.H.: Twistor Theory for Riemannian Symmetric Spaces. In: Lecture Notes in Mathematics, vol. 1424. Springer (1990)
Cohen, N., Grama, L., Negreiros, C.J.C.: Equigeodesics on flag manifolds. Houston J. Math. 37, 113–125 (2011)
Eells, J., Lemaire, L.: Selected Topics in Harmonic Maps, CBMS Regional Conference Series in Mathematics 50, AMS, (1983)
Gasparim, E., Grama, L., San Martin, L.A.B.: Adjoint orbits of semi-simple Lie groups and Lagrangean submanifolds. Proc. Edinburgh Math. Soc. 60, 361–385 (2017)
Durán, C., Mendoza, A., Rigas, A.: Blakers-Massey elements and exotic diffeomorphisms of \(S^6\) and \(S^{14}\) via geodesics. Trans. Am. Math. Soc. 356, 5025–5043 (2004)
Helgason, S.: Differential Geometry. Academic Press, Lie Groups and Symmetric Spaces, Cambridge (1978)
Hilgert, J., Neeb, K.: Structure and Geometry of Lie Groups. Springer, Berlin (2012)
Negreiros, C.J.C.: Some remarks about harmonic maps into flag manifolds. Indiana Univ. Math. J. 37(3), 617–636 (1988)
Patrão, M., San Martin, L.A.B.: The isotropy representation of a real flag manifold: split real forms. Indag. Math. 26, 547–579 (2015)
San Martin, L.A.B., Negreiros, C.J.C.: Invariant almost Hermitian structures on flag manifolds. Adv. Math. 178, 277–310 (2003)
de Siebenthal, J.: Sur certains modules dans une algèbre de Lie semi-simple. Comment. Math. Helvetici 44(1), 1–3 (1969)
Silva, J.L., Rabelo, L.: Half-shifted Young diagrams and homology of real Grassmannians. arXiv:1604.02177 (2016)
Wang, H.C.: Closed manifolds with homogeneous complex structures. Am. J. Math. 76, 1–32 (1954)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
L. Grama is partially supported by Grants 2018/13481-0 (FAPESP) and 305036/2019-0 (CNPq).
Rights and permissions
About this article
Cite this article
Grama, L., Seco, L. Second Homotopy Group and Invariant Geometry of Flag Manifolds. Results Math 75, 94 (2020). https://doi.org/10.1007/s00025-020-01213-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00025-020-01213-4