Preconditioned Inversion of 3D Borehole to Surface Electromagnetic for Reservoir Exploration

Abstract

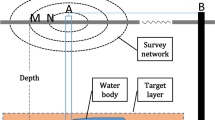
The accurate interpretation of three-dimensional borehole to surface electromagnetic (BSEM) data has two challenging problems: the accuracy of forward modeling and the efficiency of the inversion process. In this paper, we used the least squares method to develop a preconditioned algorithm combined parametric inversion and resistivity image to overcome these difficulties for reservoir exploration. To improve accuracy, we suggest using the full integral equation (IE) solution for forward modeling. Taking into account that the IE method requires the mesh discretization of an anomalous body only, the parametric inversion based on the IE method would provide an preconditioned inverse domain consisting of the complex structure via a few parameters. Based on the predicted preconditioned inverse domain, the conventional Green’s functions can be recomputed only once and kept unchanged in iterative inversion process of resistivity image, which increases significantly the size of the inverse domain and the computation for IE modeling. The resistivity of background and complex structures embedded in layered stratum are considered to simulate the practical application in preconditioned inversion. Synthetic examples with noise studies are conducted to demonstrate that the preconditioned algorithm can be applied quickly and effectively in a complex structure inversion for BSEM in reservoir exploration.

Appendix: Some Values of Partial Derivatives \(\frac{{\partial u_{{_{j} }} }}{{\partial \sigma_{i} }}\)

In the framework of IE method, taking into account that the model parameterization is to divide a model into blocks of unknown constant conduction, the Eq. (17) can also be written as

$$\frac{{\partial E(r_{{_{j} }}^{'} )}}{{\partial \sigma_{i} }} = \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{E}^{i} \cdot E^{a} (r_{i}^{{}} ) + \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{E} \cdot \Delta \sigma \cdot \frac{{\partial E^{a} (r)}}{{\partial \sigma_{i} }}$$

(21)

where

$$\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{E}^{i} = \left[ {\begin{array}{*{20}c} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{1i} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{1i} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{1i} } \\ \vdots & \vdots & \vdots \\ {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{Mi} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{Mi} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{Mi} } \\ \end{array} } \right],\;E^{a} (r_{i} ) = \left[ {\begin{array}{*{20}c} {E_{x}^{a} (r_{i} )} \\ {E_{y}^{a} (r_{i} )} \\ {E_{z}^{a} (r_{i} )} \\ \end{array} } \right]$$

$$\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{E} = \left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{11} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{11} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{11} } \\ \end{array} } & \ldots & \ldots & {\begin{array}{*{20}c} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{1N} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{1N} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{1N} } \\ \end{array} } \\ \vdots & \cdots & \cdots & \vdots \\ \vdots & \cdots & \cdots & \vdots \\ {\begin{array}{*{20}c} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{M1} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{M1} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{M1} } \\ \end{array} } & \cdots & \cdots & {\begin{array}{*{20}c} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{MN} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{MN} } & {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{MN} } \\ \end{array} } \\ \end{array} } \right]$$

$$\frac{{\partial E^{a} (r)}}{{\partial \sigma_{i} }} = \left[ {\begin{array}{*{20}c} {\frac{{\partial E_{x}^{a} (r_{1}^{{}} )}}{{\partial \sigma_{i} }}} & {\frac{{\partial E_{y}^{a} (r_{1}^{{}} )}}{{\partial \sigma_{i} }}} & {\frac{{\partial E_{z}^{a} (r_{1}^{{}} )}}{{\partial \sigma_{i} }}} & \cdots & {\frac{{\partial E_{x}^{a} (r_{N}^{{}} )}}{{\partial \sigma_{i} }}} & {\frac{{\partial E_{y}^{a} (r_{N}^{{}} )}}{{\partial \sigma_{i} }}} & {\frac{{\partial E_{z}^{a} (r_{N}^{{}} )}}{{\partial \sigma_{i} }}} \\ \end{array} } \right]^{T}$$

The Eq. (19) can be expressed as

$$\frac{{\partial E^{a} (r)}}{{\partial \sigma_{i} }} = - M^{ - 1} \cdot \frac{\partial M}{{\partial \sigma_{i} }} \cdot E^{a} (r)$$

(22)

where

$$M = \left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\Delta \sigma_{1} G_{xx}^{11} - 1} & {\Delta \sigma_{1} G_{xy}^{11} } & {\Delta \sigma_{1} G_{xz}^{11} } \\ {\Delta \sigma_{1} G_{yx}^{11} } & {\Delta \sigma_{1} G_{yy}^{11} - 1} & {\Delta \sigma_{1} G_{yz}^{11} } \\ {\Delta \sigma_{1} G_{zx}^{11} } & {\Delta \sigma_{1} G_{zy}^{11} } & {\Delta \sigma_{1} G_{zz}^{11} - 1} \\ \end{array} } & \cdots & {\begin{array}{*{20}c} {\Delta \sigma_{N} G_{xx}^{1N} } & {\Delta \sigma_{N} G_{xy}^{1N} } & {\Delta \sigma_{N} G_{xz}^{1N} } \\ {\Delta \sigma_{N} G_{yx}^{1N} } & {\Delta \sigma_{N} G_{yy}^{1N} } & {\Delta \sigma_{N} G_{yz}^{1N} } \\ {\Delta \sigma_{N} G_{zx}^{1N} } & {\Delta \sigma_{N} G_{zy}^{1N} } & {\Delta \sigma_{N} G_{zz}^{1N} } \\ \end{array} } \\ \vdots & \ddots & \vdots \\ {\begin{array}{*{20}c} {\Delta \sigma_{1} G_{xx}^{N1} } & {\Delta \sigma_{1} G_{xy}^{N1} } & {\Delta \sigma_{1} G_{xz}^{N1} } \\ {\Delta \sigma_{1} G_{yx}^{N1} } & {\Delta \sigma_{1} G_{yy}^{N1} } & {\Delta \sigma_{1} G_{yz}^{N1} } \\ {\Delta \sigma_{1} G_{zx}^{N1} } & {\Delta \sigma_{1} G_{zy}^{N1} } & {\Delta \sigma_{1} G_{zz}^{N1} } \\ \end{array} } & \cdots & {\begin{array}{*{20}c} {\Delta \sigma_{N} G_{xx}^{NN} - 1} & {\Delta \sigma_{N} G_{xy}^{NN} } & {\Delta \sigma_{N} G_{xz}^{NN} } \\ {\Delta \sigma_{N} G_{yx}^{NN} } & {\Delta \sigma_{N} G_{yy}^{NN} - 1} & {\Delta \sigma_{N} G_{yz}^{NN} } \\ {\Delta \sigma_{N} G_{zx}^{NN} } & {\Delta \sigma_{N} G_{zy}^{NN} } & {\Delta \sigma_{N} G_{zz}^{NN} - 1} \\ \end{array} } \\ \end{array} } \right]$$

$$E^{a} (r) = \left[ {\begin{array}{*{20}c} {E_{x}^{a} (r_{1}^{{}} )} & {E_{y}^{a} (r_{1}^{{}} )} & {E_{z}^{a} (r_{1}^{{}} )} & \cdots & {E_{x}^{a} (r_{N}^{{}} )} & {E_{y}^{a} (r_{N}^{{}} )} & {E_{z}^{a} (r_{N}^{{}} )} \\ \end{array} } \right]^{T}$$

where $E_{x}^{a} (r_{i}^{{}} )$, $E_{y}^{a} (r_{i}^{{}} )$ and $E_{z}^{a} (r_{i}^{{}} )$ are the three electrical field components of i th anomalous cell, which can be computed by solving the Eq. (3), the ‘T’ denotes the transposition of matrix, the $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{x}^{{}}$, $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{y}^{{}}$ and $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{G}_{z}^{{}}$ are the three components of the Green function correspond to receivers, and the $G_{xx}^{{}}$, $G_{xy}^{{}}$ and $G_{xz}^{{}}$ are the components of the Green function correspond to the receivers inside the anomalous body.