Abstract
In the first part of the paper, we propose a different viewpoint on the theory of higher Airy structures (or Airy ideals), which may shed light on its origin. We define Airy ideals in the \(\hbar \)-adic completion of the Rees Weyl algebra and show that Airy ideals are defined exactly such that they are always related to the canonical left ideal generated by derivatives by automorphisms of the Rees Weyl algebra of a simple type, which we call transvections. The standard existence and uniqueness result in the theory of Airy structures then follow immediately. In the second part of the paper, we construct Airy ideals generated by the nonnegative modes of the strong generators of the principal \({\mathcal {W}}\)-algebra of \(\mathfrak {sp}_{2N}\) at level \(-N-1/2\), following the approach developed in Borot et al. (Mem Am Math Soc, 2021). This provides an example of an Airy ideal in the Heisenberg algebra that requires realizing the zero modes as derivatives instead of variables, which leads to an interesting interpretation for the resulting partition function.
Similar content being viewed by others
Data Availability Statement
Data sharing was not applicable to this article as no datasets were generated or analyzed during the current study.
Notes
We could work over any field \({\mathbb {K}}\) of characteristic zero instead of \({\mathbb {C}}\).
This completion should not be confused with the \(\hbar \)-adic completion of Rees algebras and modules discussed in the previous section.
We will often abuse notation and still say that this ideal is “generated” by the \(P_i\), even though an ideal generated by a set only contains finite linear combination of the elements in the set, regardless of whether the set is finite or countably infinite. For us, we always include both finite and infinite linear combinations when the generating set is countably infinite.
For instance, we need to be careful that we don’t encounter situations like the differential operator \(\sum _{a \in A} \partial _a\) acting on \(\sum _{b \in A} x_b\), since \(\sum _{a \in A} \partial _a \left( \sum _{b \in B} x_b \right) = \sum _{a \in A} 1\) which is of course divergent. But, while \(\sum _{a \in A} \partial _a\) is in \({\mathcal {D}}_A\), \(\sum _{b \in A} x_b\) is not in \({\mathcal {M}}_A\) since it is not a polynomial. We never run into this kind of issues because all infinite sums collapse to finite sums, since all elements of \({\mathcal {M}}_A\) are polynomials, even if we work with infinitely many variables.
We note here that the argument of the exponential is not in \(\widehat{{\mathcal {M}}}_A^\hbar \), since the degree of the polynomials is two more than the power of \(\hbar \). But this is fine, as after acting with differential operators on Z using the standard action of differential operators, we get polynomials in \(\widehat{{\mathcal {M}}}_A^\hbar \) times Z, as stated.
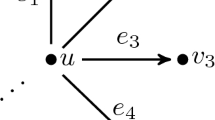
The name “transvection” comes from section 4 of [6], suitably generalized to completed Rees Weyl algebras.
As in footnote 5, we note here that the argument of the exponential is not in \(\widehat{{\mathcal {M}}}_A^\hbar \), but this is not a problem.
The use of homogeneous polynomials \(F_{g,n}(x_A)\) of degree n instead of the polynomials \(q^{(k+1)}(x_A)\) of degree \(\le k+1\) previously used in Lemma 2.25 is simply to connect with the existing literature on the topic, but it is straightforward to show that it is equivalent: the polynomials \(q^{(k+1)}(x_A)\) of degree \(\le k+1\) are reconstructed as \(q^{(k+1)}(x_A) = \sum _{\begin{array}{c} g \in \frac{1}{2} {\mathbb {N}}, n \in {\mathbb {N}}^* \\ 2\,g-1+n=k \end{array}} F_{g,n}(x_A) \).
To be precise, we would need to define the Rees Fock module slightly more generally here, since if we expand the exponential the polynomial coefficients will be 3 times the \(\hbar \)-degree, but this can be done easily without complication.
References
Andersen, J., Borot, G., Chekhov, L., Orantin, N.: The ABCD of topological recursion (2017). arXiv:1703.03307 [math-ph]
Arakawa, T.: Introduction to \(W\)-algebras and their representation theory. In: Callegaro, F., Novale, G.C., Caselli, F., de Concini, C., de Sole, A. (eds.) Perspectives in Lie Theory, pp. 179–250. Springer, Berlin (2017) . arXiv:1605.00138 [math.RT]
Atiyah, M., MacDonald, I.: Introduction to Commutative Algebra. Addison-Wesley-Longman, Edinburgh (1969)
Bakalov, B., Milanov, T.: \(W\)-constraints for the total descendant potential of a simple singularity. Compos. Math. 149(5), 840–888 (2013). arXiv:1203.3414 [math.QA]
Bakalov, B., Kac, V.: Twisted modules over lattice vertex algebras. arXiv:math/0402315
Belov-Kanel, A., Kontsevich, M.: Automorphisms of the Weyl algebra. Lett. Math. Phys. 74, 181–199 (2005). arXiv:math.RA/0512169
Borot, G., Bouchard, V., Chidambaram, N., Creutzig, T., Noshchenko, D.: Higher Airy structures, \(W\)-algebras and topological recursion. Mem. Am. Math, Soc (2021). arXiv:1812.08738 [math-ph]
Borot, G., Bouchard, V., Chidambaram, N., Creutzig, T.:. Whittaker vectors for \({\cal{W}}\)-algebras from topological recursion (2021). arXiv:2104.04516 [math-ph]
Borot, G., Kramer, R., Schüler, Y.: Higher Airy structures and topological recursion on singular spectral curves (2020). arXiv:2010.0351 [math-ph]
Bouchard, V., Cisomak, P., Hadasz, L., Osuga, K., Ruba, B., Sułkowski, P.: Super quantum Airy structures. Commun. Math. Phys. 380, 449–522 (2020). arXiv:1907.08913 [math-ph]
Bouchard, V., Eynard, B.: Think globally, compute locally. JHEP 02(143), 1–35 (2013). arXiv:1211.2302 [math-ph]
Bouchard, V., Eynard, B.: Reconstructing WKB from topological recursion. J. Éc. Polytech. Math. 4, 845–908 (2017). arXiv:1606.04498 [math-ph]
Bouchard, V., Hutchinson, J., Loliencar, P., Meiers, M., Rupert, M.: A generalized topological recursion for arbitrary ramification. Ann. Henri Poincaré 15(1), 143–169 (2014). arXiv:1208.6035 [math-ph]
Bouchard, V., Mastel, K.: A new class of higher quantum Airy structures as modules of \(W(\mathfrak{gl}_r)\)-algebras (2020). arXiv:2009.13047 [math-ph]
Chekhov, L., Eynard, B.: Matrix eigenvalue model: Feynman graph technique for all genera. JHEP 6(12), 026 (2006). arXiv:math-ph/0604014
Creutzig, T., Genra, N., Linshaw, A.: Category \({\cal{O}}\) for vertex algebras of \(\mathfrak{osp}_{1|2n}\). arXiv:2203.08188 [math.RT]
Creutzig, T., Linshaw, A.: Orbifolds of symplectic fermion algebras. Trans. Am. Math. Soc. 369(1), 467–494 (2017). arXiv:1404.2686 [math.RT]
Creutzig, T., Linshaw, A.: Trialities of orthosymplectic \(W\)-algebras (2021). arXiv:2102.10224 [math.RT]
Creutzig, T., Linshaw, A.: Cosets of affine vertex algebras inside larger structures. J. Algebra 517, 396–438 (2019). arXiv:1407.8512 [math.RT]
Eynard, B., Orantin, N.: Invariants of algebraic curves and topological expansion. Commun. Num. Theor. Phys. 1(2), 347–452 (2007). arXiv:math-ph/0702045
Eynard, B., Orantin, N.: Topological recursion in random matrices and enumerative geometry. J. Phys. A: Math. Theor. 42(29), 293001 (2009)
Frenkel, E., Ben-Zvi, D.: Vertex Algebras and Algebraic Curves. Mathematical Surveys and Monographs, vol. 88. American Mathematical Society, Providence, RI (2001)
Friedan, D., Martinec, E.J., Shenker, S.H.: Conformal invariance, supersymmetry and string theory. Nucl. Phys. B 271, 93–165 (1986)
Kersey, S.: Invertibility of submatrices of the Pascal matrix and Birkhoff interpolation. arXiv:1303.6159 [math.NA]
Kontsevich, M., Soibelman, Y.: Airy structures and symplectic geometry of topological recursion. In: Topological Recursion and Its Influence in Analysis, Geometry, and Topology, volume 100 of Proceedings of Symposia in Pure Mathematics, pp. 433–490. AMS (2018). arXiv:1701.09137 [math.AG]
Li, H.: Vertex algebras and vertex Poisson algebras. Commun. Contemp. Math. 6(1), 61–110 (2004). arXiv:math.QA/0209310
Milanov, T.: \(W\)-algebra constraints and topological recursion for \(A_N\)-singularity. Int. J. Math. 27, 1650110 (2016). with an Appendix by Danilo Lewanski, arXiv:1603.00073 [math-ph]
Acknowledgements
We would like to thank Raphaël Belliard and Reinier Kramer for interesting discussions. The authors acknowledge the support of the Natural Sciences and Engineering Research Council of Canada.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors have no competing interests to declare that are relevant to the content of this article.
Additional information
Communicated by Katrin Wendland.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Bouchard, V., Creutzig, T. & Joshi, A. Airy Ideals, Transvections, and \({\mathcal {W}}(\mathfrak {sp}_{2N})\)-Algebras. Ann. Henri Poincaré 25, 2669–2730 (2024). https://doi.org/10.1007/s00023-023-01374-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00023-023-01374-2