Skip to main content
SpringerLink
Log in

Diffraction for the Dirac–Coulomb Propagator

  • Original Paper
  • Published:
Annales Henri Poincaré Aims and scope Submit manuscript

Abstract

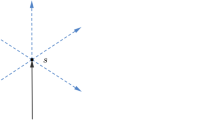
The Dirac equation in \(\mathbb {R}^{1,3}\) with potential \({\textsf{Z}}/r\) is a relativistic field equation modeling the hydrogen atom. We analyze the singularity structure of the propagator for this equation, showing that the singularities of the Schwartz kernel of the propagator are along an expanding spherical wave away from rays that miss the potential singularity at the origin, but also may include an additional spherical wave of diffracted singularities emanating from the origin. This diffracted wavefront is \(1-{\epsilon }\) derivatives smoother than the main singularities, for all \({\epsilon }>0,\) and is a conormal singularity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

Notes

  1. Readers consulting other references should be aware that there are at least two conventions in the literature. Indeed, many physics texts (e.g., Akhiezer and Berestetsky [1] and Rose [37]) ask that the gamma matrices satisfy a Riemannian anticommutation relation and then set \(x_{0} = ict\).

  2. More generally, we remark that we can replace the smooth term by a term that is smooth on the blowup of the origin with no change in the arguments of this section.

  3. Recall that \({\textrm{b}}\)-Sobolev spaces are by default defined with respect to the b-density rather than the metric density.

  4. We assume our quantization is arranged so that it yields properly supported operators.

References

  1. Akhiezer, A.I., Berestetskii, V.B.: Quantum Electrodynamics, Authorized English Edition Revised and Enlarged by the Authors: Translated from the Second Russian Edition by G. M. Volkoff. Interscience Monographs and Texts in Physics and Astronomy, Vol. XI, Interscience Publishers Wiley, New York-London-Sydney (1965)

  2. Baskin, D., Marzuola, J.L.: The radiation field on product cones (2019)

  3. Baskin, D., Vasy, A., Wunsch, J.: Asymptotics of radiation fields in asymptotically Minkowski space. Am. J. Math. 137(5), 1293–1364 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  4. Baskin, D., Vasy, A., Wunsch, J.: Asymptotics of scalar waves on long-range asymptotically Minkowski spaces. Adv. Math. 328, 160–216 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  5. Boussaid, N., Golénia, S.: Limiting absorption principle for some long range perturbations of Dirac systems at threshold energies. Commun. Math. Phys. 299(3), 677–708 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  6. Boussaid, N., D’Ancona, P., Fanelli, L.: Virial identity and weak dispersion for the magnetic Dirac equation. J. Math. Pures Appl. 95(2), 137–150 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  7. Cacciafesta, Federico, D’Ancona, P.: Endpoint estimates and global existence for the nonlinear Dirac equation with potential. J. Differ. Equ. 254(5), 2233–2260 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  8. Cacciafesta, F., Séré, É.: Local smoothing estimates for the massless Dirac-Coulomb equation in 2 and 3 dimensions. J. Funct. Anal. 271(8), 2339–2358 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  9. Cheeger, J., Taylor, M.: On the diffraction of waves by conical singularities. II. Commun. Pure Appl. Math. 35(4), 487–529 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  10. Cheeger, Jeff, Taylor, M.: On the diffraction of waves by conical singularities. I. Commun. Pure Appl. Math. 35(3), 275–331 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  11. D’Ancona, P., Fanelli, L.: Decay estimates for the wave and Dirac equations with a magnetic potential. Commun. Pure Appl. Math. 60(3), 357–392 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  12. Darwin, C.G.: The wave equations of the electron. Proc. R. Soc. Lond. Ser. A Contain. Pap. Math. Phys. Character 118(780), 654–680 (1928)

    ADS  MATH  Google Scholar 

  13. Duistermaat, J.J., Hörmander, L.: Fourier integral operators. II. Acta Math. 128, 183–269 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  14. Erdoğan, M.B., Green, W.R., Toprak, E.: Dispersive estimates for Dirac operators in dimension three with obstructions at threshold energies. Am. J. Math. 141(5), 1217–1258 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  15. Ford, G.A., Hassell, A., Hillairet, L.: Wave propagation on Euclidean surfaces with conical singularities. I: geometric diffraction. J. Spectr. Theory 8(2), 605–667 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  16. Friedlander, F.G.: Sound Pulses. Cambridge University Press, New York (1958)

    MATH  Google Scholar 

  17. Gérard, C., Wrochna, M.: Construction of Hadamard states by pseudo-differential calculus. Comm. Math. Phys. 325(2), 713–755 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  18. Gil, J.B., Mendoza, G.A.: Adjoints of elliptic cone operators. Am. J. Math. 125(2), 357–408 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  19. Grieser, D.: Basics of the \(b\)-calculus, Approaches to singular analysis (Berlin, 1999). Oper. Theory Adv. Appl. 125, 30–84 (2001)

    MATH  Google Scholar 

  20. Hintz, P., Vasy, A.: The global non-linear stability of the Kerr-de Sitter family of black holes. Acta Math. 220(1), 1–206 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  21. Hörmander, L.: The Analysis of Linear Partial Differential Operators. IV, Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], Vol 275. Springer-Verlag, Berlin (1994). Fourier integral operators, Corrected reprint of the 1985 original

  22. Hörmander, L.: The Analysis of Linear Partial Differential Operators. III, Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], Vol 274. Springer-Verlag, Berlin (1985). Pseudodifferential operators

  23. Kato, T.: Perturbation Theory for Linear Operators, Die Grundlehren der Mathematischen Wissenschaften, vol. 132. Springer-Verlag New York, Inc., New York (1966)

    Book  Google Scholar 

  24. Keller, J.B.: One hundred years of diffraction theory. IEEE Trans. Antennas Propag. 33(2), 123–126 (1985)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  25. Lax, P.D., Phillips, R.S.: Scattering Theory. Academic Press, New York (1989)

    MATH  Google Scholar 

  26. Lesch, M.: Operators of Fuchs type, conical singularities, and asymptotic methods, Teubner-Texte zur Mathematik [Teubner Texts in Mathematics], B. G. Teubner Verlagsgesellschaft mbH, Stuttgart 136 (1997)

  27. Mazzeo, R.: Elliptic theory of differential edge operators. I. Commun. Partial Differ. Equ. 16(10), 1615–1664 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  28. Melrose, I., Richard B.: Spectral and scattering theory for the Laplacian on asymptotically Euclidian spaces, Spectral and scattering theory (sanda, 1992), Dekker, New York, pp 85– 130 (1994)

  29. Melrose, R., Vasy, A., Wunsch, J.: Diffraction of Singularities for the Wave Equation on Manifolds with Corners, Astérisque, Vol 351, pp vi+135 (2013)

  30. Melrose, R.B.: Microlocal parametrices for diffractive boundary value problems. Duke Math. J. 42, 605–635 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  31. Melrose, R.B.: The Atiyah-Patodi-Singer iNdex Theorem. AK Peters, Wellesley (1993)

    Book  MATH  Google Scholar 

  32. Melrose, R.B., Sjöstrand, J.: Singularities in boundary value problems I. Commun. Pure Appl. Math. 31, 593–617 (1978)

    Article  MATH  Google Scholar 

  33. Melrose, R.B., Sjöstrand, J.: Singularities in boundary value problems II. Commun. Pure Appl. Math. 35, 129–168 (1982)

    Article  MATH  Google Scholar 

  34. Melrose, R., Wunsch, J.: Propagation of singularities for the wave equation on conic manifolds. Invent. Math. 156(2), 235–299 (2004)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  35. Melrose, R., András, V., Jared, W.: Propagation of singularities for the wave equation on edge manifolds. Duke Math. J. 144(1), 109–193 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  36. Qian, R.: Diffractive Theorems for the Wave Equation with Inverse Square Potential, 2009. Northwestern University Ph.D. thesis (2009)

  37. Rose, Morris Edgar: Relativistic Electron Theory. Wiley, New Jersey (1961)

    MATH  Google Scholar 

  38. Sommerfeld, A.: Mathematische theorie der diffraktion. Math. Ann. 47, 317–374 (1896)

    Article  MathSciNet  MATH  Google Scholar 

  39. Szmytkowski, R.: Recurrence and differential relations for spherical spinors. J. Math. Chem. 42(3), 397–413 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  40. Tang, S.-H., Zworski, M.: Resonance expansions of scattered waves. Commun. Pure Appl. Math. 53(10), 1305–1334 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  41. Taylor, M.E.: Grazing rays and reflection of singularities to wave equations. Commun. Pure Appl. Math. 29, 1–38 (1978)

    Article  ADS  MathSciNet  Google Scholar 

  42. Vainberg, B.: Asymptotic Methods in Equations of Mathematical Physics. Gordon and Breach, New York (1988)

    Google Scholar 

  43. Vasy, A.: Propagation of singularities for the wave equation on manifolds with corners. Ann. Math. 168(3), 749–812 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  44. Vasy, A.: Microlocal analysis of asymptotically hyperbolic and Kerr-de Sitter spaces (with an appendix by Semyon Dyatlov). Invent. Math. 194(2), 381–513 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  45. Weidmann, J.: Oszillationsmethoden für systeme gewöhnlicher differentialgleichungen. Math. Z. 119, 349–373 (1971)

    Article  MathSciNet  MATH  Google Scholar 

  46. Yang, M.: Propagation of polyhomogeneity, diffraction, and scattering on product cones. J. Spectr. Theory 12(2), 381–415 (2022). (MR4487478)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Texas A & M University, College Station, United States

    Dean Baskin

  2. Northwestern University, Evanston, United States

    Jared Wunsch

Authors
  1. Dean Baskin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jared Wunsch
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jared Wunsch.

Additional information

Communicated by Jan Derezinski.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The authors are grateful to Christian Gérard and Michał Wrochna for suggesting the problem and providing helpful insight into its importance, as well as for helpful comments on an early version of the manuscript. They are also grateful to Richard Melrose, András Vasy, and especially Oran Gannot for many helpful conversations. Two anonymous referees made a number of corrections and suggestions that improved the presentation. The research for this paper began during a Research in Paris stay at the Institut Henri Poincaré. Part of this material is based upon work supported by the National Science Foundation under Grant No. DMS-1440140 while the authors were in residence at the Mathematical Sciences Research Institute in Berkeley, California, during the Fall 2019 semester. DB was supported by NSF CAREER grant DMS-1654056. JW was supported by NSF grant DMS-1600023 and Simons Foundation Grant 631302.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Baskin, D., Wunsch, J. Diffraction for the Dirac–Coulomb Propagator. Ann. Henri Poincaré 24, 2607–2659 (2023). https://doi.org/10.1007/s00023-023-01279-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00023-023-01279-0

Search

Navigation