Computer-Assisted Proof of Loss of Ergodicity by Symmetry Breaking in Expanding Coupled Maps

Abstract

From a dynamical viewpoint, basic phase transitions of statistical mechanics can be regarded as a loss of ergodicity. While many random interacting particle models exhibiting such transitions at the thermodynamics limit exist, finite-dimensional examples with deterministic dynamics on a chaotic attractor are rare, if at all existent. Here, the dynamics of a family of N coupled expanding circle maps is investigated in a parameter regime where absolutely continuous invariant measures are known to exist. At first, empirical evidence is given of symmetry breaking of the ergodic components upon increase in the coupling strength, suggesting that loss of ergodicity should occur for every integer $N>2$. Then, a numerical algorithm is proposed which aims to rigorously construct asymmetric ergodic components of positive Lebesgue measure. Due to the explosive growth of the required computational resources, the algorithm successfully terminates for small values of N only. However, this approach shows that phase transitions should be provable for systems of arbitrary number of particles with erratic dynamics, in a purely deterministic setting, without any reference to random processes.

Appendices

Appendix A: Symmetry Breaking for $G_{2,\epsilon }$

The map $G_{2,\epsilon }$’s explicitly expression is given by $G_{2,\epsilon }=2(1-\epsilon )\text {Id}_{\mathbb {T}^{2}}+\tfrac{2\epsilon }{3}B_2 \text {mod}\ 1$, where

$$\begin{aligned} \left\{ \begin{array}{l} (B_{2}(x))_1=2h(x_1)-h(x_2)+h(x_1+x_2)\\ (B_{2}(x))_2=2h(x_2)-h(x_1)+h(x_1+x_2) \end{array}\right. \end{aligned}$$

$G_{2,\epsilon }$ commutes with each transformation in the natural representation of $\mathbb {Z}_2\times S_3$ on $\mathbb {T}^2$. This group can be generated by the sign flip $-\text {Id}_{\mathbb {T}^2}$ and the transformations $\sigma _{213},\ \sigma _{132}$ and $\sigma _{321}$, which are induced by the transpositions in $S_3$. (NB: subscripts here denote transposition images of the ordered list $\{1,2,3\}$ - abbreviated as 123.) The sign flip writes

$$\begin{aligned} - \text {Id}x = (-x_1,-x_2)\ (\text {mod}\ 1), \end{aligned}$$

and it corresponds to the central symmetry wrt $(\frac{1}{2},\frac{1}{2})$ in the square $(0,1)^2$. Similarly, we have

$$\begin{aligned} \sigma _{321} x=(-x_2,-x_1)\ (\text {mod}\ 1), \end{aligned}$$

which corresponds to the orthogonal reflection wrt to the anti-diagonal $x_1+x_2=1$. Moreover, $\sigma _{213}$ (resp. $\sigma _{132}$) is defined by

$$\begin{aligned} \sigma _{213} x=(-x_1,x_1+x_2)\ (\text {mod}\ 1)\quad \left( \text {resp.}\ \sigma _{132} x=(x_1+x_2,-x_2)\ (\text {mod}\ 1)\right) , \end{aligned}$$

which is the reflection wrt the axis $x_1=0$ along the direction $x_1+2x_2=\text {cst}$ (resp. wrt the axis $x_2=0$ along the direction $2x_1+x_2=\text {cst}$).

The representation on $\mathbb {T}^2$ of $\mathbb {Z}_2\times S_3$ itself can be listed as follows:

$$\begin{aligned} \{&\text {Id},-\text {Id},\ \sigma _{213},\ \sigma _{132},\ \sigma _{321},\ \sigma _{213}\circ \sigma _{321},\ \sigma _{321}\circ \sigma _{213},\ -\sigma _{213},\ -\sigma _{132},\ -\sigma _{321},\\&-\sigma _{213}\circ \sigma _{321},\ -\sigma _{321}\circ \sigma _{213}\}. \end{aligned}$$

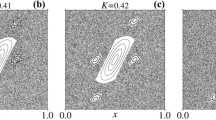
Clearly, all ergodic components for $\epsilon >\epsilon _2$ break the sign-flip symmetry (Fig. 1). More precisely, each component remains invariant under the action of one transformation above and is mapped onto another component under any other transformation. In particular, the chartreuse and dark green components are invariant under $\sigma _{213}$, blue and light green components are invariant under $\sigma _{132}$ and red and orange components are invariant under $\sigma _{321}$.

Appendix B: Symmetry Breaking for $G_{3,\epsilon }$

The map $G_{3,\epsilon }$ writes $G_{3,\epsilon }=2(1-\epsilon )\text {Id}_{\mathbb {T}^{3}}+\tfrac{\epsilon }{2}B_3\ \text {mod}\ 1$, where

$$\begin{aligned} \left\{ \begin{array}{l} (B_{3}(x))_1=2h(x_1)-h(x_2)+h(x_1+x_2)-h(x_2+x_3)+h(x_1+x_2+x_3)\\ (B_{3}(x))_2=2h(x_2)-h(x_1)-h(x_3)+h(x_1+x_2)+h(x_2+x_3)\\ (B_{3}(x))_2=2h(x_3)-h(x_2)-h(x_1+x_2)+h(x_2+x_3)+h(x_1+x_2+x_3) \end{array}\right. \end{aligned}$$

As before, any transformation in the representation of $S_4$ on $\mathbb {T}^3$ can be obtained as a composition of transpositions, whose expressions are given by (NB: the $(\text {mod}\ 1)$ are not specified for the sake of space):

$$\begin{aligned}&\sigma _{2134} x=(-x_1,x_1+x_2,x_3),&\sigma _{3214} x=(-x_2,-x_1,x_1+x_2+x_3)\\&\sigma _{4231} x=(-x_2-x_3,x_2,-x_1-x_2),&\sigma _{1324} x=(x_1+x_2,-x_2,x_2+x_3)\\&\sigma _{1432} x=(x_1+x_2+x_3,-x_3,-x_2),&\sigma _{1243} x=(x_1,x_2+x_3,-x_3) \end{aligned}$$

The ergodic components that emerge at $\epsilon =\epsilon _3$ all break the sign-flip symmetry $-\text {Id}$ in $\mathbb {T}^3$. As before, these six components are only partly asymmetric; they remain invariant under the action of seven transformations in $\mathbb {Z}_2\times S_4$, and they are exchanged under other transformations. In particular, the blue component is invariant under

$$\begin{aligned}&\sigma _{3214},\ \sigma _{1432},\ -\sigma _{1243}\circ \sigma _{2134},\ -\sigma _{1324}\circ \sigma _{4231},\ -\sigma _{1324}\circ \sigma _{1432}\circ \sigma _{2134}\\&\text {and}\ -\sigma _{1243}\circ \sigma _{4231}\circ \sigma _{2134}, \end{aligned}$$

and, obviously, under the composition $\sigma _{3214}\circ \sigma _{1432}=\sigma _{1432}\circ \sigma _{3214}$.

As for the eight components emerging at $\epsilon \simeq 0.437$, their asymmetries are stronger than for the previous components, as they remain invariant under only five transformations. For instance, the fuschia component is invariant under

$$\begin{aligned} \sigma _{2134},\ \sigma _{4231},\ \sigma _{1432}, \end{aligned}$$

and, obviously, under the compositions $\sigma _{4231}\circ \sigma _{2134}=\sigma _{2134}\circ \sigma _{4231}=\sigma _{2134}\circ \sigma _{1432}=\sigma _{1432}\circ \sigma _{4231}$ and $\sigma _{1432}\circ \sigma _{2134}=\sigma _{4231}\circ \sigma _{1432}$.

Appendix C: Proof of Lemma 3

We first establish the expression of $\overline{O(m)}_i$. A similar analysis yields the expression of $\underline{O(m)}_i$. Let $i\in \{1,\dots ,E\}$ and suppose that we aim to maximize the quantity $\sum _{j=1}^D\alpha _{ij}x_j$ given the 2E linear inequality constraints:

$$\begin{aligned} \sum _{j=1}^D\alpha _{kj}x_j\le {\overline{m}}_{k}\quad \text {and}\quad -\sum _{j=1}^D\alpha _{kj}x_j\le -\underline{m}_{k}, \quad 1\le k\le E. \end{aligned}$$

(C.1)

This problem exactly fits the KKT approach to linear programming [5]. The corresponding KKT conditions state in particular that every maximizer $(x_j^*)_{j=1}^D$ must cancel the gradient of the following Lagrangian:

$$\begin{aligned} \sum _{j=1}^D\alpha _{ij}x_j-\sum _{k=1}^{E}\gamma _k\left( \sum _{j=1}^D\alpha _{kj}x_j-{\overline{m}}_{k}\right) +\sum _{k=1}^{E}\gamma '_k\left( \sum _{j=1}^D\alpha _{kj}x_j-\underline{m}_{k}\right) , \end{aligned}$$

for a unique pair of vectors $(\gamma _k)_{k=1}^{E},(\gamma '_k)_{k=1}^{E}$ with nonnegative components. In other words, this pair of vectors must satisfy the equation:

$$\begin{aligned} \sum _{k=1}^{E}(\gamma _k-\gamma '_k)\alpha _{kj}=\alpha _{ij},\ 1\le j\le D. \end{aligned}$$

(C.2)

The complementary slackness conditions in the KKT setting then state that we must also have

$$\begin{aligned} \gamma _k\left( \sum _{j=1}^D\alpha _{kj}x_j^*-{\overline{m}}_{k}\right) =0\ \text {and}\ \gamma '_k\left( \sum _{j=1}^D\alpha _{kj}x_j^*-\underline{m}_{k}\right) =0,\ 1\le k\le E. \nonumber \\ \end{aligned}$$

(C.3)

Now, the conditions $\underline{m}_{k}<{\overline{m}}_{k}$ imply that the constraints $\sum _{j=1}^D\alpha _{kj}x_j^*\le {\overline{m}}_{k}$ and $-\sum _{j=1}^D\alpha _{kj}x_j^*\le -\underline{m}_{k}$ cannot be simultaneously active, viz. we must have $\gamma _k\gamma '_k=0$ for all k. A single multiplier $\lambda _k=\gamma _k-\gamma '_k$ results for each k, and equation (C.2) implies that the vector $(\lambda _k)_{k=1}^{E}$ must solve equation (4.2).

Equation (C.3) also implies that for $\lambda _k\ne 0$, we have

$$\begin{aligned} \sum _{j=1}^D\alpha _{kj}x_j^*=\left\{ \begin{array}{ccl} {\overline{m}}_k&{}\text {if}&{}\lambda _k=\gamma _k> 0\\ \underline{m}_k&{}\text {if}&{}\lambda _k=-\gamma '_k<0 \end{array}\right. ={\overline{e}}_{k}(\lambda _k,m). \end{aligned}$$

Together with Eq. (4.2), this equality yields

$$\begin{aligned} \sum _{j=1}^D\alpha _{ij}x_j^*=\sum _{k=1}^{E}\lambda _k\sum _{j=1}^D\alpha _{kj}x_j^*=\sum _{k=1}^{E}\lambda _k{\overline{e}}_{k}(\lambda _k,m), \end{aligned}$$

which is exactly the expression involved in the definition of $\overline{O(m)}_i$.

When $E>D$, the system (4.2) is underdetermined. Hence, it may have infinitely many solutions. The following considerations show that we may only retain solutions in $\Lambda _i$.

Consider the partition of $\mathbb {R}^{E}$ into orthants in the interior of which the sign of every coordinate $\lambda _k$ is constant. Choose any orthant that contains a family of (infinitely many) solutions to (4.2) in its interior.¹⁵ Those coordinates $\lambda _k$ with identical sign ($E>2$) must have opposite variations when varying the solution in the family. However, the functional $(\lambda _k)_{k=1}^{E}\mapsto \sum _{k=1}^{E}\lambda _{k}{\overline{e}}_k(\lambda _k,m)$ is linear (and hence with constant gradient) inside the orthant. Therefore, it certainly reaches its maximum on the boundary of the orthant, i.e., when at least one coordinate of $(\lambda _k)_{k=1}^{E}$ vanishes.

Repeating the reasoning inside orthant boundaries subspaces $\mathbb {R}^{E-1}$, and then for all subsequent subspaces $\mathbb {R}^{E'}$ for decreasing $E'$, from $E-2$ to $D+1$, as long as the resulting systems remain underdetermined, this shows that one may only consider solutions of (4.2) with $E-D$ vanishing coordinates.

Yet, the system with D unknowns/equations may still be degenerate. Then, one removes one superfluous equation from (4.2) and repeats the argument to conclude that the maximum must occur for a vector with $E-D+1$ vanishing coordinates. Repeating this reasoning as long as a degeneracy occurs, we may eventually get a system of two unknowns/equations. This system may be problematic if degenerate and the two coordinates have opposite signs. In fact, the only problematic scenario is if the functional $\sum _{k=1}^{E}\lambda _{k}\underline{e}_k(\lambda _k,m)$ increases when the positive coordinates increases. (Otherwise, the maximum is reached when one coordinate vanishes.) In this case, the maximum would be $+\infty$, which is certainly impossible. This analysis concludes that $\overline{O(m)}_i$ maximizes the quantity $\sum _{j=1}^D\alpha _{ij}x_j$, under the constraints (C.1).

As a consequence, if $P_m^\alpha \ne \emptyset$, then for every $x\in \overline{P_m^\alpha }$ (the closure of $P_m^\alpha$), we must have

$$\begin{aligned} \underline{O(m)}_i\le \sum _{j=1}^D\alpha _{ij}x_j\le \overline{O(m)}_i,\ 1\le i\le E, \end{aligned}$$

viz. $\overline{P_m^\alpha }\subset \overline{P_{O(m)}^\alpha }$ (and then $P_{O(m)}^\alpha \ne \emptyset$). On the other hand, that the canonical vector $(\delta _{k,i})_{k=1}^{E}$ belongs to $\Lambda _i$ implies

$$\begin{aligned} \underline{m}_i\le \underline{O(m)}_i\quad \text {and}\quad \ \overline{O(m)}_i\le {\overline{m}}_i,\ 1\le i\le E, \end{aligned}$$

hence $\overline{P_{O(m)}^\alpha }\subset \overline{P_m^\alpha }$. It results that $P_{O(m)}^\alpha =P_m^\alpha$ when $P_m^\alpha \ne \emptyset$.

Now, the complementary slackness conditions (C.3) imply that the maximum $\overline{O(m)}_i$ and minimum $\underline{O(m)}_i$ are given by combinations of values at active constraints. Besides, we must have $\overline{O(m)}_i={\overline{m}}_i$ and $\underline{O(m)}_i=\underline{m}_i$ when the constraints at i are active, by the definitions of $\overline{O(m)}_i$ and $\underline{O(m)}_i$. It follows that the values of $\overline{O(m)}_i$ and $\underline{O(m)}_i$ do not change when we replace m by O(m) in the constraints (C.1) (viz. O is a projection operator), and the complementary slackness conditions imply that all constraints must be active in the updated optimization problem, i.e., all constraints in the definition of $P_{O(m)}^\alpha$ must be active.

Moreover, that $\overline{O(m)}_i$ and $\underline{O(m)}_i$ are coordinate extremal values in $P_{O(m)}^\alpha =P_m^\alpha$ immediately imply that

$$\begin{aligned} \underline{O(m)}_i< \overline{O(m)}_i,\ 1\le i\le E \end{aligned}$$

is an existence condition for $P_m^\alpha$.

Proof of item (iii). If a maximizer $(x_j^*)_{j=1}^D$ satisfies

$$\begin{aligned} \sum _{j=1}^D\alpha _{ij}x_j^*=\overline{O(m)}_i<\min _{(\lambda _k)\in \Lambda _i\atop (\lambda _k)\ne (\delta _{k,i})}\sum _{k=1}^{E}\lambda _k{\overline{e}}_k(\lambda _k,m), \end{aligned}$$

then, by continuity, the inequality

$$\begin{aligned} \sum _{j=1}^D\alpha _{ij}x_j<\min _{(\lambda _k)\in \Lambda _i\atop (\lambda _k)\ne (\delta _{k,i})}\sum _{k=1}^{E}\lambda _k{\overline{e}}_k(\lambda _k,m) \end{aligned}$$

holds for all points in the intersection of the plane $\sum _{j=1}^D\alpha _{ij}x_j=\overline{O(m)}_i$ with a sufficiently small neighborhood of $(x_j^*)_{j=1}^D$; hence, this plane defines a facet of $P_m^\alpha$. On the opposite, if

$$\begin{aligned} \overline{O(m)}_i=\min _{(\lambda _k)\in \Lambda _i\atop (\lambda _k)\ne (\delta _{k,i})}\sum _{k=1}^{E}\lambda _k{\overline{e}}_k(\lambda _k,m), \end{aligned}$$

then let $\delta =(\delta _i)_{i=1}^D$ be a perturbation of the maximizer $x^*$ in the plane $\sum _{j=1}^D\alpha _{ij}x_j=\overline{O(m)}_i$, i.e., such that $\sum _{j=1}^D\alpha _{ij}\delta _j=0$. Then for those $(\lambda _k)$ for which the previous equality holds, we must also have

$$\begin{aligned} \sum _{k=1}^{E}\lambda _k\sum _{j=1}^D\alpha _{kj}\delta _j=0\quad \Rightarrow \quad \sum _{j=1}^D\alpha _{kj}\delta _j=0,\ \forall k\ :\ \lambda _k\ne 0. \end{aligned}$$

Since the vectors $(\alpha _{kj})_{j=1^D}$ are all distinct in $\mathbb {R}\mathbb {P}^D$, this means that $\delta$ must be limited to a set of co-dimension $1+\#\{k\ :\ \lambda _k\ne 0\}>1$. Therefore, it can certainly not span a facet of $P_m^\alpha$. The proof of Lemma C is complete. $\square$

Appendix D: Mathematical Statements Related to Polytope Chopping Tests

The setting in this section assumes that two polytopes $P_m^\alpha$ and $P_{m'}^\alpha$ (where the constraint vectors m and $m'$ are assumed to be optimized) intersect but are not contained in one another. We aim to determine (simple) conditions so that $P_m^\alpha {\setminus } P_{m\cap m'}^\alpha$ consists of either one or two polytopes defined by inequality constraints of the same type. We shall rely on the following set of indices:

$$\begin{aligned} S=\left\{ 1\le i\le E:\underline{m}_i<\underline{m'}_i\ \text {and/or}\ \overline{m'}_i<{\overline{m}}_i\right\} . \end{aligned}$$

Claim 4

In the current setting, assume that a following instance occurs:¹⁶

• a unique $i^-$ exists such that $\underline{m}_{i^-}<\underline{m'}_{i^-}$ and $\underline{O(m\cap m')}_{i^-}=\underline{m'}_{i^-}$

• a unique $i^+$ exists such that $\overline{m'}_{i^+}<{\overline{m}}_{i^+}$ and $\overline{O(m\cap m')}_{i^+}=\overline{m'}_{i^+}$.

Then, the intersection $P_{m\cap m'}^\alpha :=P_m^\alpha \cap P_{m'}^\alpha$ can be characterized as follows:

$$\begin{aligned} \left( \underline{(m\cap m')}_i,\overline{(m\cap m')}_i\right) =\left\{ \begin{array}{ccl} (\underline{m}_i,{\overline{m}}_i)&{}\text {if}&{}i\not \in S\ \text {or}\ i\ne i^-,i^+\\ (\underline{m'}_{i^-},{\overline{m}}_{i^-})&{}\text {if}&{}i=i^-\ne i^+\\ (\underline{m}_{i^+},\overline{m'}_{i^+})&{}\text {if}&{}i=i^+\ne i^-\\ (\underline{m'}_i,\overline{m'}_i)&{}\text {if}&{}i=i^-=i^+ \end{array}\right. \end{aligned}$$

The algorithm relies on the following consequence to define complementary piece(s) when an index of type $i^-$ or $i^+$ appears in testing intersections.

Corollary 5

If $i^-$ exists, let the constraint vector $m^-$ be defined by

$$\begin{aligned} (\underline{m^-}_i,\overline{m^-}_i)=\left\{ \begin{array}{ccl} (\underline{m}_i,{\overline{m}}_i)&{}\text {if}&{}i\ne i^-\\ (\underline{m}_{i^-},\underline{m'}_{i^-})&{}\text {if}&{}i=i^-\end{array}\right. \end{aligned}$$

and similarly, let $m^+$ be defined by

$$\begin{aligned} (\underline{m^+}_i,\overline{m^+}_i)=\left\{ \begin{array}{ccl} (\underline{m}_i,{\overline{m}}_i)&{}\text {if}&{}i\ne i^+\\ (\overline{m'}_{i^+},{\overline{m}}_{i^+})&{}\text {if}&{}i=i^+\end{array}\right. \end{aligned}$$

when assuming $i^+$ exists. We have

$$\begin{aligned} P_m^\alpha =P_{m^-}^\alpha \cup P_{m\cap m'}^\alpha \cup P_{m^+}^\alpha \ \text {mod}\ 0 \end{aligned}$$

where $P_{m^-}^\alpha$ (resp. $P_{m^+}^\alpha$) has to be replaced by $\emptyset$ if $i^-$ (resp. $i^+$) does not exist.

The proof of the Corollary is immediate. The definitions of $m^-$ and $m^+$ immediately follow from the characterizations of $m\cap m'$ and $i^-,i^+$. That the corresponding polytopes are non-empty is a consequence of the fact that m and $m'$ are optimized (so that there exists $x^-\in P_{m^-}^\alpha$ such that $x_{i^-}^-=\underline{m}_{i^-}$ and $x^+\in P_{m^+}^\alpha$ such that $x_{i^+}^+=\underline{m}_{i^+}$).

The Claim remains valid if one considers the semi-optimization procedure $O'$ (obtained by replacing the $\Lambda _i$ by $\Lambda '_i$). However, $P_{m^-}^\alpha \ne \emptyset$ and $P_{m^+}^\alpha \ne \emptyset$ can no longer be granted. So in using $O'$ instead of O when testing chopping, the algorithm may include spurious polytopes in the constructing collection.

Proof of the Claim. Consider all possible cases of ordering of the coordinates of m and $m'$ under the assumption $m\cap m'\ne \emptyset$. Then, from the definition of $m\cap m'$ in Sect. 4.2.2, we must have

$$\begin{aligned} \left( \underline{(m\cap m')}_i,\overline{(m\cap m')}_i\right) =\left\{ \begin{array}{ccl} (\underline{m}_i,{\overline{m}}_i)&{}\text {if}&{}i\not \in S\\ (\underline{m'}_{i},{\overline{m}}_{i})&{}\text {if}&{}\underline{m}_i<\underline{m'}_i<{\overline{m}}_i\le \overline{m'}_i\\ (\underline{m}_{i},\overline{m'}_{i})&{}\text {if}&{}\underline{m'}_i\le \underline{m}_i<\overline{m'}_i<{\overline{m}}_i\\ (\underline{m'}_i,\overline{m'}_i)&{}\text {if}&{}\underline{m}_i< \underline{m'}_i<\overline{m'}_i<{\overline{m}}_i \end{array}\right. \end{aligned}$$

Assume now that $i\in S$; otherwise, there is nothing to prove. Consider first the case $\underline{m}_i<\underline{m'}_i<{\overline{m}}_i\le \overline{m'}_i$. Then, we must have $i\ne i^+$ and we can let $\overline{(m\cap m')}_i={\overline{m}}_i$. So,

• either $i=i^-$ and we get $\underline{(m\cap m')}_{i^-}=\underline{m'}_{i^-}$ from the definition of $i^-$.

• or $i\ne i^-$ and then we must have $\underline{m}_{i}<\underline{m'}_{i}<\underline{O(m\cap m')}_{i}$. Obviously, $\underline{m'}_{i}$ cannot define an active constraint of $P_{m\cap m'}^\alpha$ and we may set $\underline{(m\cap m')}_{i}=\underline{m}_{i}$ without affecting this set.

The second case $\underline{m'}_i\le \underline{m}_i<\overline{m'}_i<{\overline{m}}_i$ can be treated similarly. The third case is even simpler because either $i=i^-=i^+$ and then we get $(\underline{m'}_i,\overline{m'}_i)$, or $i\in \{i^-, i^+\}$ and $i^+\ne i^-$ and we get one of the previous cases. $\square$

Appendix E: Algorithm for InAsUP Construction

Declarations, initializations

Preliminaries: Computations of atom constraint vectors ${{\varvec{m}}}_{{\varvec{a}}}$ and constant part of ${\varvec{\Gamma }}_{{\varvec{D}}},{\varvec{\epsilon }},{{\varvec{a}}}$

Implementation

$^{18}$ In practice, this loop consists of two distinct loops; one for $m''\in \text {UP}\cap m_a$ followed by one for $m''\in \text {EP}\cap m_a$.

$^{19}$ Due to chopping, $m'$ may consists of several elements.

Annex: Definitions of Functions