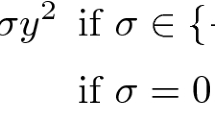Quadratic Hyperboloids in Minkowski Geometries

Abstract

A Minkowski plane is Euclidean if and only if at least one hyperbola is a quadric. We discuss the higher dimensional consequences too.

1 Introduction

Let ${\mathcal I}$ be an open, strictly convex, bounded domain in ${\mathbb R^n}$, (centrally) symmetric to the origin. Then function $d:\mathbb R^n\times \mathbb R^n\rightarrow \mathbb R$ defined by

$$\begin{aligned} d(\varvec{x} ,\varvec{y} ) =\inf \{\lambda >0:(\varvec{y}-\varvec{x})/\lambda \in \mathcal I\} \end{aligned}$$

is a metric on ${\mathbb R^n}$[1, IV.24], and is called Minkowski metric on ${\mathbb R^n}$. It satisfies the strict triangle inequality, i.e., $d(A,B)+d(B,C)=d(A,C)$ is valid if and only if $B\in \overline{AC}$. A pair $({\mathbb R^n,d})$, where d is a Minkowski metric, is called Minkowski geometry, and ${\mathcal I}$ is called the indicatrix of it. In a Minkowski geometry $({\mathbb R^n,d})$,

• D1 a set $\mathcal H_{d;F_1,F_2}^a:=\{X:2a=|d(F_1,X)-d(F_2,X)|\}$, where $a<d(F_1,F_2)/2$, is called a hyperbola if $n=2$, and a hyperboloid in higher dimensions,

where $F_1,F_2\in {\mathbb R^n}$ are called the focuses, and $a>0$ is called the radius.

A hypersurface in ${\mathbb R^n}$ is called a quadric if it is the zero set of an irreducible polynomial of degree two in n variables. We call a hypersurface quadratic if it is part of a quadric. Since every isometric mapping between two Minkowski geometries is a restriction of an affinity, and every affinity maps quadrics to quadrics, the quadraticity of a metrically defined hypersurface is a geometric property in each Minkowski geometry. Thus, the question arises whether the metrically defined hypersurfaces are quadrics. This question is answered for conics in [6].

We prove that (Theorem 4.3) a Minkowski plane is a model of the Euclidean plane, which means that the indicatrix is a bounded quadric [1, IV.25.4], if and only if at least one of the hyperbolas is a quadric, and that (Theorem 4.4) a Minkowski plane is analytic if and only if at least one of the hyperbolas is analytic.

As for higher dimensions, we prove (Theorem 5.1) that a Minkowski geometry is a model of the Euclidean geometry if and only if every central planar section of at least one quadric is either a hyperbola or an ellipse.

Similar problems for the ellipsoids were solved in [7].

2 Notations and Preliminaries

Points of ${\mathbb R^n}$ are labeled as $A,B,\dots$, vectors are denoted by $\overrightarrow{AB}$ or $\varvec{a},\varvec{b},\dots$, but we use these latter notations also for points if the origin is fixed. The open segment with end points A and B is denoted by $\overline{AB}$, while $\overline{A}B$ denotes the open ray starting from A passing through B, finally, $AB=\overline{A}B\cup A\overline{B}$.

On an affine plane, the affine ratio (A, B; C) of the collinear points A, B and C satisfies $(A,B;C)\overrightarrow{BC}=\overrightarrow{AC}$ [1, III.15.10], and the cross ratio of the collinear points A, B and C, D is $(A,B;C,D)=(A,B;C)/(A,B;D)$ [1, VI.40.17].

It is easy to observe in D1 that a hyperboloid intersects line $F_1F_2$, the main axis, in exactly two points, whose distance is twice the radius. Further notions are the (linear) eccentricity $c=d(F_1,F_2)/2$, the numerical eccentricity $\varepsilon =c/a$. The metric midpoint of the segment $\overline{F_1F_2}$ is called the center.

Notations $\varvec{u}_\varphi =(\cos \varphi ,\sin \varphi )$ and $\varvec{u}_\varphi ^\perp :=(\cos (\varphi +\pi /2),\sin (\varphi +\pi /2))$ are frequently used. It is worth noting that, by these, we have $\frac{\,{\mathrm d}}{\,{\mathrm d}\varphi }\varvec{u}_\varphi =\varvec{u}_\varphi ^\perp$.

A quadric in the plane has the equation of the form

in a suitable affine coordinate system ${\mathfrak s}$, and we call it elliptic, parabolic, or hyperbolic, if $\sigma =1$, $\sigma =0$, or $\sigma =-1$, respectively.

We usually polar parameterize the boundary $\partial {\mathcal D}$ of a non-empty domain ${\mathcal D}$ in ${\mathbb R^2}$ starlike with respect to a point $P\in {\mathcal D}$ so that $\varvec{r}:[-\pi ,\pi )\rightarrow {\mathbb R^2}$ is defined by $\varvec{r}(\varphi )= r(\varphi )\varvec{u}_\varphi ,$ where r is the radial function of ${\mathcal D}$ with base point P.

We call a curve analytic if the coordinates of its points depend on its arc length analytically.

3 Utilities

In this section, the underlying plane is Euclidean.

Lemma 3.1

The border of a convex domain is an analytic curve if and only if any one of its radial functions is analytic.

Proof

Let ${\mathcal D}$ be an open convex domain containing the origin $O=(0,0)$. Let $s\mapsto \varvec{p}(s)$ be an arc length parametrization of $\partial \mathcal D$, where $s\ge 0$, and let $\varphi \mapsto \varvec{r}(\varphi )= r(\varphi )\varvec{u}_\varphi$ be a polar parametrization of $\partial \mathcal D$ on $[-\pi ,\pi )$ such that $\varvec{p}(0)=\varvec{r}(-\pi )$. Then,

$$\begin{aligned} s(\xi ) =\int _{-\pi }^\xi |\dot{\varvec{r}}(\varphi )| \mathrm{d}\varphi =\int _{-\pi }^\xi \sqrt{\dot{r}^2(\varphi )+r^2(\varphi )} \mathrm{d}\varphi , \end{aligned}$$

(3.1)

hence the function $s:\xi \mapsto s(\xi )$ is strictly monotonously increasing, and therefore its inverse function $\sigma :s(\xi )\mapsto \xi$ exists and is strictly monotonously increasing.

First, assume the analyticity of r. Then, as r is bounded from below by a positive number, the integrand on the right-hand side of (3.1) is analytic, and therefore s is analytic. As $\dot{s}(\xi )$ is positive by (3.1), the analyticity of $\sigma$ follows from the analytic inverse function theorem [3, Theorem 4.2], and this implies the analyticity of ${\varvec{p}}(s) =\varvec{r}(\sigma (s))= r(\sigma (s))\varvec{u}_{\sigma (s)}.$

Conversely, assume that ${\varvec{p}}$ is analytic. As the derivatives of the cosine and sine functions do not vanish simultaneously, $\varvec{u}_{\sigma (s)}={\varvec{p}}(s)/|{\varvec{p}}(s)|$ proves that $\sigma$ is analytic. As the derivative $\dot{\sigma }(t)=1/\dot{s}(\sigma (t))$ vanishes nowhere, analyticity of s follows again by the analytic inverse function theorem [3, Theorem 4.2]. Then the analyticity of $r(\xi )=\langle {\varvec{p}}(s(\xi )),\varvec{u}_{\xi }\rangle$ follows.

The lemma is proved. $\square$

Notice that the differentiation of the last formula in the proof and then the substitution of the derivative of (3.1) give

$$\begin{aligned} \dot{r}(\xi ) = \langle \dot{\varvec{p}}(s(\xi )),\varvec{u}_\xi \rangle \sqrt{\dot{r}^2(\xi )+r^2(\xi )}. \end{aligned}$$

(3.2)

Let ${\mathcal H}$ be a hyperbola with center O and focuses $F_1$ and $F_2$. Let us label the intersection points of $F_1F_2$ and ${\mathcal {H}}$ so that $A\in \overline{F_1B}$. We clearly have $O\in \overline{AB}\subset \overline{F_1F_2}$, so we can choose a point W on $F_1F_2$ such that $F_2\in \overline{BW}$.

There exists an angle $\Phi \in (0,\pi /2)$ such that a unique point H exists on ${\mathcal H}$ for every $\varphi \in [0,\Phi )\cup (\pi -\Phi ,\pi )$, such that $\angle WOH=\varphi$.

Given $\varphi _0\in (0,\Phi )$, let $H_0=H(\varphi _0)$, $\alpha _0=\angle WF_1H_0$ and $\beta _0=\angle WF_2H_0$. Assuming that $H_{2i}$ is defined for an $i\in {\mathbb N}$, we define sequences recursively as follows (see Fig. 1): $H_{2i+1}:=\overline{F_1 H_{2i}}\cap \mathcal {H}$, $\alpha _{2i+1}:=\alpha _{2i}$, and $\beta _{2i+1}:=\angle WF_2H_{2i+1}$; then $H_{2i+2}:=\overline{F_2 H_{2i+1}}\cap \mathcal {H}$, $\alpha _{2i+2}=\angle WF_1H_{2i+2}$, and $\beta _{2i+2}:=\beta _{2i+1}$. We clearly have $\varphi _{2i}\in (0,\Phi )$ and $\varphi _{2i+1}\in (\pi -\Phi ,\pi )$ for every $i\in \mathbb N$.

Fig. 1

Sequence of angles

Lemma 3.2

If $i\rightarrow \infty$, then $\alpha _{2i}$ and $\varphi _{2i}$ tend to zero, $\beta _{2i}$, $\beta _{2i+1}$, and $\varphi _{2i+1}$ tend to $\pi$, and $\alpha _{2i+2}/\alpha _{2i}$ tends to $(F_1,F_2;A,B)$.

Proof

We clearly have $\varphi _{2i}<\Phi <\pi /2\ \text { and }\ \varphi _{2i+1}>\pi -\Phi >\pi /2,$ and therefore

$$\begin{aligned} \begin{aligned} \alpha _{2i}&<\pi -\beta _{2i}\quad \text {and}\quad \pi -\beta _{2i+1}<\alpha _{2i+1}\quad (\text {or}\quad \pi -\beta _{2i+2}<\alpha _{2i}),\\ \alpha _{2i+2}&<\pi -\beta _{2i+2}\quad \text {and}\quad \pi -\beta _{2i+1}<\alpha _{2i}, \end{aligned} \end{aligned}$$

hence $\beta _{2i+2}>\beta _{2i},\ \alpha _{2i+2}<\alpha _{2i}, \text { and } \pi -\beta _{2i+2}<\alpha _{2i}<\pi -\beta _{2i}.$

Thus, the sequences $\beta _{2i}$, $\beta _{2i+1}$ increase monotonously, while the sequences $\alpha _{2i}$, $\alpha _{2i+1}$ decrease monotonously. As these sequences are bounded, they are convergent.

Assuming $\lim _{i\rightarrow \infty }\beta _{2i}<\pi$, i.e., $\lim _{i\rightarrow \infty }(\pi -\beta _{2i})>0$, $\lim _{i\rightarrow \infty }\frac{\pi -\beta _{2i+2}}{\pi -\beta _{2i}}=1$, and $\lim _{i\rightarrow \infty }\frac{\alpha _{2i}}{\pi -\beta _{2i}}=1$ follow, hence the sinus law for triangle $\triangle F_1F_2H_{2i}$ implies

$$\begin{aligned} \lim \limits _{i\rightarrow \infty }\frac{d(F_2,H_{2i})}{d(H_{2i},F_1)}=\lim \limits _{i\rightarrow \infty }\frac{\sin \alpha _{2i}}{\sin (\pi -\beta _{2i})}\cdot \lim _{i\rightarrow \infty }\frac{\pi -\beta _{2i}}{\alpha _{2i}}=1, \end{aligned}$$

which, by the continuity of d, gives $d(F_2,B)=d(B,F_1),$ a contradiction.

Thus $\lim _{i\rightarrow \infty }\beta _{2i}=\pi$, hence $\beta _{2i+1}$, and $\varphi _{2i+1}$ also tend to $\pi$, and $\alpha _{2i}$, $\alpha _{2i+1}$, and $\varphi _{2i}$ tend to zero.

So, observing Fig. 1, we see that

$$\begin{aligned} \begin{aligned} h_1(\alpha _{2i})\!&:=d(F_1,\!H_{2i})\rightarrow d(F_1,B),&h_1(\alpha _{2i+1})\!&:=d(F_1,\!H_{2i+1})\rightarrow d(F_1,A),\\ h_2(\beta _{2i})\!&:=d(F_2,\!H_{2i})\rightarrow d(F_2,B),&h_2(\beta _{2i+1})\!&:=d(F_2,\!H_{2i+1})\rightarrow d(F_2,A). \end{aligned} \end{aligned}$$

(3.3)

The sine law for triangles $\triangle F_1F_2H_{2i}$ and $\triangle F_1F_2H_{2i+1}$ gives

$$\begin{aligned} \frac{h_2(\beta _{2i+1})}{h_1(\alpha _{2i+1})}=\frac{\sin \alpha _{2i+1}}{\sin (\pi -\beta _{2i+1})} \quad \text {and}\quad \frac{h_2(\beta _{2i+2})}{h_1(\alpha _{2i+2})}=\frac{\sin \alpha _{2i+2}}{\sin (\pi -\beta _{2i+2})}, \end{aligned}$$

respectively. Multiplying these by $\cos \beta _{2i+1}/\cos \alpha _{2i+1}$ and $\cos \beta _{2i+2}/\cos \alpha _{2i+2}$, respectively, and taking the ratio of the resulting fractions, we obtain

$$\begin{aligned} \frac{\tan \alpha _{2i+2}}{\tan \alpha _{2i}} =\frac{h_2(\beta _{2i+2})\cos \beta _{2i+2}}{h_1(\alpha _{2i+2})\cos \alpha _{2i+2}} \frac{h_1(\alpha _{2i+1})\cos \alpha _{2i+1}}{h_2(\beta _{2i+1})\cos \beta _{2i+1}}. \end{aligned}$$

By (3.3), the right-hand side tends to $(F_1,F_2;A,B)$, so the proof is complete. $\square$

Let $\varvec{r}_1$ and $\varvec{r}_2$ be curves in the plane with analytic arc length parametrization on $[-1,1]$ such that $\varvec{r}_1(0)=\varvec{r}_2(0)$ and $\dot{\varvec{r}}_1(0)=\dot{\varvec{r}}_2(0)$. Let $\ell$ be the line through $\varvec{r}_1(0)$ that is orthogonal to $\dot{\varvec{r}}_1(0)$, and let $F_1,F_2$, and B be different points on $\ell$ such that $B\in \overline{F_1F_2}$ and $\varvec{r}_1(0)\notin \{B,F_1,F_2\}$. Let $\varvec{h}$ be an analytic arc length parameterization of a curve such that $B=\varvec{h}(0)$ and $\dot{\varvec{h}}(0)=\varvec{u}_{\pi /2}$. Every point $H=\varvec{h}(s)$ determines two straight lines $\ell _{1}:=F_1H$ and $\ell _{2}:=F_2H$ closing small angles $\alpha$ and $\gamma =\pi -\beta$ with $\ell$, respectively. Let the straight line $\bar{\ell }_{j}$ ($j=1,2$) through the midpoint O of the segment $\overline{F_1F_2}$ be parallel to $\ell _{j}$. See Fig. 2.

Fig. 2

Specially placed curves with different lines

Denote the intersections of $\ell _1$ and $\ell _2$ with $\varvec{r}_1$ and $\varvec{r}_2$ by $\bar{C}_1$, $\bar{D}_1$ and $\bar{C}_2$, $\bar{D}_2$, respectively. Let $s_{i}$ be the arc length parameter of $\varvec{r}_i$ ($i=1,2$), and define $\delta (\alpha )=\langle C_1- D_1,\varvec{u}_\alpha \rangle$ and $\delta (\gamma )=\langle C_2- D_2,\varvec{u}_{\gamma }\rangle$, where $\gamma =\beta -\pi$.

Lemma 3.3

If the curves ${\varvec{r}}_1^{}$ and ${\varvec{r}}_2^{}$ are different in every neighborhood of the point $K:=\varvec{r}_1(0)$, and H tends to B on the curve $\varvec{h}$, then

$$\begin{aligned} \frac{\delta (\alpha )}{\delta (\gamma )}\rightarrow (F_2,F_1;B)^k, \ \text { for an integer}\ k\ge 2. \end{aligned}$$

(3.4)

Proof

If ${\varvec{r}}_1^{(i)}(0)={\varvec{r}}_2^{(i)}(0)$ for every $i\in \mathbb N$, then, by the analyticity of $\varvec{r}_1$ and $\varvec{r}_2$, ${\varvec{r}}_1^{}={\varvec{r}}_2^{}$ in a neighborhood of K, so $k:=\min \{i\in \mathbb N:{\varvec{r}}_1^{(i)}(0)\ne {\varvec{r}}_2^{(i)}(0)\}$ is well defined and is at least two.

Letting $H^\perp$ be the orthogonal projection of H onto $\ell$, L’Hôpital’s rule gives

$$\begin{aligned} \begin{aligned} \frac{|F_2-B|}{|F_1-B|}&=\lim _{s\rightarrow 0}\frac{|F_2-H^\perp |}{|F_1-H^\perp |} =\lim _{s\rightarrow 0}\frac{\tan \alpha }{-\tan \gamma } =-\lim _{s\rightarrow 0}\frac{\dot{\alpha }}{\dot{\gamma }}. \end{aligned} \end{aligned}$$

(3.5)

If $\lim _{s\rightarrow 0}\frac{\delta (\alpha )}{\delta (\gamma )}$ exists, then L’Hôpital’s rule can be used, so we get

$$\begin{aligned} \lim _{s\rightarrow 0}\frac{\delta (\alpha )}{\delta (\gamma )}&=\!\lim _{s\rightarrow 0}\frac{\dot{\delta }(\alpha )\dot{\alpha }}{\dot{\delta }(\gamma )\dot{\gamma }} =\!\lim _{s\rightarrow 0}\frac{\dot{\delta }(\alpha )}{\dot{\delta }(\gamma )} \lim _{s\rightarrow 0}\frac{\dot{\alpha }}{\dot{\gamma }} =\dots =\!\lim _{s\rightarrow 0}\frac{\delta ^{(k)}(\alpha )}{\delta ^{(k)}(\gamma )} \Big (\!\lim _{s\rightarrow 0}\frac{\dot{\alpha }}{\dot{\gamma }}\!\Big )^k\!\! \\&=\!\Big (\!\lim _{s\rightarrow 0}\frac{\dot{\alpha }}{\dot{\gamma }}\!\Big )^k . \end{aligned}$$

This proves the lemma. $\square$

4 One Hyperbola in a Minkowski Plane

We start by considering the Minkowski plane $(\mathbb R^2,d_{\mathcal I})$ with indicatrix ${\mathcal I}$.

By [4, (ii) of Theorem 3] every straight line parallel to the main axis intersects a hyperbola in exactly two points, hence if a hyperbola is a quadric, then it is a hyperbolic quadric.

Fig. 3

A hyperbola in a Minkowski plane

Let A, B be the intersections of line $F_1F_2$ with $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ such that $A\in \overline{F_1B}$ and $B\in \overline{AF_2}$. Let ${\mathcal I_O}$ be the translate of the indicatrix centered at the midpoint O of $\overline{F_1F_2}$, and let I, J be the intersections of line $F_1F_2$ with $\partial \mathcal I_O$, so that $I\in O\overline{F_1}$ and $J\in O\overline{F_2}$. Furthermore, let $t_A$, $t_B$ and $t_I$, $t_J$, respectively, denote the tangents of the appropriate curve $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ or $\partial \mathcal I_O$ at A, B and I, J, respectively. See Fig. 3.

Given the Euclidean metric $d_e$, we let r be the radial function of $\mathcal {I}_O$ with respect to O, $\alpha =\angle (HF_1O)$, $\gamma =\angle (HF_2B)$ ($\beta :=\pi -\gamma$) and $\varphi =\angle (HOB)$ for the points H on the B-branch (that contains B) of $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$. Finally, we define the lengths $h_1(\alpha ):=d_e(F_1,H)$, $h_2(\beta ):=d_e(F_2,H)$, and $h(\varphi ):=d_e(O,H)$. Then $d_{\mathcal I}(F_1,H)={h_1(\alpha )}/{r(\alpha )}$, and $d_{\mathcal I}(F_2,H)={h_2({\beta })}/{r({\beta })}$, so we have

$$\begin{aligned} 2a=\frac{h_1(\alpha )}{r(\alpha )}-\frac{h_2(\beta )}{r(\beta )}. \end{aligned}$$

(4.1)

Lemma 4.1

If the hyperbola $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ is a quadric, then $t_A\parallel t_B\parallel t_I\parallel t_J$.

Proof

Since $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ is a quadric, $\varphi$ and H are bijectively related, hence the functions $\alpha (\varphi )$, $\beta (\varphi )$ are well defined.

The symmetry of ${\mathcal I}$ entails that $t_I\parallel t_J$, and it also follows that the affine center of the quadric $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ coincides with its metric center O, hence $t_A\parallel t_B$ too.

Choose a Euclidean metric $d_e$ so that $t_A\perp F_1F_2\perp t_B$.

Differentiating (4.1) with respect to $\varphi$ leads to

$$\begin{aligned} 0 =\frac{\frac{d h_1(\alpha )}{d\alpha }r(\alpha )-h_1(\alpha )\frac{dr(\alpha )}{d\alpha }}{r^2(\alpha )}\frac{d\alpha }{\mathrm{d}\varphi } -\frac{\frac{d h_2(\beta )}{d\beta }r(\beta )-h_2(\beta )\frac{dr(\beta )}{d\beta }}{r^2(\beta )}\frac{d\beta }{\mathrm{d}\varphi } . \end{aligned}$$

(4.2)

As $\varphi =0$ implies $\alpha =0=\pi -\beta$, and $\frac{d h_1}{d\alpha }(0)=\frac{d h_2}{d\beta }(\pi )=0$ by $t_A\perp F_1F_2\perp t_B$, (4.2) gives at $\varphi =0$ that

$$\begin{aligned} r'(0)\Big [-h_1(0)\frac{d\alpha }{\mathrm{d}\varphi }(0)+h_2(\pi )\frac{d\beta }{\mathrm{d}\varphi }(0)\Big ]=0. \end{aligned}$$

Applying (3.5) for the present configuration, we obtain that the second factor in the left-hand side is negative, hence $r'(0)=0$. Thus $t_J\perp F_1F_2$, so the lemma follows. $\square$

Lemma 4.2

If the hyperbola $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ is an analytic curve in a neighborhood of A and B, then the curve $\partial \mathcal I_O$ is analytic in a neighborhood of I and J.

Proof

By Lemma 3.1 and its proof, the functions $h_1$, $h_2$, the angles $\alpha (s)$, $\beta (s)$, and the inverses of the angles, where s is the arc length parameter, are clearly analytic, hence we deduce that $\beta (\alpha )$ and $\alpha (\beta )$ are also analytic functions.

As $x\mapsto 1/x$ is analytic in a neighborhood of 1, to prove that $r(\alpha )$ is analytic in a neighborhood of 0, it is enough to prove that $\bar{r}(\alpha ):=1/r(\alpha )$ is analytic in some neighborhood of 0. Bearing this in mind, we reformulate (4.1) as

$$\begin{aligned} \bar{r}(\alpha ) =\frac{h_2(\gamma (\alpha ))}{h_1(\alpha )}\bar{r}(\gamma (\alpha )) +\frac{2a}{h_1(\alpha )}. \end{aligned}$$

(4.3)

Introduce the functions $f(\alpha ):=\gamma (\alpha )$, $g(\alpha ):=\frac{h_2(\gamma (\alpha ))}{h_1(\alpha )}$, and $e(\alpha ):=\frac{2a}{h_1(\alpha )}$. Then f, g and e are analytic in a neighborhood of 0, $f(0)=0$, $\frac{df}{d\alpha }(0)=\frac{h_2(0)}{h_1(0)}<1$, $g(0)=\frac{h_2(0)}{h_1(0)}<1$, and $h(0)=\frac{2a}{h_1(0)}<1$. Furthermore, by (4.3), the function $\phi (\alpha ):=\bar{r}(\alpha )$ solves the functional equation $\phi (\alpha )=g(\alpha )\phi (f(\alpha )) + h(\alpha )$. However, by [3, Theorem 4.6], such a functional equation has a unique solution, which additionally is analytic in a neighborhood of 0. Consequently, $r(\alpha )$ is the reciprocal of that unique analytic solution, so $\partial \mathcal I_O$ is analytic around J, and, by its symmetry, around I too. $\square$

Theorem 4.3

A Minkowski plane is a model of the Euclidean plane if and only if at least one hyperbola is a quadric.

Proof

As every hyperbola is a quadric in the Euclidean plane, we only have to prove that a Minkowski plane is Euclidean if at least one hyperbola is a quadric.

Assume that $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ is a quadric.

We have $t_A\parallel t_I\parallel t_J\parallel t_B$ by Lemma 4.1, and, as every (planar) quadric is an analytical curve, the border $\partial \mathcal I_O$ is analytic in a neighborhood of I and J by Lemma 4.2, where O is the midpoint of $\overline{F_1F_2}$. Furthermore, by the central symmetry of $\mathcal I_O$ and the definition of $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$, we have $c=d_{\mathcal I}(F_1,O)$, $\overrightarrow{AF_1}=\overrightarrow{F_2B}$ and $\overrightarrow{IA}=\overrightarrow{BJ}$, so O is the (affine) midpoint of both $\overline{IJ}$ and $\overline{AB}$. Additionally, we have $a\cdot d_{\mathcal I}(O,J)=d_{\mathcal I}(O,B)$, because the definition of $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ implies

$$\begin{aligned} \begin{aligned} 2d_{\mathcal I}(O,B)&=2d_{\mathcal I}(O,F_2)-2d_{\mathcal I}(F_2,B) \\&=d_{\mathcal I}(F_1,O)+d_{\mathcal I}(O,F_2)-d_{\mathcal I}(F_2,B) +2a-d_{\mathcal I}(F_1,B) =2a. \end{aligned} \end{aligned}$$

Being a hyperbolic quadric, $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ has two asymptotes $\ell _+$ and $\ell _-$ through O. Let $C_1$ and $C_2$ be the points where they intersect the straight line $t_A$.

Fix the affine coordinate system such as $O=(0,0)$, $J=(1,0)$, and $C_1=(c,\sqrt{c^2-a^2})$, and choose the Euclidean metric $d_e$ so that $\{(1,0),(0,1)\}$ is an orthonormal basis.

Let ${\mathcal C}$ denote the unit circle of $d_e$. See Fig. 4.

Fig. 4

Coinciding hyperbolas $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}\equiv \mathcal H_{d_e;F_1,F_2}^{a}$

Then both $\mathcal H_{d_{e};F_1,F_2}^{a}$ and $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ are hyperbolic quadrics, and have two common tangents $t_A$ and $t_B$, two common asymptotes, and two common points A and B, hence they coincide.

By the definition of $\mathcal H_{d_{e};F_1,F_2}^{a}$ we have $h_1(\alpha )-h_2(\beta )=2a,$ which together with (4.1) implies

$$\begin{aligned} \delta (\alpha ) =\delta (\beta )\frac{h_2(\beta )}{h_1(\alpha )+2a\delta (\beta )}, \end{aligned}$$

(4.4)

where $\delta (\alpha )=1-r(\alpha )$ is the radial difference of ${\mathcal C}$ and $\partial \mathcal I_O$.

If in every neighborhood of I curves $\mathcal C$ and $\partial \mathcal I_O$ differ, then (4.4) implies

$$\begin{aligned} \lim _{\varphi \rightarrow 0}\frac{\delta (\alpha )}{\delta (\beta )} =\frac{c-a}{c+a}=(F_2,F_1;B), \end{aligned}$$

which, by (3.4), implies $(F_2,F_1;B)=1$. This contradicts $a>0$, so the curves ${\mathcal C}$ and $\partial \mathcal I_O$ coincide in a neighborhood of I.

However, if $\delta (\beta _0)\ne 0$ for a $\beta _0$, then no value of the 0-convergent sequence $\beta _{2i}$ constructed in Lemma 3.2 can vanish by (4.4), therefore no $\beta _0$ can exist for which $\delta (\beta _0)\ne 0$. Similarly follows that no $\alpha$ exists for which $\delta (\alpha )\ne 0$, hence $\mathcal C$ and $\partial \mathcal I_O$ coincide. $\square$

This kind of implication extends over to analyticity too.

Theorem 4.4

The indicatrix of a Minkowski plane is analytic if and only if one of the hyperbolas of the Minkowski plane is analytic.

Proof

First, assume that the Minkowski plane $(\mathbb R^2,d_{\mathcal I})$ is analytic.

We use the notations introduced in the previous sections, and consider the hyperbola $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$.

Fix an arbitrary point $H_0\in \mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$, and let the point $R_i\in {\mathcal I}$ ($i=1,2$) be such that $O\overline{R_i}\parallel F_i\overline{H_0}$. Let the straight line $t_i$ ($i=1,2$) be tangent to ${\mathcal I}$ at $R_i$. Let $d_e$ be the Euclidean metric which satisfies $t_2\perp OR_2$, $d_e(O,R_1)=d_e(O,R_2)$, and $d_e(O,J)=1$. Then we have

$$\begin{aligned} \begin{aligned} h_2^2(\beta )&=h_1^2(\alpha )+4c^2-4h_1(\alpha )c\cos \alpha , \ \text { and }\ \beta =\arcsin \frac{h_1(\alpha )\sin \alpha }{h_2(\beta )}. \end{aligned} \end{aligned}$$

Substituting this into (4.1) results in the analytic equation

$$\begin{aligned} F(\alpha ,h_1(\alpha )) :=\Big (2a-\frac{h_1(\alpha )}{r(\alpha )}\Big )^2 -\frac{h_1^2(\alpha )+4c^2-4h_1(\alpha )c\cos \alpha }{r^2\big (\arcsin \frac{h_1(\alpha )\sin \alpha }{\sqrt{h_1^2(\alpha )+4c^2-4h_1(\alpha )c\cos \alpha }}\big )} =0. \end{aligned}$$

Since

$$\begin{aligned} \begin{aligned} \partial _2F(\alpha ,h_1(\alpha )) =&2\frac{-h_2(\beta )}{r(\beta )}\frac{-1}{r(\alpha )} -\frac{2h_1(\alpha )-4c\cos \alpha }{r^2(\beta )} +\\&+2\frac{h_2^2(\beta )}{r^3(\beta )} \frac{\dot{r}(\beta )}{\cos \beta } \Big ( \frac{\sin \alpha }{h_2(\beta )}-\frac{1}{2} \frac{h_1(\alpha )\sin \alpha (2h_1(\alpha )-4c\cos \alpha )}{h_2^3(\beta )} \Big ), \end{aligned} \end{aligned}$$

$\partial _2F(\alpha ,h_1(\alpha ))$ vanishes if and only if

$$\begin{aligned} -\frac{h_2(\beta )}{r(\alpha )} +\frac{h_1(\alpha )-2c\cos \alpha }{r(\beta )} = \frac{h_2(\beta )\sin \alpha }{r(\beta )\cos \beta } \frac{\dot{r}(\beta )}{r(\beta )} \Big (1- \frac{h_1(\alpha )(h_1(\alpha )-2c\cos \alpha )}{h_2^2(\beta )} \Big ). \end{aligned}$$

By (3.2), we have $\frac{\dot{r}(\beta )}{r(\beta )}=\cot \theta$, where $\theta$ is the angle between $F_1\overline{H}$ and the tangent vector at H of $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$. Furthermore, it can be easily seen that $h_1(\alpha )-2c\cos \alpha =h_2(\beta )\cos (\beta -\alpha )$. Thus, the above equation is equivalent to

$$\begin{aligned} -\frac{h_2(\beta )}{r(\alpha )} +\frac{h_2(\beta )\cos (\beta -\alpha )}{r(\beta )} = \frac{\cot \theta }{r(\beta )\cos \beta } (h_2(\beta )\sin \alpha -h_1(\alpha )\cos (\beta -\alpha )\sin \alpha ). \end{aligned}$$

Since $h_2(\beta )\sin \beta =h_1(\alpha )\sin \alpha$, this equation simplifies to

$$\begin{aligned} \frac{-1}{r(\alpha )} +\frac{\cos (\beta -\alpha )}{r(\beta )} = \frac{\cot \theta }{r(\beta )} \frac{\sin \alpha -\sin \beta \cos (\beta -\alpha )}{\cos \beta } =-\sin (\beta -\alpha )\frac{\cot \theta }{r(\beta )}. \end{aligned}$$

In sum, $\partial _2F(\alpha ,h_1(\alpha ))$ vanishes if and only if

$$\begin{aligned} \frac{-r(\beta )}{r(\alpha )}+\sin (\beta -\alpha )(\cot \theta +\cot (\beta -\alpha ))=0. \end{aligned}$$

(4.5)

At $H_0$ we have $\theta \!=\!\pi /2$, and $r(\beta )\!=\!r(\alpha )$, therefore (4.5) reduces to $\cos (\beta \!-\!\alpha )\!=\!1$, resulting in $\beta =\alpha$, a contradiction. Thus $\partial _2F(\alpha ,h_1(\alpha ))\ne 0$ at $H_0$, hence the analytic implicit function theorem [3, Theorem 4.1] implies the analyticity of $h_1$ in a neighborhood of $\alpha$. As the point $H_0$ was chosen arbitrarily on $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$, this proves that $\mathcal H_{d_{\mathcal I};F_1,F_2}^{a}$ is analytic.

Assuming now that the hyperbola is analytic, Lemma 4.2 proves the analyticity of the boundary of the indicatrix, where $F_1F_2$ intersects it. By (4.3) we have

$$\begin{aligned} \bar{r}(\beta (\alpha )) =\frac{h_1(\alpha )}{-h_2(\beta (\alpha ))}\bar{r}(\alpha )+\frac{2a}{h_2(\beta (\alpha ))}. \end{aligned}$$

This shows that if $\bar{r}$ is analytic in an interval $(-\varepsilon ,\varepsilon )$, then it is also analytic in the interval $(-\beta (\varepsilon ),\beta (\varepsilon ))$. According to Lemma 3.2, this means that the boundary of the indicatrix is analytic at all the directions. $\square$

5 Quadrics in a Minkowski Geometry

Before presenting the proof of Theorem 5.1, let us rephrase its statement for the planar case: if one hyperbola is a quadric, then the Minkowski plane is a model of the Euclidean geometry.

In a Minkowski geometry $(\mathbb R^n,d)$

• (D2) a set $\mathcal E_{d;F_1,F_2}^a:=\!\{E:2a=d(F_1,E)+d(E,F_2)\}$, where $a>d(F_1,F_2)/2$, is called an ellipse if $n=2$, and an ellipsoid in higher dimensions,

where $F_1,F_2\in \mathcal M$ are called the focuses, and $a>0$ is called the radius.

Theorem 5.1

A Minkowski geometry is a model of the Euclidean geometry if and only if through a point every planar section of at least one quadric is either a hyperbola or an ellipse.

Proof

As every planar section of each hyperbolic quadric is either a hyperbola or an ellipse in the Euclidean geometry, it is enough to prove that a Minkowski geometry is Euclidean if every planar section of at least one quadric is either a hyperbola or an ellipse.

Let the quadric ${\mathcal Q}$ be such that its every planar section is either a hyperbola or an ellipse. If the planar section is a hyperbola, then Theorem 4.3 implies that the parallel central planar section of the indicatrix is an ellipse. If the planar section is an ellipse, then [7, Theorem 4.3] implies that the parallel central planar section of the indicatrix is an ellipse. Thus, the statement of the theorem follows immediately from [2, II.16.12], which states for any integers $1<k<n$ that the border $\partial \mathcal K$ of a convex body $\mathcal K\subset \mathbb {R}^n$ is an ellipsoid if and only if every k-plane through an inner point of ${\mathcal K}$ intersects $\partial \mathcal K$ in a k-dimensional ellipsoid. $\square$

We omit the easy proof of the following result that closes this paper.

Theorem 5.2

A Minkowski geometry is a model of the Euclidean geometry if and only if there is a hyperplane and a point in it such that every line in the hyperplane through the point is parallel to main axis of some ellipsoid that is a quadric.