Skip to main content
Download PDF

Reconstructing parton distribution functions from Ioffe time data: from Bayesian methods to neural networks

Journal of High Energy Physics volume 2019, Article number: 57 (2019) Cite this article

A preprint version of the article is available at arXiv.

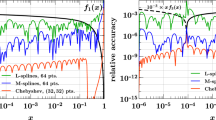
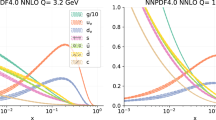
Abstract

The computation of the parton distribution functions (PDF) or distribution amplitudes (DA) of hadrons from first principles lattice QCD constitutes a central open problem in high energy nuclear physics. In this study, we present and evaluate the efficiency of several numerical methods, well established in the study of inverse problems, to reconstruct the full x-dependence of PDFs. Our starting point are the so called Ioffe time PDFs, which are accessible from Euclidean time simulations in conjunction with a matching procedure. Using realistic mock data tests, we find that the ill-posed incomplete Fourier transform underlying the reconstruction requires careful regularization, for which both the Bayesian approach as well as neural networks are efficient and flexible choices.

References

  1. R. Feynman, Photon-hadron interactions, Advanced Books Classics, Avalon Publishing (1998).

  2. K.-F. Liu, Parton degrees of freedom from the path integral formalism, Phys. Rev. D 62 (2000) 074501 [hep-ph/9910306] [INSPIRE].

  3. W. Detmold and C.J.D. Lin, Deep-inelastic scattering and the operator product expansion in lattice QCD, Phys. Rev. D 73 (2006) 014501 [hep-lat/0507007] [INSPIRE].

  4. V. Braun and D. Mueller, Exclusive processes in position space and the pion distribution amplitude, Eur. Phys. J. C 55 (2008) 349 [arXiv:0709.1348] [INSPIRE].

    ADS  Article  Google Scholar 

  5. X. Ji, Parton physics on a Euclidean lattice, Phys. Rev. Lett. 110 (2013) 262002 [arXiv:1305.1539] [INSPIRE].

    ADS  Article  Google Scholar 

  6. Y.-Q. Ma and J.-W. Qiu, Extracting parton distribution functions from lattice QCD calculations, Phys. Rev. D 98 (2018) 074021 [arXiv:1404.6860] [INSPIRE].

    ADS  Google Scholar 

  7. A.V. Radyushkin, Quasi-parton distribution functions, momentum distributions and pseudo-parton distribution functions, Phys. Rev. D 96 (2017) 034025 [arXiv:1705.01488] [INSPIRE].

    ADS  MathSciNet  Google Scholar 

  8. A.J. Chambers et al., Nucleon structure functions from operator product expansion on the lattice, Phys. Rev. Lett. 118 (2017) 242001 [arXiv:1703.01153] [INSPIRE].

    ADS  Article  Google Scholar 

  9. C. Best et al., Pion and rho structure functions from lattice QCD, Phys. Rev. D 56 (1997) 2743 [hep-lat/9703014] [INSPIRE].

  10. Zeuthen-Rome (ZeRo) collaboration, Non-perturbative pion matrix element of a twist-2 operator from the lattice, Eur. Phys. J. C 40 (2005) 69 [hep-lat/0405027] [INSPIRE].

  11. C. Alexandrou et al., Nucleon Spin and Momentum Decomposition Using Lattice QCD Simulations, Phys. Rev. Lett. 119 (2017) 142002 [arXiv:1706.02973] [INSPIRE].

    ADS  Article  Google Scholar 

  12. M. Oehm et al., 〈x〉 and 〈x 2〉 of the pion PDF from lattice QCD with N f = 2 + 1 + 1 dynamical quark flavors, Phys. Rev. D 99 (2019) 014508 [arXiv:1810.09743] [INSPIRE].

  13. K. Orginos, A. Radyushkin, J. Karpie and S. Zafeiropoulos, Lattice QCD exploration of parton pseudo-distribution functions, Phys. Rev. D 96 (2017) 094503 [arXiv:1706.05373] [INSPIRE].

    ADS  MathSciNet  Google Scholar 

  14. A. Radyushkin, One-loop evolution of parton pseudo-distribution functions on the lattice, Phys. Rev. D 98 (2018) 014019 [arXiv:1801.02427] [INSPIRE].

    ADS  Google Scholar 

  15. J.-H. Zhang, J.-W. Chen and C. Monahan, Parton distribution functions from reduced Ioffe-time distributions, Phys. Rev. D 97 (2018) 074508 [arXiv:1801.03023] [INSPIRE].

    ADS  Google Scholar 

  16. T. Izubuchi et al., Factorization theorem relating euclidean and light-cone parton distributions, Phys. Rev. D 98 (2018) 056004 [arXiv:1801.03917] [INSPIRE].

    ADS  Google Scholar 

  17. J. Karpie, K. Orginos, A. Radyushkin and S. Zafeiropoulos, Parton distribution functions on the lattice and in the continuum, EPJ Web Conf. 175 (2018) 06032 [arXiv:1710.08288] [INSPIRE].

    Article  Google Scholar 

  18. X. Xiong, X. Ji, J.-H. Zhang and Y. Zhao, One-loop matching for parton distributions: nonsinglet case, Phys. Rev. D 90 (2014) 014051 [arXiv:1310.7471] [INSPIRE].

    ADS  Google Scholar 

  19. I.W. Stewart and Y. Zhao, Matching the quasiparton distribution in a momentum subtraction scheme, Phys. Rev. D 97 (2018) 054512 [arXiv:1709.04933] [INSPIRE].

    ADS  MathSciNet  Google Scholar 

  20. H.-W. Lin, J.-W. Chen, S.D. Cohen and X. Ji, Flavor structure of the nucleon sea from lattice QCD, Phys. Rev. D 91 (2015) 054510 [arXiv:1402.1462] [INSPIRE].

    ADS  Google Scholar 

  21. C. Alexandrou et al., Lattice calculation of parton distributions, Phys. Rev. D 92 (2015) 014502 [arXiv:1504.07455] [INSPIRE].

    ADS  Google Scholar 

  22. J.-W. Chen et al., Nucleon helicity and transversity parton distributions from lattice QCD, Nucl. Phys. B 911 (2016) 246 [arXiv:1603.06664] [INSPIRE].

    ADS  Article  MATH  Google Scholar 

  23. C. Alexandrou et al., Light-cone parton distribution functions from lattice QCD, Phys. Rev. Lett. 121 (2018) 112001 [arXiv:1803.02685] [INSPIRE].

    ADS  Article  Google Scholar 

  24. J.-H. Zhang et al., Pion distribution amplitude from lattice QCD, Phys. Rev. D 95 (2017) 094514 [arXiv:1702.00008] [INSPIRE].

    ADS  Google Scholar 

  25. X. Ji, J.-H. Zhang and Y. Zhao, More on large-momentum effective theory approach to parton physics, Nucl. Phys. B 924 (2017) 366 [arXiv:1706.07416] [INSPIRE].

    ADS  Article  MATH  Google Scholar 

  26. A.V. Radyushkin, Structure of parton quasi-distributions and their moments, Phys. Lett. B 788 (2019) 380 [arXiv:1807.07509] [INSPIRE].

    ADS  Article  MATH  Google Scholar 

  27. J. Karpie, K. Orginos and S. Zafeiropoulos, Moments of Ioffe time parton distribution functions from non-local matrix elements, JHEP 11 (2018) 178 [arXiv:1807.10933] [INSPIRE].

    ADS  MathSciNet  Article  MATH  Google Scholar 

  28. J.-W. Chen et al., Parton distribution function with nonperturbative renormalization from lattice QCD, Phys. Rev. D 97 (2018) 014505 [arXiv:1706.01295] [INSPIRE].

    ADS  Google Scholar 

  29. W. Broniowski and E. Ruiz Arriola, Partonic quasidistributions of the proton and pion from transverse-momentum distributions, Phys. Rev. D 97 (2018) 034031 [arXiv:1711.03377] [INSPIRE].

    ADS  Google Scholar 

  30. Y.-Q. Ma and J.-W. Qiu, Exploring partonic structure of hadrons using ab initio lattice QCD calculations, Phys. Rev. Lett. 120 (2018) 022003 [arXiv:1709.03018] [INSPIRE].

    ADS  Article  Google Scholar 

  31. K.-F. Liu and S.-J. Dong, Origin of difference between \( \overline{d} \) and ū partons in the nucleon, Phys. Rev. Lett. 72 (1994) 1790 [hep-ph/9306299] [INSPIRE].

  32. G.S. Bali et al., Pion distribution amplitude from Euclidean correlation functions: exploring universality and higher-twist effects, Phys. Rev. D 98 (2018) 094507 [arXiv:1807.06671] [INSPIRE].

    ADS  Google Scholar 

  33. R.S. Sufian et al., Pion valence quark distribution from matrix element calculated in lattice QCD, arXiv:1901.03921 [INSPIRE].

  34. H.-W. Lin et al., Parton distributions and lattice QCD calculations: a community white paper, Prog. Part. Nucl. Phys. 100 (2018) 107 [arXiv:1711.07916] [INSPIRE].

    ADS  Article  Google Scholar 

  35. K. Cichy and M. Constantinou, A guide to light-cone PDFs from lattice QCD: an overview of approaches, techniques and results, arXiv:1811.07248 [INSPIRE].

  36. C. Monahan, Recent developments in x-dependent structure calculations, PoS(LATTICE 2018)018 [arXiv:1811.00678] [INSPIRE].

  37. LP3 collaboration, Improved parton distribution functions at the physical pion mass, Phys. Rev. D 98 (2018) 054504 [arXiv:1708.05301] [INSPIRE].

  38. G. Backus and F. Gilbert, The resolving power of gross Earth data, Geophys. J. Int. 16 (1968) 169.

    ADS  Article  MATH  Google Scholar 

  39. S.A. Teukolsky, W.T. Vetterling and B.P. Flannery, Numerical recipes in C: the art of scientific computing, Cambridge University Press, Cambridge U.K. (1992).

    MATH  Google Scholar 

  40. B.B. Brandt, A. Francis, H.B. Meyer and D. Robaina, Pion quasiparticle in the low-temperature phase of QCD, Phys. Rev. D 92 (2015) 094510 [arXiv:1506.05732] [INSPIRE].

    ADS  Google Scholar 

  41. R.-A. Tripolt, P. Gubler, M. Ulybyshev and L. Von Smekal, Numerical analytic continuation of Euclidean data, Comput. Phys. Commun. 237 (2019) 129 [arXiv:1801.10348] [INSPIRE].

    ADS  MathSciNet  Article  Google Scholar 

  42. J. Liang, K.-F. Liu and Y.-B. Yang, Lattice calculation of hadronic tensor of the nucleon, EPJ Web Conf. 175 (2018) 14014 [arXiv:1710.11145] [INSPIRE].

    Article  Google Scholar 

  43. M.V. Ulybyshev, C. Winterowd and S. Zafeiropoulos, Direct detection of metal-insulator phase transitions using the modified Backus-Gilbert method, EPJ Web Conf. 175 (2018) 03008 [arXiv:1710.06675] [INSPIRE].

    Article  Google Scholar 

  44. M. Ulybyshev, C. Winterowd and S. Zafeiropoulos, Collective charge excitations and the metal-insulator transition in the square lattice Hubbard-Coulomb model, Phys. Rev. B 96 (2017) 205115 [arXiv:1707.04212] [INSPIRE].

    ADS  Article  Google Scholar 

  45. S. Forte, L. Garrido, J.I. Latorre and A. Piccione, Neural network parametrization of deep inelastic structure functions, JHEP 05 (2002) 062 [hep-ph/0204232] [INSPIRE].

  46. NNPDF collaboration, Parton distributions from high-precision collider data, Eur. Phys. J. C 77 (2017) 663 [arXiv:1706.00428] [INSPIRE].

  47. NNPDF collaboration, Parton distributions for the LHC Run II, JHEP 04 (2015) 040 [arXiv:1410.8849] [INSPIRE].

  48. R.D. Ball et al., A first unbiased global NLO determination of parton distributions and their uncertainties, Nucl. Phys. B 838 (2010) 136 [arXiv:1002.4407] [INSPIRE].

    ADS  Article  MATH  Google Scholar 

  49. NNPDF collaboration, Reweighting NNPDFs: the W lepton asymmetry, Nucl. Phys. B 849 (2011) 112 [Erratum ibid. B 854 (2012) 926] [arXiv:1012.0836] [INSPIRE].

  50. J. Rojo, Machine Learning tools for global PDF fits, talk given at the 13th Conference on Quark Confinement and the Hadron Spectrum (Confinement XIII), July 31–August 6, Maynooth, Ireland (2018), arXiv:1809.04392 [INSPIRE].

  51. J. Skilling and S.F. Gull, Bayesian maximum entropy image reconstruction, Lecture Notes — Monograph Series volume 20, Institute of Mathematical Statistics, Hayward, U.S.A. (1991).

  52. M. Asakawa, T. Hatsuda and Y. Nakahara, Maximum entropy analysis of the spectral functions in lattice QCD, Prog. Part. Nucl. Phys. 46 (2001) 459 [hep-lat/0011040] [INSPIRE].

  53. A. Rothkopf, Improved maximum entropy analysis with an extended search space, J. Comput. Phys. 238 (2013) 106 [arXiv:1110.6285] [INSPIRE].

    ADS  MathSciNet  Article  Google Scholar 

  54. Y. Burnier and A. Rothkopf, Bayesian approach to spectral function reconstruction for euclidean quantum field theories, Phys. Rev. Lett. 111 (2013) 182003 [arXiv:1307.6106] [INSPIRE].

    ADS  Article  Google Scholar 

  55. A. Buckley et al., LHAPDF6: parton density access in the LHC precision era, Eur. Phys. J. C 75 (2015) 132 [arXiv:1412.7420] [INSPIRE].

    ADS  Article  Google Scholar 

  56. B. Carpenter et al., Stan: a probabilistic programming language, J. Stat. Softw. 76 (2017).

Download references

Open Access

This article is distributed under the terms of the Creative Commons Attribution License (CC-BY 4.0), which permits any use, distribution and reproduction in any medium, provided the original author(s) and source are credited.

Author information

Authors and Affiliations

  1. Department of Physics, The College of William & Mary, Williamsburg, VA, 23187, U.S.A.

    Joseph Karpie & Kostas Orginos

  2. Thomas Jefferson National Accelerator Facility, Newport News, VA, 23606, U.S.A.

    Joseph Karpie & Kostas Orginos

  3. Faculty of Science and Technology, University of Stavanger, 4021, Stavanger, Norway

    Alexander Rothkopf

  4. Institute for Theoretical Physics, Heidelberg University, Philosophenweg 12, 69120, Heidelberg, Germany

    Savvas Zafeiropoulos

Authors
  1. Joseph Karpie
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kostas Orginos
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Alexander Rothkopf
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Savvas Zafeiropoulos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Savvas Zafeiropoulos.

Additional information

ArXiv ePrint: 1901.05408

Rights and permissions

Open Access  This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made.

The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.

To view a copy of this licence, visit https://creativecommons.org/licenses/by/4.0/.

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Karpie, J., Orginos, K., Rothkopf, A. et al. Reconstructing parton distribution functions from Ioffe time data: from Bayesian methods to neural networks. J. High Energ. Phys. 2019, 57 (2019). https://doi.org/10.1007/JHEP04(2019)057

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/JHEP04(2019)057

Keywords

  • Lattice QCD
  • Lattice Quantum Field Theory