Abstract
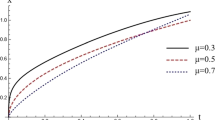
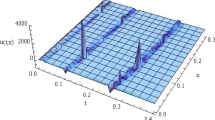
This paper deals with the solutions of linear inhomogeneous time-fractional partial differential equations in applied mathematics and fluid mechanics. The fractional derivatives are described in the Caputo sense. The fractional Green function method is used to obtain solutions for time-fractional wave equation, linearized time-fractional Burgers equation, and linear time-fractional KdV equation. The new approach introduces a promising tool for solving fractional partial differential equations.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
A. Hanyga,Multidimensional solutions of time-fractional diffusion-wave equations, Proc. R. Soc. Lond. A458 (2002), 933–957.
Ch. Fox,The G and H functions as symmetrical Fourier kernels, Trans. Amer. Math. Soc.98 (1961), 395–429.
F. Huang and F. Liu,The time fractional diffusion equation and fractional advection-dispersion equation, ANZIAM J.46 (2005), 1–14.
F. Huang and F. Liu,The fundamental solution of the space-time fractional advection-dispersion equation, J. Appl. Math. & Computing18(1-2) (2005), 339–350.
F. Mainardi,Fractional relaxation-oscillation and fractional diffusion-wave phenomena, Chaos Solitons and Fractals7 (1996), 1461–1477.
F. Mainardi, Y. Luchko and G. Pagnini,The fundamental solution of the space-time fractional diffusion equation, Frac. Calc. Appl. Anal.4 (2001), 153–192
G. Samko, A. Kilbas and O. Marichev,Fractional integrals and derivatives: Theory and applications, Gordon and Breach, Amsterdam, 1993.
H. Beyer and S. Kempfle,Definition of physically consistent damping laws with fractional derivatives, Z. Angew Math. Mech.75 (1995), 623–635.
I. Podlubny,The laplace transform method for linear differential equations of fractional order, Slovac Academy of Science, Slovak Republic, 1994.
I. Podlubny,Fractional differential equations, Academic Press, San Diego, CA, 1999.
K. B. Oldham and J. Spanier,The fractional calculus, Academic Press, New York, 1974
L. Debnath and D. Bhatta,Solutions to few linear fractional inhomogeneous partial differential equations in fluid mechanics, Fract. Calc. Appl. Anal., 7, No 1 (2004), pp. 21–36.
L. M. Campos,On the solution of some simple fractional differential equations, Internat. J. Math. & Math. Sci.13(3) (1990), 481–496.
M. Wyss and W. Wyss,Evolution, its fractional extension and generalization, Fract. Calc. Appl. Anal.4(3) (2001), 273–284.
N. Asmar,Partial differential equations and boundary value problems, Prentice Hall, New Jersey (1999).
R. Gorenflo and F. Mainardi,Fractional calculus: Integral and differential equations of fractional order: From, Fractals and fractional calculus, Carpinteri & Mainardi, New York, 1997.
R. Gorenflo and F. Mainardi,Fractional calculus and stable probability distributions, Arch. Mech.50 (1998), 377–388.
S. Miller and B. Ross,An introduction to the fractional calculus and fractional differential eqautions, John Wiley & Sons, USA, 1993.
W. Schneider and W. Wyss,Fractional diffusion and wave equations, J. Math. Phys.30 (1989), 134–144.
W. Wyss,The fractional Black-Scholes equation, Fract. Calc. Appl. Anal.3(1) (2000), 51–61.
Y. Luchko and H. Srivastava,The exact solution of certain differential equations of fractional order by using operational calculus, Comput. Math. Appl.29 (1995), 73–85.
Y. Luchko and R. Gorenflo,Scale-invariant solutions of a partial differential equation of fractional order, Fract. Calc. Appl. Anal.3(1) (1998), 63–78.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Momani, S., Odibat, Z.M. Fractional green function for linear time-fractional inhomogeneous partial differential equations in fluid mechanics. J. Appl. Math. Comput. 24, 167–178 (2007). https://doi.org/10.1007/BF02832308
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF02832308