Skip to main content
SpringerLink
Log in

On the analysis of time-periodic nonlinear dynamical systems

  • Recent Advances In Mechanical Engineering
  • Published:
Sadhana Aims and scope Submit manuscript

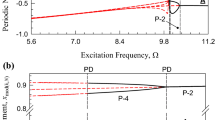
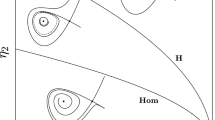
Abstract

In this study, a general technique for the analysis of time-period nonlinear dynamical systems is presented. The method is based on the fact that all quasilinear periodic systems can be replaced by similar systems whose linear parts are time invariant via the well-known Liapunov-Floquet (L-F) transformation. A general procedure for the computation of L-F transformation in terms of Chebyshev polynomials is outlined. Once the transformation has been applied, a periodic orbit in original coordinates has a fixed point representation in the transformed coordinates. The stability and bifurcation analysis of the transformed equations are studied by employing thetime-dependent normal form theory and time-dependent centre manifold reduction. For the two examples considered, the three generic codimension-one bifurcations, viz, Hopf, flip and tangent, are analysed. The methodology is semi-analytic in nature and provides a quantitative measure of stability even under critical conditions. Unlike the perturbation or averaging techniques, this method is applicable even to those systems where the periodic term in the linear part does not contain a small parameter or a generating solution does not exist due to the absence of the time-invariant term in the linear part.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Arnold V I 1988Geometrical methods in the theory of ordinary differential equations (New York: Springer-Verlag)

    Google Scholar 

  • Awreicewicz J 1989Bifurcation and chaos in simple dynamical systems (Singapore: World Scientific)

    Google Scholar 

  • Bernussou J 1977Point mapping stability (New York: Pergamon)

    MATH  Google Scholar 

  • Birkhoff G D 1966Dynamical system (Providence: Am. Math. Soc.) vol. 9

    Google Scholar 

  • Bogoliubov N N, Mitropolsky Yu A 1961Asymptotic methods in the theory of nonlinear oscillations (New York: Gordon and Breach)

    Google Scholar 

  • Bramwell A R S 1976Helicopter dynamics (London: Edward Arnold)

    Google Scholar 

  • Bruno A D 1989Local methods in nonlinear differential equations (Berlin, Heidelberg: Providence: Springer-Verlag)

    MATH  Google Scholar 

  • Carr J 1981Applications of center manifold theory (New York: Springer-Verlag)

    Google Scholar 

  • Chow S N, Wang D 1985 Normal forms of bifurcating periodic orbits.Contemporary Mathematics. Vol. 56 (Proceedings of a summer research conference, July 1985) (eds) M Golubitsky, J Guckenheimer (Providence, RI: Am. Math. Soc.)

    Google Scholar 

  • Coddington E A, Levinson N 1955Theory of ordinary differential equations (New York: McGraw Hill)

    MATH  Google Scholar 

  • Cullum J, Willoughby R A (eds) 1986 A practical procedure for computing eigenvalues of large sparse nonsymmetric matrices.Large scale eigenvalue problem (New York: Elsevier Science)

    Google Scholar 

  • Doedel E J, Kernévez J P 1986 AUTO: Software for continuation and bifurcation problems in ordinary differential equations. Applied Mathematics Report, California Institute of Technology

  • Flashner H, Hsu C S 1983 A study of nonlinear periodic systems via the point mapping method.Int. J. Numer. Meth. Eng. 19: 185–215

    Article  MATH  MathSciNet  Google Scholar 

  • Floquét G 1883 Sur les équations differentials linéaires a coefficients périodiques.Annales Scientifiques de l’Ecole Normale Superieue series 2, vol. 12

  • Friedmann P, Hammond C E, Woo T H 1977 Efficient numerical treatment of periodic systems with application to stability problems.Int. J. Num. Meth. Eng. 11: 1117–1136

    Article  MATH  MathSciNet  Google Scholar 

  • Gaonkar G H, Peters D A 1986 Review of Floquet theory in stability and response analysis of dynamic systems with periodic coefficients.Recent trend in aeroelasticity, structures, and structural dynamics (ed) P Hajela (Gainsville, FL: University of Florida Press) p 101

    Google Scholar 

  • Gaonkar G H, Simha Prasad D S, Sastry D 1981 On computing Floquet transition matrices of rotorcraft.J. Am. Helicopter Soc. 26: 56–61

    Google Scholar 

  • Guttalu R S, Flashner H 1989 Periodic solutions of nonlinear autonomous systems by approximate point mappings.J. Sound Vibr. 129: 291–311

    Article  MathSciNet  Google Scholar 

  • Guttalu R S, Flashner H 1990 Analysis of dynamical systems by truncated point mapping and cell mapping.Nonlinear dynamics in engineering systems (ed) W Schiehlen (New York: Springer-Verlag)

    Google Scholar 

  • Guckenheimer J, Holmes P 1983Nonlinear oscillations, dynamical systems, and bifurcations of vector fields (New York: Springer-Verlag)

    MATH  Google Scholar 

  • Hale J, Kocak H 1991Dynamics and bifurcations (New York: Springer-Verlag)

    MATH  Google Scholar 

  • Hsu C S 1987Cell-to-cell Mapping (New York: Springer-Verlag)

    MATH  Google Scholar 

  • Hsu C S, Cheng W H 1973 Applications of the theory of impulsive parametric excitation and new treatments of general parametric excitation problems.J. Appl. Mech. 40: 78–86

    MATH  Google Scholar 

  • Hsu C S, Cheng W H 1974 Steady-state response of a dynamical system under combined parametric and forcing excitations.J. Appl. Mech. 41: 371–378

    MATH  Google Scholar 

  • Jin J-D, Matsuzaki Y 1988 Bifurcations in a two-degree-of-freedom elastic system with follower forces.J. Sound and Vibr. 126: 265–277

    Article  MathSciNet  Google Scholar 

  • Johnson W 1980Helicopter theory (Princeton, NJ: University Press)

    Google Scholar 

  • Joseph P, Pandiyan R, Sinha S C 1993 Optimal control of mechanical systems subjected to periodic loading via Chebyshev polynomials.Optimal control applications and methods pp 75–90

  • Kretz M 1976 Research in multicyclic and active control of rotary wings.Vertica 1: 95–105

    Google Scholar 

  • Lalanne M, Ferraris G 1990Rotordynamics prediction in engineering (Chichester: John Wiley and Sons)

    Google Scholar 

  • Lindh K G, Likins P W 1970 Infinite determinant methods for stability analysis of periodic-coefficient differential equations.AIAA J. 8: 680–686

    MATH  Google Scholar 

  • Lindtner E, Steindl A, Troger H 1990 Generic one-parameter bifurcations in the motion of a simple robot.Continuation techniques and bifurcation problems (ISNM 92) (eds) H D Mittelmann, D Roose (Birkhauser); also inJ. Comput. Appl. Math. (1989) 26: 199–218

  • Lukes D L 1982Differential equations: Classical to controlled (New York: Academic Press)

    MATH  Google Scholar 

  • Malkin I G 1962 Some basic theorems of the theory of stability of motion in critical cases.Stability and dynamic systems, Translations, American Mathematical Society, Series 1 (Am. Math. Soc.) 5: 242–290

    Google Scholar 

  • Mckillip R M Jr 1985 Periodic control of individual-blade-control helicopter rotor.Vertica 9: 199–225

    Google Scholar 

  • Nayfeh A H 1973Perturbation methods (New York: Wiley)

    MATH  Google Scholar 

  • Pandiyan R 1994Analysis and control of nonlinear dynamic systems with periodically varying parameters. Ph D dissertation, Dept. Mechanical Engineering, Auburn University

  • Pandiyan R, Sinha S C 1995 Analysis of time-periodic nonlinear dynamical systems undergoing bifurcations.Nonlinear Dynamics 8: 21–43

    MathSciNet  Google Scholar 

  • Pandiyan R, Bibb J S, Sinha S C 1993 Liapunov-Floquet transformation: Computation and application to periodic systems. InDynamics and vibration of time-varying systems and structures 14th ASME Biennial Conference on Mechanical Vibration and Noise (eds) S C Sinha, R M Evan-Iwanowski (New York: ASME Press) pp 337–348; also inJ. Vibr. Acoust. 118: 209–219

    Google Scholar 

  • Peters D A, Hohenemser K H 1971 Application of the Floquet transition matrix to problems of lifting rotor stability.J. Am. Helicopter Soc. 16: 25–33

    Article  Google Scholar 

  • Poincaré H 1899Les Methodes Nouvelles de la Mécanique Céleste (Paris: Gauthier-Villars)

    MATH  Google Scholar 

  • Rosenblat S, Cohen D S 1980 Periodically perturbed bifurcation I. Simple bifurcation.Studies in Appl. Math. 63: 1–23

    MATH  MathSciNet  Google Scholar 

  • Rosenblat S, Cohen D S 1981 Periodically perturbed bifurcation II. Hopf Bifurcation.Studies in Appl. Math. 65: 95–112

    MathSciNet  Google Scholar 

  • Sanders J A, Verhulst F 1985Averaging methods in nonlinear dynamical systems (New York: Springer-Verlag)

    MATH  Google Scholar 

  • Sethna P R, Schapiro S M 1977 Nonlinear behavior of flutter unstable dynamical systems with gyroscopic and circulatory forces.J. Appl. Mech. 44: 755–762

    MATH  Google Scholar 

  • Seydel R 1981 Numerical computation of periodic orbits that bifurcate from stationary solutions of ordinary differential equation.Appl. Math. Comput. 9: 257–271

    Article  MATH  MathSciNet  Google Scholar 

  • Seydel R 1987 New methods for calculating stability of periodic solutions.Comput. Math. Appl. 14: 505–510

    Article  MATH  MathSciNet  Google Scholar 

  • Seydel R 1988From equilibrium to chaos: Practical bifurcation and stability analysis (New York: Elsevier)

    MATH  Google Scholar 

  • Sinha S C, Joseph P 1994 Control of general dynamic systems with periodically varying parameters via Liapunov-Floquet Transformation.ASME J. Dynamic Syst., Measurements Control 116: 650–658

    MATH  Google Scholar 

  • Sinha S C, Juneja V 1991 An approximate analytical solution for systems with periodic coefficients via symbolic computation.AIAA/ASME/ASCE/AHS/ASC 32nd Structures, Structural Dynamics and Materials Conference (A Collection of Papers, Part 1) pp 790–797

  • Sinha S C, Pandiyan R 1994 Analysis of quasilinear dynamical systems with periodic coefficients via Liapunov-Floquet transformation.Int. J. Non-Linear Mech. 29: 687–702

    Article  MATH  MathSciNet  Google Scholar 

  • Sinha S C, Wu D-H 1991 An efficient computational scheme for the analysis of periodic systems.J. Sound Vibr. 15: 345–375

    MathSciNet  Google Scholar 

  • Sinha S C, Wu D-H, Juneja V, Joseph P 1993a Analysis of dynamic systems with periodically varying parameters via Chebyshev polynomials.ASME J. Vibr. Acoust. 115: 96–102

    Article  Google Scholar 

  • Sinha S C, Senthilnathan N R, Pandiyan R 1993b A new numerical technique for the analysis of parametrically excited nonlinear systems.Nonlinear Dynamics 4: 483–498

    Article  Google Scholar 

  • Stoker J J 1950Nonlinear vibration (New York: Interscience)

    Google Scholar 

  • Wu D-H, Sinha S C 1994 A new approach in the analysis of linear systems with periodic coefficients for applications in rotorcraft dynamics.Aeronaut. J. R. Aeronaut. Soc. January: 9–16

  • Yakubovitch V A, Starzhinskii V M 1975Linear differential equations with periodic coefficients (New York: Wiley-Halsted) vols. 1 and 2

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Nonlinear Systems Research Laboratory, Department of Mechanical Engineering, Auburn University, 36849, AL, USA

    S C Sinha

Authors
  1. S C Sinha
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S C Sinha.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Sinha, S.C. On the analysis of time-periodic nonlinear dynamical systems. Sadhana 22, 411–434 (1997). https://doi.org/10.1007/BF02744481

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02744481

Keywords

Search

Navigation