Abstract
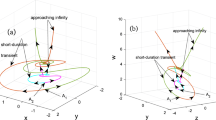
We consider a class of reversible, two-degree of freedom Hamiltonian systems possessing homoclinic orbits to a saddle-center: an equilibrium having two non-zero real and two nonzero imaginary eigenvalues. Under mild nondegeneracy conditions, we construct a two-parameter unfolding and show that there is a countable infinity of “secondary” homoclinic bifurcations in any neighborhood of the original system. We also demonstrate the existence of families of periodic orbits and of shifts on two symbols (horseshoes). The lack of hyperbolicity and the presence of conserved quantities make the analysis somewhat delicate. We discuss specific examples for which the nondegeneracy conditions can be explicitly checked but indicate that this is not always possible. We illustrate our results with numerical work.
Similar content being viewed by others
References
Amick, C. J., and Kirchgässner, K. (1989). A theory of solitary water-waves in the presence of surface tension.Arch. Ration. Mech. Anal. 105, 1–49.
Chow, S.-N., Deng, B., and Fiedler, B. (1990). Homoclinic bifurcation at resonant eigenvalues.J. Dynam. Diff. Eq. 2, 177–244.
Conley, C. C. (1968). Twist mappings, linking, analyticity, and periodic solutions which pass close to an unstable periodic solution. In Auslander, J., and Gottschalk, W. H. (eds.),Topological Dynamics, Benjamin, New York, pp. 129–153.
Conley, C. C. (1969). On the ultimate behavior of orbits with respect to an unstable critical point. I. Oscillating, asymptotic, and capture orbits.J. Diff. Eq. 5, 136–158.
Churchill, R. C., and Rod, D. L. (1980). Pathology in dynamical systems. III. Analytic Hamiltonians.J. Diff. Eq. 37, 23–38.
Churchill, R. C., Pecelli, G., Sacolick, S., and Rod, D. L. (1977). Coexistence of stable and random motion.Rocky Mt. J. Math. 7, 445–456.
Devaney, R. L. (1979). Homoclinic orbits to hyperbolic equilibria. In Gurel, O., and Rössler, O. (eds.),Bifurcation Theory and Applications in Scientific Disciplines, Annals of the New York Academy of Sciences, New York, p. 316.
Devaney, R. L. (1976). Homoclinic orbits in Hamiltonian systems.J. Diff. Eq. 21, 431–438.
Gaspard, P. (1984). Generation of a countable set of homoclinic flows through bifurcation in multidimensional systems.Bull. Class. Sci. Acad. Roy. Belg. Ser. 5 LXX, 61–83.
Glendinning, P. (1989). Subsidiary bifurcations near bifocal homoclinic orbits.Math. Proc. Cambridge Phil. Soc. 105, 597–605.
Guckenheimer, J., and Holmes, P. (1983).Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields, Appl. Math. Sciences Vol. 42, Springer-Verlag, New York.
Holmes, P, J., and Mielke, A. (1988). Spatially complex equilibria of buckled rods.Arch. Ration. Mech. Anal. 101, 319–348.
Johnson, J. M., and Bajaj, A. K. (1989). Amplitude modulated and chaotic dynamics in resonant motion of strings.J. Sound Vibrat. 128, 87–107.
Kokubu, H. (1988). Homoclinic and heteroclinic bifurcations of vector fields.Jap. J. Appl. Math. 5, 455–501.
Lasagni, F. M. (1988). Canonical Runge-Kutta methods.J. Appl. Math. Phys. (ZAMP) 39, 952–953.
Lerman, L. M. (1987). Hamiltonian systems with a separatrix loop of a saddle center. InMethods of the Qualitative Theory of Differential Equations (Russian), Gor'kov, Gos. Univ., Gorki, pp. 89–103 (Math. Rev. 90g: 58 035).
Llibre, J., and Simó, C. (1980). On the Hénon-Heiles potential.Actas del III CEDYA, Santiago de Compostela, pp. 183–206.
Llibre, J., Martínez, R., and Simó, C. (1985). Transversality of the invariant manifolds associated to the Lyapunov family of periodic orbits nearL 2 in the restricted three-body problem.J. Diff. Eq. 58, 104–156.
Miles, J. W. (1984). Resonant, nonplanar motion of a stretched string.J. Acoust. Soc. Am. 75, 1505–1510.
Mielke, A. (1990). Topological methods for discrete dynamical systems.GAMM-Mitteilungen, Heft 2, p. 19–37.
Mielke, A. (1991).Hamiltonian and Lagrangian Flows on Center Manifolds with Applications to Elliptic Variational Problems, Lect. Notes Math., Vol. 1489, Springer-Verlag, New York.
Moser, J. (1958). On the generalization of a theorem of A. Liapunoff.Comm. Pure Appl. Math. 11, 257–271.
Moser, J. (1973).Stable and Random Motions in Dynamical Systems, Princeton University Press, Princeton, N.J.
Moltena, T. C. A., and Tufillaro, N. B. (1990). Torus doubling and chaotic string vibrations: Experimental results.J. Sound Vibrat. 137, 327–330.
O'Reilly, O., (1990).The Chaotic Vibration of a String, Ph.D. thesis, Cornell University, Ithacar, N.Y.
O'Reilly, O., and Holmes, P. (1992). Nonlinear, nonplanar and nonperiodic vibrations of a string.J. Sound Vibrat. (in press).
Pérouème, M. C. (1989). Perturbations de systemes reversibles—dedoublement d'orbites homoclines. Manuscript Université de Nice, Nice, France.
Rüssmann, H. (1964). Über das Verhalten analytischer Hamiltonscher Differentialgleichungen in der Nähe einer Gleichgewichtslösung.Math. Ann. 154, 285–300.
Shil'nikov, L. P. (1970). A contribution to the problem of the structure of an extended neighborhood of a rough equilibrium state of saddle-focus type.Math. USSR Sbornik 10, 91–102.
Turaev, D. V., and Shil'nikov, L. P. (1989). On Hamiltonian systems with homoclinic saddle curves.Soviet Math. Dokl. 39, 165–168.
Wiggins, S. (1988).Global Bifurcations and Chaos, Appl. Math. Sciences Vol. 73, Springer-Verlag, New York.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Mielke, A., Holmes, P. & O'Reilly, O. Cascades of homoclinic orbits to, and chaos near, a Hamiltonian saddle-center. J Dyn Diff Equat 4, 95–126 (1992). https://doi.org/10.1007/BF01048157
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF01048157
Key words
- Homoclinic orbit
- bifurcation
- reversible
- Hamiltonian
- saddle-center
- periodic orbit
- horseshoe
- Shil'nikov phenomena