Abstract
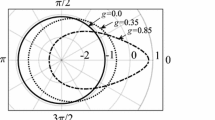
Numerical calculation of photon migration in biological tissue using the radiative transfer equation (RTE) has attracted great interests in biomedical optics and imaging. Because biological tissue is a highly forward-peaked scattering medium, renormalization of the phase function in numerical calculation of the RTE is crucial. This paper proposes a simple approach of renormalizing the phase function by the double exponential formula, which was heuristically modified from the original one. Firstly, the validity of the proposed approach was tested by comparing numerical results for an average cosine of the polar scattering angle calculated by the proposed approach with those by the conventional approach in highly forward-peaked scattering. The results show that calculation of the average cosine converged faster using the proposed approach than using the conventional one as a total number of discrete angular directions increases. Next, the accuracy of the numerical solutions of the RTE using the proposed approach was examined by comparing the numerical solutions with the analytical solutions of the RTE in a homogeneous medium with highly forward-peaked scattering. It was found that the proposed approach reduced the errors of the numerical solutions from those using the conventional one especially at a small value of the total number of the directions. This result suggests that the proposed approach has a possibility to improve the accuracy for the numerical results of the RTE in the highly scattering medium.






Similar content being viewed by others
References
D. Balsara, Fast and accurate discrete ordinates methods for multidimensional radiative transfer. Part I, basic methods. J. Quant. Spectrosc. Radiat. Transf. 69, 671–707 (2001)
P. Boulet, A. Collin, J.L. Consalvi, On the finite volume method and the discrete ordinates method regarding radiative heat transfer in acute forward anisotropic scattering media. J. Quant. Spectrosc. Radiat. Transf. 104, 460–473 (2007)
B.G. Carlson, in Quadrature Tables of Equal Weight EQn Over the Unit sphere. Los Alamos Scientific Laboratory Report 4734 (1971)
S. Chandrasekhar, Radiative Transfer (Dover, New York, 1960)
W.F. Cheong, S.A. Prahl, A.J. Welch, A review of the optical properties of biological tissue. IEEE J. Quantum Electron. 26(12), 2166–2185 (1990)
W.A. Fiveland, The selection of discrete ordinate quadrature sets for anisotropic scattering. ASME, HTD-vol. 160, Fundamentals of radiation heat transfer pp. 89–96 (1991)
H. Fujii, S. Okawa, K. Nadamoto, E. Okada, Y. Yamada, Y. Hoshi, M. Watanabe, Numerical modeling of photon migration in the cerebral cortex of the living rat using the radiative transport equation. Proc. SPIE 2015 9333(933313), 1–5 (2015)
H. Fujii, S. Okawa, K. Nadamoto, E. Okada, Y. Yamada, Y. Hoshi, M. Watanabe, Numerical modeling of photon migration in human neck based on the radiative transport equation. Journal of Applied Nonlinear Dynamics 5(1), 117–125 (2016) eprint arXiv:1603.03769 [physics.med-ph]
H. Fujii, S. Okawa, Y. Yamada, Y. Hoshi, Hybrid model of light propagation in random media based on the time-dependent radiative transfer and diffusion equations. J. Quant. Spectrosc. Radiat. Transf. 147, 145–154 (2014)
A.P. Gibson, J.C. Hebden, S.R. Arridge, Recent advances in diffuse optical imaging. Phys. Med. Biol. 50(4), R1–R43 (2005)
S. Gottlieb, C.W. Shu, Total variation diminishing Runge–Kutta schemes. Math. Comput. 67(221), 73–85 (1998)
W. Han, J.A. Eichholz, G. Wang, On a family of differential approximations of the radiative transfer equation. J. Math. Chem. 50, 689–702 (2012)
L.G. Henyey, L.J. Greenstein, Diffuse radiation in the galaxy. J. Astrophys. 93, 70–83 (1941)
B. Hunter, Z. Guo, Conservation of asymmetry factor in phase function discretization for radiative transfer analysis in anisotropic scattering media. Int. J. Heat Mass Transf. 55, 1544–1552 (2012)
J.H. Joseph, W.J. Wiscombe, J.A. Weinman, The delta-Eddington approximation for radioactive flux transfer. J. Atmos. Sci. 33, 2452–2459 (1976)
A.D. Klose, U. Netz, J. Beuthan, A.H. Hielscher, Optical tomography using the time-independent equation of radiative transfer Part 1: forward model. J. Quant. Spectrosc. Radiat. Transf. 72, 691–713 (2002)
L. Liu, L. Ruan, H. Tan, On the discrete ordinates method for radiative heat transfer in anisotropically scattering media. Int. J. Heat Mass Transf. 45(15), 3259–3262 (2002)
F. Martelli, A. Sassaroli, A. Pifferi, A. Torricelli, L. Spinelli, G. Zaccanti, Heuristic Green’s function of the time dependent radiative transfer equation for a semi-infinite medium. Opt. Express 15(26), 18168–18175 (2007)
L.D. Montejo, A.D. Klose, A.H. Hielscher, Implementation of the equation of radiative transfer on block-structured grids for modeling light propagation in tissue. Biomed. Opt. Express 1(3), 861–878 (2010)
M. Mori, M. Sugihara, The double-exponential transformation in numerical analysis. J. Comput. Appl. Math. 127, 287–296 (2001)
H. Ning, T.E. Simos, A low computational cost eight algebraic order hybrid method with vanished phase-lag and its first, second, third and fourth derivatives for the approximate solution of the Schrödinger equation. J. Math. Chem. 53(6), 1295–1312 (2015)
J.C.J. Paasschens, Solution of the time-dependent Boltzmann equation. Phys. Rev. E 56(1), 1135–1141 (1997)
D.F. Papadopoulos, T.E. Simos, A modified Runge–Kutta–Nyström method by using phase lag properties for the numerical solution of orbital problems. Appl. Math. Inf. Sci. 7(2), 433–437 (2013)
T.E. Simos, Closed Newton–Cotes trigonometrically-fitted formulae of high order for long-time integration of orbital problems. Appl. Math. Lett. 22, 1616–1621 (2009)
H. Takahasi, M. Mori, Double exponential formulas for numerical indefinite integration. Publ. RIMS Kyoto Univ. 9, 721–741 (1974)
H. Zheng, W. Han, On simplified spherical harmonics equations for the radiative transfer equation. J. Math. Chem. 49, 1785–1797 (2011)
Acknowledgments
This work was partially supported by JSPS KAKENHI Grant No.15K17980, 15K06125, and 25287028; and by Japan Agency for Medical Research and Development.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Fujii, H., Okawa, S., Yamada, Y. et al. Renormalization of the highly forward-peaked phase function using the double exponential formula for radiative transfer. J Math Chem 54, 2048–2061 (2016). https://doi.org/10.1007/s10910-016-0670-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10910-016-0670-3
Keywords
- Radiative transfer equation
- Renormalization approach of the phase function
- Highly forward-peaked scattering media
- Double-exponential formula