Skip to main content
SpringerLink
Log in

Method for Generating Asynchronous Self-Sustained Oscillations of a Mechanical System with Two Degrees of Freedom

  • Published:
Mechanics of Solids Aims and scope Submit manuscript

Abstract

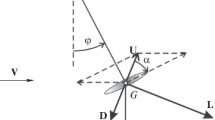
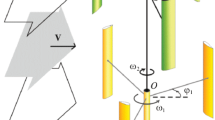
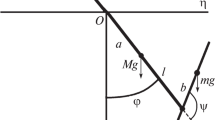
An autonomous nonconservative mechanical system with two degrees of freedom is studied. The system is subject to a feedback control with two control impact gain factors. It is required to select the values of these factors in such a way as to ensure existence of asynchronous self-sustained oscillations with prescribed properties. An iterative method is proposed to search for the corresponding values of control impact gain factors. This approach is based on constructing auxiliary second-order systems and generating limit cycles in these systems. The algorithm that is used for this purpose represents a modification of the Andronov–Pontryagin method, but does not require the presence of a small parameter in the system. The efficiency of this approach is illustrated on a problem of generating asynchronous self-sustained oscillations/rotations in a model of an aerodynamic pendulum. The applicability conditions of the algorithm and the possible modifications are discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

REFERENCES

  1. A. A. Andronov, “Les cycles limites de Poincaré et la théorie des oscillations auto-entretenues,” C. R. Seances Acad. Sci. 189, 559–561 (1929).

    Google Scholar 

  2. L. S. Pontryagin, “On dynamical systems close to Hamiltonian systems,” Zh. Eksp. Teor. Fiz. 4 (9), 883–885 (1934).

    Google Scholar 

  3. A. D. Morozov, Resonances, Cycles and Chaos in Quasi-Conservative Systems (Regular and Chaotic Dynamics, Izhevsk, Moscow, 2005) [in Russian].

    Google Scholar 

  4. S. A. Korolev and A. D. Morozov, “On periodic perturbations of self-oscillating pendulum equations,” Russ. J. Nonlinear Dyn. 6 (1), 79–89 (2010).

    Google Scholar 

  5. M. Bonnin, “Existence, number, and stability of limit cycles in weakly dissipative, strongly nonlinear oscillators,” Nonlinear Dyn. 62 (1-2), 321–332 (2010).

    Article  MathSciNet  MATH  Google Scholar 

  6. A. D. Morozov and O. S. Kostromina, “On periodic perturbations of asymmetric Duffing-van-der-Pol equation,” Int. J. Bifurcation Chaos 24 (5), 1450061 (2014).

    Article  MathSciNet  MATH  ADS  Google Scholar 

  7. L. Gavrilov and I. D. Iliev, “Perturbations of quadratic Hamiltonian two-saddle cycles,” Ann. Inst. Henri Poincare (C) Non Linear Anal. 32 (2), 307–324 (2015).

    MATH  Google Scholar 

  8. V. N. Tkhai, “Stabilizing the oscillations of a controlled mechanical system,” Autom. Remote Control 80 (11), 1996–2004 (2019).

    Article  MathSciNet  MATH  Google Scholar 

  9. L. A. Klimina, “Rotational modes of motion for an aerodynamic pendulum with a vertical rotation axis,” Moscow Univ. Mech. Bull. 64 (5), 126–129 (2009).

    Article  Google Scholar 

  10. L. A. Klimina and B. Ya. Lokshin, “On a constructive method of search for rotary and oscillatory modes in autonomous dynamical systems,” Russ. J. Nonlinear Dyn. 13 (1), 25–40 (2017).

    MATH  Google Scholar 

  11. L. A. Klimina, B. Y. Lokshin, and V. A. Samsonov, “Bifurcation diagram of the self-sustained oscillation modes for a system with dynamic symmetry,” J. Appl. Math. Mech. 81 (6), 442–449 (2017).

    Article  MathSciNet  MATH  Google Scholar 

  12. A. D. Morozov and E. L. Fedorov, “On the investigation of equations with one degree of freedom, close to nonlinear integrable ones,” Differ. Uravn. 19 (9), 1511–1516 (1983).

    MathSciNet  Google Scholar 

  13. I. A. Khovanskaya (Pushkar’), “Weak infinitesimal Hilbert’s 16th problem,” Proc. Steklov Inst. Math. 254 (1), 201–230 (2006).

    Article  MathSciNet  MATH  Google Scholar 

  14. A. M. Samoilenko, “Numerical analytical method of investigating periodic systems of ordinary differential equations. I,” Ukr. Mat. Zh. 17 (4), 82–93 (1965).

    Google Scholar 

  15. M. I. Rontó, A. M. Samoilenko, and S. I. Trofimchuk, “The theory of the numerical-analytic method: achievements and new trends of development. IV,” Ukr. Math. J. 50 (12), 1888–1907 (1998).

    Article  MATH  Google Scholar 

  16. L. A. Klimina, “Method for finding periodic trajectories of centrally symmetric dynamical systems on the plane,” Differ. Equations 55 (2), 159–168 (2019).

    Article  MathSciNet  MATH  Google Scholar 

  17. L. A. Klimina and Y. D. Selyutskiy, “Method to construct periodic solutions of controlled second-order dynamical systems,” J. Comput. Syst. Sci. Int. 58 (4), 503–514 (2019).

    Article  MATH  Google Scholar 

  18. L. A. Klimina, “Method for constructing periodic solutions of a controlled dynamic system with a cylindrical phase space,” J. Comput. Syst. Sci. Int. 59 (2), 139–150 (2020).

    Article  MATH  Google Scholar 

  19. F. Schilder, H. M. Osinga, and W. Vogt, “Continuation of quasi-periodic invariant tori,” SIAM J. Appl. Dyn. Syst. 4 (3), 459–488 (2005).

    Article  MathSciNet  MATH  ADS  Google Scholar 

  20. K. Kamiyama, M. Komuro, and T. Endo, “Bifurcation of quasi-periodic oscillations in mutually coupled hard-type oscillators: demonstration of unstable quasi-periodic orbits,” Int. J. Bifurcation Chaos 22 (6), 1230022 (2012).

    Article  MATH  ADS  Google Scholar 

  21. J. Bush, M. Gameiro, S. Harker, H. Kokubu, K. Mischaikow, I. Obayashi, and P. Pilarczyk, “Combinatorial-topological framework for the analysis of global dynamics,” Chaos: Int. J. Nonlinear Sci. 22 (4), 047508 (2012).

    Article  MathSciNet  MATH  Google Scholar 

  22. K. Kamiyama, M. Komuro, and T. Endo, “Algorithms for obtaining a saddle torus between two attractors,” Int. J. Bifurcation Chaos 23 (9), 1330032 (2013).

    Article  MathSciNet  MATH  ADS  Google Scholar 

  23. B. Zhou, F. Thouverez, and D. Lenoir, “A variable-coefficient harmonic balance method for the prediction of quasi-periodic response in nonlinear systems,” Mech. Syst. Signal Proc. 64, 233–244 (2015).

    Article  ADS  Google Scholar 

  24. G. Chen and J. F. Dunne, “A fast continuation scheme for accurate tracing of nonlinear oscillator frequency response functions,” J. Sound Vib. 385, 284–299 (2016).

    Article  ADS  Google Scholar 

  25. I. N. Barabanov and V. N. Tkhai, “Designing a stable cycle in weakly coupled identical systems,” Autom. Remote Control 78 (2), 217–223 (2017).

    Article  MathSciNet  MATH  Google Scholar 

  26. L. A. Klimina, “Method for constructing self-sustained rotations of a controlled mechanical system with two degrees of freedom,” J. Comput. Syst. Sci. Int. 59 (6), 817–827 (2020).

    Article  MATH  Google Scholar 

  27. L. A. Klimina, A. A. Masterova, V. A. Samsonov, and Y. D. Selyutskiy, “ Numerical–Analytical method for searching for the autorotations of a mechanical system with two rotational degrees of freedom,” Mech. Solids 56 (3), 392–403 (2021) (in press).

  28. S. A. Campbell, I. Ncube, and J. Wu, “Multistability and stable asynchronous periodic oscillations in a multiple-delayed neural system,” Phys. D: Nonlinear Phenom. 214 (2), 101–119 (2006).

    Article  MathSciNet  MATH  ADS  Google Scholar 

  29. A. S. Kosmodamianskii, V. I. Vorobiev, and A. A. Pugachev, “The temperature effect on the performance of a traction asynchronous motor,” Russ. Electr. Eng. 82 (8), 445–448 (2011).

    Article  Google Scholar 

  30. A. A. Grin’ and S. V. Rudevich, “Dulac-Cherkas test for determining the exact number of limit cycles of autonomous systems on the cylinder,” Differ. Equations 55 (3), 319–327 (2019).

    Article  MathSciNet  MATH  Google Scholar 

  31. A. A. Grin’, “Transversal curves for finding the exact number of limit cycles,” Differ. Equations 56 (4), 415–425 (2020).

    Article  MathSciNet  MATH  Google Scholar 

  32. Z. D. Georgiev, I. M. Uzunov, and T. G. Todorov, “Analysis and synthesis of oscillator systems described by a perturbed double-well duffing equation,” Nonlinear Dyn. 94 (1), 57–85 (2018).

    Article  MATH  Google Scholar 

  33. A. A. Andronov, E. A. Leontovich, I. I. Gordon, and A. G. Maier, Theory of Dynamic System Bifurcations on a Plane (Nauka, Moscow, 1967) [in Russian].

    Google Scholar 

  34. A. M. Formalskii, Stabilization and Motion Control of Unstable Objects (Walter de Gruyter, Berlin, Boston, 2015).

    Book  MATH  Google Scholar 

  35. L. A. Klimina and A. M. Formalskii, “Three-link mechanism as a model of a person on a swing,” J. Comput. Syst. Sci. Int. 59 (5), 728–744 (2020).

    Article  MATH  Google Scholar 

  36. A. Y. Aleksandrov and A. A. Tikhonov, “Averaging technique in the problem of Lorentz attitude stabilization of an earth-pointing satellite,” Aerospace Sci. Technol. 104, 105963 (2020).

    Article  Google Scholar 

  37. V. I. Kalenova and V. M. Morozov, “Novel approach to attitude stabilization of satellite using geomagnetic Lorentz forces,” Aerospace Sci. Technol. 106, 106105 (2020).

    Article  Google Scholar 

  38. P. Ashwin and O. Burylko, “Weak chimeras in minimal networks of coupled phase oscillators,” Chaos: Interdiscip. J. Nonlinear Sci. 25 (1), 013106 (2015).

    Article  MathSciNet  MATH  Google Scholar 

  39. Y. D. Selyutskiy, A. P. Holub, and M. Z. Dosaev, “Elastically mounted double aerodynamic pendulum,” Int. J. Struct. Stab. Dyn. 19 (5), 1941007-1–1941007-13 (2019). https://doi.org/10.1142/S0219455419410074

  40. B. Ya. Lokshin, V. A. Privalov, and V. A. Samsonov, Introduction to the Problem of a Body Moving in a Resistant Medium (MSU, Moscow, 1986) [in Russian].

    MATH  Google Scholar 

  41. B. Y. Lokshin and V. A. Samsonov, “The self-induced rotational and oscillatory motions of an aerodynamic pendulum,” J. Appl. Math. Mech. 77 (4), 360–368 (2013).

    Article  MathSciNet  MATH  Google Scholar 

  42. V. A. Samsonov and Y. D. Seliutski, “Phenomenological model of interaction of a plate with a flow,” J. Math. Sci. 146 (3), 5826–5839 (2007).

    Article  MathSciNet  MATH  Google Scholar 

  43. L. Borkowski, P. Perlikowski, T. Kapitaniak, and A. Stefanski, “Experimental observation of three-frequency quasiperiodic solution in a ring of unidirectionally coupled oscillators,” Phys. Rev. E 91 (6), 062906 (2015).

    Article  ADS  Google Scholar 

  44. A. D. Bruno, Local Method of Nonlinear Analysis of Differential Equations (Springer-Verlag, Berlin, Heidelberg, 1989).

    Book  Google Scholar 

  45. A. D. Bruno, Power Geometry in Algebraic and Differential Equations (Elsevier, Amsterdam, 2000).

    Google Scholar 

Download references

Funding

The study is supported by the Interdisciplinary Scientific and Educational School of Moscow University “Mathematical Methods of Complex Systems’ Analysis.”

Author information

Authors and Affiliations

  1. Institute of Mechanics, Lomonosov Moscow State University, 119192, Moscow, Russia

    L. A. Klimina

Authors
  1. L. A. Klimina
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to L. A. Klimina.

Additional information

Translated by E. Oborin

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Klimina, L.A. Method for Generating Asynchronous Self-Sustained Oscillations of a Mechanical System with Two Degrees of Freedom. Mech. Solids 56, 1167–1180 (2021). https://doi.org/10.3103/S0025654421070141

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.3103/S0025654421070141

Keywords:

Search

Navigation