Abstract
In this paper, we study the existence of periodic solutions for Rayleigh equation with a singularity of repulsive type
where \(\alpha \geqslant 1\) is a constant, and φ and p are T-periodic functions. The proof of the main result relies on a known continuation theorem of coincidence degree theory. The interesting point is that the sign of the function \(\varphi (t)\) is allowed to change for \(t\in [0,T]\).
Similar content being viewed by others
1 Introduction
Singular differential equations arise in many disciplines such as physics, fluid dynamics, and ecology (see [1–6] and the references therein). In recent years, the periodic problem of second-order differential equations with singularities has been widely studied. The first study in this area seems to be the paper of Nagumo [7] in 1944. After some works of Forbat and Huaux [8], the interest increased with the pioneering paper of Lazer and Solimini [9]. They considered the existence of periodic solutions suggested by the two fundamental examples (\(\alpha >0\), and \(h:R\rightarrow R\) is a continuous T-periodic function)
(the singularity of attractive type) and
(the singularity of repulsive type). By using topological degree methods they obtained that a necessary and sufficient condition for the existence of positive periodic solutions for equation (1.1) is \(\overline{h}>0\), and if we assume in addition that \(\alpha \ge 1\), then a necessary and sufficient condition for the existence of positive periodic solutions for equation (1.2) is \(\overline{h}<0\). After that, some methods associated with nonlinear functional analysis theory have been widely applied to the studied problem in many papers such as the variational methods used in [10–13], fixed point theorems used in [14–19], upper and lower solutions methods used in [20, 21], and continuation theorems of coincidence degree used in [22–31]. For example, Torres [14] studied the periodic problem for the equation with singularity of repulsive type
where \(\varphi,b,h\in L^{1}[0,T]\), and \(\mu >0\) is a constant. The function φ is required to satisfy
This is due to the fact that (1.4), together with some other conditions, can guarantee the Green function \(G(t,s)\) associated with the boundary value problem for Hill’s equation
satisfying \(G(t,s)\ge 0\) for all \((t,s)\in [0,T]\times [0,T]\); then, the solution to problem (1.5) is given by
Formula (1.6) is crucial in [14–17] for applying some fixed point theorems on cones. Wang [25] studied the problem of periodic solutions for the singular delay Liénard equation of repulsive type
where \(f:[0,+\infty)\rightarrow R\) is continuous, \(\varphi:R\rightarrow R\) is continuous T-periodic, and \(\tau >0\) and \(\mu \ge 1\) are constants. To balance the forces of \(\varphi (t)x\) at \(x=+\infty \) and \(\frac{1}{x^{\mu }}\) at \(x=0\), φ is also required to satisfy
In [26, 28], the authors studied the periodic problem of the equation
In (1.9), the function φ is required to satisfy \(\int_{0}^{T}\varphi (s)\,ds>0\), which means that the sign of the function φ is allowed to change. Now, the question is that how to investigate the existence of T-periodic solutions for a Rayleigh equation with a singularity of repulsive type
where \(f:R\rightarrow R\) is continuous with \(f(0)=0\), \(\alpha \geq 1\), and φ, \(p:R\rightarrow R\) are continuous and T-periodic.
Motivated by this, the aim of this paper is to search for positive T-periodic solutions for (1.10). Using a known continuation theorem of theorem of coincidence degree theory (see [32, 33], and [34]), we obtain a new result on the existence of positive periodic solutions for equation (1.10). In present paper, the sign of φ in (1.10) is allowed to change for \(t\in [0,T]\). Although this condition is the same as that in [26, 28], for studying the periodic problem of (1.9), the methods used in [26, 28] for estimating a priori bounds of positive T-periodic solutions to (1.9) cannot be directly applied to (1.10). This is due to the fact that mechanism of the first-order derivative term \(f(x'(t))\) influencing a priori bounds of positive T-periodic solutions to (1.10) is different from the corresponding ones of \(f(x(t))x'(t)\) in (1.10). For example, if \(x(t)\) is a positive T-periodic function such that \(x\in C^{1}(R,R)\), then \(\int_{0}^{T}f(x(t))x'(t)\,dt=0\), but, generally, \(\int_{0}^{T}f(x'(t))\,dt \neq 0\).
2 Preliminary lemmas
Let \(C_{T}=\{x\in C(R,R):x(t+T)=x(t), \forall t\in R\}\) with the norm \(\vert x\vert _{\infty }=\max_{t\in [0,T]}\vert x(t)\vert \), and let \(C^{1}_{T}=\{x' \in C^{1}(R,R):x'(t+T)=x'(t), \forall t\in R\}\) with the norm \(\Vert x\Vert =\max \{\vert x\vert _{\infty },\vert x'\vert _{\infty }\}\). Clearly, \(C_{T}\) and \(C_{T}^{1}\) are both Banach spaces. For any T-periodic solution \(\varphi (t)\) with \(\varphi \in C_{T}\), by \(\varphi_{+}(t)\) and \(\varphi_{-}(t)\) we denote \(\max \{\varphi (t),0\}\) and \(-\min \{ \varphi (t),0\}\), respectively, and \(\overline{\varphi }=\frac{1}{T} \int^{T}_{0}\varphi (s)\,ds\). Then \(\varphi (t)=\varphi_{+}(t)-\varphi _{-}(t)\) for all \(t\in R\), and \(\overline{\varphi }=\overline{\varphi _{+}}-\overline{\varphi_{-}}\). Furthermore, for each \(u\in C_{T}\), let \(\Vert u\Vert _{p}:=(\int_{0}^{T}\vert u(s)\vert ^{p}\,ds)^{1/p}\), \(p\in [1,+\infty)\).
The following result can be easily obtained by using Theorem 4 in [32], Chapter 6 of [33], and Theorem 3.1 in [34].
Lemma 2.1
Assume that there exist positive constants \(N_{0}\), \(N_{1}\), and \(N_{2}\) with \(0< N_{0}< N_{1}\) such that the following conditions hold.
-
1.
For each \(\lambda \in (0,1]\), each possible positive T-periodic solution x to the equation
$$u''+\lambda f\bigl(u'\bigr)+\lambda \varphi (t)u-\frac{\lambda }{u^{\alpha }}= \lambda p(t) $$satisfies the inequalities \(N_{0}< x(t)< N_{1}\) and \(\vert x'(t)\vert < N_{2}\) for all \(t\in [0,T]\).
-
2.
Each possible solution c to the equation
$$\frac{1}{c^{\alpha }}-c\overline{\varphi }+\overline{p}=0 $$satisfies the inequality \(N_{0}< c< N_{1}\).
-
3.
The inequality
$$\biggl(\frac{1}{N_{0}^{\alpha }}-N_{0}\overline{\varphi }+\overline{p} \biggr) \biggl(\frac{1}{N_{1}^{\alpha }}-N_{1}\overline{\varphi }+ \overline{p} \biggr)< 0 $$holds.
Then equation (1.10) has at least one positive T-periodic solution u such that \(N_{0}< u(t)< N_{1}\) for all \(t\in [0,T]\).
Now, we list the following assumptions, which will be used in Section 3 for investigating the existence of positive T-periodic solutions to (1.10).
- \({[H_{1}]}\) :
-
There exist constants \(L>0\), \(\sigma >0\), and \(n\geq 1\) such that
$$ \biggl\vert \int^{T}_{0}f\bigl(x'(t)\bigr)\,dt \biggr\vert \leq L \int^{T}_{0}\bigl\vert x'(t)\bigr\vert \,dt,\quad \forall x\in C^{1}_{T} $$(2.1)and
$$ yf(y)\geq \sigma \vert y\vert ^{n+1}, \quad \forall y\in R. $$(2.2) - \({[H_{2}]}\) :
-
The function φ satisfies \(\overline{\varphi_{+}}>\overline{ \varphi_{-}}\);
- \({[H_{3}]}\) :
-
\(\Vert \varphi \Vert _{2}<\sigma T^{-\frac{1}{2}}\) and \((LT^{- \frac{1}{2}}+T^{\frac{1}{2}}\overline{\varphi_{+}})\Vert \varphi \Vert _{2} < \sigma (\overline{\varphi_{+}}-\overline{\varphi_{-}})\).
Remark 2.1
If assumption \([H_{2}]\) holds, then there are constants \(D_{1}\) and \(D_{2}\) with \(0< D_{1}< D_{2}\) such that
and
Now, we embed equation (1.10) into the following equations family with parameter \(\lambda \in (0,1]\):
Let
and let
where B will be determined by (2.13). Clearly, \(M_{0}\) is independent of \((\lambda,x)\in (0,1]\times \Omega \).
Lemma 2.2
Assume that assumptions \([H_{1}]\)-\([H_{3}]\) hold. Then for each function \(x\in \Omega \), there exists a point \(t_{0}\in [0,T]\) such that
where \(M_{0}\) is defined by (2.5)
Proof
If the conclusion does not hold, then there is a function \(x_{0}\in \Omega \) satisfying
From (2.4) we get
Integrating (2.7) over the interval \([0,T]\), we get
that is,
Since \(\varphi_{+}(t)\geq 0\) and \(\varphi_{-}(t)\geq 0\) for all \(t\in [0,T]\), it follows from the integral mean value theorem and condition (2.1) in \([H_{1}]\) that there are two points \(\xi,\zeta \in [0,T]\) such that
which, together with the fact of \(M_{0}\ge 1\) in (2.5), yields
that is,
Since
it follows from (2.8), (2.9), and \([H_{2}]\) that
On the other hand, multiplying both sides of (2.7) by \(x_{0}'(t)\) and integrating it over the interval \([0,T]\), we get
From condition (2.2) in \([H_{1}]\) we have
that is,
We infer from (2.10) and (2.11) that
According to (2.12), we list two cases.
- Case 1::
-
If \(n>1\), then we see that there exists \(B_{0}>0\) such that \((\int^{T}_{0}\vert x_{0}'(t)\vert ^{n+1}\,dt)^{\frac{1}{n+1}}\leq B_{0}\);
- Case 2::
-
If \(n=1\), then by assumption \([H_{3}]\) there exists \(B_{1}>0\) such that \((\int^{T}_{0}\vert x_{0}'(t)\vert ^{2}\,dt)^{ \frac{1}{2}}\leq B_{1}\).
Letting \(B=\max \{B_{0},B_{1}\}\), it follows from Case 1 or Case 2 that
Substituting (2.13) into (2.10), we have
By the definition of \(M_{0}\) in (2.5) we have
that is,
which contradicts (2.6). This contradiction proves Lemma 2.2. □
Lemma 2.3
Assume that \([H_{2}]\) holds. Then there exists a positive constant \(\gamma >0\) such that, for each \(x\in \Omega \), there is a point \(t_{1}\in [0,T]\) satisfying
Proof
Let \(x(t_{1})=\max_{t\in [0,T]}x(t)\). Then \(x''(t_{1}) \leq 0\) and \(x'(t_{1})=0\), which, together with (2.3), yields
Since \(f(0)=0\), we have
Multiplying both sides of (2.14) by \(x^{\alpha }(t_{1})\), we get
Set \(S(u)=u^{\alpha +1}\max \varphi (t)+u^{\alpha }\vert p\vert _{\infty }-1\) for \(u\in [0,+\infty)\). By assumption \([H_{2}]\) we have
So \(S(u)\) has zero points on \((0,+\infty)\). Let γ be the minimum zero point of \(S(u)\) on \((0,+\infty)\). Then \(S(\gamma)=0\). It follows from (2.15) that
The proof is complete. □
3 Main result
Theorem 3.1
Assume that \([H_{1}]\)-\([H_{3}]\) hold. Then equation (1.10) has at least one positive T-periodic solution.
Proof
Firstly, we will show that there exist \(N_{1}>0\) and \(N_{2}>0\) such that each positive T-periodic solution \(x(t)\) of equation (2.3) satisfying
Suppose that x is an arbitrary positive T-periodic solution of equation (2.3). Then
This implies that \(x\in \Omega \). So by Lemma 2.2 there exists a point \(t_{0}\in [0,T]\) such that
and then
Integrating (3.2) over the interval \([0,T]\), we get
On the other hand, similarly to the proof of (2.11), we have
Substituting (3.3) into (3.5), we have
According to (3.6), we list two cases.
- Case 1::
-
If \(n>1\), then there exists \(\rho_{0}>0\) such that \((\int ^{T}_{0}\vert x'(t)\vert ^{n+1}\,dt)^{\frac{1}{n+1}}\leq \rho_{0}\);
- Case 2::
-
If \(n=1\), then by assumption \([H_{3}]\) there exists \(\rho_{1}>0\) such that \((\int^{T}_{0}\vert x'(t)\vert ^{2}\,dt)^{ \frac{1}{2}}\leq \rho_{1}\).
Letting \(\rho =\max \{\rho_{0},\rho_{1}\}\), it follows from Case 1 or Case 2 that
and according to (3.3), we have
Clearly, there is a point \(t_{2}\in [0,T]\) such that \(x'(t_{2})=0\). Multiplying both sides of (3.2) by \(x'(t)\) and integrating it over the interval \([t_{2},t]\), we get
and then
Since
it follows from (3.9) that
that is,
which implies that
On the other hand, from (3.4) and condition (2.1) in \([H_{1}]\) we have
where ρ is determined in (3.7). Substituting this formula into (3.10), we obtain
So we have
We further show that there exists a constant \(\gamma_{0}\in (0,\gamma)\) such that each positive T= periodic solution of (2.3) satisfies
In fact, suppose that \(x(t)\) is an arbitrary positive T-periodic solution of (2.3). Then
By Lemma 2.3 we see that there is a point \(t_{1}\in [0,T]\) such that
For \(t\in [t_{1},t_{1}+T]\), multiplying both sides of (3.14) with \(x'(t)\) and integrating it over the interval \([t_{1},t]\) (or \([t,t_{1}]\)), we get
which results in
that is,
According to (2.2) in \([H_{1}]\), we get \(\int^{t}_{t_{1}}f(x'(s))x'(s)\,ds \ge 0\). Thus, it follows from the last formula that
which, together with (3.8) and (3.11), yields
that is,
Since \(\alpha \ge 1\), it follows that there exists \(\gamma_{0}\in (0, \gamma)\) such that
which, together with (3.15), implies that
So (3.13) holds.
Let \(n_{0}=\min \{D_{1},\gamma_{0}\}\) and \(n_{1}\in (N_{1}+D_{2},+ \infty)\) be two constants. Then from (3.8), (3.12), and (3.13) we see that each possible positive T-periodic solution x to (2.3) satisfies
This implies that condition 1 and condition 2 of Lemma 2.1 hold. In addition, from Remark 2.1 we can infer that
and
which results in
Therefore, condition 3 of Lemma 2.1 holds. Thus, by Lemma 2.1 we see that equation (1.10) has at least one positive T-periodic solution. The proof is complete. □
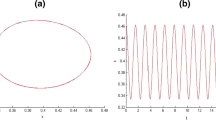
Example 3.1
Consider the equation
where \(a\in (0,\infty)\). Corresponding to (1.10), we see that \(f(x)=10x-\frac{x^{3}}{1+x^{2}}\), \(\varphi (t)=a(1+2\sin t)\), \(p(t)=\cos t\), and \(T=2\pi \).
Firstly, from (3.16) we see that \(f(0)=0\) and
Obviously, \([H_{2}]\) is satisfied. Secondly, integrating \(f(x')\) over the internal \([0,T]\), we get
which implies that we can chose \(L=1\) such that assumption \([H_{1}]\) holds. Besides, from
we see that the constant σ can be chosen as \(\sigma =9\) such that assumption \([H_{1}]\) is satisfied. Last, let \(L=1\), \(\sigma =9\), \(n=1\). Then we get
If
then \([H_{3}]\) holds. Thus, by Theorem 3.1 we have that equation (3.16) has at least one positive 2π-periodic solution.
References
Yuan, H, Xu, X: Existence and uniqueness of solutions for a class of non-Newtonian fluids with singularity and vacuum. J. Differ. Equ. 245, 2871-2916 (2008)
Jebelean, P, Mawhin, J: Periodic solutions of singular nonlinear differential perturbations of the ordinary p-Laplacian. Adv. Nonlinear Stud. 2(3), 299-312 (2002)
Bevc, V, Palmer, JL, Süsskind, C: On the design of the transition region of axi-symmetric magnetically focused beam valves. J. Br. Inst. Radio Eng. 18, 696-708 (1958)
Ye, Y, Wang, X: Nonlinear differential equations in electron beam focusing theory. Acta Math. Appl. Sin. 1, 13-41 (1978) [In Chinese]
Huang, J, Ruan, S, Song, J: Bifurcations in a predator-prey system of Leslie type with generalized Holling type III functional response. J. Differ. Equ. 257(6), 1721-1752 (2014)
Plesset, MS: The dynamics of cavitation bubbles. J. Appl. Mech. 16, 228-231 (1949)
Nagumo, M: On the periodic solution of an ordinary differential equation of second order. In: Zenkoku Shijou Suugaku Danwakai, pp. 54-61. Springer, Berlin (1944) (in Japanese). English translation in Mitio Nagumo collected papers, 1993
Forbat, F, Huaux, A: Détermination approchée et stabilité locale de la solution périodique d’une équation différentielle non linéaire. Mém. Public. Soc. Sci., Artts Lettres Hainaut 76, 3-13 (1962)
Lazer, AC, Solimini, S: On periodic solutions of nonlinear differential equations with singularities. Proc. Am. Math. Soc. 99, 109-114 (1987)
Tanaka, K: A note on generalized solutions of singular Hamiltonian systems. Proc. Am. Math. Soc. 122, 275-284 (1994)
Terracini, S: Remarks on periodic orbits of dynamical systems with repulsive singularities. J. Funct. Anal. 111, 213-238 (1993)
Gaeta, S, Manásevich, R: Existence of a pair of periodic solutions of an ode generalizing a problem in nonlinear elasticity via variational methods. J. Math. Anal. Appl. 123, 257-271 (1988)
Fonda, A: Periodic solutions for a conservative system of differential equations with a singularity of repulsive type. Nonlinear Anal. 24, 667-676 (1995)
Torres, PJ: Weak singularities may help periodic solutions to exist. J. Differ. Equ. 232, 277-284 (2007)
Jiang, D, Chu, J, Zhang, M: Multiplicity of positive periodic solutions to superlinear repulsive singular equations. J. Differ. Equ. 211, 282-302 (2005)
Chu, J, Torres, PJ, Zhang, M: Periodic solutions of second order non-autonomous singular dynamical systems. J. Differ. Equ. 239, 196-212 (2007)
Li, X, Zhang, Z: Periodic solutions for second order differential equations with a singular nonlinearity. Nonlinear Anal. 69, 3866-3876 (2008)
Cheng, Z, Ren, J: Multiplicity results of positive solutions for fourth-order nonlinear differential equation with singularity. Math. Methods Appl. Sci. 38, 5284-5304 (2016)
Cheng, Z, Ren, J: Positive solutions for fourth-order singular nonlinear differential equation with variable-coefficient. Math. Methods Appl. Sci. 39, 2251-2274 (2016)
Hakl, R, Torres, PJ, Zamora, M: Periodic solutions of singular second order differential equations: upper and lower functions. Nonlinear Anal. 74, 7078-7093 (2011)
Hakl, R, Torres, PJ: On periodic solutions of second-order differential equations with attractive-repulsive singularities. J. Differ. Equ. 248, 111-126 (2010)
Zhang, M: Periodic solutions of Liénard equations with singular forces of repulsive type. J. Math. Anal. Appl. 203(1), 254-269 (1996)
Martins, R: Existence of periodic solutions for second-order differential equations with singularities and the strong force condition. J. Math. Anal. Appl. 317, 1-13 (2006)
Lu, S: Homoclinic solutions for a class of prescribed mean curvature Liénard equations. Adv. Differ. Equ. 2015, 239 (2015). https://doi.org/10.1186/s13662-015-0579-3
Wang, Z: Periodic solutions of Liénard equation with a singularity and a deviating argument. Nonlinear Anal., Real World Appl. 16(1), 227-234 (2014)
Hakl, R, Torres, PJ, Zamora, M: Periodic solutions of singular second order differential equations: the repulsive case. Topol. Methods Nonlinear Anal. 39, 199-220 (2012)
Lu, S, Kong, F: Periodic solutions for a kind of prescribed mean curvature Liéard equation with a singularity and a deviating argument. Adv. Differ. Equ. 2015, 151 (2015). https://doi.org/10.1186/s13662-015-0474-y
Lu, S: A new result on the existence of periodic solutions for Liénard equations with a singularity of repulsive type. J. Inequal. Appl. 2017, 37 (2017). https://doi.org/10.1186/s13660-016-1285-8
Lu, S, Zhong, T, Chen, L: Periodic solutions for p-Laplacian Rayleigh equations with singularities. Bound. Value Probl. 2016, 96 (2016). https://doi.org/10.1186/s13661-016-0605-8
Lu, S, Zhong, T, Gao, Y: Periodic solutions of p-Laplacian equations with singularities. Adv. Differ. Equ. 2016, 146 (2016). https://doi.org/10.1186/s13662-016-0875-6
Lu, S, Wang, Y, Guo, Y: Existence of periodic solutions of a Liénard equation with a singularity of repulsive type. Bound. Value Probl. 2017, 95 (2017). https://doi.org/10.1186/s13661-017-0826-5
Mawhin, J: Équations intégrales et solutions périodiques des systèmes différentiels non linéaires. Bulletin de la Classe des Sciences de l’Académie Royale de Belgique 55(5), 934-947 (1969)
Mawhin, J: Topological degree and boundary value for nonlinear differential equations. In: Furi, M, Zecca, P (eds.) Topological Methods for Ordinary Differential Equations. Lecture Notes in Math., vol. 1537, pp. 74-142. Springer, Berlin (1993)
Manásevich, R, Mawhin, J: Periodic solutions for nonlinear systems with p-Laplacian-like operators. J. Differ. Equ. 145, 367-393 (1998)
Acknowledgements
The work is sponsored by the talent foundation of NUIST (2012r101).
Author information
Authors and Affiliations
Contributions
All the authors read and approved the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing interests.
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Guo, Y., Wang, Y. & Zhou, D. A new result on the existence of periodic solutions for Rayleigh equation with a singularity. Adv Differ Equ 2017, 394 (2017). https://doi.org/10.1186/s13662-017-1449-y
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s13662-017-1449-y