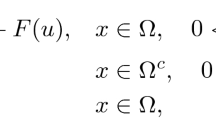1 Introduction

Fractional differential equations have received considerable attentions during the past few decades because they are useful for modeling many practical phenomena. And a large amount of results such as existence, uniqueness, stability, etc. of the solution have been obtained for the fractional differential equations (see [1–5] and the references therein).

In recent years, fractional partial differential equations have been applicated in the study of viscoelasticity, biology, anomalous diffusion, such as [6–10]. Based on the existing inequalities, Jleli [6] presented the Lyapunov inequalities for fractional partial differential equations. The authors in [8] obtained the approximate analytical solutions for two different types of nonlinear time-fractional systems of partial differential equations using the fractional natural decomposition method. And a maximum principle for the generalized time-fractional diffusion equation with the Caputo fractional derivative is established by Luchko [7].

Furthermore, initial-boundary value problems for both ordinary fractional differential equations and fractional partial differential equations are studied in the literatures (see [11–15] and the references therein). The authors in [14] established an existence result for a class of nonlinear fractional partial differential equations with the standard Caputo fractional derivative of order \(1<\alpha\leq2\). In [15], Wang discussed the nonlocal initial value problem for fractional differential equations with the Hilfer fractional derivative.

Zhu [16] and Ouyang [17] investigated the existence and uniqueness of the solution of the following nonlinear fractional reaction-diffusion equation with initial-boundary values and delays:

$$\begin{aligned}& \begin{gathered}[b] {}^{C}D_{t}^{\alpha}u(x,t)- a(t)u_{xx}(x,t) \\ \quad= g\bigl(t,u\bigl(x,\tau_{1}(t)\bigr),u\bigl(x, \tau_{2}(t)\bigr) , \ldots,u\bigl(x,\tau_{l}(t)\bigr)\bigr), \quad(x,t)\in\Omega\times R_{+}, \end{gathered} \end{aligned}$$
(1)
$$\begin{aligned}& u(x,t)=0, \qquad(x,t)\in\partial\Omega\times R_{+}, \qquad u(x,0)= \varphi(x),\qquad x\in\Omega, \end{aligned}$$
(2)

where \(0\leq\tau_{i}(t)\leq t\), \(t\in R_{+}\) (\(i=1,2,\ldots,l\)), l is a positive integer number, \(a(t):R_{+} \rightarrow R\) is continuous and \(\varphi(x) \in L^{2}(\Omega)\). \({}^{C}D_{t}^{\alpha}\) is the standard Caputo fractional derivative of order α (\(0<\alpha \leq1\)).

In [18], Umarov generalized the classical Duhamel principle for the Cauchy problem to general inhomogeneous fractional distributed differential-operator equations of the form

$$\begin{aligned}& L^{\Lambda}[u]\equiv \int_{0}^{\mu} f(\alpha,A)D_{\ast}^{\alpha }u(t) \,d\Lambda(\alpha)=h(t), \quad t>0, \end{aligned}$$
(3)
$$\begin{aligned}& u^{(k)}(0)=\varphi_{k}, \quad k=0,\ldots,m-1, \end{aligned}$$
(4)

where \(\mu\in(m-1,m]\), \(h(t)\) and \(\varphi_{k}\), \(k=0,1,\ldots,m\) are given X-valued vector-functions. \(D_{\ast}^{\alpha}\) denotes the operator of fractional differentiation of order \(0<\alpha<1\) in the sense of Caputo.

The stability of the solution of a definite solution problem is of great importance in the theory of partial differential equations. However, we have not found any related references which investigate the stability of solutions of initial value problems for time-fractional diffusion equations. Motivated by this fact, in this paper we establish a fractional Duhamel principle, then apply it to study the stability and boundedness of the solution of the time-fractional diffusion equation

$$ {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2}u_{xx}(x,t)= h(x,t), \quad0< \alpha< 1, x\in R, t>0, $$
(5)

with the initial condition

$$ u(x,0)=\varphi(x), \quad x\in R, $$
(6)

where \(a\neq0\), \(\varphi(x)\in L^{p} (R)\), \(p\geq1\). \(h(x,t)\) is a continuously differentiable function and \(h(x,0)=0\). \({}^{C}_{0}D_{t}^{\alpha}\) represents the following Caputo fractional derivative of order \(\alpha>0\):

$$ {}^{C}_{0}D_{t}^{\alpha}u(x,t)= \textstyle\begin{cases} \frac{1}{\Gamma(n-\alpha)} \int_{0}^{t}(t-\tau)^{n-\alpha+1} \frac{\partial^{n}u(x,\tau)}{\partial\tau^{n}} \,d\tau, & n-1< \alpha< n, \\ \frac{ \partial^{n}u(x,t)}{ \partial t^{n}}, & \alpha=n, \end{cases} $$
(7)

where Γ is the Gamma function and \(n=[\alpha]\) denotes the integer part of α. Moreover, the Caputo fractional derivative of α is also defined as \(\frac{\partial^{\alpha}u(x,t)}{\partial t^{\alpha}}={{}_{0}}I_{t}^{n-\alpha}\frac{\partial ^{n}}{\partial t^{n}}u(x,t)\).

The rest of this article is organized as follows. Section 2 is devoted to some preliminaries. In Section 3, we present our main results of this paper. An illustrative example is provided in Section 4.

2 Preliminaries

In this section, we introduce some definitions and lemmas which will be used later.

Definition 2.1

[1]

The two-parameter Mittag-Leffler function is defined as

$$ E_{\alpha,\beta}(z)=\sum^{\infty}_{k=0} \frac{z^{k}}{\Gamma(\alpha k + \beta)}, \quad\alpha,\beta>0, $$
(8)

where Γ is the Gamma function.

The Laplace transform of the Mittag-Leffler function in two parameters is

$$ L \bigl[t^{\alpha k+\beta-1}E_{\alpha ,\beta }^{(k)}\bigl(\pm a t^{\alpha }\bigr);s \bigr]=\frac{k!s^{\alpha -\beta }}{(s^{\alpha }\mp a)^{k+1}},\quad \operatorname{Re}(s)> \vert a \vert ^{\frac{1}{\alpha }}. $$
(9)

Definition 2.2

[1]

The Laplace transform of the Caputo fractional derivative \({}^{C}_{0}D_{t}^{\alpha}f(t)\) is

$$\begin{aligned} L \bigl[{}^{C}_{0}D_{t}^{\alpha}f(t);s \bigr] =& \int_{0}^{+\infty }e^{-st} \bigl({}^{C}_{0}D_{t}^{\alpha}f(t) \bigr)\,dt \\ =& s^{\alpha}\tilde{f}(s) -\sum_{k=0}^{n-1}s^{\alpha -k-1}f^{(k)}(0), \quad n-1< \alpha \leq n, \end{aligned}$$
(10)

where \(\tilde{f}(s)\) is the Laplace transform of \(f(t)\).

Particularly, for \(0<\alpha \leq1\),

$$ L \bigl[{}^{C}_{0}D_{t}^{\alpha}f(t);s \bigr]=s^{\alpha}\tilde {f}(s)-s^{\alpha -1}f(0). $$
(11)

Definition 2.3

[1]

The Fourier transform of a continuous function \(h(x)\) absolutely integrable in R is defined by

$$ \hat{h}(\xi)= F\bigl\{ h(x);\xi\bigr\} = \int_{R} e^{i\xi x}h(x)\,dx, \quad\xi \in R, $$
(12)

and the inverse Fourier transform is defined by

$$ h(x)=F^{-1}\bigl\{ \hat{h}(\xi);x\bigr\} =\frac{1}{2\pi} \int_{R} e^{-i\xi x}\hat {h}(\xi)\,d\xi, \quad x\in R. $$
(13)

Lemma 2.1

[18]

Suppose \(v(t,\tau)\) is an X-valued function defined for all \(t\geq \tau\geq0\), the derivatives \(\frac{\partial^{j}v(t,\tau)}{\partial t^{j}}\), \(0\leq j\leq k-1\), are jointly continuous in the X-norm, and \(\frac{\partial^{k}v(t,\tau)}{\partial t^{k}}\in L^{1}(0,t;X)\) for all \(t>0\). Let \(u(t)=\int_{0}^{t} v(t,\tau)\,d\tau\). Then

$$ \frac{d^{k}}{dt^{k}}u(t)=\sum^{k-1}_{j=0} \frac{d^{j}}{dt^{j}} \biggl[\frac {\partial^{k-1-j}}{\partial t^{k-1-j}}v(t,\tau)\bigg\vert _{\tau=t} \biggr]+ \int_{0}^{t} \frac{\partial^{k}}{\partial t^{k}}v(t,\tau)\,d\tau. $$
(14)

Lemma 2.2

[19]

For every \(\alpha\in(0,1)\), the uniform estimate

$$ \frac{1}{1+\Gamma(1-\alpha)x}\leq E_{\alpha}(-x)\leq\frac {1}{1+[\Gamma(1+\alpha)]^{-1}x} $$
(15)

holds over \(R^{+} \), where \(E_{\alpha}(-x)\) denotes \(E_{\alpha,1}(-x)\).

Remark 2.1

Obviously, \(0< E_{\alpha,1}(-x)<1\), for any \(x>0\) by Lemma 2.2.

Lemma 2.3

[20]

Let \(0<\alpha<1\). Then

$$ _{0}I^{ \alpha}_{t}\bigl( {{}^{C}_{0}}D_{t}^{ \alpha} f(t)\bigr)=f(t)-f(0). $$
(16)

Lemma 2.4

[21]

The Fourier transform of the Dirac delta function \(\delta(x)\) is

$$ F\bigl\{ \delta(x);\xi\bigr\} = \int_{R} e^{i\xi x}\delta(x)\,dx=1 $$
(17)

and the inverse Fourier transform of the Dirac delta function \(\delta (x)\) is

$$ \delta(x)=F^{-1}\{1\}=\frac{1}{2\pi} \int_{R} e^{-i\xi x} \,dx. $$
(18)

Lemma 2.5

[21]

The Dirac delta function \(\delta(x)\) has the following property:

$$ \int_{R} \delta(x)\,dx=1. $$
(19)

Lemma 2.6

[21], Hausdorff-Young inequality

If \(f\in L^{1} \), \(g\in L^{p} \) (\(p\geq1\)), then \(h=f\ast g \in L^{p}\) and

$$ \Vert h \Vert _{L^{p}}\leq \Vert f \Vert _{L^{1}}\cdot \Vert g \Vert _{L^{p}}, $$
(20)

where \(f\ast g=\int_{R} f(x-y)g(y)\,dy\) denotes the convolution between f and g.

3 Main results

In this section, we first consider the situation of \(h(x,t)=0\) in the IVP (5)-(6). That is, we discuss the homogeneous IVP

$$\begin{aligned}& {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2}u_{xx}(x,t)=0, \quad0< \alpha< 1, x\in R, t>0, \end{aligned}$$
(21)
$$\begin{aligned}& u(x,0)=\varphi(x), \quad x\in R. \end{aligned}$$
(22)

Lemma 3.1

The solution of the homogeneous the IVP (21)-(22) has the form

$$ u(x,t)= \int_{R} G(x-y,t)\varphi(y)\,dy, $$
(23)

where \(G(x,t)=\frac{1}{2\pi} \int_{R} e^{-i\xi x}E_{\alpha,1}(-a^{2}\xi ^{2}t^{\alpha})\,d\xi\) is the Green function.

Proof

Applying the Laplace transform to equation (21) with respect to the variable t yields

$$ s^{\alpha}\tilde{u}(x,s)-s^{\alpha-1}\varphi(x)-a^{2} \tilde{u}_{xx}(x,s)=0, $$
(24)

then applying the Fourier transform with respect to variable x, we obtain

$$ s^{\alpha}\hat{\tilde{u}}(\xi,s)-s^{\alpha-1} \hat{\varphi}(\xi )-a^{2} (-i\xi)^{2}\hat{\tilde{u}}(\xi,s) =0, $$
(25)

where \(i^{2}=-1\). So we have

$$ \hat{\tilde{u}}(\xi,s)=\frac{s^{\alpha-1}}{s^{\alpha}+a^{2}\xi ^{2}}\hat{\varphi}(\xi) =\frac{s^{\alpha-1}}{s^{\alpha}-(-a^{2}\xi^{2})} \hat{\varphi}(\xi). $$
(26)

Applying the inverse Laplace transform yields

$$ \hat{u}(\xi,t)=E_{\alpha,1}\bigl(-a^{2}\xi^{2}t^{\alpha}\bigr)\hat{\varphi}(\xi). $$
(27)

Furthermore, by using the inverse Fourier transform and Fubini’s theorem, we get

$$\begin{aligned} u(x,t) =& \frac{1}{2\pi} \int_{R} e^{-i\xi x}E_{\alpha,1}\bigl(-a^{2} \xi ^{2}t^{\alpha}\bigr)\hat{\varphi}(\xi)\,d\xi \\ =& \frac{1}{2\pi} \int_{R} e^{-i\xi x}E_{\alpha,1}\bigl(-a^{2} \xi^{2}t^{\alpha}\bigr) \int_{R} e^{i\xi y}\varphi(y)\,dy \,d\xi \\ =& \int_{R} \frac{1}{2\pi} \int_{R} e^{-i\xi(x-y)}E_{\alpha ,1}\bigl(-a^{2} \xi^{2}t^{\alpha}\bigr) \,d\xi\varphi(y)\,dy \\ =& \int_{R} G(x-y,t)\varphi(y)\,dy, \end{aligned}$$
(28)

where

$$ G(x,t)=\frac{1}{2\pi} \int_{R} e^{-i\xi x}E_{\alpha,1}\bigl(-a^{2} \xi ^{2}t^{\alpha}\bigr)\,d\xi $$
(29)

is the Green function. This completes the proof. □

Property 3.1

The Green function \(G(x,t)\) has the following property:

$$ \int_{R} G(x,t)\,dx< 1, \quad t>0. $$
(30)

Proof

By Lemma 2.2, it follows

$$\begin{aligned} G(x,t) =& \frac{1}{2\pi} \int_{R} e^{-i\xi x}E_{\alpha,1}\bigl(-a^{2} \xi ^{2}t^{\alpha}\bigr)\,d\xi \\ \leq& \frac{1}{2\pi} \biggl\vert \int_{R} e^{-i\xi x}E_{\alpha ,1}\bigl(-a^{2} \xi^{2}t^{\alpha}\bigr)\,d\xi \biggr\vert \\ \leq& \frac{1}{2\pi} \int_{R} e^{-i\xi x} \bigl\vert E_{\alpha ,1} \bigl(-a^{2}\xi^{2}t^{\alpha}\bigr) \bigr\vert \,d\xi \\ < & \frac{1}{2\pi} \int_{R} e^{-i\xi x}\cdot1 \,d\xi \\ =&\delta(x). \end{aligned}$$
(31)

Lemma 2.5 implies that

$$ \int_{R} G(x,t)\,dx< \int_{R} \delta(x) \,dx =1, \quad t>0, $$
(32)

which completes the proof. □

3.1 Fractional Duhamel principle

We now consider equation (5) with the initial data \(u(x,0)=\varphi(x)=0\). That is, we study the nonhomogeneous IVP

$$\begin{aligned}& {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2}u_{xx}(x,t)=h(x,t), \quad0< \alpha< 1, x\in R, t>0, \end{aligned}$$
(33)
$$\begin{aligned}& u(x,0)=0, \quad x\in R. \end{aligned}$$
(34)

A fractional Duhamel principle is firstly given, which can reduce the nonhomogeneous the IVP (33)-(34) to the corresponding homogeneous IVP.

Theorem 3.1

Fractional Duhamel principle

The solution of the nonhomogeneous the IVP (33)-(34) is given by

$$ u(x,t)= \int_{0}^{t}w(x,t;\tau)\,d\tau, $$
(35)

where \(w(x,t;\tau)\) is the solution of the homogeneous equation

$$ {}^{C}_{0}D_{t}^{\alpha}w(x,t)- a^{2}w_{xx}(x,t)=0, \quad0< \alpha< 1, x\in R, t> \tau, $$
(36)

satisfying

$$ t=\tau: w(x,\tau)= {{}^{C}_{0}}D_{\tau}^{1-\alpha}h(x, \tau), $$
(37)

where \(h(x,t)\) is a continuously differentiable function.

Proof

Assume that \(w(x,t;\tau)\) is the solution of the IVP (36)-(37). We next prove that \(u(x,t)=\int_{0}^{t}w(x,t;\tau)\,d\tau\) is the solution of the IVP (33)-(34). Let \(k=1\) in Lemma 2.1, then

$$ \frac{\partial}{\partial t}u(x,t) =w(x,t;\tau)\vert_{\tau=t}+ \int _{0}^{t}\frac{\partial}{\partial t}w(x,t;\tau) \,d\tau. $$
(38)

Thus it follows that

$$\begin{aligned} & {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2}u_{xx}(x,t) \\ &\quad = {}_{0}I^{1-\alpha}_{t} \frac{\partial}{\partial t}u(x,t)- a^{2}u_{xx}(x,t) \\ &\quad = {}_{0}I^{1-\alpha}_{t}\frac{\partial}{\partial t} \int_{0}^{t}w(x,t;\tau)\,d\tau- \int_{0}^{t}a^{2}w_{xx}(x,t; \tau) \,d\tau \\ &\quad ={} _{0}I^{1-\alpha}_{t} \biggl[w(x,t;\tau) \vert_{\tau=t} + \int_{0}^{t} \frac{\partial}{\partial t}w(x,t;\tau)\,d\tau \biggr] - \int _{0}^{t}a^{2}w_{xx}(x,t; \tau) \,d\tau \\ &\quad ={}_{0}I^{1-\alpha}_{t}\bigl( {{}^{C}_{0}}D_{t}^{1-\alpha}h(x,t) \bigr)+ \int_{0}^{t} \biggl[{{}_{0}}I^{1-\alpha}_{t} \frac{\partial}{\partial t}w(x,t;\tau )-a^{2}w_{xx}(x,t;\tau) \biggr] \,d\tau \\ &\quad = h(x,t)-h(x,0)+ \int_{0}^{t} \bigl[{{}^{C}_{0}D_{t}^{\alpha}} w(x,t;\tau)- a^{2}w_{xx}(x,t;\tau) \bigr] \,d\tau \\ &\quad = h(x,t). \end{aligned}$$
(39)

In addition, \(u(x,0)=0\). Therefore, \(u(x,t)=\int_{0}^{t}w(x,t;\tau)\, d\tau\) is the solution of the IVP (33)-(34). The proof is completed. □

Corollary 3.1

(i) The the IVP (36)-(37) has the solution. In fact, let \(t'=t-\tau\) in (36)-(37), then the IVP (36)-(37) can be turned into the form

$$\begin{aligned}& {}^{C}_{0}D_{t}^{\alpha}w \bigl(x,t';\tau\bigr)- a^{2}w_{xx} \bigl(x,t';\tau\bigr)=0, \quad 0< \alpha< 1, x\in R, t'>0, \end{aligned}$$
(40)
$$\begin{aligned}& t'=0: w(x,0;\tau)={{}^{C}_{0}}D_{\tau}^{1-\alpha}h(x, \tau), \quad x\in R. \end{aligned}$$
(41)

Lemma  3.1 implies that the solution of the problem (40)-(41) can be obtained by

$$ w\bigl(x,t';\tau\bigr)= \int_{R} G\bigl(x-y,t'\bigr){{}^{C}_{0}}D_{\tau}^{1-\alpha}h(y, \tau)\,dy. $$
(42)

Hence, the solution of the IVP (36)-(37) can be represented as

$$ w(x,t-\tau;\tau)= \int_{R} G(x-y,t-\tau){}^{C}_{0}D_{\tau}^{1-\alpha}h(y, \tau)\,dy. $$
(43)

(ii) Furthermore, by Theorem  3.1, the solution of the IVP (33)-(34) has the form

$$ u(x,t)= \int_{0}^{t}w(x,t-\tau;\tau)\,d\tau= \int_{0}^{t} \int_{R} G(x-y,t-\tau){{}^{C}_{0}}D_{\tau}^{1-\alpha }h(y, \tau)\,dy\,d\tau. $$
(44)

Combining Lemma 3.1 with Corollary 3.1, we can get the following theorem.

Theorem 3.2

The solution of the nonhomogeneous the IVP (5)-(6) has the form

$$ u(x,t)=u_{1}(x,t)+u_{2}(x,t), $$
(45)

where \(u_{1}(x,t)\), \(u_{2}(x,t)\) are solutions of the IVPs (21)-(22), (33)-(34), respectively. That is,

$$ u(x,t)= \int_{R} G(x-y,t)\varphi(y)\,dy+ \int_{0}^{t} \int_{R} G(x-y,t-\tau) {{}^{C}_{0}}D_{\tau}^{1-\alpha}h(y, \tau)\,dy\,d\tau, $$
(46)

where \(G(x,t)=\frac{1}{2\pi}\int_{R} e^{-i\xi x}E_{\alpha,1}(-a^{2}\xi ^{2}t^{\alpha})\,d\xi\) is the Green function.

Theorem 3.3

When \(t\rightarrow0\), the solution (46) of the Cauchy problem (5)-(6) is bounded by the initial data

$$ \bigl\Vert u(x,t) \bigr\Vert _{L^{p}(R)} \leq \bigl\Vert u(x,0) \bigr\Vert _{L^{p}(R)}, \quad x\in R, p\geq1. $$
(47)

Proof

From (46) and Lemma 2.4, we have

$$ \begin{aligned}[b] \lim_{t \rightarrow0} \bigl\Vert u(x,t) \bigr\Vert _{L^{p}(R)} &= \biggl\Vert \int_{R} G(x-y,0)u(y,0)\,dy \biggr\Vert _{L^{p}(R )} \\ &= \biggl\Vert \int_{R} \delta(x-y) u(y,0)\,dy \biggr\Vert _{L^{p}(R)} \\ &= \bigl\Vert \delta(x)\ast u(x,0) \bigr\Vert _{L^{p}(R)}, \quad p \geq1. \end{aligned} $$
(48)

Then the inequality (48), Lemma 2.6 and the property of the Dirac delta function \(\delta(x)\) imply

$$\begin{aligned} \lim_{t \rightarrow0} \bigl\Vert u(x,t) \bigr\Vert _{L^{p}(R)} =& \bigl\Vert \delta(x)\ast u(x,0) \bigr\Vert _{L^{p}(R)} \\ \leq& \bigl\Vert \delta(x) \bigr\Vert _{L^{1}(R)}\cdot \bigl\Vert u(x,0) \bigr\Vert _{L^{p}(R)} \\ \leq& \bigl\Vert u(x,0) \bigr\Vert _{L^{p}(R)}, \end{aligned}$$
(49)

for \(p\geq1\), which completes the proof. □

3.2 Stability of solution

This section presents the stability of the solution of the nonhomogeneous the IVP (5)-(6).

Definition 3.1

Suppose that H is a linear normed space with the norm \(\Vert \cdot \Vert _{H}\), \(u_{1}(x,t)\), \(u_{2}(x,t)\) are solutions of the IVP (5)-(6) corresponding to initial datum \(\varphi_{1}(x)\), \(\varphi_{2}(x)\), respectively. For any \(\varepsilon >0\), if there exists a constant \(\delta>0\) such that \(\Vert \varphi_{1}(x)-\varphi_{2}(x) \Vert <\delta\) implies \(\Vert u_{1}(x,t)-u_{2}(x,t) \Vert <\varepsilon\), then we say that the solution of the IVP (5)-(6) is stable.

Theorem 3.4

Stability

Assume \(\varphi(x)\in L^{p}(R)\), \(p\geq1\). Then the solution \(u(x,t)\) of the nonhomogeneous IVP (5)-(6) is stable.

Proof

Suppose that \(u_{1}(x,t)\) is the solution of the nonhomogeneous IVP

$$\begin{aligned}& {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2}u_{xx}(x,t)=h(x,t), \quad0< \alpha< 1, x\in R, t>0, \end{aligned}$$
(50)
$$\begin{aligned}& u(x,0)=\varphi_{1}(x), \quad x\in R \end{aligned}$$
(51)

and that \(u_{2}(x,t)\) is the solution of the nonhomogeneous IVP

$$\begin{aligned}& {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2} u_{xx}(x,t)=h(x,t), \quad0< \alpha< 1, x\in R, t>0, \end{aligned}$$
(52)
$$\begin{aligned}& u(x,0)= \varphi_{2}(x), \quad x\in R. \end{aligned}$$
(53)

Then the superposition principle implies that \(u_{1}(x,t)-u_{2}(x,t)\) is the solution of the following homogeneous IVP:

$$\begin{aligned}& {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2} u_{xx}(x,t)=0, \quad0< \alpha< 1, x\in R, t>0, \end{aligned}$$
(54)
$$\begin{aligned}& u(x,0)=\varphi_{1}(x)-\varphi_{2}(x), \quad x\in R. \end{aligned}$$
(55)

By Lemma 3.1, we get

$$ u_{1}(x,t)-u_{2}(x,t)= \int_{R} G(x-y,t) \bigl[ \varphi_{1}(y)- \varphi_{2}(y) \bigr]\,dy, $$
(56)

where \(G(x,t)=\frac{1}{2\pi}\int_{R} e^{-i\xi x}E_{\alpha,1}(-a^{2}\xi ^{2}t^{\alpha})\,d\xi\) is the Green function. Taking the \(L^{p}\)-norm (\(p\geq1\)) on both sides of equation (56), then Lemma 2.6 yields

$$ \begin{aligned}[b] \bigl\Vert u_{1}(x,t)-u_{2}(x,t) \bigr\Vert _{L^{p}(R)} &= \biggl\Vert \int_{R} G(x-y,t) \bigl[\varphi_{1}(y)-\varphi _{2}(y) \bigr] \,dy \biggr\Vert _{L^{p}(R)} \\ &= \bigl\Vert G(x,t)\ast \bigl[\varphi_{1}(x)-\varphi_{2}(x) \bigr] \bigr\Vert _{L^{p}(R)} \\ &\leq \bigl\Vert G(x,t) \bigr\Vert _{L^{1}(R)} \cdot \bigl\Vert \varphi_{1}(x)-\varphi_{2}(x) \bigr\Vert _{L^{p}(R)} \end{aligned} $$
(57)

for \(t>0\). Then from Lemma 2.6 and the property of the Green function \(G(x,t)\) it follows that

$$\begin{aligned} \bigl\Vert u_{1}(x,t)-u_{2}(x,t) \bigr\Vert _{L^{p}(R)} \leq& \bigl\Vert G(x,t) \bigr\Vert _{L^{1}(R)} \cdot \bigl\Vert \varphi_{1}(x)-\varphi_{2}(x) \bigr\Vert _{L^{p}(R)} \\ < & \bigl\Vert \varphi_{1}(x)-\varphi_{2}(x) \bigr\Vert _{L^{p}(R)},\quad t>0. \end{aligned}$$
(58)

For any \(\varepsilon>0\), choose \(\delta<\varepsilon\). Then \(\Vert \varphi_{1}(x)-\varphi_{2}(x) \Vert _{L^{p}(R)}<\delta\) implies \(\Vert u_{1}(x,t)-u_{2}(x,t) \Vert _{L^{p}(R)}<\varepsilon\), \(t>0\). By Definition 3.1, the solution \(u(x,t)\) of the nonhomogeneous the IVP (5)-(6) is stable. The proof is completed. □

4 Illustrative example

In this section, we provide an example to show the application of our stability result.

Example 4.1

Consider the following nonhomogeneous equation:

$$ {}^{C}_{0}D_{t}^{\alpha}u(x,t)- a^{2} \frac{\partial^{2} u(x,t)}{\partial x^{2}} =2t^{1-\alpha}\bigl(x^{2}+x+1 \bigr), \quad0< \alpha< 1, x\in R, t>0, $$
(59)

with the initial condition

$$ u(x,0)=x^{2}, \quad x\in R. $$
(60)

The well-known formula (\(0<\alpha<1\))

$$ {}^{C}D_{t}^{\alpha}t^{\beta}= \frac{\Gamma(\beta+1)}{\Gamma(\beta -\alpha+1)} t^{\beta-\alpha},\quad\beta>0, $$
(61)

and Theorem 3.2 imply that the solution of the IVP (59)-(60) is

$$ u(x,t)= \int_{R} G(x-y,t)y^{2}\,dy+2\Gamma(2-\alpha) \int_{0}^{t} \int_{R} G(x-y,t-\tau) \bigl(y^{2}+y+1\bigr)\,dy\,d\tau. $$
(62)

Suppose that \(u_{1}(x,t)\), \(u_{2}(x,t)\) are solutions of the IVP (59)-(60) corresponding to initial datum \(x_{1}^{2}\), \(x_{2}^{2}\), respectively. Then, for \(p\geq1\), we have

$$\begin{aligned} \bigl\Vert u_{1}(x,t)-u_{2}(x,t) \bigr\Vert _{L^{p}(R)} \leq& \bigl\Vert G(x,t) \bigr\Vert _{L^{1}(R)} \cdot \bigl\Vert x_{1}^{2}-x_{2}^{2} \bigr\Vert _{L^{p}(R)} \\ < & \bigl\Vert x_{1}^{2}-x_{2}^{2} \bigr\Vert _{L^{p}(R)},\quad t>0. \end{aligned}$$
(63)

For any \(\varepsilon>0\), choose \(\delta<\varepsilon\). Then \(\Vert x_{1}^{2}-x_{2}^{2} \Vert _{L^{p}(R)}<\delta\) implies \(\Vert u_{1}(x,t)-u_{2}(x,t) \Vert _{L^{p}(R)}<\varepsilon\), \(t>0\). According to Definition 3.1, the solution (62) of the IVP (59)-(60) is stable.