1 Introduction

In the early twentieth century, Jackson discovered a new mathematical direction of q-difference calculus. Its basic definition and properties can be seen in the literature [1, 2]. Since then, due to q-difference calculus having important applications in mathematical physics, quantum mechanics, complex analysis, and other fields, many scholars have studied q-difference equations and obtained a variety of useful results. Fractional q-difference calculus is an extension of q-difference calculus, which originated from Al-Salam [3] and Agarwal [4], and some results can be found in the literature [5, 6]. Up to now, fractional q-difference calculus is still a hot topic of research. In recent years, there has been tremendous interest in developing the solvability of fractional q-difference equations.

It is of great significance to investigate the boundary value problems (BVPs) of fractional q-difference equations. As is known, it can be applied to many aspects of real life, such as engineering, physics, chemistry, mechanics, the electrodynamics of composite media, and so on. More and more researchers devote themselves to the research, and have come up with a great deal of interesting and novel theories and results for various BVPs of fractional q-difference equations, see [7–19] and references therein. However, in spite of BVPs for fractional q-difference equations attracting extensive attention from experts and scholars, relevant conclusions are still few in number. In particular, the solvability theory of higher-order nonlinear fractional q-difference equations needs further exploration.

In [8], Ferreira investigated the BVP for the nonlinear fractional q-difference equation

$$\begin{aligned} \textstyle\begin{cases} (D_{q}^{\alpha}y)(x)=- f(x,y(x)), \quad 0< x< 1, \\ y(0)=(D_{q}y)(0)=0, \qquad (D_{q}y)(1)=\beta \geq 0, \end{cases}\displaystyle \end{aligned}$$

where \(2<\alpha \leq 3\) and f:[0,1]×R↦R is a nonnegative continuous function. The author obtained the existence of positive solutions for BVP by applying a fixed-point theorem in cones.

Recently, in [11], Zhai and Ren obtained the existence and uniqueness of solutions for the nonlinear fractional q-difference equation with three-point boundary conditions

$$\begin{aligned} \textstyle\begin{cases} (D_{q}^{\alpha}u)(t)+f(t,u(t))=b,\quad 0< t< 1, 2< \alpha < 3, \\ u(0)=(D_{q}u)(0)=0, \qquad (D_{q}u)(1)=\beta (D_{q}u)(\eta ), \end{cases}\displaystyle \end{aligned}$$

by using a new fixed-point theorem of increasing \(\psi -(h,r)\)-concave operators defined in ordered sets, where \(0<\beta \eta ^{\alpha -2}<1\), \(0< q<1\), \(b\geq 0\) is a constant, \(D_{q}^{\alpha}\) denotes the Riemann–Liouville-type fractional q-derivative of order α.

On the basis of the above works, we mainly investigate the BVP of the nonlinear fractional q-difference equation

$$\begin{aligned} \textstyle\begin{cases} (D_{q}^{\gamma}\varpi )(t)+ f(t,\varpi (t))=\xi , \quad 0< t< 1, \\ \varpi (0)=(D_{q}\varpi )(0)=\cdots =(D_{q}^{n-2}\varpi )(0)=0,\qquad (D_{q}^{ \delta}\varpi )(1)=a(D_{q}^{\delta}\varpi )(\eta ), \end{cases}\displaystyle \end{aligned}$$
(1)

where \(0< q<1\), \(n-1<\gamma \leq n\) (\(n>2\)), \(1\leq \delta \leq n-2\), \(0< \eta <1\), \(0< a\eta ^{\gamma -\delta -1}<1\) and \(\xi \geq 0\) is a constant. Using a novel fixed-point theorem of \(\psi -(h,r)\)-concave operators defined in an ordered set \(P_{h,r}\) ([20]), we discuss the existence and uniqueness of iterative solutions for BVP (1), which is an increasing technique of dealing with nonlinear fractional q-difference BVPs.

The present paper is organized as follows. The second section shows the definitions, lemmas, theorems, and assumptions used in this paper. The third section expounds the main conclusions of this paper and gives the corresponding proof. The fourth section cites an example to verify the main conclusions. The last section of this paper contains a few concluding remarks.

2 Preliminaries

In this section, we first introduce some definitions and results of fractional q-calculus.

The q-integral of a function f in the interval \([0,b]\) is given by

$$\begin{aligned} (I_{q}f) (x)= \int _{0}^{x}f(t)\,d_{q}t=x(1-q)\sum _{n=0}^{\infty}f\bigl(xq^{n} \bigr)q^{n},\quad x \in [0,b]. \end{aligned}$$

If \(a\in [0,b]\) and f is defined in the interval \([0,b]\), its integral from a to b is defined by

$$\begin{aligned} \int _{a}^{b}f(t)\,d_{q}t= \int _{0}^{b}f(t)\,d_{q}t- \int _{0}^{a}f(t)\,d_{q}t. \end{aligned}$$

Lemma 2.1

([7])

Let \(\alpha >0\), then we have the following formulas:

$$\begin{aligned} &\bigl[a(t-s)\bigr]^{(\alpha )}=a^{\alpha}(t-s)^{(\alpha )}, \\ &{{}_{t}D_{q}}(t-s)^{(\alpha )}=[\alpha ]_{q}(t-s)^{(\alpha -1)}. \end{aligned}$$

Remark 2.2

([8])

If \(\alpha >0\) and \(a\leq b\leq t\), then \((t-a)^{(\alpha )}\geq (t-b)^{(\alpha )}\).

Definition 2.3

([8])

Let \(\beta \geq 0\) and f be a function defined on \([0,1]\). The fractional q-integral of Riemann–Liouville type is

$$\begin{aligned} \bigl(I_{q}^{\beta}f\bigr) (s)=\frac{1}{\Gamma _{q}(\beta )} \int _{0}^{s}(s-qt)^{( \beta -1)}f(t)\,d_{q}t,\quad s\in [0,1]. \end{aligned}$$

Obviously, \((I_{q}^{\beta}f)(s)=(I_{q}f)(s)\) when \(\beta =1\).

Definition 2.4

([8])

The fractional q-derivative of Riemann–Liouville type of \(\beta \geq 0\) is defined by

$$\begin{aligned} \bigl(D_{q}^{\beta}f\bigr) (s)=\bigl(D_{q}^{l}I_{q}^{l-\beta}f \bigr) (s), \quad s\in [0,1], \end{aligned}$$

where l is the smallest integer greater than or equal to β. In particular, if \(\beta =1\), then \((D_{q}^{\beta}f)(s)=(D_{q}f)(s)\).

Lemma 2.5

([8])

Let \(\alpha ,\beta \geq 0\) and f be a function defined on \([0,1]\). Then, the following formulas hold:

  1. 1.

    \((I_{q}^{\beta}I_{q}^{\alpha}f)(x)=(I_{q}^{\alpha +\beta}f)(x)\),

  2. 2.

    \((D_{q}^{\alpha}I_{q}^{\alpha}f)(x)=f(x)\).

Remark 2.6

Assume that \(g(t)\in [0,1]\) and α, β are two constants such that \(\alpha >2 \geq \beta \geq 1\). Then,

$$\begin{aligned} D_{q}^{\beta} \int _{0}^{t}(t-qs)^{(\alpha -1)}g(s)\,d_{q}s= \frac{\Gamma _{q}(\alpha )}{\Gamma _{q}(\alpha -\beta )} \int _{0}^{t}(t-qs)^{( \alpha -\beta -1)}g(s)\,d_{q}s. \end{aligned}$$

Lemma 2.7

([8])

Let \(\alpha >0\) and p be a positive integer. Then, the following equality holds:

$$\begin{aligned} \bigl(I_{q}^{\alpha}D_{q}^{p}f \bigr) (x)=\bigl(D_{q}^{p}I_{q}^{\alpha}f \bigr) (x) -\sum_{k=0}^{p-1} \frac{x^{\alpha -p+k}}{\Gamma _{q}(\alpha +k-p+1)}\bigl(D_{q}^{k}f\bigr) (0). \end{aligned}$$

Lemma 2.8

([6])

For \(\lambda \in (-1,\infty )\) and \(\alpha \geq 0\), then the following equality holds:

$$\begin{aligned} I_{q}^{\alpha}(t-a)^{(\lambda )}= \frac{\Gamma _{q}(\lambda +1)}{\Gamma _{q}(\alpha +\lambda +1)}(t-a)^{( \alpha +\lambda )},\quad 0< a< t. \end{aligned}$$

In particular, for \(\lambda =0\) and \(a=0\), we have \(I_{q}^{\alpha}(1)(t)=\frac{t^{\alpha}}{\Gamma _{q}{(\alpha +1)}}\). In conclusion, we can obtain

$$\begin{aligned} \int _{0}^{t}(t-qs)^{(\alpha -1)}\,d_{q}s= \Gamma _{q}(\alpha )I_{q}^{ \alpha}(1) (t)={ \frac{1}{[\alpha ]_{q}}t^{\alpha}}. \end{aligned}$$

Next, we introduce a concave operator that plays an important role in the proof of the main results.

Let \((X,\Arrowvert \cdot \Arrowvert )\) be a real Banach space with a partial order induced by a cone P of X, i.e., \(x\leq y\) if and only if \(y-x\in P\).

Definition 2.9

For any \(x,y\in X\), we define x and y as equivalent, if there exist \(\mu >0\) and \(\nu >0\) such that \(\mu x\leq y\leq \nu x \), denoted by \(x\sim y\).

To formulate our hypotheses, we define two important sets. For given \(h>\theta \), define the set \(P_{h}=\{x\in X\mid x\sim h\}\), and it is obvious that \(P_{h}\) ⊂P. Let \(r\in P\) with \(\theta \leq r\leq h\), we define \(P_{h,r}=\{ x\in X\mid x+r\in P_{h}\}\), namely \(P_{h,r}\) ={\(x\in X\mid\) there exist \(\mu =\mu (h,r,x)>0\), \(\nu =\nu (h,r,x)>0\) such that \(\mu h\leq x+r\leq \nu h\)}. It is easy to see that \(P_{h}=P_{h,\theta}\).

Definition 2.10

([20])

Suppose \(T:P_{h,r}\to E\) is a given operator that satisfies: for any \(x\in P_{h,r}\), \(\lambda \in (0,1)\), there exists \(\psi (\lambda )>\lambda \) such that

$$\begin{aligned} T\bigl(\lambda x+(\lambda -1)r\bigr)\geq \psi (\lambda )Tx+\bigl(\psi (\lambda )-1\bigr)r. \end{aligned}$$

Then, T is called a \(\psi -(h,r)\)-concave operator.

Lemma 2.11

([20])

Assume that T is an increasing \(\psi -(h,r)\)-concave operator and P is normal, \(Th\in P_{h,r}\). Then, T has a unique fixed point \(x^{*}\) in \(P_{h,r}\). Further, for any \(v_{0}\in P_{h,r}\), the sequence \(v_{n}=Av_{n-1}\), \(n=1,2,\dots \), then \(\Arrowvert v_{n}-x^{*}\Arrowvert \to 0\) as \(n\to \infty \).

Lemma 2.12

([21])

Assume that T is an increasing \(\psi -(h,\theta )\)-concave operator and P is normal, \(Th\in P_{h}\). Then, T has a unique fixed point \(x^{*}\) in \(P_{h}\). Further, for any \(v_{0}\in P_{h}\), the sequence \(v_{n}=Av_{n-1}\), \(n=1,2,\dots \), then \(\Arrowvert v_{n}-x^{*}\Arrowvert \to 0\) as \(n\to \infty \).

Now, we propose some assumptions that will be used in this paper, as shown below:

\((H_{1})\):

\(f\in C([0,1]\times [-\hat{r},+\infty ),(-\infty ,+\infty ))\) and \(f(t,u)\leq f(t,v)\) for \(-\hat{r}\leq u\leq v<+\infty \);

\((H_{2})\):

\(\forall \lambda \in (0,1)\) and \(y\in [0,\hat{r}]\), there exists \(\psi (\lambda )>\lambda \) such that

$$\begin{aligned} f\bigl(t, \lambda x+(\lambda -1)y\bigr)\geq \psi (\lambda )f(t,x), \quad \forall t \in [0,1], x\in (-\infty ,+\infty ); \end{aligned}$$
\((H_{3})\):

\(f(t,0)\geq 0\) with \(f(t,0)\not \equiv 0\) for every \(t\in [0,1]\);

\((H_{4})\):

\(f\in C([0,1]\times [0,+\infty ),[0,+\infty ))\) and \(f(t,0)\not \equiv 0\) for every \(t\in [0,1]\);

\((H_{5})\):

\(\forall t\in [0,1], f(t,x)\) is increasing with respect to x;

\((H_{6})\):

\(\forall \lambda \in (0,1)\), there exists \(\psi (\lambda )>\lambda \) such that

$$\begin{aligned} f(t,\lambda x)\geq \psi (\lambda )f(t,x), \quad \forall t\in [0,1], x\in [0,+ \infty ). \end{aligned}$$

3 Result of existence and uniqueness

Let \(X=C[0,1]\) be the Banach space endowed with the norm \(\|\varpi \|=\sup \{|\varpi (t)|: t\in [0,1]\}\) and define the standard normal cone P by \(P=\{\varpi \in X\mid \varpi (t)\geq 0, t\in [0,1]\}\).

If \(\xi >0\), \(\forall t\in [0,1]\), we note that

$$\begin{aligned} \begin{aligned} r(t)& = \frac{\xi (1-q)^{2}}{\Gamma _{q}(\gamma -1)} \biggl[ \frac{1-a\eta ^{\gamma -\delta}}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})}t^{ \gamma -1} \\ &\quad{} - \frac{1}{(1-q^{\gamma})(1-q^{\gamma -1})}t^{\gamma} \biggr] \end{aligned} \end{aligned}$$
(2)

and

$$\begin{aligned} \hat{r}(t)=\max \bigl\{ r(t):t\in [0,1]\bigr\} ,\qquad h(t)=\mathcal{H} t^{\gamma -1}, \end{aligned}$$

where

$$\begin{aligned} \mathcal{H}\geq \frac{\xi}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})\Gamma _{q}(\gamma -1)}. \end{aligned}$$
(3)

Lemma 3.1

Let \(y\in C[0,1], a\eta ^{\gamma -\delta -1}\ne 1\) and \(\eta \in (0,1)\). Then, the BVP

$$\begin{aligned} \textstyle\begin{cases} (D_{q}^{\gamma}\varpi )(t)+y(t)=0, \quad 0< t< 1, \\ \varpi (0)=(D_{q}\varpi )(0)=\cdots =(D_{q}^{n-2}\varpi )(0)=0,\qquad (D_{q}^{ \delta}\varpi )(1)=a(D_{q}^{\delta}\varpi )(\eta ), \end{cases}\displaystyle \end{aligned}$$
(4)

has a unique solution

$$\begin{aligned} \varpi (t)= \int _{0}^{1}\overline{G}(t,qs)y(s)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)y(s)\,d_{q}s, \end{aligned}$$

where

$$\begin{aligned}& \overline{G}(t,s)=\frac{1}{\Gamma _{q}(\gamma )}\textstyle\begin{cases} (1-s)^{(\gamma -\delta -1)}t^{\gamma -1}-(t-s)^{(\gamma -1)} , & 0 \leq s\leq t\leq 1, \\ (1-s)^{(\gamma -\delta -1)}t^{\gamma -1}, & 0 \leq t\leq s\leq 1, \end{cases}\displaystyle \\& \begin{aligned} \overline{H}(t,s)& = \frac{\Gamma _{q}(\gamma -\delta )}{\Gamma _{q}(\gamma -1)}{{}_{t}D_{q}^{ \delta} \overline{G}(t,s)} \\ & = \frac{[\gamma -1]_{q}}{\Gamma _{q}(\gamma )}\textstyle\begin{cases} (1-s)^{(\gamma -\delta -1)}t^{\gamma -\delta -1}-(t-s)^{(\gamma - \delta -1)} , & 0\leq s\leq t\leq 1, \\ (1-s)^{(\gamma -\delta -1)}t^{\gamma -\delta -1}, & 0 \leq t\leq s\leq 1. \end{cases}\displaystyle \end{aligned} \end{aligned}$$

Proof

Let \(\varpi (t)\) be a solution of (4). In view of Lemma 2.5 and Lemma 2.7, we have

$$\begin{aligned} \varpi (t)=c_{1}t^{\gamma -1}+c_{2}t^{\gamma -2}+ \cdots +c_{n}t^{ \gamma -n}-\frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{t}(t-qs)^{( \gamma -1)}y(s)\,d_{q}s, \end{aligned}$$

where \(c_{1}, c_{2}, \ldots , c_{n}\) are some constants to be determined. Since \((D_{q}^{i}\varpi )(0)=0\) (\(0\leq i\leq n-2\)), it follows that \(c_{2}=c_{3}=\cdots =c_{n}=0\). Thus,

$$\begin{aligned} \varpi (t)=c_{1}t^{\gamma -1}-\frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{t}(t-qs)^{( \gamma -1)}y(s)\,d_{q}s. \end{aligned}$$

By Remark 2.6, we have

$$\begin{aligned} \bigl(D_{q}^{\delta}\varpi \bigr) (t)=c_{1} \frac{\Gamma _{q}(\gamma )}{\Gamma _{q}(\gamma -\delta )}t^{\gamma - \delta -1}-\frac{1}{ \Gamma _{q}(\gamma -\delta )} \int _{0}^{t}(t-qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s. \end{aligned}$$

Using the boundary condition \((D_{q}^{\delta}\varpi )(1)=a(D_{q}^{\delta}\varpi )(\eta )\), we obtain

$$\begin{aligned} c_{1}=\frac{1}{(1-a\eta ^{\gamma -\delta -1})\Gamma _{q}(\gamma )} \biggl[ \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s -a \int _{0}^{ \eta}(\eta -qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s \biggr]. \end{aligned}$$

Hence,

$$\begin{aligned} \varpi (t)& = \frac{t^{\gamma -1}}{\Gamma _{q}(\gamma )(1-a\eta ^{\gamma -\delta -1})} \biggl[ \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s \\ &\quad{} - a \int _{0}^{\eta}(\eta -qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s \biggr] - \frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{t}(t-qs)^{(\gamma -1)}y(s)\,d_{q}s. \end{aligned}$$

Namely,

$$\begin{aligned} \varpi (t)& = \frac{t^{\gamma -1}(1-a\eta ^{\gamma -\delta -1}+a\eta ^{\gamma -\delta -1})}{\Gamma _{q}(\gamma )(1-a\eta ^{\gamma -\delta -1})} \biggl[ \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s \\ &\quad{} - a \int _{0}^{\eta}(\eta -qs)^{(\gamma -\delta -1)}y(s)\,d_{q}s \biggr] - \frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{t}(t-qs)^{(\gamma -1)}y(s)\,d_{q}s \\ & = \int _{0}^{1}\overline{G}(t,qs)y(s)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)y(s)\,d_{q}s. \end{aligned}$$

The proof is completed. □

Remark 3.2

When \(\delta =1\), the function \(\overline{G}(t,s)\) can be reduced to the following form:

$$\begin{aligned} \overline{G}(t,s)=\frac{1}{\Gamma _{q}(\gamma )}\textstyle\begin{cases} (1-s)^{(\gamma -2)}t^{\gamma -1}-(t-s)^{(\gamma -1)} , & 0 \leq s\leq t\leq 1, \\ (1-s)^{(\gamma -2)}t^{\gamma -1}, & 0\leq t \leq s\leq 1, \end{cases}\displaystyle \end{aligned}$$

which appeared in [11].

Remark 3.3

When \(\delta =1\), then \(\overline{H}(t,s)={{}_{t}}D_{q}\overline{G}(t,s)\), which is the relationship between \(G(t,s)\) and \(H(t,s)\) in [11].

Lemma 3.4

The function \(\overline{G}(t,qs)\) is continuous on \([0,1]\times [0,1]\) and satisfies

  1. (1)

    \(\overline{G}(t,qs)\geq 0\), for any \(t,s\in [0,1]\);

  2. (2)

    \(\overline{G}(t,qs)\) is strictly increasing in t;

  3. (3)

    \(\overline{G}(t,qs)\leq \frac{1}{\Gamma _{q}(\gamma )}(1-qs)^{( \gamma -\delta -1)}t^{\gamma -1}\leq \frac{1}{\Gamma _{q}(\gamma )}\), for any \(t,s\in [0,1]\).

Proof

Let \(g_{1}(t,qs)=(1-qs)^{(\gamma -\delta -1)}t^{\gamma -1}-(t-qs)^{( \gamma -1)}\), \(g_{2}(t,qs)=(1-qs)^{(\gamma -\delta -1)}t^{\gamma -1}\).

(1) For \(t,s\in [0,1]\), obviously, \(g_{2}(t,qs)\geq 0\). We just need to prove \(g_{1}(t,qs)\geq 0\), for \(t\neq 0\),

$$\begin{aligned} \begin{aligned} g_{1}(t,qs)& = (1-qs)^{(\gamma -\delta -1)}t^{\gamma -1}-(t-qs)^{( \gamma -1)} \\ & = t^{\gamma -1} \biggl[(1-qs)^{(\gamma -\delta -1)}-\biggl(1- \frac{qs}{t}\biggr)^{( \gamma -1)} \biggr] \\ & \geq t^{\gamma -1} \bigl[(1-qs)^{(\gamma -\delta -1)}-(1-qs)^{( \gamma -1)} \bigr]\geq 0. \end{aligned} \end{aligned}$$

Consequently, \(\overline{G}(t,qs)\geq 0\).

(2) For \(s\in [0,1]\), \(t\neq 0\),

$$\begin{aligned}& \begin{aligned} {}_{t}D_{q}g_{1}(t,qs)& = [\gamma -1]_{q} \bigl[(1-qs)^{(\gamma -\delta -1)}t^{ \gamma -2}-(t-qs)^{(\gamma -2)} \bigr] \\ & = [\gamma -1]_{q}t^{\gamma -2} \biggl[(1-qs)^{(\gamma -\delta -1)}- \biggl(1- \frac{qs}{t}\biggr)^{(\gamma -2)} \biggr] \\ & \geq [\gamma -1]_{q}t^{\gamma -2} \bigl[(1-qs)^{(\gamma -\delta -1)}-(1-qs)^{( \gamma -2)} \bigr]\geq 0, \end{aligned} \\& {}_{t}D_{q}g_{2}(t,qs) = [\gamma -1]_{q}t^{\gamma -2}(1-qs)^{(\gamma - \delta -1)}\geq 0. \end{aligned}$$

Therefore, \(\overline{G}(t,qs)\) is an increasing function in the first variable.

(3) It is easy to see that this conclusion is correct. The proof is completed. □

Remark 3.5

According to Remark 3.3, \(\overline{H}(t,qs)\) has common properties with \(\overline{G}(t,qs)\), that is \(\overline{H}(t,qs)\geq 0\) and \(\overline{H}(t,qs)\leq \frac{[\gamma -1]_{q}}{\Gamma _{q}(\gamma )}(1-qs)^{( \gamma -\delta -1)}t^{\gamma -\delta -1}\leq \frac{[\gamma -1]_{q}}{\Gamma _{q}(\gamma )}\).

Theorem 3.6

Suppose that \((H_{1})\)–\((H_{3})\) hold, then the BVP (1) has a unique solution \(\varpi ^{*}\in P_{h,r}\). Moreover, define a sequence to be

$$\begin{aligned} \varphi _{n}(t)& = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varphi _{n-1}(s)\bigr)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \\ &\quad{} \times \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varphi _{n-1}(s)\bigr)\,d_{q}s \\ &\quad{} - \frac{\xi (1-q)^{2}(1-a\eta ^{\gamma -\delta})}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})\Gamma _{q}(\gamma -1)}t^{ \gamma -1} \\ &\quad{} + \frac{\xi (1-q)^{2}}{(1-q^{\gamma})(1-q^{\gamma -1})\Gamma _{q}(\gamma -1)}t^{ \gamma},\quad n=1,2,\dots , \end{aligned}$$

for any given \(\varphi _{0}\in P_{h,r}\), we have \(\varphi _{n}(t)\to \varpi ^{*}(t)\) as \(n\to \infty \).

Proof

For \(t\in [0,1]\), we obtain

$$\begin{aligned} r(t)& = \frac{\xi (1-q)^{2}}{\Gamma _{q}(\gamma -1)} \biggl[ \frac{1-a\eta ^{\gamma -\delta}}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})}t^{ \gamma -1}- \frac{1}{(1-q^{\gamma -1})(1-q^{\gamma})}t^{\gamma} \biggr] \\ & \geq \frac{\xi (1-q)^{2}}{\Gamma _{q}(\gamma -1)}t^{\gamma -1} \frac{(1-a\eta ^{\gamma -\delta})(1-q^{\gamma})-(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -\delta})}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})(1-q^{\gamma})} \\ & \geq \frac{a\xi (1-q)^{2}(\eta ^{\gamma -\delta -1}-\eta ^{\gamma -\delta})}{\Gamma _{q}(\gamma -1)(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma})}t^{ \gamma -1}\geq 0 \end{aligned}$$

and

$$\begin{aligned} r(t)& = \frac{\xi (1-q)^{2}(1-a\eta ^{\gamma -\delta})}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})\Gamma _{q}(\gamma -1)}t^{ \gamma -1} \\ &\quad{} - \frac{\xi (1-q)^{2}}{(1-q^{\gamma -1})(1-q^{\gamma})\Gamma _{q}(\gamma -1)}t^{ \gamma} \\ & \leq \frac{\xi}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})\Gamma _{q}(\gamma -1)}t^{ \gamma -1}\leq \mathcal{H}t^{\gamma -1}=h(t),\quad t\in [0,1]. \end{aligned}$$

Thus, \(0\leq r(t)\leq h(t)\), we have \(r\in P\). In addition, \(P_{h,r}=\{\varpi \in C[0,1]\mid \varpi +r\in P_{h}\}\).

According to Lemmas 2.8 and 3.1, if ϖ is a solution of the BVP (1), then

$$\begin{aligned} \varpi (t)& = \int _{0}^{1}\overline{G}(t,qs) \bigl[f\bigl(s, \varpi (s)\bigr)-\xi \bigr]\,d_{q}s \\ &\quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs) \bigl[f \bigl(s,\varpi (s)\bigr)-\xi \bigr]\,d_{q}s \\ & = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varpi (s)\bigr)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varpi (s)\bigr)\,d_{q}s \\ &\quad{} - \frac{\xi (1-q)^{2}}{\Gamma _{q}(\gamma -1)} \biggl[ \frac{t^{\gamma -1}(1-a\eta ^{\gamma -\delta})}{(1-q^{\gamma -1})(1-q^{\gamma -\delta})(1-a\eta ^{\gamma -\delta -1})}- \frac{t^{\gamma}}{(1-q^{\gamma})(1-q^{\gamma -1})} \biggr] \\ & = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varpi (s)\bigr)\,d_{q}s \\ &\quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varpi (s)\bigr)\,d_{q}s-r(t). \end{aligned}$$

Therefore, for any \(\varpi \in P_{h,r}\) and \(t\in [0,1]\), we define the operator

$$\begin{aligned} T\varpi (t)& = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varpi (s)\bigr)\,d_{q}s \\ &\quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varpi (s)\bigr)\,d_{q}s-r(t). \end{aligned}$$

It is easy to see \(\varpi (t)\) is the solution of the BVP (1) if and only if ϖ is the fixed point of T.

Initially, we show that T is a \(\psi -(h,r)\)-concave operator. For any \(\lambda \in (0,1)\), \(\varpi \in P_{h,r}\), from the condition \((H_{2})\), we can obtain that

$$\begin{aligned} & T\bigl(\lambda \varpi +(\lambda -1)r\bigr) (t) \\ &\quad \geq \psi (\lambda ) \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varpi (s)\bigr)\,d_{q}s \\ &\quad \quad{} + \frac{at^{\gamma -1}\psi (\lambda )}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varpi (s)\bigr)\,d_{q}s-r(t) \\ &\quad = \psi (\lambda )T\varpi (t)+ \bigl[\psi (\lambda )-1 \bigr]r(t). \end{aligned}$$

Thus, we have \(T(\lambda \varpi +(\lambda -1)r)\geq \psi (\lambda )T\varpi + [ \psi (\lambda )-1 ]r\), \(\lambda \in (0,1)\), \(\varpi \in P_{h,r}\). It follows that T is a \(\psi -(h,r)\)-concave operator.

On the other hand, we prove that \(T:P_{h,r}\to X\) is increasing. Due to \(\varpi \in P_{h,r}\), we have \(\varpi +r\in P_{h}\), and there exists \(\iota >0\) such that \(\varpi (t)+r(t)\geq \iota h(t)\), thus we obtain

$$\begin{aligned} \varpi (t)\geq \iota h(t)-r(t)\geq -r(t)\geq -\hat{r},\quad t\in [0,1]. \end{aligned}$$

By condition \((H_{1})\), we know \(T:P_{h,r}\to X\) is increasing.

Now, we prove that \(Th\in P_{h,r}\), which is what we need to prove \(Th+r\in P_{h}\). By Lemma 3.4 and \((H_{1})\), we obtain

$$\begin{aligned} &Th(t)+r(t) \\ &\quad = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,h(s) \bigr)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f \bigl(s,h(s)\bigr)\,d_{q}s \\ &\quad = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s, \mathcal{H}s^{\gamma -1}\bigr)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \mathcal{H}s^{\gamma -1}\bigr)\,d_{q}s \\ &\quad \leq \frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{1}(1-qs)^{(\gamma - \delta -1)}t^{\gamma -1}f(s, \mathcal{H})\,d_{q}s \\ &\quad \quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})\Gamma _{q}(\gamma )} \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}f(s, \mathcal{H})\,d_{q}s \\ &\quad = h(t) \biggl[\frac{1}{\mathcal{H}\Gamma _{q}(\gamma )}+ \frac{a}{\mathcal{H}(1-a\eta ^{\gamma -\delta -1})\Gamma _{q}(\gamma )} \biggr] \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}f(s, \mathcal{H})\,d_{q}s \end{aligned}$$

and

$$\begin{aligned} &Th(t)+r(t) \\ &\quad \geq \frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{1} \bigl[(1-qs)^{( \gamma -\delta -1)}t^{\gamma -1}-(t-qs)^{(\gamma -1)} \bigr]f(s,0)\,d_{q}s \\ &\quad \geq \frac{1}{\Gamma _{q}(\gamma )} \int _{0}^{1} \bigl[(1-qs)^{( \gamma -\delta -1)}-(1-qs)^{(\gamma -1)} \bigr]t^{\gamma -1}f(s,0)\,d_{q}s \\ &\quad = \frac{h(t)}{\mathcal{H}\Gamma _{q}(\gamma )} \int _{0}^{1} \bigl[(1-qs)^{( \gamma -\delta -1)}-(1-qs)^{(\gamma -1)} \bigr]f(s,0)\,d_{q}s. \end{aligned}$$

Let

$$\begin{aligned} &\mu = \biggl[\frac{1}{\mathcal{H}\Gamma _{q}(\gamma )}+ \frac{a}{\mathcal{H}(1-a\eta ^{\gamma -\delta -1})\Gamma _{q}(\gamma )} \biggr] \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}f(s, \mathcal{H})\,d_{q}s, \\ &\nu = \frac{1}{\mathcal{H}\Gamma _{q}(\gamma )} \int _{0}^{1} \bigl[(1-qs)^{( \gamma -\delta -1)}-(1-qs)^{(\gamma -1)} \bigr]f(s,0)\,d_{q}s. \end{aligned}$$

Under the conditions of \(\Gamma _{q}(\gamma )>0\), \(\mathcal{H}>0\), and assumptions \((H_{1})\), \((H_{3})\), we can obtain

$$\begin{aligned} \int _{0}^{1}(1-qs)^{(\gamma -\delta -1)}f(s, \mathcal{H})\,d_{q}s\geq \int _{0}^{1} \bigl[(1-qs)^{(\gamma -\delta -1)}-(1-qs)^{(\gamma -1)} \bigr]f(s,0)\,d_{q}s>0, \end{aligned}$$

that is \(\mu \geq \nu >0\) holds. Therefore, we have \(Th+r\in P_{h}\).

Eventually, by Lemma 2.11, we obtain that the operator T has a unique fixed point \(\varpi ^{*}\in P_{h,r}\), and

$$\begin{aligned} \varpi ^{*}(t)& = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varpi ^{*}(s)\bigr)\,d_{q}s \\ &\quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varpi ^{*}(s)\bigr)\,d_{q}s-r(t), \quad t \in [0,1]. \end{aligned}$$

Consequently, \(\varpi ^{*}(t)\) is the unique solution of the BVP (1) in \(P_{h,r}\). For any \(\varphi _{0}\in P_{h,r}\), the sequence \(\varphi _{n}=T\varphi _{n-1}\), \(n=1,2,\dots \), satisfies \(\varphi _{n}\to \varpi ^{*}\) as \(n\to \infty \). That is,

$$\begin{aligned} \varphi _{n}(t)& = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varphi _{n-1}(s)\bigr)\,d_{q}s \\ &\quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varphi _{n-1}(s)\bigr)\,d_{q}s \\ &\quad{} - \frac{\xi (1-q)^{2}(1-a\eta ^{\gamma -\delta})}{(1-a\eta ^{\gamma -\delta -1})(1-q^{\gamma -1})(1-q^{\gamma -\delta})\Gamma _{q}(\gamma -1)}t^{ \gamma -1} \\ &\quad{} + \frac{\xi (1-q)^{2}}{(1-q^{\gamma})(1-q^{\gamma -1})\Gamma _{q}(\gamma -1)}t^{ \gamma}, \end{aligned}$$

where \(n=1,2,\dots \), and \(\varphi _{n}(t)\to \varpi ^{*}(t)\) as \(n\to \infty \). The proof is completed. □

Remark 3.7

Suppose the conditions of Theorem 3.6 hold and

$$\begin{aligned} &\int _{0}^{1}\overline{G}(t,qs)f(s,0)\,d_{q}s+ \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f(s,0)\,d_{q}s \\ &\quad \not \equiv \xi \int _{0}^{1}\overline{G}(t,qs)\,d_{q}s+ \frac{a\xi t^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)\,d_{q}s, \quad \forall t\in [0,1]. \end{aligned}$$

Then, the BVP (1) has a unique nontrivial solution in \(P_{h,r}\). Meanwhile, we can construct an iterative scheme approximating the unique solution.

Corollary 3.8

Suppose that \((H_{1})\)–\((H_{3})\) hold, then the BVP

$$\begin{aligned} \textstyle\begin{cases} (D_{q}^{\gamma}\varpi )(t)+f(t,\varpi (t))=\xi , \quad 0< t< 1, \\ \varpi (0)=(D_{q}\varpi )(0)=\cdots =(D_{q}^{n-2}\varpi )(0)=0,\qquad (D_{q}^{ \delta}\varpi )(1)=0, \end{cases}\displaystyle \end{aligned}$$

has a unique solution \(\varpi ^{*}\in P_{h,r}\), where h, r are given as in (2) and (3). Further, for any \(\varphi _{0}\in P_{h,r}\), making an iterative sequence

$$\begin{aligned} \varphi _{n}(t)& = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varphi _{n-1}(s)\bigr)\,d_{q}s- \frac{\xi (1-q)^{2}}{(1-q^{\gamma -1})(1-q^{\gamma -\delta})\Gamma _{q}(\gamma -1)}t^{ \gamma -1} \\ &\quad{} + \frac{\xi (1-q)^{2}}{(1-q^{\gamma})(1-q^{\gamma -1})\Gamma _{q}(\gamma -1)}t^{ \gamma}, \quad n=1,2,\dots , \end{aligned}$$

we have \(\varphi _{n}(t)\to \varpi ^{*}(t)\) as \(n\to \infty \).

If \(\xi =0\), we can obtain the uniqueness of positive solutions for the BVP (1) by using Lemma 2.12. The proof is similar to Theorem 3.6.

Theorem 3.9

Suppose that \((H_{4})\)–\((H_{6})\) are satisfied, and \(\xi =0\). Then, the BVP (1) has a unique positive solution \(\varpi ^{*}\) in \(P_{h}\), where \(h(t)=t^{\gamma -1}\), \(t\in [0,1]\). Moreover, for any initial value \(\varphi _{0}\in P_{h}\), from the sequence

$$\begin{aligned} \varphi _{n}(t)& = \int _{0}^{1}\overline{G}(t,qs)f\bigl(s,\varphi _{n-1}(s)\bigr)\,d_{q}s \\ &\quad{} + \frac{at^{\gamma -1}}{(1-a\eta ^{\gamma -\delta -1})[\gamma -1]_{q}} \int _{0}^{1}\overline{H}(\eta ,qs)f\bigl(s, \varphi _{n-1}(s)\bigr)\,d_{q}s,\quad n=1,2, \dots , \end{aligned}$$

we obtain \(\varphi _{n}(t)\to \varpi ^{*}(t)\) as \(n\to \infty \).

4 Application example

To illustrate the main result, we present in this section one significant example.

Example 4.1

Consider the following BVP:

$$\begin{aligned} \textstyle\begin{cases} (D_{q}^{\frac{9}{2}} \varpi )(t)+f(t,\varpi (t))=1, \quad 0< t< 1, \\ \varpi (0)=(D_{q}\varpi )(0)=(D_{q}^{2}\varpi )(0)=(D_{q}^{3}\varpi )(0)=0,\qquad (D_{q}^{ \frac{5}{2}}\varpi )(1)=\frac{1}{2}(D_{q}^{\frac{5}{2}}\varpi )( \frac{1}{2}), \end{cases}\displaystyle \end{aligned}$$
(5)

where

$$\begin{aligned} f(t,\varpi )&= \biggl\{ \biggl(\varpi + \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})} \biggr)t^{ \frac{7}{2}} \\ &\quad {}- \biggl( \frac{4{,}700{,}016+438{,}912\sqrt{2}}{7{,}268{,}464+454{,}279\sqrt{2}}\varpi + \frac{16{,}448+1536\sqrt{2}}{64{,}897\Gamma _{q}(\frac{7}{2})} \biggr)t^{ \frac{9}{2}} \biggr\} ^{\frac{1}{3}}, \end{aligned}$$

and \(q=\frac{1}{2}\), \(\gamma =\frac{9}{2}\), \(\delta =\frac{5}{2}\), \(a=\eta = \frac{1}{2}\), \(\xi =1\). It can be easily seen that

$$\begin{aligned} r(t)=\frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})}t^{ \frac{7}{2}}-\frac{16{,}448+1536\sqrt{2}}{64{,}897\Gamma _{q}(\frac{7}{2})}t^{ \frac{9}{2}},\qquad h(t)=\mathcal{H}t^{\frac{7}{2}}, \end{aligned}$$

where \(\mathcal{H}\geq \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})}\), for any \(t\in (0,1)\).

Then, we obtain

$$\begin{aligned} r(t)\geq \frac{10{,}273{,}792+61{,}468\sqrt{2}}{74{,}177{,}271\Gamma _{q}(\frac{7}{2})}t^{ \frac{7}{2}}\geq 0 \end{aligned}$$

and

$$\begin{aligned} r(t)\leq \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})}t^{ \frac{7}{2}}\leq \mathcal{H}t^{\frac{7}{2}}=h(t). \end{aligned}$$

Moreover, \(\hat{r}(t)=\frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})}\) for \(t\in [0,1]\). We see that \(f:[0,1]\times [- \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})},+\infty ) \to (-\infty ,+\infty )\) is continuous and increasing with respect to the second variable, and

$$\begin{aligned} f(t,0)= \biggl(\frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})}t^{ \frac{7}{2}}-\frac{16{,}448+1536\sqrt{2}}{64{,}897\Gamma _{q}(\frac{7}{2})}t^{ \frac{9}{2}} \biggr)^{\frac{1}{3}}=\bigl(r(t)\bigr)^{\frac{1}{3}}\geq 0, \end{aligned}$$

with \(f(t,0)\not \equiv 0\), \(t\in [0,1]\). Thus, the conditions \((H_{1})\) and \((H_{3})\) are satisfied.

It is apparent that

$$\begin{aligned} f\bigl(t,\varpi (t)\bigr)= \biggl[\frac{r(t)}{\hat{r}}\varpi (t)+r(t) \biggr]^{ \frac{1}{3}} \end{aligned}$$

and

$$\begin{aligned} \frac{r(t)}{\hat{r}}=t^{\frac{7}{2}}- \frac{4{,}700{,}016+438{,}912\sqrt{2}}{7{,}268{,}464+454{,}279\sqrt{2}}t^{\frac{9}{2}}, \quad t \in [0,1]. \end{aligned}$$

Using Remark 4 in [20], we have

$$\begin{aligned} f\bigl(t,\lambda x+(\lambda -1)y\bigr)\geq \psi (\lambda )f(t,x), \quad \lambda \in (0,1), x \in (-\infty ,+\infty ), y\in [0,\hat{r}], \end{aligned}$$

where \(\psi (\lambda )=\lambda ^{\frac{1}{3}}>\lambda \), \(\lambda \in (0,1)\), it follows that the condition \((H_{2})\) is satisfied. According to Theorem 3.6, the BVP (5) has a unique solution \(\varpi ^{*}\in P_{h,r}\). For \(\varphi _{0}\in P_{h,r}\), if

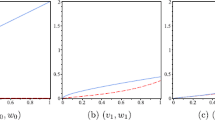
$$\begin{aligned} \varphi _{n}(t)& = \int _{0}^{1}\overline{G}(t,qs) \biggl\{ \biggl(\varphi _{n-1}(s)+ \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})} \biggr)t^{ \frac{7}{2}} \\ &\quad{} - \biggl(\frac{4{,}700{,}016+438{,}912\sqrt{2}}{7{,}268{,}464+454{,}279\sqrt{2}}\varphi _{n-1}(s)+ \frac{16{,}448+1536\sqrt{2}}{64{,}897\Gamma _{q}(\frac{7}{2})} \biggr)t^{ \frac{9}{2}} \biggr\} ^{\frac{1}{3}}\,d_{q}s \\ &\quad{} + \frac{128+8\sqrt{2}}{381}t^{\frac{7}{2}} \int _{0}^{1}\overline{H} \biggl( \frac{1}{2},qs \biggr) \times \biggl\{ \biggl(\varphi _{n-1}(s)+ \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})} \biggr)t^{ \frac{7}{2}} \\ &\quad{} - \biggl(\frac{4{,}700{,}016+438{,}912\sqrt{2}}{7{,}268{,}464+454{,}279\sqrt{2}}\varphi _{n-1}(s)+ \frac{16{,}448+1536\sqrt{2}}{64{,}897\Gamma _{q}(\frac{7}{2})} \biggr)t^{ \frac{9}{2}} \biggr\} ^{\frac{1}{3}}\,d_{q}s \\ &\quad{} - \frac{448+28\sqrt{2}}{1143\Gamma _{q}(\frac{7}{2})}t^{\frac{7}{2}}+ \frac{16{,}448+1536\sqrt{2}}{64{,}897\Gamma _{q}(\frac{7}{2})}t^{ \frac{9}{2}},\quad n=1,2,\dots , \end{aligned}$$

we have \(\varphi _{n}(t)\to \varpi ^{*}(t)\) as \(n\to \infty \), \(t\in [0,1]\).

5 Conclusion

This research establishes the existence and uniqueness results of solutions for the BVPs of a high-order nonlinear fractional q-difference equation, according to a novel fixed-point theorem of increasingly \(\psi -(h,r)\)-concave operators defined in ordered sets, we approach the unique solution by constructing an iterative sequence, which enriches the methods to solve the boundary value problems of fractional q-difference equations, and provides the theoretical guarantee for the application of fractional q-difference equations in fields such as aerodynamics, the electrodynamics of complex medium, capacitor theory, electrical circuits, control theory, and so on. This paper does not need to limit the existence of upper and lower solutions, which is the advantage of this paper compared with other articles. In the future, we are committed to finding new ways to continue our research.