Common fixed-point results of fuzzy mappings and applications on stochastic Volterra integral equations

Abstract

The objective of the present research is to establish and prove some new common fuzzy fixed-point theorems for fuzzy set-valued mappings involving Θ-contractions in a complete metric space. For this purpose, a novel integral-type contraction condition is applied to obtain these results. In this way, several useful and classical results have been generalized. Moreover, a concrete example is created to furnish our results. An application to stochastic Volterra integral equations has been given to enhance the validity of our results.

1 Introduction

The fuzzy logics were created using a group structure with ambiguous knowledge. Due to the flexibility of FSs in dealing with unreliability, this is even better for humanistic logic based on authentic reality and limitless knowledge. This notion is unquestionably a basic aspect of classical sets. Another important feature of this information is that it enables evaluation of the negative and positive elements of incorrect notions. Fuzzy mathematics is an area of mathematics that deals with FS theory. Zadeh [32] in 1965 proposed FSs to demonstrate knowledge/analysis with nonstatistical uncertainty. Many developments and generalizations in FS theory have been made in the last few years; for further information, please see [19, 31, 33–38] and references therein. Across chemistry, biology, technology, mathematical analysis, machine intelligence, mechanical theory, and several other subjects, FS theory has a wide range of applications. In the study of mathematical analysis, FP results offer optimum conditions for simulating the solutions of linear and nonlinear operator equations. In 1922, Banach [14] proposed and demonstrated a theorem that guaranteed the existence and uniqueness of a FP in a CMS X of the self-map f on X with contractive condition $d(f\mu , f\nu )\leq \alpha \,d(\mu , \nu )$, where $\alpha \in (0,1)$. This result is known as Banach’s FP theorem. By introducing the concept of fuzzy contraction mappings in association with the $d_{\infty}$-metric for FSs, Heilpern [22] provided a fuzzy extension of the Banach [14] and Nadler [29] FP theorems. Following this conclusion, several authors (e.g., [4, 7–13, 25, 26]) generalized it and investigated the presence of (common) FPs of fuzzy approximate quantity-valued mappings meeting contractive class conditions on metric-linear spaces.

Branciari [16] introduced FPs of mappings that satisfy integral-type contractive conditions. Namely, given a MS $(X, d)$, Branciari considered a self-mapping T on X satisfying the contractive criteria of the form

$$ \int _{0}^{d(Tx, Ty)}\Delta (t)\,dt \leq \lambda \int _{0}^{d(x, y)} \Delta (t)\,dt $$

for all $x,y \in X$, where $\lambda \in (0,1)$ and $\Delta : [0,\infty ) \rightarrow [0,\infty )$ is a Lebesgue integrable function and is summable on every compact subset of $[0,\infty )$ and satisfies $\int _{0}^{\epsilon}\Delta (t)\,dt > 0$, for all $\epsilon > 0$.

This paper is organized as follows: In Sect. 2, some fundamental notions are reviewed, including FM, fuzzy FP, the Hausdorff metric, Θ-contraction, etc. In Sect. 3, the existence of common fuzzy FPs of fuzzy functions for Θ-contractions in connection with integral-type contractions are established. Moreover, a significant example is constructed for the validity of the results. Section 4 gives an application of our research work. In Sect. 5, some concluding remarks and future directions are given.

2 Preliminaries

This section recalls some fundamental notions, like fuzzy set (FS), fuzzy mapping (FM), fuzzy fixed point (FFP), fuzzy coincidence point, the Hausdorff metric, Θ-contraction, etc. Let $(X,d)$ be a metric space (MS). Let $\operatorname{CB}(X)$ be the collection of all closed and bounded subsets of X.

Definition 2.1

([15])

For $M,N\in \operatorname{CB}(X)$, take

$$\begin{aligned}& d(x,M)=\inf _{y\in M}d(x, y),\\& d(M,N)=\inf _{x\in M, y\in N}d(x, y). \end{aligned}$$

The Hausdorff metric H on $\operatorname{CB} ( X )$ induced by d is defined by

$$ H ( M,N ) =\max \bigl\{ \sup _{a\in M} d ( a,N ) ,\sup_{b\in N} d ( M,b ) \bigr\} . $$

Let Π be the class of functions $\Delta : [0,\infty ) \rightarrow [0,\infty )$ so that:

• (i) Δ is Lebesgue integrable and summable on each compact subset of $[0,\infty )$;

• (ii) $\int _{0}^{\tau}\Delta (\upsilon )\,d\upsilon >0$, for each $\tau > 0$.

Definition 2.2

([32])

Let X be a nonempty set. A fuzzy set P in X is characterized by a membership (characteristic) function $f_{P} (x)$ that associates with each point in X, a real number in $[0, 1]$. Let $\mathcal{F}(X)$ be the family of all FSs in X. If P is a FS and $x\in X$, then the functional values $P(x)$ are called the grade of membership of x in P.

Definition 2.3

([22])

The α-level set of a FS P in X, denoted by $[ P ] _{\alpha }$, is defined by

$$\begin{aligned}& [ P ] _{\alpha }=\bigl\{ x \in X: P(x)\geqslant \alpha \bigr\} \quad \text{if } \alpha \in (0,1],\\& [ P ] _{0}=\overline{\bigl\{ x \in X: P(x)>0\bigr\} }. \end{aligned}$$

Here, N̅ denotes the closure of N. For a subset P of X, the characteristic function of P is denoted by $\chi _{P}$.

A FS P in a metric-linear space V is said to be an approximate quantity if and only if $[ P ] _{\alpha}$ is compact and convex in V for each $\alpha \in (0,1]$ and $\sup_{x\in V}P(x)=1$.

Some subcollections of $\mathcal{F}_{L}(X)$ and $\mathcal{F}_{L}(V)$ are defined as follows:

$$\begin{aligned}& \mathcal{W}(V)= \bigl\{ P\in \mathcal{F}(V):P \text{ is an approximate quantity in } V \bigr\} ,\\& \mathfrak{C} ( X ) = \bigl\{ P\in \mathcal{F}(X): [ P ] _{\alpha }\in \operatorname{CB} ( X ) ,\text{ for each } \alpha \in (0,1] \bigr\} . \end{aligned}$$

For $P,B\in \mathcal{F}(X)$, $P\subset B$ means $P(x)\leq B(x)$ for each $x\in X$. If there is $\alpha \in (0,1]$ so that $[ P ] _{\alpha }, [ B ] _{\alpha }\in \operatorname{CB} ( X )$, then define

$$\begin{aligned}& p_{\alpha }(P,B) = \inf_{x\in [ P ] _{\alpha },y\in [ B ] _{\alpha }} d(x, y), \\& D_{\alpha }(P,B) = H\bigl( [ P ] _{\alpha }, [ B ] _{ \alpha } \bigr). \end{aligned}$$

Let $\alpha \in (0,1]$. If $[ P ] _{\alpha }, [ B ] _{\alpha }\in \operatorname{CB} ( X )$, define $p,d_{\infty}:\mathfrak{C} ( X ) \times \mathfrak{C} ( X ) \rightarrow \mathbb{R}$ (induced by the Hausdorff metric H) by

$$\begin{aligned}& p(P,B) = \sup _{\alpha }p_{\alpha }(P,B), \\& d_{\infty}(P,B) = \sup _{\alpha }D_{\alpha }(P,B). \end{aligned}$$

Definition 2.4

([10])

For an arbitrary set W and a metric space X, a FM is a function F from W into $\mathcal{F}(X)$. A fuzzy mapping F is a fuzzy subset on $W\times X$ with membership function $F(x)(y)$. The functional-value $F(x)(y)$ is the grade of membership of y in $F(x)$. The family of all mappings from W into $\mathcal{F}(X)$ is denoted by $( \mathcal{F}(X) ) ^{W}$.

Definition 2.5

([10])

An α-fuzzy FP of FM S defined on a MS $(X, d)$ is an element $u\in X$ so that $u\in [Su]_{\beta}$, for some $\beta \in (0,1]$.

Definition 2.6

([10])

A common α-fuzzy FP of two FMs $F, T:W\rightarrow \mathcal{F}(X)$, is a point $u\in W$ if $u\in [Fu]_{\alpha}\cap [Tu]_{\alpha}$.

Definition 2.7

([24])

Consider a mapping Θ from $(0,\infty )$ to $(1,\infty )$ so that:

($\Theta _{1}$):

Θ is nondecreasing;

($\Theta _{2}$):

for any sequence $\{\gamma _{n}\}\subset (0,\infty )$, $\lim_{n\rightarrow \infty} \Theta (\gamma _{n})=1$ iff

$$ \lim_{n\rightarrow \infty}\gamma _{n}=0^{+}; $$

($\Theta _{3}$):

there are $u \in (0, 1)$ and $0 < l < \infty$ so that $\lim_{\gamma \rightarrow 0^{+}} \frac{\Theta (\gamma )-1}{\gamma ^{u}}=l$.

A function $T:X\rightarrow X$ is known as a Θ-contraction if there are Θ that satisfies $(\Theta _{1})-(\Theta _{3})$ and a number k between 0 and 1 so that for all $x, y\in X$,

$$ d(Tx, Ty) \neq 0 \quad \Rightarrow\quad \Theta \bigl(d(Tx, Ty)\bigr) \leq \bigl[\Theta \bigl(d(x, y)\bigr)\bigr]^{k}. $$

(1)

Theorem 2.1

([24])

Let $(X, d)$ be a CMS and $T:X\rightarrow X$ be a Θ-contraction, then T has a unique FP.

In 2017, Hancer et al. [21] added the following general condition $(\Theta _{4})$:

($\Theta _{4}$):

$\Theta (\inf J) = \inf \Theta (J)$, where $J\subset (0,\infty )$ with $\inf (J) > 0$.

The set of all continuous functions Θ satisfying $(\Theta _{1})-(\Theta _{4})$ is denoted by Ξ.

For further study on Θ-contractions, please see [2, 5, 23, 27, 30].

Lemma 2.1

([29])

For a MS X, let M and N be nonempty and belong to $\operatorname{CB}(X)$. If $m\in M$, then $d ( m,N ) \leqslant H ( M,N )$.

Lemma 2.2

([22])

For a complete metric-linear space $(V,d_{v})$ and a FM $T:V\longrightarrow \mathcal{W}(V)$, let $x_{0}\in V$. Then, there is $x_{1}\in X$ such that $\chi _{\{x_{1}\}}\subset T(x_{0})$.

Lemma 2.3

([28])

If $\{\rho _{n}\}$ is a sequence in $[0,\infty )$ and $\varphi \in \Phi$, then $\lim_{n\rightarrow \infty}\int _{0}^{\rho _{n}}\varphi (\upsilon )\,d\upsilon =0$ if and only if $\rho _{n}\rightarrow 0$ as $n\rightarrow \infty$.

3 Integral-type fuzzy fixed-point theorems

In this chapter, an integral-type contraction condition is used to establish some common fuzzy FPs of FS-valued mappings involving Θ-contractions in a MS.

Theorem 3.1

Let $(\Upsilon , d)$ be a CMS and $\Phi , \Psi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ be two FMs. Suppose for each $\mu \in \Upsilon$, there exist $\alpha _{\Phi (\mu )}$, $\alpha _{\Psi (\mu )}\in (0,1]$ such that $[\Phi \mu ]_{\alpha _{\Phi (\mu )}}$ and $[\Psi \mu ]_{\alpha _{\Psi (\mu )}}$ are nonempty, and belong to $\operatorname{CB}(\Upsilon )$. Assume that there are $\Theta \in \Xi$, $\Delta \in \Pi$ and $k\in (0,1)$ such that

$$ \int _{0}^{\Theta (H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [ \Psi \nu ]_{\alpha _{\Psi (\nu )}} ) )} \Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu , \nu ) ) ]^{k}} \Delta (t)\,dt $$

(2)

for all $\mu , \nu \in \Upsilon$ with $H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Psi \nu ]_{\alpha _{ \Psi (\nu )}} ) > 0$. Then, there is some $z\in \Upsilon$ such that $z\in [\Phi z]_{\alpha _{\Phi (z)}}\cap [\Psi z]_{\alpha _{\Psi (z)}}$.

Proof

Let $\mu _{0}\in \Upsilon$ be arbitrary. By hypothesis, there is $\alpha _{\Phi (\mu _{0})}\in (0,1]$ so that $[\Phi \mu _{0}]_{\alpha _{\Phi (\mu _{0})}}$ is a nonempty, bounded, and closed subset of ϒ. Take $\alpha _{\Phi (\mu _{0})}=\alpha _{1}$. Let $\mu _{1}\in [\Phi \mu _{0}]_{\alpha _{\Phi (\mu _{0})}}$. For this $\mu _{1}$, there is $\alpha _{\Psi (\mu _{1})}\in (0,1]$ so that $[\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}}$ is a nonempty, bounded, and closed subset of ϒ. Due to Lemma 2.1,

$$ \Theta \bigl(d\bigl(\mu _{1}, [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}} \bigr) \bigr) \leq \Theta \bigl(H \bigl([\Phi \mu _{0}]_{\alpha _{\Phi ( \mu _{0})}}, [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}} \bigr) \bigr). $$

(3)

From $(\Theta _{1})$, (2), and (3), we obtain

$$\begin{aligned} \int _{0}^{\Theta (d(\mu _{1}, [\Psi \mu _{1}]_{\alpha _{\Psi ( \mu _{1})}}) )} \Delta (t)\,dt & \leq \int _{0}^{\Theta (H ([\Phi \mu _{0}]_{\alpha _{\Phi (\mu _{0})}}, [\Psi \mu _{1}]_{ \alpha _{\Psi (\mu _{1})}} ) )} \Delta (t)\,dt \\ & \leq \int _{0}^{ [\Theta (d(\mu _{0}, \mu _{1}) ) ]^{k}} \Delta (t)\,dt. \end{aligned}$$

From $(\Theta _{4})$, we have

$$ \Theta \bigl(d\bigl(\mu _{1}, [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}} \bigr) \bigr) = \inf_{\nu \in [\Psi \mu _{1}]_{\alpha _{\Psi ( \mu _{1})}} } \Theta (d(\mu _{1}, \nu ). $$

Thus,

$$ \inf_{\nu \in [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}} } \Theta (d(\mu _{1}, \nu ) \leq \bigl[ \Theta \bigl(d( \mu _{0}, \mu _{1}) \bigr) \bigr]^{k}. $$

(4)

Now, from (4), there is $\mu _{2}\in [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}}$ such that

$$ \int _{0}^{\Theta (d(\mu _{1}, \mu _{2}) )}\Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu _{0}, \mu _{1}) ) ]^{k}}\Delta (t)\,dt. $$

(5)

For this $\mu _{2}$ there is $\alpha _{\Phi (\mu _{2})}\in (0,1]$ such that $[\Phi \mu _{2}]_{\alpha _{\Phi (\mu _{2})}}$ is a nonempty, bounded, and closed subset of ϒ. Due to Lemma 2.1,

$$ \Theta \bigl(d\bigl(\mu _{2}, [\Phi \mu _{2}]_{\alpha _{\Phi (\mu _{2})}} \bigr) \bigr) \leq \Theta \bigl(H \bigl([\Phi \mu _{2}]_{\alpha _{\Phi ( \mu _{2})}}, [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}} \bigr) \bigr). $$

(6)

From $(\Theta _{1})$, (2) and (6), we obtain

$$\begin{aligned} \int _{0}^{\Theta (d(\mu _{2}, [\Phi \mu _{2}]_{\alpha _{\Phi ( \mu _{2})}}) )} \Delta (t)\,dt & \leq \int _{0}^{\Theta (H ([\Phi \mu _{2}]_{\alpha _{\Phi (\mu _{2})}}, [\Psi \mu _{1}]_{ \alpha _{\Psi (\mu _{1})}} ) )} \Delta (t)\,dt \\ & \leq \int _{0}^{ [\Theta (d(\mu _{1}, \mu _{2}) ) ]^{k}} \Delta (t)\,dt. \end{aligned}$$

From $(\Theta _{4})$, we have

$$ \Theta \bigl(d\bigl(\mu _{1}, [\Psi \mu _{1}]_{\alpha _{\Psi (\mu _{1})}} \bigr) \bigr) = \inf_{\nu \in [\Psi \mu _{1}]_{\alpha _{\Psi ( \mu _{1})}} } \Theta (d(\mu _{1}, \nu ). $$

Thus,

$$ \inf_{\nu _{1}\in [\Phi \mu _{2}]_{\alpha _{\Phi (\mu _{2})}} } \Theta (d(\mu _{2}, \nu _{1} ) \leq \bigl[\Theta \bigl(d(\mu _{1}, \mu _{2}) \bigr) \bigr]^{k}. $$

(7)

Now, from (7), there is $\mu _{3}\in [\Phi \mu _{2}]_{\alpha _{\Phi (\mu _{2})}}$ so that

$$ \int _{0}^{\Theta (d(\mu _{2}, \mu _{3}) )}\Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu _{1}, \mu _{2}) ) ]^{k}}\Delta (t)\,dt. $$

(8)

Continuing this process, we generate a sequence $\{\mu _{n}\}$ in ϒ so that

$$ \mu _{2n+1}\in [\Phi \mu _{2n}]_{\alpha _{\Phi (\mu _{2n})}} $$

and

$$ \mu _{2n+2}\in [\Psi \mu _{2n+1}]_{\alpha _{\Psi (\mu _{2n+1})}} $$

with

$$ \int _{0}^{\Theta (d(\mu _{2n+1}, \mu _{2n+2}) )}\Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu _{2n}, \mu _{2n+1}) ) ]^{k}}\Delta (t)\,dt $$

(9)

and

$$ \int _{0}^{\Theta (d(\mu _{2n+2}, \mu _{2n+3}) )}\Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu _{2n+1}, \mu _{2n+2}) ) ]^{k}}\Delta (t)\,dt. $$

(10)

Combining (9) and (10), one writes

$$ \int _{0}^{\Theta (d(\mu _{n}, \mu _{n+1}) )}\Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu _{n-1}, \mu _{n}) ) ]^{k}}\Delta (t)\,dt, $$

(11)

which further implies that

$$\begin{aligned} \int _{0}^{\Theta (d(\mu _{n}, \mu _{n+1}) )}\Delta (t)\,dt & \leq \int _{0}^{ [\Theta (d(\mu _{n-1}, \mu _{n}) ) ]^{k}}\Delta (t)\,dt \\ & \leq \int _{0}^{ [\Theta (d(\mu _{n-2}, \mu _{n-1}) ) ]^{k^{2}}}\Delta (t)\,dt \\ & \leq \int _{0}^{ [\Theta (d(\mu _{n-3}, \mu _{n-2}) ) ]^{k^{3}}}\Delta (t)\,dt \\ &\cdots \\ & \leq \int _{0}^{ [\Theta (d(\mu _{0}, \mu _{1}) ) ]^{k^{n}}}\Delta (t)\,dt. \end{aligned}$$

Since $\Theta \in \Xi$, we have at the limit $n\rightarrow \infty$,

$$ \lim_{n\rightarrow \infty } \Theta \bigl(d(\mu _{n}, \mu _{n+1}) \bigr) = 1. $$

(12)

Thus,

$$ \lim_{n\rightarrow \infty } d(\mu _{n}, \mu _{n+1}) = 0^{+}, $$

(13)

by $(\Theta _{2})$. In view of $(\Theta _{3})$, there are $q\in (0,1)$ and $l\in (0,\infty ]$ so that

$$ \lim_{n\rightarrow \infty } \frac{\Theta (d(\mu _{n}, \mu _{n+1}) ) - 1}{[d(\mu _{n}, \mu _{n+1})]^{q}} = l. $$

(14)

Case 1. Let $l < \infty$ and $\frac{l}{2} = C > 0$. Hence, there is $n_{0}\in \mathbb{N}$ so that for all $n > n_{0}$,

$$ \biggl\vert \frac{\Theta (d(\mu _{n}, \mu _{n+1}) ) - 1}{[d(\mu _{n}, \mu _{n+1})]^{q}} - l \biggr\vert \leq C. $$

That is,

$$ \frac{\Theta (d(\mu _{n}, \mu _{n+1}) ) - 1}{[d(\mu _{n}, \mu _{n+1})]^{q}} \geq l - C = C. $$

Then,

$$ n\bigl[d(\mu _{n}, \mu _{n+1})\bigr]^{q} \leq Dn \bigl[\Theta \bigl(d(\mu _{n}, \mu _{n+1}) \bigr) - 1\bigr], $$

where $D = \frac{1}{C}$.

Case 2. Suppose $l = \infty$. Let $C>0$ be a real. Easily, there is $n_{0}\in \mathbb{N}$ so that

$$ C \leq \frac{\Theta (d(\mu _{n}, \mu _{n+1}) ) - 1}{[d(\mu _{n}, \mu _{n+1})]^{q}} $$

for all $n > n_{0}$. This implies that

$$ n\bigl[d(\mu _{n}, \mu _{n+1})\bigr]^{q} \leq Dn \bigl[\Theta \bigl(d(\mu _{n}, \mu _{n+1}) \bigr) - 1\bigr],\quad n> n_{0}, $$

where $D = \frac{1}{C}$. In both cases, there are $D > 0$ and $n_{0} \in \mathbb{N}$ so that for all $n > n_{0}$,

$$ n\bigl[d(\mu _{n}, \mu _{n+1})\bigr]^{q} \leq Dn \bigl[\Theta \bigl(d(\mu _{n}, \mu _{n+1}) \bigr) - 1\bigr]. $$

(15)

Now, we have

$$ n\bigl[d(\mu _{n}, \mu _{n+1})\bigr]^{q} \leq Dn \bigl(\bigl[\Theta \bigl(d(\mu _{0}, \mu _{1}) \bigr) \bigr]^{k^{n}} - 1 \bigr). $$

(16)

As $n\rightarrow \infty$, the above inequality yields that

$$ \lim_{n\rightarrow \infty}n\bigl[d(\mu _{n}, \mu _{n+1}) \bigr]^{q} = 0. $$

Hence, there is an integer $n_{1}$ so that for all $n > n_{1}$,

$$ n\bigl[d(\mu _{n}, \mu _{n+1})\bigr]^{q} \leq 1. $$

This implies that

$$ d(\mu _{n}, \mu _{n+1}) \leq \frac{1}{n^{\frac{1}{q}}} $$

for all $n > n_{1}$. Hence,

$$ \int _{0}^{d(\mu _{n}, \mu _{n+1})}\Delta (t)\,dt \leq \int _{0}^{ \frac{1}{n^{1/q}}}\Delta (t)\,dt $$

(17)

for all $n > n_{1}$. Now, to prove that $\{\mu _{n}\}$ is a Cauchy sequence, suppose $m,n\in \mathbb{N}$ such that $m > n > n_{1}$. We have

$$\begin{aligned} \int _{0}^{d(\mu _{n}, \mu _{m})}\Delta (t)\,dt &\leq \sum _{i=n}^{m-1} \int _{0}^{d(\mu _{i}, \mu _{i+1})}\Delta (t)\,dt \\ & \leq \sum _{i=n}^{m-1} \int _{0}^{\frac{1}{i^{1/q}}}\Delta (t)\,dt \leq \sum ^{\infty}_{i=n} \int _{0}^{\frac{1}{i^{1/q}}}\Delta (t)\,dt. \end{aligned}$$

(18)

Since $0< q<1$, the series $\sum_{i=n}^{\infty}\int _{0}^{\frac{1}{i^{1/q}}} \Delta (t)\,dt$ converges. When $n,m\rightarrow \infty$, we obtain $d(\mu _{n}, \mu _{m}) \rightarrow 0$. Hence, $\{\mu _{n}\}$ is a Cauchy sequence in $(\Upsilon , d)$. Since ϒ is complete, there is $z\in \Upsilon$ so that $\lim_{n\rightarrow \infty}\mu _{n}\rightarrow z$. Now, we will show that $z\in [\Psi z]_{\alpha _{\Psi (z)}}$. On the contrary, suppose that $z\notin [\Psi z]_{\alpha _{\Psi (z)}}$, then there are $p \in \mathbb{N}$ and a sequence $\{\mu _{n_{t}}\}$ of $\{\mu _{n}\}$ such that $d(\mu _{n_{t+1}}, [\Psi z]_{\alpha _{\Psi (z)}} ) > 0$ $\forall n_{t} \geq p$. By using $(\Theta _{1})$ and Lemma 2.1, we have

$$ \Theta \bigl[d\bigl(\mu _{n_{t+1}}, [\Psi z]_{\alpha _{\Psi (z)}} \bigr) \bigr] \leq \Theta \bigl[H \bigl([\Phi \mu _{2n_{t}}]_{\alpha _{ \Phi (\mu _{2n_{t}})}}, [\Psi z]_{\alpha _{\Psi (z)}} \bigr) \bigr]. $$

(19)

Now, from (2) and (19), we have

$$\begin{aligned} \int ^{\Theta [d(\mu _{n_{t+1}}, [\Psi z]_{\alpha _{\Psi (z)}} ) ]}_{0}\Delta (t)\,dt & \leq \int ^{\Theta [H ([\Phi \mu _{2n_{t}}]_{\alpha _{\Phi (\mu _{2n_{t}})}}, [\Psi z]_{\alpha _{ \Psi (z)}} ) ]}_{0}\Delta (t)\,dt \\ & \leq \int ^{ [\Theta (d(\mu _{2n_{t}}, z) ) ]^{k}}_{0} \Delta (t)\,dt. \end{aligned}$$

Letting $t\rightarrow \infty$, then by using the continuity of Θ, the above inequality implies that

$$ \int ^{\Theta [d(z, [\Psi z]_{\alpha _{\Psi (z)}} ) ]}_{0} \Delta (t)\,dt \leq 0. $$

That is,

$$ \Theta \bigl[d\bigl(z, [\Psi z]_{\alpha _{\Psi (z)}} \bigr) \bigr] \leq 0. $$

Hence, $z\in [\Psi z]_{\alpha _{\Psi (z)}}$. Similarly, $z\in [\Phi z]_{\alpha _{\Phi (z)}}$. Thus, $z\in [\Phi z]_{\alpha _{\Phi (z)}}\cap [\Psi z]_{\alpha _{\Psi (z)}}$. □

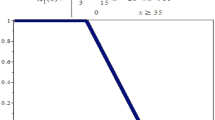
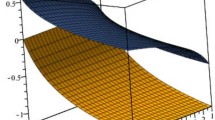
Example 3.1

Let $\Upsilon = [0,\infty )$ and define $d: \Upsilon \times \Upsilon \rightarrow \mathbb{R}_{+}$ by

$$ d(\mu ,\nu )= \vert \mu -\nu \vert . $$

Define two mappings $\Phi , \Psi : \Upsilon \rightarrow \mathcal{F}(\Upsilon )$ for $\alpha \in [0,1 ]$ as

$$ \Phi (\mu ) (t)= \textstyle\begin{cases} \alpha , & \text{if } 0\leq t \leq 2\mu , \\ \frac{\alpha}{2}, & \text{if } 2\mu < t \leq 6\mu , \\ \frac{\alpha}{6}, & \text{if } 6\mu < t \leq 10\mu , \\ \frac{\alpha}{10}, & \text{if } 10\mu < t < \infty , \end{cases} $$

and

$$ \Psi (\mu ) (t)= \textstyle\begin{cases} \alpha , & \text{if } 0\leq t \leq 3\mu , \\ \frac{\alpha}{3}, & \text{if } 3\mu < t \leq 6\mu , \\ \frac{\alpha}{6}, & \text{if } 6\mu < t \leq 9\mu , \\ \frac{\alpha}{9}, & \text{if } 9\mu < t < \infty . \end{cases} $$

The α-level sets are

$$\begin{aligned}& [\Phi \mu ]_{\alpha} = [0,2\mu ],\\& [\Psi \mu ]_{\alpha} = [0,3\mu ]. \end{aligned}$$

Consider $\Theta (t) = 2^{\sqrt[k]{t}}$. Then, there is some $k=\frac{1}{\sqrt{3}}\in (0,1 )$ such that

$$ \int _{0}^{\Theta (H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [ \Psi \nu ]_{\alpha _{\Psi (\nu )}} ) )} \Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu , \nu ) ) ]^{k}} \Delta (t)\,dt $$

for all $\mu , \nu \in \Upsilon$ with $H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Psi \nu ]_{\alpha _{ \Psi (\nu )}} ) > 0$. Hence, Theorem 3.1 can be applied to find $0\in \Upsilon$ such that $0\in [\Phi 0]_{\alpha} \cap [\Psi 0]_{\alpha}$.

Corollary 3.1

Let $(\Upsilon , d)$ be a CMS and $\Phi , \Psi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ be two fuzzy maps. Suppose for each $\mu \in \Upsilon$, there exist $\alpha _{\Phi (\mu )}$, $\alpha _{\Psi (\mu )}\in (0,1]$ such that $[\Phi \mu ]_{\alpha _{\Phi (\mu )}}$ and $[\Psi \mu ]_{\alpha _{\Psi (\mu )}}$ are nonempty, and belong to $\operatorname{CB}(\Upsilon )$. Assume that there are $\Theta \in \Xi$ and $k\in (0,1)$ so that

$$ \Theta \bigl(H \bigl([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Psi \nu ]_{ \alpha _{\Psi (\nu )}} \bigr) \bigr) \leq \bigl[\Theta \bigl(d( \mu , \nu ) \bigr) \bigr]^{k} $$

(20)

for all $\mu , \nu \in \Upsilon$ with $H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Psi \nu ]_{\alpha _{ \Psi (\nu )}} ) > 0$. Then, there is $z\in \Upsilon$ so that $z\in [\Phi z]_{\alpha _{\Phi (z)}}\cap [\Psi z]_{\alpha _{\Psi (z)}}$.

Proof

By letting $\Delta (t)\equiv 1$ in Theorem 3.1, we will obtain the required result. □

Theorem 3.2

Let $(\Upsilon , d)$ be a CMS and $\Phi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ be a FM. Suppose for each $\mu \in \Upsilon$, there is $\alpha _{\Phi (\mu )}\in (0,1]$ such that $[\Phi \mu ]_{\alpha _{\Phi (\mu )}}$ is nonempty, and belongs to $\operatorname{CB}(\Upsilon )$. If there are $\Theta \in \Xi$, $\Delta \in \Pi$ and $k\in (0,1)$ so that for all $\mu , \nu \in \Upsilon$,

$$ \int _{0}^{\Theta (H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [ \Phi \nu ]_{\alpha _{\Phi (\nu )}} ) )} \Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu , \nu ) ) ]^{k}} \Delta (t)\,dt $$

(21)

with $H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Phi \nu ]_{\alpha _{ \Phi (\nu )}} ) > 0$, then there is $z\in \Upsilon$ so that $z\in [\Phi z]_{\alpha _{\Phi (z)}}$.

Corollary 3.2

Let $(\Upsilon , d)$ be a CMS and $\Phi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ be a FM. Suppose for each $\mu \in \Upsilon$, there are $\alpha _{\Phi (\mu )}\in (0,1]$ such that $[\Phi \mu ]_{\alpha _{\Phi (\mu )}}$ is nonempty, and belong to $\operatorname{CB}(\Upsilon )$. If there are $\Theta \in \Xi$ and $k\in (0,1)$ so that

$$ \Theta \bigl(H \bigl([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Phi \nu ]_{ \alpha _{\Phi (\nu )}} \bigr) \bigr) \leq \bigl[\Theta \bigl(d( \mu , \nu ) \bigr) \bigr]^{k} $$

(22)

for all $\mu , \nu \in \Upsilon$ with $H ([\Phi \mu ]_{\alpha _{\Phi (\mu )}}, [\Phi \nu ]_{\alpha _{ \Phi (\nu )}} ) > 0$, then there is $z\in \Upsilon$ so that $z\in [\Phi z]_{\alpha _{\Phi (z)}}$.

Proof

Put $\Delta (t)=1$ in Theorem 3.2 to obtain the required result. □

Now, we will establish common FP results.

Theorem 3.3

Let $(\Upsilon , d)$ be a CMS and $A, B:\Upsilon \rightarrow \mathcal{CB}(\Upsilon )$ be two multivalued maps. Assume that there are $\Theta \in \Xi$, $\Delta \in \Pi$ and $k\in (0,1)$ so that for all $\mu , \nu \in \Upsilon$,

$$ \int ^{\Theta (H (A\mu , B\nu ) )}_{0}\Delta (t)\,dt \leq \int ^{ [\Theta (d(\mu , \nu ) ) ]^{k}}_{0} \Delta (t)\,dt $$

(23)

with $H (A\mu , B\nu ) > 0$. Then, there is some $z\in \Upsilon$ such that $z\in Az\cap Bz$.

Proof

Consider $\alpha :\Upsilon \rightarrow (0,1]$. Let $\Phi ,\Psi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ be two fuzzy maps defined by

$$ \Phi (\mu ) (t)= \textstyle\begin{cases} \alpha (t), & \text{if } t \in A\mu , \\ 0, & \text{if } t \notin A\mu \end{cases} $$

and

$$ \Psi (\mu ) (t)= \textstyle\begin{cases} \alpha (t), & \text{if } t \in B\mu , \\ 0, & \text{if } t \notin B\mu . \end{cases} $$

Then,

$$ [\Phi \mu ]_{\alpha (\mu )} = \bigl\{ t : \Phi (\mu ) (t) \geq \alpha ( \mu ) \bigr\} = A\mu $$

and

$$ [\Psi \mu ]_{\alpha (\mu )} = \bigl\{ t : \Psi (\mu ) (t) \geq \alpha ( \mu ) \bigr\} = B\mu . $$

Thus, Theorem 3.1 can be applied to obtain $z\in \Upsilon$ so that

$$ z\in [\Phi z]_{\alpha (z)}\cap [\Psi z]_{\alpha (z)} = Az\cap Bz. $$

 □

Corollary 3.3

Let $(\Upsilon , d)$ be a CMS and $A, B:\Upsilon \rightarrow \mathcal{CB}(\Upsilon )$ be two multivalued maps. If there are $\Theta \in \Xi$ and $k\in (0,1)$ so that

$$ \Theta \bigl(H (A\mu , B\nu ) \bigr) \leq \bigl[ \Theta \bigl(d(\mu , \nu ) \bigr) \bigr]^{k} $$

(24)

for all $\mu , \nu \in \Upsilon$ with $H (A\mu , B\nu ) > 0$, then there is some $z\in \Upsilon$ so that $z\in Az\cap Bz$.

Proof

By considering $\Delta (t) = 1$ in Theorem 3.3, we will obtain the required result. □

Corollary 3.4

Let $(\Upsilon , d)$ be a CMS and $A:\Upsilon \rightarrow \mathcal{CB}(\Upsilon )$ be a multivalued map. If there are $\Theta \in \Xi$, $\Delta \in \Pi$ and $k\in (0,1)$ so that

$$ \int ^{\Theta (H (A\mu , A\nu ) )}_{0}\Delta (t)\,dt \leq \int ^{ [\Theta (d(\mu , \nu ) ) ]^{k}}_{0} \Delta (t)\,dt $$

(25)

for all $\mu , \nu \in \Upsilon$ with $H (A\mu , A\nu ) > 0$, then there is $z\in \Upsilon$ so that $z\in Az\cap Bz$.

Theorem 3.4

Let $(\Upsilon , d)$ be a complete metric-linear space and $\Phi , \Psi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ be two fuzzy maps. If there are $\Theta \in \Xi$, $\Delta \in \Pi$ and $k\in (0,1)$ so that

$$ \int _{0}^{\Theta (d_{\infty} (\Phi (\mu ), \Psi (\nu ) ) )} \Delta (t)\,dt \leq \int _{0}^{ [\Theta (p( \mu , \nu ) ) ]^{k}} \Delta (t)\,dt $$

(26)

for all $\mu , \nu \in \Upsilon$ with $d_{\infty} (\Phi (\mu ), \Psi (\nu ) ) > 0$, then there is $z\in \Upsilon$ so that $\{z\}\subset \Phi (z)$ and $\{z\}\subset \Psi (z)$.

Proof

Consider $\mu \in \Upsilon$, Lemma 2.2 implies that there is some $\nu \in \Upsilon$ such that $\nu \in [\Phi \mu ]_{1}$. Also, we can find $w\in \Upsilon$ such that $w\in [\Psi \mu ]_{1}$. Hence, for each $\mu \in \Upsilon$, $[\Phi \mu ]_{\alpha (\mu )}$ and $[\Psi \mu ]_{\alpha (\mu )}$ are nonempty, and belong to $\operatorname{CB}(\Upsilon )$. Since $\alpha (\mu ) = \alpha (\nu ) = 1$, one writes

$$ H \bigl([\Phi \mu ]_{\alpha (\mu )}, [\Psi \nu ]_{\alpha (\nu )} \bigr) \leq d_{\infty} \bigl(\Phi (\mu ), \Psi (\nu ) \bigr) $$

∀ $\mu ,\nu \in \Upsilon$. Since Θ is nondecreasing, one obtains

$$\begin{aligned} \Theta \bigl(H \bigl([\Phi \mu ]_{\alpha (\mu )}, [\Psi \nu ]_{ \alpha (\nu )} \bigr) \bigr) & \leq \Theta \bigl(d_{\infty} \bigl( \Phi (\mu ), \Psi (\nu ) \bigr) \bigr) \\ & \leq \bigl[\Theta \bigl(p (\mu ,\nu ) \bigr) \bigr]^{k} \end{aligned}$$

for all $\mu ,\nu \in \Upsilon$. This implies that

$$\begin{aligned} \int _{0}^{\Theta (H ([\Phi \mu ]_{\alpha (\mu )}, [\Psi \nu ]_{\alpha (\nu )} ) )} \Delta (t)\,dt & \leq \int _{0}^{ \Theta (d_{\infty} (\Phi (\mu ), \Psi (\nu ) ) )} \Delta (t)\,dt \\ & \leq \int _{0}^{ [\Theta (p (\mu ,\nu ) ) ]^{k}} \Delta (t)\,dt \end{aligned}$$

for all $\mu ,\nu \in \Upsilon$. Now, since $[\Phi \mu ]_{1} \subseteq [\Phi \mu ]_{\alpha}$ for any $\alpha \in (0,1]$, and so $d (\mu , [\Phi \mu ]_{\alpha} ) \leq d (\mu , [ \Phi \mu ]_{1} )$, for every $\alpha \in (0,1]$. Thus, we have $p (\mu , \Phi (\mu ) ) \leq d (\mu , [\Phi \mu ]_{1} )$. Similarly, $p (\mu , \Psi (\mu ) ) \leq d (\mu , [\Psi \mu ]_{1} )$.

Moreover,

$$ \int _{0}^{\Theta (H ([\Phi \mu ]_{1}, [\Psi \nu ]_{1} ) )} \Delta (t)\,dt \leq \int _{0}^{ [\Theta (d (\mu ,\nu ) ) ]^{k}} \Delta (t)\,dt. $$

Due to Theorem 3.1, we obtain $z\in \Upsilon$, so that $z\in [\Phi z]_{1}\cap [\Psi z]_{1}$, i.e., $\{z\}\subset \Phi (z) \text{and} \{z\}\subset \Psi (z)$. □

Here, we consider that Ψ̂ is the set-valued mapping induced from FM $\Psi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$, i.e.,

$$ \widehat{\Psi}\mu = \Bigl\{ \nu : \Psi (\mu ) (\nu ) = \max_{t \in \Upsilon} \Psi (\mu ) (t) \Bigr\} . $$

Corollary 3.5

Let $(\Upsilon , d)$ be a CMS. Consider two fuzzy maps $\Phi , \Psi :\Upsilon \rightarrow \mathcal{F}(\Upsilon )$ such that ∀ $\mu \in \Upsilon$, $\widehat{\Phi}(\mu )$, $\widehat{\Psi}(\mu )$ are nonempty, and belong to $\operatorname{CB}(\Upsilon )$. Suppose there are $k\in (0,1)$, $\Delta \in \Pi$ and $\Theta \in \Xi$ such that

$$ \int _{0}^{\Theta (H (\widehat{\Phi}(\mu ), \widehat{\Psi}( \nu ) ) )}\Delta (t)\,dt \leq \int _{0}^{ [\Theta (d(\mu , \nu ) ) ]^{k}}\Delta (t)\,dt $$

for all $\mu ,\nu \in \Upsilon$ with $H (\widehat{\Phi}(\mu ), \widehat{\Psi}(\nu ) ) > 0$. Then, there is a point $u\in \Upsilon$ such that $\Phi (u)(u) \geq \Phi (u)(\mu )$ and $\Psi (u)(u) \geq \Psi (u)(\mu )$ $\forall \mu \in \Upsilon$.

Proof

From Theorem 3.3, we obtain $u\in \Upsilon$ such that $u\in \widehat{\Phi}(u)\cap \widehat{\Psi}(u)$. Then, by Lemma 2.2, we have $\Phi (u)(u) \geq \Phi (u)(\mu )$ and $\Psi (u)(u) \geq \Psi (u)(\mu )$ $\forall \mu \in \Upsilon$. □

4 Application to stochastic Volterra integral equations

Stochastic integral equations arise in nearly every field of science and engineering. In recent time, researchers are becoming more interested in developing and unifying the concepts of probability theory and functional analysis, thereby establishing a variety of methods for studying the existence of solutions of integrodifferential equations (e.g., see [1, 3, 6]). However, problems abound that can be solved more effectively by the use of FS techniques than by classical probability-based methods [18, 32]. In continuation of this development, in this section, we investigate the existence of a common solution of a system of stochastic Volterra integral equations by using the idea of fuzzy maps.

With respect to our main objective here, a note on notations is in order. The stochastic integral equations and the notations are recorded randomly from [3, 17, 20] as follows. Denote by $(\Omega , \mathfrak{A}, \mathfrak{P} )$, a probability measure space, where Ω is a nonempty set, $\mathfrak{A}$ is a σ-algebra of subsets of Ω, and $\mathfrak{P}$ is a complete probability measure on $\mathfrak{A}$. Let $\mathbb{R}_{+}=[0, \infty )$. The space of all continuous and bounded functions on $\mathbb{R}_{+}$ with values in $L_{2}:=L_{2}(\Omega , \mathfrak{A}, \mathfrak{P})$ is represented by $C:= C (\mathbb{R}_{+}, L_{2}(\Omega , \mathfrak{A}, \mathfrak{P}) )$. We shall study the existence condition for a solution of the following system of Volterra stochastic differential equations:

$$\begin{aligned}& \mu (t;\omega ) = h(t;\omega )+ \int _{0}^{t}k_{1}(t, \zeta ;\omega )f \bigl( \zeta ,\mu (\zeta ;\omega )\bigr)\,d\zeta, \end{aligned}$$

(27)

$$\begin{aligned}& \mu (t;\omega )= h(t;\omega )+ \int _{0}^{t}k_{2}(t,\zeta ;\omega )f \bigl( \zeta ,\mu (\zeta ;\omega )\bigr)\,d\zeta , \end{aligned}$$

(28)

where $t\geq 0$ and (i) ω is a point of Ω, (ii) $h(t;\omega )$ is called the stochastic free term defined for $t\geq 0$, (iii) $\mu (t;\omega )$ is the unknown stochastic variable for each $t\geq 0$, (iv) $k_{1}$ and $k_{2}$ are stochastic kernels defined for $0\leq \zeta \leq t<\infty$, (v) $f(t, \mu )$ is a scalar function defined for $t\geq 0$. By a random solution $\mu (t;\omega )$ of the stochastic integral equations (27) and (28), we mean a function $\mu (t;\omega )$ that belongs to $C (\mathbb{R}_{+}, L_{2} (\Omega , \mathfrak{A}, \mathfrak{P} ) )$ and satisfies the equations a.e.

Theorem 4.1

Consider the system of Volterra stochastic integral equations (27) and (28). Assume that the following conditions hold:

• (i) $f:C\longrightarrow C$, $h:\mathbb{R}_{+}\longrightarrow L_{2}$, $k_{1}, k_{2}:\mathbb{R}_{+}\times \mathbb{R}_{+}\times L_{2} \longrightarrow L_{2}$ are continuous;

• (ii) $\|f(t, \mu (t;\omega ))-f(t, \nu (t;\omega ))\|\leq \|\mu (t;\omega )- \nu (t;\omega )\|$, where $\mu (t;\omega ), \nu (t;\omega )\in C$;

• (iii) There exist $\eta > 1$, $K>0$, $\lambda \geq 0$ and a nondecreasing function

  $\Theta : (0, \infty )\longrightarrow (1, \infty )$ satisfying

  $$ \int _{0}^{\Theta (\lambda K\|\mu -\nu \|)}\varphi (t)\,dt\leq \int _{0}^{[ \Theta (\|\mu -\nu \|)]^{\frac{1}{\eta}}}\varphi (t)\,dt,$$

  provided $\lambda < \frac{1}{K}$ and $\varphi \in \Phi$, where $K:=\|k_{1}(t, \zeta ,\omega )-k_{2}(t,\zeta ,\omega )\|$.

  Then, there exists a common random solution of equations (27) and (28) in C.

Proof

Let $\Upsilon = C:=C (\mathbb{R}_{+}, L_{2}(\Omega , \mathfrak{A}, \mathfrak{P}) )$ be endowed with the uniform norm $\|.\|$. Then, $(\Upsilon , \|.\|)$ is a Banach space. Assume that $k_{1}$, $k_{2}$ are such that $P_{\mu}, Q_{\mu}\in \Upsilon$, where

$$\begin{aligned}& P_{\mu}= h(t;\omega )+ \int _{0}^{t}k_{1}(t,\zeta ;\omega )f \bigl(\zeta , \mu (\zeta ;\omega )\bigr)\,d\zeta, \end{aligned}$$

(29)

$$\begin{aligned}& Q_{\mu}= h(t;\omega )+ \int _{0}^{t}k_{2}(t,\zeta ;\omega )f \bigl(\zeta , \mu (\zeta ;\omega )\bigr)\,d\zeta . \end{aligned}$$

(30)

Consider two arbitrary mappings $M, N: \Upsilon \longrightarrow (0, 1]$ and a pair of FMs $F, G:\Upsilon \longrightarrow \mathcal{F}(\Upsilon )$ defined as

$$ (F\mu ) (t)= \textstyle\begin{cases} M(\mu ), & \text{if } \mu (t)= P_{\mu }, \\ 0, & \text{otherwise}, \end{cases}\displaystyle \qquad (G\mu ) (t)= \textstyle\begin{cases} N(\mu ), & \text{if } \mu (t)= Q_{\mu }, \\ 0, & \text{otherwise}. \end{cases} $$

If we take $\alpha _{F(\mu )}= M(\mu )$ and $\alpha _{G(\mu )}= N(\mu )$, then we obtain

$$\begin{aligned}& [F\mu ]_{\alpha _{F(\mu )}}= \bigl\{ t: (F\mu ) (t)\geq M(\mu )\bigr\} = \{P_{\mu} \},\\& [G\mu ]_{\alpha _{G(\mu )}}= \bigl\{ t: (G\mu ) (t)\geq M(\mu )\bigr\} = \{Q_{\mu} \}. \end{aligned}$$

Therefore,

$$ H \bigl([F\mu ]_{\alpha _{F(\mu )}},[G\mu ]_{\alpha _{G(\mu )}} \bigr)= \Vert P_{\mu}-Q_{\mu} \Vert .$$

Since Θ is nondecreasing, we obtain using condition (ii),

$$ \Theta \bigl((\lambda K) \bigl\Vert f\bigl(t, \mu (\zeta ; \omega )\bigr)-f \bigl(t, \nu (\zeta ; \omega )\bigr) \bigr\Vert \bigr)\leq \Theta \bigl((\lambda K) \bigl\Vert \mu (\zeta ;\omega )-\nu ( \zeta ;\omega ) \bigr\Vert \bigr).$$

Consequently,

$$\begin{aligned} \int _{0}^{\Theta (H ([F\mu ]_{\alpha _{F(\mu )}},[G\mu ]_{ \alpha _{G(\mu )}} ) )}\varphi (t)\,dt =& \int _{0}^{ \Theta (\|P_{\mu}-Q_{\mu}\| )}\varphi (t)\,dt \\ \leq & \int _{0}^{\Theta (\int _{0}^{t} (k_{1}(t,\zeta , \omega )-k_{2}(t, \zeta ,\omega ) )\|f(\zeta ,\mu (\zeta ; \omega ))-f(\zeta ,\nu (\zeta ;\omega ))\|\,d\zeta )} \varphi (t)\,dt \\ \leq & \int _{0}^{\Theta ((\lambda K)\|\mu (\zeta ;\omega )- \nu (\zeta ;\omega )\| )} \varphi (t)\,dt \\ \leq & \int _{0}^{\Theta (\|\mu -\nu \| )^{ \frac{1}{\eta}}}\varphi (t)\,dt \\ \leq & \int _{0}^{ [\Theta (d(\mu , \nu )) ]^{ \frac{1}{\eta}}}\varphi (t)\,dt. \end{aligned}$$

Thus, for $k= \frac{1}{\eta}\in (0,1)$, all the conditions of Theorem 4.1 are satisfied to obtain $z\in \Upsilon$ such that $z\in [Fz]_{\alpha _{F}(z)}\cap [Gz]_{\alpha _{G(z)}}$, which corresponds to a common random solution of equations (27) and (28). □

5 Conclusion

FP theory plays an essential role in mathematics and applied sciences, such as mathematical modeling, optimization, economic theories and many more disciplines. Vagueness is an immense module in the life of an individual. To handle uncertainty in real-life problems, FS theory achieved a great success and popularity. Due to fuzzy techniques, outstanding results in science and technology are obtained that added an awesome modification in solving daily-life problems. In this paper, modern fuzzy techniques are applied in obtaining common FPs of two mutivalued mappings defined on a CMS. For this purpose, an integral-type Θ-contraction is applied. In this way, we have generalized many useful and practical results in the existing literature. The latest and classic results are presented as direct and indirect consequences of our results. A nontrivial and stimulating example is erected for embellishment of our main result. Moreover, to show the strength and importance of the research work, as an application we have investigated the existence of a common solution of a system of stochastic Volterra integral equations by using the idea of fuzzy maps.