1 Introduction


Semileptonic decays offer a unique laboratory to determine the weak \(b-u\) and \(b-c\) coupling, as they can be studied with good precision experimentally and their decay rates can be predicted reliably from theory. Inside the Standard Model of particle physics, the \(b \rightarrow q \ell \bar{\nu }_\ell\) process is generated by the four-Fermi operator of

$$\begin{aligned} \mathcal {O}_{\textrm{SM}} = 2 \sqrt{2} \, G_F \, V_{qb} \, \left( \overline{q} \, \gamma ^\mu \, P_L \, b \right) \left( \overline{\ell }\, \gamma _\mu \, P_L \, \nu _\ell \right) \, , \end{aligned}$$
(1)

which factorizes hadronic and leptonic currents at leading electroweak order and \(\ell\) represents either an electron or a muon. We also introduce the projection operator \(P_L = \frac{1}{2} ( 1 - \gamma ^5 )\) and Fermi’s constant, \(G_F^{-1} = 8 \, m_W^2 / (\sqrt{2} \, g_2^2) = \sqrt{2} \nu ^2\) with \(\nu = 246.22 \, \textrm{GeV}\) with \(g_2\) denoting the SU(2) weak copuling constant and \(V_{qb}\) denoting the corresponding quark-mixing Cabibbo–Kobayashi–Maskawa (CKM) matrix element. The determination of the CKM matrix elements \(|V_{ub}|\) and \(|V_{cb}|\) from exclusive processes requires the knowledge of several matrix elements, which can be represented by form factors. For instance, for semileptonic \(B \rightarrow P\) (pseudoscalar) and \(B \rightarrow V\) (vector) transitions two (\(f_+\), \(f_0\)) and four (\(g, f, a_+, a_-\)) independent form factors are present:

$$\begin{aligned} \langle P | \overline{q} \, \gamma ^\mu \, b | \overline{B} \rangle&= f_+ \left( p + p' \right) ^\mu + \left( f_0 - f_+ \right) \, q^\mu \, \frac{m_B^2 - m_P^2}{q^2} \, , \nonumber \\ \langle V | \overline{q} \, \gamma ^\mu \, b | \overline{B} \rangle&= 2 \, i \, g \, \varepsilon ^{\mu \nu \alpha \beta } \, \epsilon _\nu ^* \, p_\alpha ' \, p_\beta \, \nonumber \\ \langle V | \overline{q} \, \gamma ^\mu \, \gamma ^5 \, b | \overline{B} \rangle&= f \, e^{* \mu } + a_+ \, \epsilon ^* \cdot p \, \left( p + p' \right) ^\mu + a_- \, \left( \epsilon ^* \cdot p \right) q^\mu . \end{aligned}$$
(2)

Here p and \(p'\) denote the four-momentum of the initial and final state hadron and \(q^\mu = \left( p - p'\right) ^\mu\). Further \(\epsilon ^*\) denotes the polarization vector of the vector state and \(\varepsilon ^{\mu \nu \alpha \beta }\) the Levi–Civita tensor. The form factors encode the non-perturbative decay dynamics of the strong interaction in the decay process, and at least a single absolute normalization is required to predict the (partial) decay rate \(\varDelta \Gamma\) sans CKM factors and determine a value for \(|V_{ub}|\) and \(|V_{cb}|\) from measured (partial) branching fraction \(\varDelta \mathcal {B}\) via

$$\begin{aligned} |V_{xb}|&= \sqrt{ \frac{ \varDelta \mathcal {B} }{ \tau _b \, \varDelta \Gamma } } \, , \end{aligned}$$
(3)

with \(\tau _b\) denoting the lifetime of the beauty hadron under study.

The most precise determinations of \(|V_{ub}|\) rely on combining measured partial branching fractions of \(B \rightarrow \pi \ell \bar{\nu }_\ell\) from BaBar, Belle and Belle II and information from lattice QCD (LQCD) as a function of

$$\begin{aligned} q^2 = \left( p - p' \right) ^2 \, . \end{aligned}$$
(4)

The LHCb collaboration also carried out measurements using semileptonic decays of beauty baryons into protons, \(\Lambda _b \rightarrow p \mu \overline{\nu }_\mu\) [1], and of \(B_s \rightarrow K \mu \overline{\nu }_\mu\) [2]. These results are measured relative to the CKM favored processes to determine ratios of \(|V_{ub}|\) / \(|V_{cb}|\). Additional determinations using \(B \rightarrow \rho \ell \bar{\nu }_\ell\) and \(B \rightarrow \omega \ell \bar{\nu }_\ell\) and predictions of the form factor normalizations of light-cone sum rules (LCSR) [3] have been performed.

The most precise determination of \(|V_{cb}|\) relies on \(B \rightarrow D^* \ell \bar{\nu }_\ell\) decays. In addition, also \(B_{s} \rightarrow D^*_{s} \ell \bar{\nu }_\ell\) [4] and \(B \rightarrow D \ell \bar{\nu }_\ell\) decays combined with LQCD predictions of the relevant form factors are used to extract \(|V_{cb}|\)Ṫhe remainder of this review will briefly summarize the status of the various determinations of \(|V_{ub}|\) and \(|V_{cb}|\) and ratios. In addition, new global averages for \(B \rightarrow \pi \ell \bar{\nu }_\ell\) and \(B \rightarrow D^* \ell \bar{\nu }_\ell\) are provided, and combined with ratio information to ascertain our current knowledge on these fundamental parameters from exclusive processes. We conclude with a new world-average for \(|V_{cb}|\) and \(|V_{ub}|\) from exclusive decays and compare with inclusive determinations.

Fig. 1
figure 1

(Left) Measured \(B \rightarrow \pi \ell \bar{\nu }_\ell\) partial branching fractions and the corresponding average. (Right) Shared systematic nuisance parameters and corresponding pulls with respect to the pre-fit uncertainty

Full size image

2 Exclusive \(|V_{ub}|\) from \(B \rightarrow \pi \ell \bar{\nu }_\ell\)

There exist several \(B \rightarrow \pi \ell \bar{\nu }_\ell\) measurements from BaBar and Belle. The analyses either rely on the full reconstruction of the second B meson of the \(\Upsilon (4S) \rightarrow B \bar{B}\) production process in hadronic modes [5], or are carried out using an inclusive tagging approach [6,7,8]. The former has the benefit that the signal B meson direction can be inferred from the tag-side B meson properties, what allows for the direct reconstruction of \(q^2\). Furthermore, with the full event reconstructed, backgrounds from other B meson decays and continuum processes can be strongly reduced. The downside of this approach is the rather low efficiency, ranging from 0.3\(-\)0.8% for \(B^\pm\) and 0.2\(-\)0.5% for \(B^0\) [9]. Inclusively tagged analyses rely on the reconstruction of the rest-of-the collision event to build a proxy for the other B decay. This allows for the reconstruction of the approximate neutrino kinematics of the \(B \rightarrow \pi \ell \bar{\nu }_\ell\) decay, which can be exploited to reduce backgrounds and reconstruct \(q^2\). This approach to reconstruct \(q^2\) was used by Ref. [7], whereas Refs. [6, 8] use the ’diamond-frame’ method, first introduced in Ref. [10]. The diamond-frame uses the reconstructed four-momentum of the final state pion and estimates the B meson direction from an average over the cone formed by the \(B-\pi \ell\) angle, which can be estimated from known quantities alone, and the expected angular distribution of the B-meson pairs, which follows a \(\sin ^2 \theta\) distribution, with \(\theta\) denoting the angle between the B production direction and the beam collision axis in the center-of-mass frame. Backgrounds are subtracted using either the invariant mass of the neutrino squared:

$$\begin{aligned} m_{\textrm{miss}}^2 = \left( p_B - p_\pi - p_\ell \right) ^2&\simeq 0 \, \textrm{GeV}^2 \, , \end{aligned}$$
(5)

for hadronically tagged measurements (with \(p_X\) denoting the reconstructed four-momentum of particle X) or the estimated energy difference and invariant mass

$$\begin{aligned} \varDelta E =&E_B - \sqrt{s}/2&\simeq 0 \, \textrm{GeV} \end{aligned}$$
(6)
$$\begin{aligned} m_{bc} =&\sqrt{ s/4 - | \textbf{p}_B|^2 }&\simeq 5.28 \textrm{GeV}, \end{aligned}$$
(7)

with \(\sqrt{s}\) denoting the center of mass energy and \((E_B, \textbf{p}_B)\) is the four-momentum of the signal B meson. The determined signal yields in bins of \(q^2\) are then corrected for detector resolution effects and efficiencies and converted in partial branching fractions \(\varDelta \mathcal {B} / \varDelta q^2\).

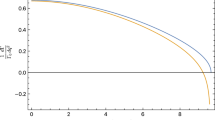
Figure 1 (left) shows the measured spectra of Refs. [5,6,7,8] for \(B^0 \rightarrow \pi ^- \ell ^+ \bar{\nu }_\ell\) and \(B^- \rightarrow \pi ^0 \ell ^- \bar{\nu }_\ell\), where the latter were transformed correcting for the life-time difference of \(B^\pm\) and \(B^0\) and the relevant isospin factors, assuming negligible isospin breaking effects in the semileptonic rates. We average the measured partial branching fractions using a \(\chi ^2\) combination and introducing shared systematic uncertainties between all measurements with nuisance parameters. This has the effect, that, e.g., the uncertainty on the number of B-meson pairs from the BaBar measurements are correctly correlated in the combination. We avoid the d’Agostini bias, described in Ref. [11], by scaling the estimated averages with the nuisance parameters. The initial combination results in a poor p value of 0.3%, with the measurement of Ref. [7] at high \(q^2\) being in tension with the other determinations. This kinematic region is very sensitive to the modelling of other \(b \rightarrow u \ell \bar{\nu }_\ell\) processes, and Ref. [7] makes several assumptions, that no longer reflect the current knowledge. To address this, we introduce an additional scaling parameter, as suggested by Ref. [12], which we allow to enlarge the uncertainties of the offending measurement. The scaling parameter is constrained using a log-normal function with a width parameter of 0.35. The resulting average is shown in Fig. 1 (left, black data points), and the resulting p value is \(40\%\).

We analyze the averaged spectrum to determine \(|V_{ub}|\) using the FLAG average [13] of the LQCD results from FNAL/MILC [14], RBC/UKQCD [15], and JLQCD [16]. The LQCD calculations express their predictions in terms of expansion coefficients \(a^{+/0}\) of the Bourrely, Caprini and Lellouch (BCL) form factor expansion [17]. The two form factors are expanded as

$$\begin{aligned} f_+(q^2)&= \frac{1}{1 - q^2 / m_{B^*}} \sum _{n = 0}^{N-1} a_n^+ \left[ z^n - (-1)^{n-N} - \frac{n}{N} z^{N} \right] \, , \end{aligned}$$
(8)
$$\begin{aligned} f_0(q^2)&= \sum _{n=0}^{N-1} a_n^0 \, z^n \, , \end{aligned}$$
(9)

with

$$\begin{aligned} z = z(q^2, t_0) = \frac{ \sqrt{(}t_+ - q^2) - \sqrt{t_+ - t_0} }{ \sqrt{(}t_+ - q^2) + \sqrt{t_+ - t_0} } \, , \end{aligned}$$
(10)

which maps \(q^2\) onto a disk with \(|z| < 1\). Furthermore

$$\begin{aligned} t_{\pm } = \left( m_B \pm m_\pi \right) ^2\, \quad t_0 = \left( m_B + m_\pi \right) \left( \sqrt{m_B} - \sqrt{m_\pi }\right) ^2 \, . \end{aligned}$$
(11)

Due to the poor compatibility of the average of LQCD information, FLAG applies an additional scaling factor to the uncertainties, which we also adopt. The resulting values for \(|V_{ub}|\) and BCL expansion parameters are

$$\begin{aligned} |V_{ub}|&= \left( 3.75 \pm 0.20 \right) \times 10^{-3} \end{aligned}$$
(12)

and we find

$$\begin{aligned} a_0^+&= 0.424 \pm 0.021 \, , \quad a_1^+ = -0.48 \pm 0.04 \, , \end{aligned}$$
(13)
$$\begin{aligned} a_2^+&= -0.66 \pm 0.17 \, , \quad a_0^0 = 0.561 \pm 0.024 \, , \end{aligned}$$
(14)
$$\begin{aligned} a_1^1&= -1.39 \pm 0.07 \, , \end{aligned}$$
(15)

with correlations of

$$\begin{aligned} C = \left( \begin{matrix} 1. &{} -0.91 &{} 0.16 &{} 0.31&{} -0.37 &{} -0.02 \\ -0.91 &{} 1. &{} -0.35 &{} -0.34&{} 0.41&{} -0.04 \\ 0.16 &{} -0.35 &{} 1. &{} -0.60 &{} -0.09 &{} 0.02 \\ 0.31 &{} -0.34&{} -0.60 &{} 1. &{} -0.20 &{} 0.11 \\ -0.37&{} 0.41 &{} -0.09 &{} -0.20 &{} 1. &{} -0.82 \\ -0.02 &{} -0.04 &{} 0.02 &{} 0.11&{} -0.82 &{} 1. \\ \end{matrix} \right) \, . \end{aligned}$$
(16)

The fit has a p value of 46%.

We note that this result is in good agreement with the determination of Ref. [18], which combines LQCD and LCSR information (Fig. 2).

Fig. 2
figure 2

Result of the BCL fit to the world average, expressed as partial branching fractions \(\varDelta \mathcal {B}/\varDelta q^2\) of \(B^0 \rightarrow \pi ^+ \ell ^- \bar{\nu }_\ell\)

Full size image

3 Exclusive \(|V_{ub}|\) from \(B\rightarrow \rho \ell \bar{\nu }_\ell\) and \(B\rightarrow \omega \ell \bar{\nu }_\ell\)

The Belle and BaBar collaborations have also provided measurements of the \(q^2\) spectrum of \(B\rightarrow \rho \ell \bar{\nu }_\ell\) and \(B\rightarrow \omega \ell \bar{\nu }_\ell\) decays [5, 7, 19]. The employed experimental techniques are very similar to the ones used to study \(B \rightarrow \pi \ell \bar{\ }nu_\ell\). Ref. [20, 21] provides an experimental average of the measured spectra. The form factors describing these decays can be predicted using LCSR calculations [3], which assume the narrow-width approximation for the \(\rho\) and \(\omega\). Going beyond this description, requires describing the full di-pion final state. The relevant \(B\rightarrow \pi \pi\) form factors were studied for the first time using LCSRs in Ref. [22, 23], where the limiting factor on the predictions are the knowledge of the non-perturbative inputs.

Because of the unstable nature of the \(\rho\) and \(\omega\) mesons, LQCD predictions are challenging, and reliable estimates of the form factors have yet to be determined. The RBC/UKQCD collaboration studied the form factors in the quenched approximation, but these predictions suffer from uncontrolled systematic uncertainties [24].

Using the LCSR input [3] and the averages of [20, 21] one finds for \(|V_{ub}|\) from \(B\rightarrow \rho \ell \bar{\nu }_\ell\):

$$\begin{aligned} |V_{ub}|= (2.96 \pm 0.29) \times 10^{-3}\,, \end{aligned}$$
(17)

and from \(B\rightarrow \omega \ell \bar{\nu }_\ell\):

$$\begin{aligned} |V_{ub}|= (2.99 \pm 0.35) \times 10^{-3}\,. \end{aligned}$$
(18)

Respectively, these values are 2.2 and 1.9 \(\sigma\) lower than those obtained from the \(B\rightarrow \pi \ell \bar{\nu }_\ell\) in Eq. (12). The predictions of the form factors from LCSR do describe the experimental \(q^2\) shapes well. A possible explanation could stem from the impact of finite width effects on the prediction of the overall normalization of the form factors or on the measurement. More precise LCSR and future input from LQCD will help to understand why the extracted values of \(|V_{ub}|\) from vector meson final states seem inconsistent with other determinations. Note that the reanalysis of Ref. [18] found a smaller discrepancy. None of the \(B\to \rho \ell \bar{\nu}_\ell\) take into account the \(\rho-\omega\) interference [25].

4 Exclusive \(|V_{ub}|\) from \(\Lambda _b \rightarrow p \mu \bar{\nu }_\mu\) and \(B_s \rightarrow K \mu \bar{\nu }_\mu\)

The LHCb collaboration has measured the ratio of \(|V_{ub}|/|V_{cb}|\) using baryon decays and the \(B_s\) system. For the baryons, the LHCb collaboration measured the rate of \(\Lambda _b^0 \rightarrow p \mu ^- \bar{\nu }_\mu\) relative to the decay rate of \(\Lambda _b^0 \rightarrow \Lambda _c^+ \mu ^- \bar{\nu }_\mu\), both for \(q^2 > 15\) GeV\(^2\) [1]. Using the LQCD prediction for the form factors from [26], this results in a ratio of

$$\begin{aligned} |V_{ub}/V_{cb}|_{q^2> 15\;\textrm{GeV}^2} = 0.080 \pm 0.006 \ , \end{aligned}$$
(19)

which uses an update on the world average of the branching fraction of \(\mathcal {B}(\Lambda _c^+\rightarrow p K^- \pi ^+) = \left( 6.26 \pm 0.29 \right) \times 10^{-2}\) [27], that enters the ratio measurement as an input.

This ratio was also extracted from measurements of the \(B_s \rightarrow K \mu \bar{\nu }_\mu\) decay rate both for \(q^2<7\) GeV\(^2\) and \(q^2>7\) GeV\(^2\) normalized to the decay of the \(B_s^0\rightarrow D_s^-\mu ^+\nu _\mu\) [2]. In these two \(q^2\) regions, they found [2]

$$\begin{aligned}&|V_{ub}/V_{cb}|_{q^2<7 \; \textrm{GeV}^2} = 0.0607 \pm 0.004 \ , \nonumber \\&|V_{ub}/V_{cb}|_{q^2 > 7 \; \textrm{GeV}^2} = 0.0946 \pm 0.008 \ , \end{aligned}$$
(20)

based on the Fermilab/MILC LQCD results [28] in the high-\(q^2\) region and on the light-cone sumrule (LCSR) calculation [29] in the low-\(q^2\) region. For the \(\bar{B}_s\rightarrow D_s\) form factors form the HPQCD [30] results are used. Recently, the required \(\bar{B}_s \rightarrow K\) form factors were studied in the whole \(q^2\) range combining LCSR and LQCD results [31], including HPQCD LQCD [32] and the new RBC/UKQCD [33] results. With those newly extracted form factors, and taking the LHCb measurements for the rates, one finds [31]

$$\begin{aligned} |V_{ub}/V_{cb}|_{q^2<7 \; \textrm{GeV}^2} {}&= 0.0681 \pm 0.0040 \ , \nonumber \\ |V_{ub}/V_{cb}|_{q^2 > 7 \; \textrm{GeV}^2} {}&= 0.0801 \pm 0.0047 \ . \end{aligned}$$
(21)

Assuming no experimental correlation between the different \(q^2\) regions, this updated form factor analysis reduces the tension between the two results to the \(1.9\sigma\) level. By only suing LQCD inputs, [31] one finds compatible values in the low and high-\(q^2\) region, but with a five times larger uncertainty in the low-\(q^2\) region. We note the excellent agreement between the high-\(q^2\) extraction and the determination from the \(\Lambda _b\) decays in Eq. (19). A naive average, assuming no experimental or theory correlations between both high-\(q^2\) ratios results in

$$\begin{aligned} |V_{ub}/V_{cb}|_{\text {high}-q^2} = 0.0801 \pm 0.0037 \, . \end{aligned}$$
(22)

5 Exclusive \(|V_{cb}|\) from \(B \rightarrow D^* \ell \bar{\nu }_\ell\) and \(B \rightarrow D \ell \bar{\nu }_\ell\)

There exist several measurements of \(B \rightarrow D^* \ell \bar{\nu }_\ell\), which focus on determinations of \(|V_{cb}|\) using the Caprini–Lellouch–Neubert (CLN) form factor parameterization [34]. This parameterization combines dispersive bounds with QCD sum rules to reduce the large number of form factor parameters that describe the \(B \rightarrow D^*\) transition. Although this parameterization proved to be very useful in the past, it has several drawbacks: it incorporates a number of numerical coefficients, whose values possess additional theoretical uncertainties, which (usually) are neglected. Furthermore, it imposes an artificial constraint on the form factor slope and curvature. Thus it has been suggested to not use this parameterization without incorporating adequate the theoretical uncertainties (see [35] for a discussion).

Ref. [21] provides a world average of measurements, which extract \(|V_{cb}|\) using the CLN form factor parameterization in combination with the LQCD normalizations at zero-recoil of Refs. [36, 37] as averaged by Ref. [38]. They report:

$$\begin{aligned} |V_{cb}|_{\textrm{CLN}} = \left( 38.46 \pm 0.68 \right) \times 10^{-3} \, . \end{aligned}$$
(23)

The measurement entering this world average do not report partial branching fractions of the recoil-parameter \(w = v \cdot v'\) with \(v^{(')}\) denoting the four-velocity of the B-meson (\(D^*\)-meson). The recoil-parameter is related to \(q^2\) via

$$\begin{aligned} w = \frac{m_B^2 + m_{D^*}^2 - q^2}{ 2 m_B m_{D^*}} \, . \end{aligned}$$
(24)

The first partial branching fraction measurement as a function of w and the 3 decay angles (\(\cos \theta _\ell , \cos \theta _V, \chi\)) was carried out by Belle using \(B^0 \rightarrow D^{*\,+} \ell ^- \bar{\nu }_\ell\) in Ref. [39] and using hadronically tagged events. This preliminary conference result is superseded by the published result of Ref. [40], which also included \(B^+\) decays. Additional measurements were carried out using inclusive tagging by Belle in Ref. [41] and Belle II in Ref. [42]. BaBar also analyzed this decay channel in Ref. [43], but no unfolded partial branching fraction measurements are available.

Fig. 3
figure 3

Measured partial branching fractions as a function of the recoil-parameter w and the 3 decay angles (\(\cos \theta _\ell\), \(\cos \theta _V\), \(\chi\)) for \(B \rightarrow D^* \ell \bar{\nu }_\ell\) from Refs. [40, 42] are shown. The black data points shows our average. For more details, see text

Full size image

Hadronically tagged measurements of this channel have high purity and can subtract remaining backgrounds using \(m_{\textrm{miss}}^2\). Inclusively tagged measurements tend to focus on \(B^0 \rightarrow D^{*\,+} \ell ^- \bar{\nu }_\ell\), and use the cosine of the \(B-D^*\ell\) angle and \(\varDelta M = M_{D^*} - M_D\) to separate signal from background contributions. The cosine of the \(B-D^*\ell\) angle is given by

$$\begin{aligned} \cos \theta _{B-D^*\ell }&= \frac{2 E_B E_{D^*\ell } - m_B^2 - m_{D^*\ell }^2}{ 2 |\textbf{p}_B| | \textbf{p}_{D^*}| } \end{aligned}$$
(25)

with \((E_X, \textbf{p}_X)\) and \(m_X\) the momentum and mass of particle X. For correctly reconstructed signal decays \(\cos \theta _{B-D^*\ell }\) should be within the geometric range of \([-1,1]\), with reconstruction effects and final-state-radiation photons resulting in a smearing and negative tail. One of the key uncertainties, that affects the precision of \(|V_{cb}|\)  is the knowledge of the efficiency of the slow pion from the \(D^* \rightarrow D \pi\) transition and the knowledge of the shape of the background shapes.

Figure. 3 shows the measured and unfolded 1D projections of (w, \(\cos \theta _\ell\), \(\cos \theta _V\), \(\chi\)) of Refs. [40, 42]. We combine the shape information of the two measurement, assuming no correlations between the individual determinations. This neglects small systematic correlations between the Belle and Belle II results. The combined spectrum has a p value of 15%, indicating agreement between the different shapes. We do not include the Belle result into the average, as no unfolded shapes are provided and special care has to be taken to correctly use the available information. cf. Ref. [44]. In addition, some deviations between the electron and muon final states were reported in Ref. [45].

To determine \(|V_{cb}|\) we combine the available lattice information from the FNAL/MILC, HPQCD, and JLQCD [46,47,48] collaborations. We first discuss the compatibility of this lattice input only, as there exists no FLAG combination of the available information as of now. We transform all available information into the heavy quark basis (\(h_{A_{1-3}}, h_V\)) and express the lattice constraints in terms of \(h_{A_1}\) and the form factor ratios \(R_{0-2}\) defined as

$$\begin{aligned} R_0&= \frac{ h_{A_1} (w+1) - h_{A_3} (w-r_*) - h_{A_2}(1 - w \, r_*) }{ (1 + r_*) h_{A_1}} \, , \end{aligned}$$
(26)
$$\begin{aligned} R_1&= \frac{h_V}{h_{A_1}} \, , \quad R_2 = \frac{h_{A_3} + r_* \, h_{A_2}}{h_{A_1}} \, , \end{aligned}$$
(27)

Figure 4 shows the result of a combined fit of the LQCD information using the Boyd–Grinstein–Lebed (BGL) parameterization [49, 50]. This parameterization makes use of dispersive bounds and applies a conformal transformation to approximate the form factors as a series expansion. The conformal transformation maximizes the descriptive power of the LQCD information or measured shapes by ensuring a fast convergence of the expansion. Following Refs. [51, 52] we introduce the conformal variable

$$\begin{aligned} z = \frac{ \sqrt{w+1} - \sqrt{2 } }{ \sqrt{w+1} + \sqrt{2} } \, , \end{aligned}$$
(28)

and parameterize the form factors in terms of \(\{ a_n, b_n, c_n \}\) expansion coefficients

$$\begin{aligned} g(z)&= \frac{1}{ P_g(z) \, \phi _g(z)} \, \sum _{n=0}^{n_a-1} \, a_n \, z^n \, , \end{aligned}$$
(29)
$$\begin{aligned} f(z)&= \frac{1}{ P_f(z) \, \phi _f(z)} \, \sum _{n=0}^{n_b-1} \, b_n \, z^n \, , \end{aligned}$$
(30)
$$\begin{aligned} F_1(z)&= \frac{1}{ P_{F_1}(z) \, \phi _{F_1}(z)} \, \sum _{n=0}^{n_c-1} \, c_n \, z^n \, , \end{aligned}$$
(31)
$$\begin{aligned} F_2(z)&= \frac{1}{ P_{F_2}(z) \, \phi _{F_2}(z)} \, \sum _{n=0}^{n_d-1} \, d_n \, z^n \, . \end{aligned}$$
(32)

Here \(n_{a/b/c/d}\) denotes the truncation order of the expansion. Note that \(c_0\) and \(b_0\) are not independent, but are related via

$$\begin{aligned} c_0 = \left( \frac{ (m_B - m_{D^*} ) \, \phi _{F_1}(0) }{ \phi _f(0)} \right) b_0 \, . \end{aligned}$$
(33)

Furthermore, \(P_j(z)\) \((j=g, f, F_1, F_2)\) are Blaschke factors, which remove poles for the region \(q^2/c^2 < (m_B^2 + m_{D^*}^2)\), and \(\phi _j(z)\) are the outer functions [51]. A fit using \(\{n_a, n_b, n_c, n_d \} = 3323\) results in a p value of 49%, indicating fair agreement between all LQCD results. A visual inspection hints some tensions in \(R_0\) and \(R_2\), but we note that the correlation between the LQCD predictions of a given group are strongly correlated.

Fig. 4
figure 4

LQCD predictions for \(h_{A_1}\) and \(R_{0-2}\) are shown. A combined BGL fit results in a p value of 77%, indicating acceptable agreement. Visually the largest disagreements are present in \(R_0\) and \(R_2\), but note that the corresponding points from HPQCD exhibit strong positive correlations

Full size image

With this informatoin, we analyze the averaged differential shapes in combination with the isospin average of the \(B \rightarrow D^* \ell \bar{\nu }_\ell\) branching fractions from \(B^0 / \overline{B}^0\) and \(B^\pm\) from [21] to determine \(|V_{cb}|\) and the relevant form factors. We study three scenarios:

  • A fit to the full available LQCD information from all three groups, expressed as constraints on \(h_{A_1}, R_{0-2}\);

  • To only the LQCD information of \(h_{A_1}\) beyond zero recoil;

  • And to only the LQCD information of \(h_{A_1}\) at zero recoil.

The corresponding fits are shown in Fig. 3. The fit, which is using the full set of LQCD information, has a p value of 22.4%. We determine the number of coefficients to describe the form factors using a nested-hypothesis test, as outlined by Ref. [53] to avoid overfitting the spectrum (see also Ref. [54]). We find \(\{n_a, n_b, n_c\} = 332\) and a value of

$$\begin{aligned} |V_{cb}|_{\textrm{BGL}} = \left( 40.48 \pm 0.63 \right) \times 10^{-3} \, . \end{aligned}$$
(34)

The fits using less LQCD information determine very similar values, \(\left( 40.59 \pm 0.58 \right) \times 10^{-3}\) and \(\left( 40.66 \pm 0.54 \right) \times 10^{-3}\), with p values of 17.8% and 7.3%, respectively, when using only \(h_{A_1}\) with beyond or zero-recoil information. The number of coefficients required to describe the available data is \(\{n_a, n_b, n_c\} = 210\) in both cases. All obtained values are notably higher than Eq. 23 and the smaller uncertainties for the scenarios using less lattice information in contrast to the full information, stems from the smaller number of fitted parameters selected by the nested hypothesis test to describe the available LQCD and experimental information.

Measurements of \(B \rightarrow D \ell \bar{\nu }_\ell\) are carried out using very similar techniques and via using the same discriminating observables. One major background is the so-called down-feed from \(B \rightarrow D^* \ell \bar{\nu }_\ell\) decays, which can dominate the very relevant phase-space near zero recoil (\(w \sim 1\)). Here the \(B \rightarrow D \ell \bar{\nu }_\ell\) decay rate vanishes due to angular momentum conservation. The contamination of \(B \rightarrow D^* \ell \bar{\nu }_\ell\) is reduced using either explicit vetos (based on finding suitable slow pions, which indicate the presence of a \(D^* \rightarrow D \pi\) transition) or with multivariate approaches. There exist many measurements, which report the CLN form factor parameters. Ref. [21] provides a world average of

$$\begin{aligned} |V_{cb}|_{\textrm{CLN}, B \rightarrow D} = \left( 39.14 \pm 0.99 \right) \times 10^{-3} \, , \end{aligned}$$
(35)

using the LQCD input of [55]. Two measurements provide information about the w distribution: [56] reports a differential spectrum, which is however the result of a CLN fit. It is thus not advisable to use the spectrum, as a correct treatment of the uncertainties cannot be carried out. In Ref. [57], fully unfolded distributions of w for electron and muon final states as well as for charged and neutral B decays were reported for the first time. Ref. [58] analyzed both sets of experimental information and find using the BGL parameterization

$$\begin{aligned} |V_{cb}|_{\textrm{BGL}, B\rightarrow D} = (40.49\pm 0.97)\cdot 10^{-3} \ \end{aligned}$$
(36)

using LQCD beyond zero recoil information from HPQCD [59] and FNAL/MILC [55], and compatible with Eq. 34.

6 Exclusive \(|V_{cb}|\) from \(B_s \rightarrow D_s^{(*)} \ell \bar{\nu }_\ell\)

The LHCb collaboration also extracted the first \(|V_{cb}|\) determination from \(B_s\) decays. Implementing a novel analysis method, the decays to \(B_s^0\rightarrow D_s^{(*)} \ell \bar{\nu }_\ell\) can be identified from the inclusive sample of \(D_s^- \mu ^+\) candidates [4]. For the form factors, the LQCD calculations of Ref. [30] are used by converting their BCL parameterization to the CLN and BGL parametrization. LHCb then reports

$$\begin{aligned} |V_{cb}|_{\textrm{CLN}} =&(41.4 \pm 1.6)\times 10^{-3} \ , \nonumber \\ |V_{cb}|_{\textrm{BGL}} =&(42.3 \pm 1.7)\times 10^{-3} \ . \end{aligned}$$
(37)

The two results are compatible when accounting for their experimental correlation (Fig. 5).

Fig. 5
figure 5

Current status of \(|V_{ub}|\) and \(|V_{cb}|\) from inclusive (black marker) and exclusive determinations (red ellipse, colored bands) is shown. The red ellipse shows the statistical average of the ratio measurements and the exclusive determinations. All contours correspond to \(\varDelta \chi ^2 = 1\) CL. The dashed line shows the 68% CL for the exclusive world average of \(|V_{ub}|\) and \(|V_{cb}|\). The tension of the world averages of inclusive and exclusive values is at the 2.85 \(\sigma\) level

Full size image

7 Summary

Determinations of \(|V_{cb}|\) with exclusive decays have reached \(\%\) level precision, due to recent progress in LQCD and experimental determinations. Furthermore, more generalized parameterizations of the form factors describing the non-perturbative transition matrix elements, result in an upward shift of its central value, which are more compatible with determinations using inclusive decays. Averaging the determined values of \(|V_{cb}|\) using the BGL parameterization from \(B \rightarrow D \ell \bar{\nu }_\ell\), \(B \rightarrow D^* \ell \bar{\nu }_\ell\) and \(B_s \rightarrow D_s^{(*)} \ell \bar{\nu }_\ell\) results in a value of

$$\begin{aligned} |V_{cb}|_{\textrm{excl}}&= \left( 40.64 \pm 0.50 \right) \times 10^{-3} \, . \end{aligned}$$
(38)

and the individual values show a compatibility with a p value of 59%. Comparing this with the inclusive average (cf. chapter inclusive \(|V_{ub}|\) and \(|V_{cb}|\) in this review) of

$$\begin{aligned} |V_{cb}|_{\textrm{incl}}&= \left( 42.00 \pm 0.47 \right) \times 10^{-3} \, , \end{aligned}$$
(39)

we see that both determinations exhibit a tension of 2 standard deviations.

The determination of \(|V_{ub}|\) from exclusive \(B \rightarrow \pi \ell \bar{\nu }_\ell\) decays has reached a precision of \(5\%\). Determinations using \(B \rightarrow \rho \ell \bar{\nu }_\ell\) and \(B \rightarrow \omega \ell \bar{\nu }_\ell\) result in lower values of \(|V_{ub}|\)  but have to rely on LCSR calculations only for the extraction and in case of the \(\rho\) have to rely on the narrow-width approximation. We thus use only \(B \rightarrow \pi \ell \bar{\nu }_\ell\) decays as a reference point and obtain for exclusive \(|V_{ub}|\)

$$\begin{aligned} |V_{ub}|_{\textrm{excl}} = \left( 3.75 \pm 0.20 \right) \times 10^{-3} \, . \end{aligned}$$
(40)

This value can be compared to measurements using inclusive semileptonic decays. Determining an arithmetic average of the world average of Ref. [21], which combines measurements of partial branching fractions from different phase-space regions and different calculations for the corresponding partial decay rates, results in:

$$\begin{aligned} |V_{ub}|_{\textrm{incl}}&= \left( 4.19 \pm 0.22 \right) \times 10^{-3} \, , \end{aligned}$$
(41)

where we added the uncertainties in quadrature. This value exhibits a tension of 1.5 standard deviations with respect to Eq. (12). Combing the exclusive values with extractions of the ratio \(|V_{ub}|\ / |V_{cb}|\) ratio information in Sect. 4 at high-\(q^2\), we quote as the new world-average

$$\begin{aligned} |V_{ub}|_{\textrm{excl, WA}}&= \left( 3.44 \pm 0.12 \right) \times 10^{-3} \, , \end{aligned}$$
(42)
$$\begin{aligned} |V_{cb}|_{\textrm{excl, WA}}&= \left( 40.81 \pm 0.50 \right) \times 10^{-3} \, , \end{aligned}$$
(43)

with a correlation of \(\rho = 0.22\). The average has a p value of 5%. The combined compatibility between the inclusive and exclusive \(|V_{ub}|\) and \(|V_{cb}|\) values is at the 2.9 \(\sigma\) level. The individual tensions are 2.9 \(\sigma\) and 1.7 \(\sigma\) for \(|V_{ub}|\) and \(|V_{cb}|\)  respectively. This indicates a systematic incompatibility between both approaches to determine the same fundamental SM parameters, whose origin remains unknown. Explanations involving new physics are challenging [60,61,62,63,64]. Loop-level determinations [65] and CKM unitarity [66, 67] favor the exclusive value of \(|V_{ub}|\) and the inclusive value of \(|V_{cb}|\).