1 Introduction

Nowadays, there are many objects that can be considered candidates for black holes, but we do not yet have direct observations proving this fact. The closest look at the surroundings of these objects so far has been brought by the Event Horizon Telescope (EHT), which has already presented images of two supermassive objects located in the centers of galaxies M87 and our Milky Way [1, 2]. Black holes are prime candidates not only for these objects, but the theory of black holes suffers from one major flaw - in the center of such objects there must be a singularity. However, the existence of singularities, even if hidden under the veil of the event horizon, does not give sleep to the majority of astrophysicists dealing with this issue.

An elegant solution to avoid the central singularity was proposed by Simpson and Visser [3]. This spherically symmetric meta-geometry (there is also a generalized rotating version [4]) describes a regular black hole or a traversable wormhole, depending on the value of the regularization length-scale parameter l. This parameter could represent the effect of quantum gravity or some still unknown effects. In the case of a wormhole, a tunnel connecting two locations in the universe (or different universes) is of the same nature as a standard Morris-Thorne wormhole. It should be recalled that a traversable wormhole created in this way usually needs a special exotic matter with a negative energy density and tension in its central region, which violates the weak-energy condition. However, at present, wormholes were introduced without the need for such exotic matter, for example, the Einstein-Dirac-Maxwell wormhole [5,6,7].

No radiation can come from the central regions of black holes [8], but we can observe radiation coming through the central regions of wormholes [9]. Differences between the GR black holes and regular black holes related to non-linear electrodynamics could be reflected by optical phenomena in the vicinity of the black hole horizon [10]. When studying them, we have to rely on the radiation coming from their close surroundings, and accretion disks. There is a special effect related to accretion discs that enable the study of differences between standard BHs, regular BHs, and WHs. In the energy spectra of most accreting sources, we observe the so-called quasiperiodic oscillations (QPOs), which can be used to study the central object. QPOs are often observed in pairs (upper and lower frequencies). The ratios of these frequencies in most (assumed) black hole sources occur in an integer ratio, mainly in the ratio of 3:2. These oscillations can bring us information about the central objects of these systems and thus tell us something about their origin. The theories of QPOs indicate the connection of these phenomena with the periodic movements of matter of the accretion disk relative to the stable circular orbits near the central object [11,12,13,14,15,16,17,18,19,20].

In the theoretical description of black hole candidates, we must not forget the observations that indicate the presence of magnetic fields. Because of their intensity, these fields have a negligible effect on the curvature of spacetime. They do, however, influence the trajectories of charged particles in a highly significant way [21,22,23,24,25,26,27].

In this paper, we investigate the epicyclic motion and its application to the observed data of QPOs in the vicinity of a Simpson–Visser regular black hole or wormhole immersed into a uniform magnetic field, and thus extend the research [28]. We will first introduce the Simpson-Visser metric and the uniform magnetic field, then we will deal with circular motions. Then epicyclic frequencies of epicyclic oscillations around these circular orbits will be calculated. When fitting the HF QPOs, we focus on the model of epicyclic resonance (ER) and relativistic precession (RP) [29]. We will show how the metric length parameter l and the magnetic field affect the aforementioned theoretical models of QPOs and investigate whether the Simpson–Visser regular black hole or wormhole can effectively describe the effects observed in real objects in the Universe. Specifically, we will focus on HF QPOs observed in the vicinity of three microquasars and several supermassive black hole candidates.

Throughout the paper, we use space-like signature \((-,+,+,+)\), and a geometric system of units in which \(G = c = 1\); we restore them when we need to compare our results with observational data. Greek indices run from 0–3, and Latin indices are as 1–3.

2 Simpson–Visser regular black holes and wormholes

The Simpson–Visser (SV) meta-geometry enables a unified description of regular black holes and wormholes by smooth interpolation between these two possibilities using a length-scale parameter l. For more details and properties see [3, 28].

The spacetime of the SV meta-geometry is described in the spherical coordinates \(x^{\alpha }=\{t,r,\theta ,\phi \}\) by the line element

$$\begin{aligned} ds^2=-f(r)dt^2 + \frac{1}{f(r)}dr^2+(r^2+l^2) d\Omega ^2, \end{aligned}$$
(1)

where the lapse function \(f(r)=1-2M/\sqrt{r^2+l^2}\) and \(d\Omega ^2=d\theta ^2+\sin ^2\theta d\phi ^2\). The spacetime turns to the Schwarzschild black hole one when \(l=0\).

2.1 Scalar invariants

The scalar invariants of the spacetime give crucial information on the character of the gravitational field. Ricci scalar, so-called scalar curvature, is one of the simplest curvature invariants of curved spacetime and is defined as \(R=g^{\mu \nu }R_{\mu \nu }\), where \(R_{\mu \nu }\) is the Ricci tensor. The positive and the negative values of the Ricci scalar correspond to the sunken and convex forms of spacetime, respectively.

$$\begin{aligned} R=\frac{2 l^2 \left( \sqrt{l^2+r^2}-3 M\right) }{\left( l^2+r^2\right) ^{5/2}}. \end{aligned}$$
(2)

The tensor is finite at the center of the BH when \(r\rightarrow 0\)

$$\begin{aligned} R=-\frac{2 \left( l-3 M \right) }{l^3}, \end{aligned}$$
(3)

however, it is finite only for non-zero l.

At the same time, it is responsible for the square of the energy-momentum tensor of the field in the spacetime around the black hole which can be defined as

$$\begin{aligned} {{\mathcal {R}}}=R_{\mu \nu }R^{\mu \nu }\equiv 1/(8\pi G)T_{\mu \nu }T^{\mu \nu }. \end{aligned}$$

The square of Ricci tensor for the spacetime around the Simpson–Visser black holes takes the form:

$$\begin{aligned} {{\mathcal {R}}}=\frac{2 l^4 \left( -6 M \sqrt{l^2+r^2}+2 l^2+2 r^2 + 9M^2\right) }{\left( l^2+r^2\right) ^5}, \end{aligned}$$
(4)

and the Ricci tensor’s limit at the center,

$$\begin{aligned} \lim _{r\rightarrow 0} {{\mathcal {R}}}=\frac{2 \left( 2 l^2-6 l M+9 M^2\right) }{l^6}. \end{aligned}$$
(5)

The \({{\mathcal {R}}}\) is finite only when l is non-zero. It is seen from Eq. (5) that the spacetime is not asymptotically Ricci flat.

Now we consider the Kretschmann scalar defined as \({{\mathcal {K}}}=R_{\mu \nu \sigma \rho }R^{\mu \nu \sigma \rho }\). Usually, the square root of the Kretschmann scalar can be interpreted as an effective gravitational energy density \(\sqrt{{{\mathcal {K}}}} \sim \rho _M\). There is

$$\begin{aligned} {{\mathcal {K}}}= & {} \frac{4}{\left( l^2+r^2\right) ^5} \Big [3 l^6+4 M^2l^2 r^2 \left( 2 \sqrt{l^2+r^2}-3 \right) \nonumber \\{} & {} +l^4 \left( -8 \sqrt{l^2+r^2}+3 r^2 + 9M^{''}\right) +12 M r^4\Big ], \end{aligned}$$
(6)

The Kretchmann scalar has the following limit at the center,

$$\begin{aligned} \lim _{r\rightarrow 0} {{\mathcal {K}}}= \frac{4 \left[ 3 l^2+M (9 M-8 l)\right] }{l^6}, \end{aligned}$$
(7)

which is finite when l is non-zero.

In the rest of our paper, we use non-dimensional units where \(r/M\rightarrow r\) and \(l/M\rightarrow l\).

Fig. 1
figure 1

Radial profiles of Ricci scalar (top panel), the square of Ricci tensor (middle panel), and Kretchmann scalar (bottom panel) for different values of l

Full size image

Figure 1 represents radial dependence of the scalar invariants such as the Ricci scalar (the left panel), the square of the Ricci tensor (in the middle panel), and the Kretchmann scalar (the right panel) for different values of the length parameter l. It is shown that the scalar invariants decrease with the increase of l. The Ricci scalar rapidly decreases near the horizon. However, the square of the Ricci tensor and Kretchmann scalars rapidly fall and is close to zero out of the horizon but inside the ISCO.

3 Magnetization of Simpson–Visser spacetime

This work assumes that the SV BH or WH is immersed in a uniform external magnetic field. In fact, obtaining analytical solutions to Maxwell’s equations for magnetic fields around black holes is more complicated. Wald has performed a simple approach for flat spherically symmetric Ricci spacetime using an analog uniform electromagnetic field 4-vector \(A_\phi \) and an axial Killing vector \(\psi _\alpha \). In order to find the Maxwell equation in curved spacetime one has to use \(\frac{1}{\sqrt{-g}}\partial _\alpha (\sqrt{-g}F^{\alpha \beta })=0\), where \(F_{\alpha \beta }=\partial _\alpha A_\beta -\partial _\beta A_\alpha \); we search the solution in the form,

$$\begin{aligned} A_\phi =\frac{1}{2}Br^2\psi (r)\sin ^2\theta , \end{aligned}$$
(8)

where \(\psi (r)\) is an arbitrary radial (correction) function corresponding to the values of l and for the Schwarzschild limit \(l=0\) the function takes \(\psi (r)=1\). One may get an ordinary differential equation for \(\psi (r)\) in the meta geometry with non-zero l by inserting Eq. (8) into Maxwell’s equation

$$\begin{aligned}{} & {} r \Big \{r \left( l^2+r^2\right) \left( \sqrt{l^2+r^2}-2 \right) \psi ''(r)\nonumber \\{} & {} \quad +\Big [4 l^2 \left( \sqrt{l^2+r^2}-2\right) \nonumber \\{} & {} \quad +2 r^2 \left( 2 \sqrt{l^2+r^2}-3\right) \Big ] \psi '(r)\Big \} \nonumber \\{} & {} \quad +2 l^2 \psi (r) \left( \sqrt{l^2+r^2}-2\right) =0. \end{aligned}$$
(9)

Equation (9) is a complicated second-order differential equation to solve analytically. Therefore, we solve it numerically and analyze the \(\psi (r)\) function for the various values of l.

Fig. 2
figure 2

Radial dependence of the numerical solution of Eq. (9), \(\psi _{num}(r)\) for different values of the parameter l

Full size image

Figure 2 presents radial profiles of the numerical solution of Eq. (9) for various values of l. It is observed from the figure that the \(\psi \) function decreases as l increases and differs from 1 near the horizon. However, in far distances, the effect of l vanishes and the function reaches 1. Here, the black line corresponds to the Schwarzschild case (\(l=0\)), and it does not depend on the radial coordinate, so it is equal to one.

According to Wald’s approach [22, 30, 31], the non-zero component of the 4-vector potential component takes the form

$$\begin{aligned} A_\phi= & {} \frac{B_0}{2}g_{\phi \phi }\sin ^2\theta \nonumber \\= & {} \frac{B_0}{2}(r^2+l^2)\sin ^2\theta , \end{aligned}$$
(10)

where \(B_0\) is the asymptotic intensity of the external magnetic field.

In order to show the Walds approach is valid for the SV metric, we look at the difference between the solution (8) and the Walds approach (10) as

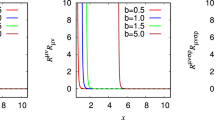
$$\begin{aligned} \Delta A_{\phi }= & {} \frac{B}{2}\left[ (r^2+l^2)-r^2\psi (r) \right] \sin ^2\theta \nonumber \\= & {} \frac{B}{2}(r^2+l^2)\left[ 1-\frac{r^2\psi (r)}{r^2+l^2} \right] \sin ^2\theta . \end{aligned}$$
(11)

Obviously when \(\Delta A_{\phi } \rightarrow 0\), one can use Eq. (10) as a solution of the Maxwell equation in our further calculations. As we mentioned above, it is not possible to solve Eq. (9) analytically. So, one can analyse the difference \((r^2+l^2)-r^2\psi _{num}(r)\), numerically, using the numerical solution of \(\psi _{num}(r)\). Thus, the term \(1-\psi _{num}(r)r^2/(r^2+l^2)\) has to be small enough to neglect the difference between the numerical solution and Wald’s approach.

Fig. 3
figure 3

Radial dependence of the difference between approximate solution (8) and Wald’s approach (10) of correction function for different values of the length parameter l

Full size image

In Fig. 3 we show the radial dependence of the difference using the correction function \(\psi \) obtained as a numerical solution of Eq. (3) for different values of l. However, in the region around \(r>4M\) (where QPOs can be generated) the difference \(1-\psi _{num}(r)r^2/(r^2+l^2)\) consists of less than 2% even for the case of \(l=20\). On the other hand, in this region, the value of the Ricci scalar is very close to zero. In other words, the spacetime is Ricci flat at the region near 3M. Consequently, there is no doubt in using Wald’s approach for the electromagnetic four-potentials for the fields around an SV black hole/wormhole for the circular stable orbits where epicyclic motion around the orbits takes place.

One may immediately find the non-zero components of the electromagnetic tensor using the definition \(F_{\mu \nu }=A_{\nu ,\mu }-A_{\mu ,\nu }\) for each configuration of the external magnetic field in the following form,

$$\begin{aligned} F_{r \phi }= & {} B_0 r \sin ^2\theta \, \end{aligned}$$
(12)
$$\begin{aligned} F_{\theta \phi }= & {} B_0 (r^2+l^2)\sin \theta \cos \theta . \end{aligned}$$
(13)

The component of the magnetic field around the black hole measured by a local observer read

$$\begin{aligned} B^{{\hat{\alpha }}}= & {} \frac{1}{2}{\sqrt{g_{\alpha \alpha }}} \eta ^{\alpha \beta \sigma \mu } F_{\beta \sigma } w_{\mu }\, \end{aligned}$$
(14)

where \(w_{\mu }\) is four-velocity of the local static observer, \(\eta _{\alpha \beta \sigma \gamma }\) is the pseudo-tensorial form of the Levi–Civita symbol \(\epsilon _{\alpha \beta \sigma \gamma }\) with the relations

$$\begin{aligned} \eta _{\alpha \beta \sigma \gamma }=\sqrt{-g}\epsilon _{\alpha \beta \sigma \gamma }\, \quad \eta ^{\alpha \beta \sigma \gamma }=-\frac{1}{\sqrt{-g}}\epsilon ^{\alpha \beta \sigma \gamma }\, \end{aligned}$$
(15)

and \(g=\mathrm{det|g_{\mu \nu }|}=-(r^2+l^2)^2\sin ^2\theta \) for spacetime metric (1); \(\epsilon _{0123}=1\) with even permutations and -1 for odd ones, and it is zero for other combinations. The non-zero components take the form

$$\begin{aligned}{} & {} B^{{\hat{r}}}=B_0\cos \theta \, \end{aligned}$$
(16)
$$\begin{aligned}{} & {} B^{{\hat{\theta }}}=B_0\sqrt{\frac{r^2f(r)}{r^2+l^2}}\sin \theta . \end{aligned}$$
(17)
Fig. 4
figure 4

Normalized angular component of the external magnetic field as a function of r for different values of the length parameter l

Full size image

The radial profile of normalized the angular component of the magnetic field to the asymptotic magnetic field value \(B^{{\hat{\theta }}}/B_0\) is illustrated in Fig. 4 for various values of the parameter l. It is observed from the figure that the magnetic field component increases with the radial coordinate. However, the component decreased due to an increase in l. It is also seen from the figure that the component equals zero on the horizon of the black hole with \(l=0,1\), while for the wormholes with \(l>2\), the magnetic field is zero at the center of the object \(r=0\).

4 Electrically charged particle’s motion and its circular orbits

Here, we investigate the dynamics of electrically charged particles around Simpson–Visser regular black holes and wormholes immersed in the uniform magnetic field with a focus on the equatorial orbits and related epicyclic oscillations. The action of electrically charged particles at the equatorial plane (where \(\theta =\pi /2\) and \({\dot{\theta }}=0\)) can be expressed in the form

$$\begin{aligned} {{\mathcal {S}}}=-Et+L\phi +{{\mathcal {S}}}_r\, \end{aligned}$$
(18)

which allows the separation of variables in the Hamilton–Jacobi equation. The equation of radial motion of charged particles can be found as

$$\begin{aligned} {\dot{r}}^2={{\mathcal {E}}}^2-V_{\textrm{eff}}(r)\, \end{aligned}$$
(19)

where \({{\mathcal {E}}}=E/m\) is the specific energy of the particles and the effective potential for the radial motion of charged particles has the form:

$$\begin{aligned} V_{\textrm{eff}}(r,\theta )=f(r)\left[ 1+\frac{\left( {{\mathcal {L}}}-B (r^2+l^2)\sin ^2\theta \right) ^2}{(r^2+l^2)\sin ^2\theta }\right] \,, \end{aligned}$$
(20)

where \({{\mathcal {L}}}=L/m\) is specific angular momentum of the particle. The effective potentials are presented in Fig. 5, where we variate length parameter l, magnetic field parameter B, and angular momentum \({\mathcal {L}}\).

Fig. 5
figure 5

Effective potentials of charged test particles for characteristic values of the length parameter l, and the parameter B

Full size image

Circular orbits are performed when \(d V_{\textrm{eff}}/dr=0\). Then one may obtain a relation for the angular momentum of a charged particle at a circular orbit at the equatorial plane (\(\theta =\pi /2\))

$$\begin{aligned} {{\mathcal {L}}}= & {} \frac{1}{3-\sqrt{l^2+r^2}} \Bigg \{B(l^2+r^2 -\Big [\left( l^2+r^2\right) \nonumber \\ {}{} & {} \times \Big (B^2 \left( l^2+r^2\right) \left( -4 \sqrt{l^2+r^2}+l^2+r^2+4\right) \nonumber \\{} & {} +\sqrt{l^2+r^2}-3\Big )\Big ]^{\frac{1}{2}}\Bigg \}. \end{aligned}$$
(21)
Fig. 6
figure 6

The radial profiles of the specific angular momentum \({\mathcal {L}}_\textrm{c}\) of a charged test particle at circular orbit for characteristic values of parameters l and B

Full size image

The angular momentum of a charged particle at a circular orbit for various magnetic field parameters B and length parameter l is plotted in Fig. 6. Assume that ISCO is located at the orbit where the angular momentum takes the minimum. Notice that for \(l=6\), there is no minimum angular momentum function, i.e., no ISCO as is known. It is observed from the figure that the angular momentum increases with increasing l outside ISCO, while the l parameter decreases the angular momentum inside ISCO. The presence of a positive magnetic interaction parameter causes a decrease in the critical angular momentum, while the negative value of the parameter causes an increase in it.

The specific energy of a charged particle corresponding to a circular orbit at the equatorial plane (\(\theta =\pi /2\)) can be found by inserting the critic value of the angular momentum (21) into the effective potential, and we express it as

$$\begin{aligned} {{\mathcal {E}}}^2= & {} \frac{\left( \sqrt{l^2+r^2}-2\right) }{\left( l^2+r^2\right) ^{3/2}} \Big (B^2 l^4+2 B^2 l^2 r^2+B^2 r^4\nonumber \\{} & {} -2 B l^2 {{\mathcal {L}}}-2 B {{\mathcal {L}}} r^2+l^2+{{\mathcal {L}}}^2+r^2\Big ). \end{aligned}$$
(22)
Fig. 7
figure 7

The figure with Fig. 6, but for the specific energy \({\mathcal {E}}_\textrm{c}\) analyses

Full size image

The specific energy of a charged particle at a circular orbit for various magnetic field parameters B and length parameter l is plotted in Fig. 7. Similarly, the effects of the length parameters on the energy cause a slight increase in the energy out of the orbits where the energy takes minimum, while inside the orbit an increase of l increases the energy, which have been observed in Fig. 6.

Now, here we investigate the innermost stable circular orbits of charged particles around Simpson–Visser black holes and wormholes. The very crucial role for our analysis is the ISCO. It comes from a simultaneous solution of equations \(d V_{\textrm{eff}}/d r=0\) and \(d^2V_{\textrm{eff}}/dr^2=0\).

Fig. 8
figure 8

ISCO position dependent on parameter l (left panel) and B (right panel) for characteristic values of the parameter B and l, respectively

Full size image

There exists a limiting value of l which split cases where exists and doesn’t exist ISCO. When there is no magnetic field this limit is \(l=6\) [28], however in the presence of the magnetic field the situation is more complex and the limiting l is dependent on the magnetic field. The influence can be read from Fig. 8.

5 Harmonic oscillations

In the case of a slight deviation of a test charged particle from the equilibrium position located in a minimum of the effective potential \(V_{\textrm{eff}}(r,\theta )\) at \(r_0\) and \(\theta _0=\pi /2\), corresponding to a stable circular orbit, the particle will start to oscillate around the minimum performing an epicyclic motion governed by linear harmonic oscillations. For harmonic oscillations around the minima of the effective potential \(V_{\textrm{eff}}\), the evolution of the displacement coordinates defined by \(r = r_0 + \delta r,\theta = \theta _0 + \delta \theta \) is governed by the equations

$$\begin{aligned} \ddot{\delta r} + \omega ^2_{\textrm{r}} \, \delta r = 0, \quad \ddot{\delta \theta } + \omega ^2_{\mathrm{\theta }} \, \delta \theta = 0, \end{aligned}$$
(23)

where dot denotes derivative with respect to the proper time \(\tau \) of the particle (\(\dot{} = d /d \tau \)), and locally measured angular frequencies of the harmonic oscillatory motion are given by [32]

$$\begin{aligned} \omega ^2_{\textrm{r}} = \frac{\partial ^2 V_{\textrm{eff}}}{\partial r^2}, \quad \omega ^2_{\mathrm{\theta }} = \frac{1}{r^2 f(r)} \, \frac{\partial ^2 V_{\textrm{eff}}}{\partial \theta ^2}. \end{aligned}$$
(24)

Locally measured latitudinal (vertical) \(\omega _{\mathrm{\theta }}\) and radial (horizontal) \(\omega _{\textrm{r}}\) angular frequency of the harmonic oscillations of charged particles in the combined gravitational and electromagnetic background is then given by

$$\begin{aligned} \omega ^2_{\mathrm{\theta }}= & {} \frac{{{\mathcal {L}}}^2}{\left( l^2+r^2\right) ^2}-B^2, \end{aligned}$$
(25)
$$\begin{aligned} \omega ^2_{\textrm{r}}= & {} \frac{1}{2} \left( 1-\frac{2}{\sqrt{l^2+r^2}}\right) \Bigg \{2 B^2-\frac{2 \mathcal{L}^2 \left( l^2-3 r^2\right) }{\left( l^2+r^2\right) ^3}\nonumber \\ {}{} & {} - \frac{2 \left[ 2 r^2 \sqrt{l^2+r^2}-l^2 \left( \sqrt{l^2+r^2}-2\right) \right] }{\left( l^2+r^2\right) ^3 \left( \sqrt{l^2+r^2}-2\right) ^2}\nonumber \\{} & {} \times \left\{ \left[ {{\mathcal {L}}}-B \left( l^2+r^2\right) \right] ^2+l^2+r^2\right\} \Bigg \} \end{aligned}$$
(26)

where \({{\mathcal {L}}}\) is the specific angular momentum at the circular orbit (21).

Recall that there exists the third fundamental angular frequency of the epicyclic particle motion, namely the Keplerian (axial) frequency \(\omega _{\mathrm{\phi }}\), given by

$$\begin{aligned} \omega _{\mathrm{\phi }} = \frac{d\phi }{d \tau } = U^\phi = \frac{{{\mathcal {L}}}}{g_{\phi \phi }} - B=\frac{{{\mathcal {L}}}}{r^2+l^2} - B. \end{aligned}$$
(27)

The locally measured angular frequencies \(\omega _{\textrm{r}},\omega _\mathrm{\theta }\) and \(\omega _{\mathrm{\phi }}\), are connected to the angular frequencies measured by the static distant observers, \(\Omega \), by the gravitational redshift transformation

$$\begin{aligned} \Omega _{i} = \omega _{i} \, \frac{d \tau }{d t} = \frac{\omega _{\beta }}{f(r) {{\mathcal {E}}}(r)}, \end{aligned}$$
(28)

where \(i \in \{ \textrm{r}, \mathrm{\theta }, \mathrm{\phi }\} \), and \((f(r) {{\mathcal {E}}}(r))^{-1} \) is the redshift factor, given by the function f(r) and the particle specific energy at the circular orbit \(\mathcal{E}(r)\).

If the fundamental frequencies of the small harmonic oscillations related to the distant observers, \(\Omega _{\beta }\), are expressed in the physical units, their dimensionless form has to be extended by the factor \(c^3/(GM)\). Then the frequencies of the charged particle measured by the distant observers are generally given by

$$\begin{aligned} \nu _{\beta } = \frac{1}{2\pi } \frac{c^3}{GM} \, \Omega _{\beta }. \end{aligned}$$
(29)
Fig. 9
figure 9

Epicyclic frequencies radial profiles for characteristic values of the parameter l in units of M and magnetic parameter B giving regular black holes and wormholes and \(M=10\,M_\odot \). The vertical black line indicates a frequency ratio of 3:2 for the Schwarzschild case and red for given l and B (for \(l=0\) and \(B=0\) vertical lines coincide)

Full size image

The behaviour of the epicyclic frequencies \(\nu _{\textrm{r}}(r), \nu _\mathrm{\theta }(r)\) and \(\nu _{\mathrm{K(r)}}\), as functions of the radial coordinate r, is demonstrated for significant values of the electromagnetic interaction parameter, \(B=0\) and \(B=\pm 0.01\) and length parameter \(l=\{0,1,2,6\}\), in Fig. 9. For small radii, \( r\ge r_{\textrm{ISCO}}\), we see the strong gravitational influence on the angular frequencies, for large radii \(r \gg ~r_\textrm{ISCO}\) the impact of the uniform magnetic field is prevailing.

Close to the ISCO, the latitudinal \(\omega _{\mathrm{\theta }}\) frequency is always larger than the radial \(\omega _{\textrm{r}}\) frequency, \(\omega _{\mathrm{\theta }}>\omega _{\textrm{r}}\), but since radial coordinate, r is increasing, there always exists radius \(r_{1:1}\) (for cases with non-zero magnetic fields) where \(\omega _{\textrm{r}}=\omega _\mathrm{\theta }\) to give one additional position where these frequencies are in a 3:2 ratio (the upper and lower frequencies are interchanged similarly to the case of shared particles orbiting magnetized black holes [23, 24, 33, 34]).

In addition, there are two effects caused by the l parameter. One is associated with the approaching of the ISCO position to the center with increasing l, thus realizing stable circular orbits even in previously forbidden regions. The growth of the parameter l further reduces all frequencies considered by us. This changes the mutual ratios and therefore also the place where the given ratio is realized (and the values of these frequencies).

Fig. 10
figure 10

The position of the 3:2 ratio of the upper and lower frequencies for the two given models

Full size image

6 QPO analyses

In fact, black holes and wormholes do not radiate due to the extremely strong gravitational field near the horizon and throat, respectively. So, any electromagnetic information about the surface of the black hole. At the same time, black holes are sources of the radiation processes in the accretion disk. In this sense, QPOs are astrophysical phenomena that have been found in Fourier analyses of the noisy continuous electromagnetic radiation of the accretion disk around the black hole. The source mechanism of electromagnetic emission in the accretion disk is connected to particle oscillations. In fact, when a charged particle oscillates, it radiates an electromagnetic wave with the frequency the same as its oscillation frequency. Thus, the dynamics of the charged test particles around BHs can explain the origin of QPOs by the particle’s oscillations in the radial and angular directions. There are several QPO models that have been proposed and developed to explain different QPO sources.

In the following parts, we focus on analyzing the two popular models explaining the high-frequency quasi-periodic oscillations (HF QPOs) phenomena [35, 36]. The first is the Epicyclic Resonance (ER) model where \(\nu _\textrm{UP}= \nu _\textrm{r}\) and \(\nu _\textrm{DOWN}= \nu _\mathrm {\theta }\). The second is the relativistic recession (RP) model where \(\nu _\textrm{UP}= \nu _\mathrm {\phi }\) and \(\nu _\textrm{DOWN}= \nu _\mathrm {\phi }-\nu _\textrm{r}\).

In Fig. 10 related to the resonant radii \(r_\mathrm {3:2}\), it is shown how for individual models the radius evolves in dependence on l for typical values of B. The resonant phenomena reflect the dependence of \(\nu _U\) and \(\nu _L\) frequencies ratio \(\nu _U/\nu _L=3/2\) on the parameter l for the characteristic values of B. It is clear from the figure that for the ER model the position of the 3:2 ratio moves dramatically closer to the center. For the RP model, this approach to the center is less pronounced.

Fig. 11
figure 11

Possible values of the frequencies of upper and lower peaks of QPOs from SV BH & WHs in the RP model (left) and ER model (right) without magnetic field and various parameters l compared to a Schwarzschild and Kerr BH

Full size image

Figure 11 demonstrates relationships between the upper and lower frequencies of twin-peaked QPOs for both RP (left panel) and ER (right panel) models for various values of the length parameter l, considering \(B=0\). Here, we have fixed the mass of the central object as \(M=10M_\odot \). In this plot, two inclined lines for the frequency ratio are 3:2 in the orange line and 1:1 in the blue line. The shaded area under the blue line is called the graveyard for the twin-peaked QPOs, where no QPO can be observed. It is observed from the figure that the Upper and lower frequencies of the QPOs with the ratio 3:2 decrease with the increase of l in both models.

Fig. 12
figure 12

The same figure with Fig. 11, but for the Schwarzschild limit \(l=0\) for different values of B

Full size image

In Fig. 12, we have provided the \(\nu _U-\nu _L\) diagram for the Schwarzschild black hole (\(l=0\)) for different B in RP and ER models. It is observed that in both models, the upper-lower frequency curve for positive values of B, positions under the gray line corresponding to \(B=0\) case, while in the negative values of B, the frequencies increase and can mimic the spin of rotating Kerr black holes providing the same values of the frequencies.

Fig. 13
figure 13

The same figure with Fig. 11, but for \(B=10^{-3}\)

Full size image

In Fig. 13, we have fixed the magnetic interaction parameter as \(B=10^{-3}\) varying the length parameter. One can see from the figure that the ratio of possible values of the upper and lower frequencies decreases with the increase of l. We can see by comparison with Fig. 12 that there are no low-frequency QPOs lower than 50 Hz, due to the presence of the magnetic parameter.

Fig. 14
figure 14

The same figure with Fig. 11, but for \(B=-10^{-3}\)

Full size image

The upper-lower frequency diagram for \(B=-10^{-3}\) is shown in Fig. 14. It is also seen from the figure that the presence of a negative magnetic parameter increases the frequencies, while the l parameter decreases and the curves cross the Schwarzschild curve. That means, at certain values of B and l, their effects compensate each other and reflect the effect of the pure Schwarzschild black hole spacetime. Similarly, the combined effects of B and l could also mimic the spin of the Kerr black hole providing the same upper and lower frequencies.

Fig. 15
figure 15

Distance \(\delta \) between the position of 3:2 ratio of the upper and lower frequencies and ISCO position dependent on the parameter l for various parameters B for ER (left) and RP (right) models

Full size image

It is also interesting to show at what distance from the ISCO to the orbit where the upper and lower frequency ratio of 3:2 is realized. Figure 15 shows a difference \(\delta \) between ISCO and the QPO with ratio 3:2 positions (\(\delta =r_\mathrm {3:2}-r_\textrm{ISCO}\)) for the ER and RP models. It demonstrates that the 3:2 ratio appears nearer to ISCO in the RP model with compare to ER one, but the magnetic field has a smaller influence on this difference in the RP model than in the ER model. The QPO orbits come closer to ISCO under the effects of B, while the orbit goes far as the l parameter increase (at \(l>2\)). According to the RP model, the QPO orbit position is very close to ISCO, and it can help to determine ISCO measurements. It is worse to note that ISCO is the inner edge of the accretion disc around black holes being one of the important parameters to be measured in astrophysical observations of black holes.

7 QPOs in Simpsonv–Visser geometry immersed into the uniform magnetic field applied on astrophysical data

Here we use our analytically calculated epicyclic (Keplerian) frequencies given in Eqs. (25–27) to put limits on possible values of the length parameter l and magnetic field parameter B as given by comparison with implications of the frequency data from QPOs observed in microquasars and active galactic nuclei.

7.1 HF QPOs in the vicinity of microquasars

We selected three HF QPO sources in the microquasars GRO 1655-40, XTE 1550-564, and GRS 1915-105, which can be candidates for black holes or wormholes using the estimates of the mass of the central object given by methods independent of the measurements of HF QPOs.

Fig. 16
figure 16

Dependence of the upper frequency on the mass of the central object compared to three selected observed microquasars for characteristic values of parameters l and B. The upper panel shows fit for ER model (solid lines are ratio \(f_\textrm{UP}:f_\textrm{DOWN}=3:2\), dashed lines are ratio \(f_\textrm{UP}:f_\textrm{DOWN}=2:3\)), lower for RP model

Full size image
Table 1 Observations of QPOs around supermassive BHs [39]
Full size table
Fig. 17
figure 17

Dependence of the upper frequency on the mass of the central object compared to selected observed supermassive black holes [3] (listed in Table 1) for characteristic values of parameters l and B for ER model. Solid lines are ratio \(f_\textrm{UP}:f_\textrm{DOWN}=3:2\), dashed lines are ratio \(f_\textrm{UP}:f_\textrm{DOWN}=2:3\)

Full size image
Fig. 18
figure 18

Dependence of the upper frequency on the mass of the central object compared to selected observed supermassive black holes [3] (listed in Table 1) for characteristic values of parameters l and B for RP model. Solid lines are ratio \(f_\textrm{UP}:f_\textrm{DOWN}=3:2\), dashed lines are ratio \(f_\textrm{UP}:f_\textrm{DOWN}=2:3\)

Full size image

In Fig. 16 we show the mixed effect of l and B on the fitting of the astrophysical data of three selected microquasars for both models. It turns out that modeling QPOs in our SV BH (WH) spacetime with a magnetic field is unable to describe selected sources for the tested value of B. The effect of the magnetic field appears to be positive for our results, however, as the l parameter increases, the theory diverges more and more from the observed data. It means that the central object can be a wormhole in the presence of a higher value of magnetic parameter than the black hole. Moreover, the RP model gives more realistic and fits best the data compared to ER model. In both models, however, the fitted curves are generally located below the Schwarzschild curves, thereby excluding the possibility of a positive outcome for microquasars. Nevertheless, based on the results from [28], we did not expect any positive influence of the parameter l on the fits of microquasars. The primary aim was to explore the combinations of the effects of the parameter l and the magnetic field manifested through the parameter b. We can achieve very good fits by assuming a rotating black hole [37, 38].

7.2 HF QPOs from the vicinity of supermassive black hole

For supermassive black holes, we used QPO data presented in [39], the authors demonstrate a need for additional phenomena to be introduced in order to explain QPOs by the standard ER and PR models. We use for it the length parameter l and uniform magnetic field described by the parameter B. We use the same database of sources as [39] (see for more details in Table 1).

Figures 17 and 18 indicate a very good agreement between our theoretical models (ER and RP) and observational data. Both models can describe almost all sources. The exceptions are the four sources SwJ, ASASSN, XMMU, and TON S. However, we can describe the last two sources with higher values of the l parameter. Here (exactly the opposite as in microquasars), the magnetic field has a negative effect and the l parameter has a positive effect on the agreement between the theoretical models of QPOs and the measured data. However, the effect of the parameter l is stronger for our chosen values of B and l and will thus allow fitting most sources.

8 Conclusion

In this work, we have investigated test-charged particle dynamics and their oscillations at the equatorial plane of Simpson–Visser black holes and wormholes immersed in an externally asymptotically uniform magnetic field. First, we have calculated scalar invariants of the spacetime around the black hole and wormhole. It is shown that the increase of the length parameter causes decreasing in the scalar invariants and also shown that the Ricci scalar of the spacetime rapidly goes to zero near the event horizon of the black hole. It means that the spacetime is an asymptotically flat one. Then we solved Maxwell equations numerically and analyzed them graphically. It is obtained that the difference between Wald’s solution and the numerical solution is tiny and almost negligible. Therefore, for simplicity, one may apply Wald’s approach for the solution of 4-potentials of the electromagnetic field near the circular orbits.

Studies of the vertical component of the external magnetic field have shown that the magnetic field decreases with the increase of l and the component becomes zero at the horizon of the black hole, while it is zero at the center of the wormhole.

Then, we have derived effective potential for the radial motion of test charged particles around uniformly magnetized Simpson–Visser black holes and wormholes. Our analyses have shown that the effective potential slightly increases with the increase of the l parameter, while negative and positive values of the magnetic interaction parameter increase and decrease the effective potential, respectively. Moreover, the energy and angular momentum of the charged particle corresponding to circular orbits is also studied and also shown that the presence of l leads to a slight decrease in them. It is found that the ISCO radius decreases with the increase of l. It is also obtained that the presence of the positive magnetic parameter causes decreasing sufficiently, however, in the case of the negative magnetic parameter ISCO radius slightly decreases.

We also have derived expressions for the frequencies of the oscillations of test charged particles in the radial and vertical directions along stable circular orbits in the Simpson–Visser black hole/wormhole spacetimes in the presence of an external magnetic field and analyzed the effects of the parameters l and B. We have shown that the frequencies increase due to the presence of Lorentz forces.

The oscillation studies of QPOs were applied in the RP and ER models of HF QPOs. It has been obtained that the increase in the length parameter causes decreasing the upper and lower frequencies of twin-peaked QPOs. It means that the QPO frequencies generated around the SV black holes are much higher than the QPO frequencies generated around the SV wormholes with the same mass. Moreover, the upper and lower frequencies of low (high) frequency QPOs increase (decrease) due to the effects of the external magnetic field. It implies that kHz and mHz QPOs may not be observed in the presence of external magnetic fields. The frequency ratio decreases/increases (increases/decreases) with the increase of l in the presence of positive/negative magnetic interactions in the RP (ER) model. The distance between ISCO and QPO orbits with the frequency ratio 3:2 increase with the increase of l, while in the case when B is negative, the distance decrease in ER model. It is shown that the QPO orbits are located closer to ISCO in the RP model in comparison to ER model. However, the effect of magnetic interaction on the distance is higher in ER model than it has been studied in ER one.

Finally, we have applied our studies to real astrophysical QPO observed in microquasars that may help to determine the central compact object in the microquasar is a black hole or wormhole however, for these objects, it is shown that a rotating black hole provides a better explanation than a wormhole. Our analyses have shown that the sources of high-frequency QPOs can not be a candidate to be wormholes. Moreover, we have demonstrated well applicability of the ER and RP models due using the SV wormholes immersed in external magnetic fields.