Abstract
It was found that in a RS-like brane model the effective action for the massive vector KK modes of a U(1) gauge field was gauge invariant Fu et al., JHEP 2019(1) (2019). https://doi.org/10.1007/jhep01(2019)021. It is interesting to investigate the factors this gauge invariance maybe depend on, such as the geometry of the space-time, the number of the extra dimensions, and the dimension of the brane. We demonstrate that the three factors do not affect the gauge invariant formulation of the effective action, but influence the localization of the gauge invariant massive vector KK modes.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
At the beginning of last century the idea of extra dimension was proposed in Kaluza–Klein theory [1]. It was so fascinating that more and more physicists paid attention on it. In the string/M theory, the space-time was required to be ten/eleven dimensions. At the time, the extra dimensions were assumed to be compact into Planck length until the concept of “brane” was built up. Two famous brane models are the Arkani-Hamed–Dimopoulos–Dvali (ADD) brane model [2, 3] and the Randall–Sundrum (RS) brane [4, 5], which open a window to solve the hierarchy problem. The major difference between the two brane models is the geometry of the space-time: for the former the bulk space-time is flat, whereas for the latter it is warped through a non-factorizable factor, which releases the constrain on the length of the extra dimensions.
The number of the extra dimensions and the dimension of the brane are two basic parameters to describe a braneworld. As our observable universe is four dimensional, the most realistic brane is supposed to be four dimensional [6,7,8,9,10,11]. However, theoretically the brane can be any dimension. The brane with \(p (p>3)\) spatial dimensions is called \(p-\hbox {brane}\) [12]. Recently, in Ref. [13] the author gave a 1-dimensional braneworld solution based on a 2-dimensional gravity theory. Further, the brane model with more than one extra dimension is also interesting [14,15,16,17,18,19]. For example, in the brane with two extra dimensions, the problem of localization for bulk U(1) gauge field can be solved [20].
In braneworld theories, the observation is happened in the brane. The higher dimensional fields act as a series of Kaluza–Klein (KK) modes in the brane. The investigation of these KK modes is very important to explore the secret of the extra dimensions [21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41]. And it also provides a new way to study some problems, such as the origin of mass and the gauge invariance of the massive U(1) gauge field [42,43,44,45,46,47,48].
Gauge theories hold a prominent place in physics. The theories usually display some gauge symmetry, which can help to identify the corresponding conserved quantities [49, 50]. Maxwell’s theory is the first example realized to be a gauge theory with the U(1)gauge symmetry, which is the key to formulate quantum electrodynamics (QED). An extension to other gauge groups lays the foundation in particle physics. The quantum chromodynamics (QCD) is one of the most studied gauge theories. Gauge theories are also applied in condensed matter [51] and in the relativistic gravity and cosmology [52]. In this article we only focus on the U(1) gauge field in braneworld background, and try to talk about the gauge invariance of the massive vector KK modes.
In Refs. [42, 43, 53, 54] the authors introduced a coupling between a U(1) gauge field and a higher-spin (Kalb–Ramond) field to get a gauge invariant effective action for the massive vector field. However, in our works [44, 47, 48], we discovered that without any additional coupling for the massless bulk U(1) field, a gauge invariant effective action for the massive vector KK modes still can be got. The key was to make a general KK decomposition for the bulk U(1) gauge field. For example, in a RS-like brane with one extra dimension y the KK decomposition was chosen as
where \(\hat{\mathcal {A}}_{\mu }^{(n)},~\phi ^{(n)}\) denoting the vector and scalar KK modes are only the functions of the brane coordinates \(x^{\mu }\), and \(W_{1}^{(n)}(y),~W_{2}^{(n)}(y)\) are only the functions of extra dimension. When substituting the KK decomposition into the bulk action for the massless U(1) field, the term \(F_{\mu y}F^{\mu y}\) in the bulk action was just decomposed into three terms \(C_1\partial _\mu \phi ^{(n)}\partial ^\mu \phi ^{(n)} \),\(C_2\hat{\mathcal {A}}_{\mu }^{(n)}\hat{\mathcal {A}}^{\mu (n)}\) and \(C_3\partial _\mu \phi ^{(n)}\hat{\mathcal {A}}^{\mu (n)} \) in the effective action, where \(C_1\sim C_3\) were different coupling constants related to different integrals of the extra dimension y. Due to some relationships between \(C_1,~C_2,~C_3\), the effective action was found to be gauge invariant.
Here the scalar KK modes play a very important role in producing the gauge invariance of the massive vector KK modes. But the expressions of \(C_1,~C_2,~C_3\) will be changed in different cases. Thus it is not sure whether the relationships between them are also changed and whether the gauge invariance can be kept. In this work, we will study the factors this gauge invariance maybe depend on, such as the geometry of the background, the number of the extra dimensions and the dimension of the brane. It has been discussed that whether the number of the extra dimensions affects the gauge invariance in the warped space-time (RS-like brane models) [48]. In the warped space-time we then turn to the effect of the dimension of the brane in Sect. 2. Moreover we discuss the case in flat space-time in Sect. 3. Finally, a brief conclusion will be given in Sect. 4.
2 Gauge invariance of the massive vector KK mode in \((n+1)\)-dimensional RS-like braneworld
If the RS-like brane has n spatial dimensions, the line element is
where \(x_n\) denotes the spatial dimension of the brane. There is no constrain on the value of n in theory. In Ref. [13] the author gave a 1-dimensional braneworld solution based on a 2-dimensional gravity theory.
In order to investigate the KK modes of a massless bulk U(1) gauge field, we usually choose a KK decomposition as
where \(e^{b_1 B}, e^{b_2 B}\) are introduced for convenience with \(b_1,b_2\) two constants. Then substituted this KK decomposition into the bulk action of the field \(S=-\frac{1}{4} \int d^{n+2} x\; \sqrt{-g}\mathcal {F}^{MN}\mathcal {F}_{MN}\) with \(F_{MN}=\frac{1}{2}(\partial _M \mathcal {A}_N-\partial _N \mathcal {A}_M)\) it can be got that
with
Here all the coupling constants are supposed to be finite. From this effective action, the equations of motion (EOM) are derived as
At the same time, substituting the KK decomposition (4) into the EOM of the bulk field, we obtain that
where
As the EOM for the KK modes are unique, through comparing the Eqs. (10) and (11) with (12) and (13), we could find some relationships between the coupling constants. To make it clearer we suppose that the vector and scalar KK modes satisfy the orthogonality conditions
Therefore, we have
If substitute the relationship (17) into the definitions of \(I_2\) and \(I_4\), we see that
so that the effective action can be rewritten as
which is just gauge invariant under the gauge transformation \(\hat{\mathcal {A}}_\mu \rightarrow \hat{\mathcal {A}}_\mu +\partial _{\mu }\gamma ^{( n )}\), \(\phi ^{( n )} \rightarrow \phi ^{( n )}+\sqrt{I_2}\gamma ^{( n )}\) with \(\gamma ^{( n )}\) a scalar field in the brane.
Because \(I_2\) denotes the mass of the vector KK mode, we set \(I_2=m^{(n)2}\), and then \(I_4^{2}=m^{(n)2}\). With the help of Eqs. (15) and (16), we find two Schroding-like equations by a coordinate transformation \(dy=e^{B}dz\) and \(b_1=-\frac{n-2}{2}\):
where
with the prime denoting the derivative of z. If there are solutions for these two Schroding-like equations under the orthornality conditions (14), the gauge invariant vector KK modes can be localized in the brane. The result is consistent with one of the cases in Ref. [44] (mainly discussed the Hodge duality on p-brane). Here we emphasize two points:
-
for \(n=0\), with the KK decomposition (4) there are only two kinds of scalar KK modes in the brane, one is from \(\mathcal {A}_y\), and another is from \(\mathcal {A}_0=\sum _{n} \varphi ^{(n)} \;W_{1}^{(n)}(y) \;e^{b_1 B}\). In the effective action (533), the term \(\hat{\mathcal {F}}_{\mu \nu } \hat{\mathcal {F}}^{\mu \nu }\) disappears. With the same derivation, the effective action is found to be
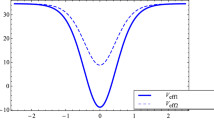
$$\begin{aligned} S_{1} =-\frac{1}{8}\;\sum _{n} \int dt\;\big [\big (\partial _0\phi ^{(n)}\big )^2 +m^{(n)2}\varphi ^{(n)2} -2m^{(n)}\varphi ^{(n)} \partial _0\phi ^{(n)}\big ]. \end{aligned}$$(24)The scalar KK modes \(\phi ^{(n)}\) are all massless, and another kind of scalar ones \(\varphi ^{(n)}\) are massive. The masses of the scalars can be solved from the Schroding-like equation (20) with the effective potential \(V_1=-B''+B^{'2}\). For example, using one of the solutions of the warp factor \(B(y)=-\kappa \ln \cosh (My)\) with \(\kappa ,~M\) constants in Ref. [13] , we find that the effective potential \(V_1\) is a PT-like potential with \(\kappa >1\). So that there are bound scalar KK modes;
-
for \(n=2\) (the brane has two spatial dimensions) the effective potential vanishes which can be seen from (22) and (23). So that we have no bound KK modes. However, for the flat case, there is no such situation.
In this case, the relationship (18) is the key leading to the gauge invariant effective action. Nonetheless it seems that the influence of the warp factor can not be ignored. We wonder if the space-time is flat, whether the gauge invariance will be destroyed.
3 Gauge invariance of the massive vector KK mode in brane within flat space-time
3.1 KK modes in brane with one extra dimension in flat space-time
First we consider a brane model with one compact extra dimension in flat space-time. For a massless bulk U(1) gauge field, with the KK decomposition as (1) and (2), its bulk action can be reduced as
with \(I_1=\int {dy} \;W_{1}^{(n)2},~C_1=\int {dy} \;W_{2}^{(n)2}, C_2=\int {dy} \;(\partial _y W_{1}^{(n)})^2,\) \(C_3= \int {dy} \;W_{2}^{(n)}\partial _y W_{1}^{(n)}\). Motivated by the discussion in RS-like brane, we think that the key to get the gauge invariant effective action is to find the relationship between the coupling constants.
To show the details, we derive the EOM for KK modes from two ways. One is from substituting the KK decomposition into the EOM for the bulk field:
and another is from the effective action (25):
Through comparing the two group of EOM, we find that
With the hypothesis of the orthornality conditions
there is just the relationship
Due to this relationship the effective action is derived to be a gauge invariant formulation:
which is gauge invariant under the gauge transformation \(\hat{\mathcal {A}}_\mu \rightarrow \hat{\mathcal {A}}_\mu +\sqrt{C_1}\partial _{\mu }\gamma ^{( n )}\), \(\phi ^{( n )} \rightarrow \phi ^{( n )}+\sqrt{C_2}\gamma ^{( n )}\) with \(\gamma ^{( n )}\) a scalar field in the brane. And two equations satisfied by the KK modes also can be derived from (30):
For these two equations, there must be some bound massive vector KK modes because of the compact extra dimensions.
If the brane has one more extra dimension, one more type of scalar KK modes will appear. All types of scalar KK modes will couple with the vector ones, respectively. Arbitrary two types of scalars will interact with each other. More coupling constants will be presented in the effective action. Although we have proved that the gauge invariance of the massive vector KK modes is not changed in the RS-like brane model [48] with different number of the extra dimension, it is desirable that whether in the flat space-time the same result can be got.
3.2 KK modes in brane with \(d~(d>1)\) extra dimensions in flat space-time
For a braneworld in flat space-time with \(d~(d>1)\) extra dimensions \(y_1,y_2\cdots y_i\) ( \(i=1,2,\cdots ,d\)), there are d types of scalar KK modes. For the component \(A_\mu \), we also use the KK decomposition (1), and for the component \(A_{i}\), we choose the KK decomposition as
where \(\phi ^{(n)}_i(x^{\mu }) \) is the scalar KK mode and \(U_{i}^{(n)}\) is the function of all the extra dimensions.
Then we substitute the KK decomposition into the bulk action. The difference with the case for one extra dimension is that in the bulk action there is the term \(F^{ij}F_{ij}\), which leads to the masses of the scalar KK modes \(\phi ^{(n)}_i\) and \(\phi ^{(n)}_j\) and the coupling between them. Thus the effective action becomes
Here \(I_1{=}\int dy_1\ldots \int d{y_d} \;W_{1}^{(n)2}\), \(C_{1_i}{=}\int dy_1\ldots \int d{y_d} (\partial _i W_{1}^{(n)})^2\), \(C_{2_i}=\int dy_1\ldots \int d{y_d} \;U_{i}^{(n)2}\), \(C_{3_i}= \int dy_1 \ldots \int d{y_d} \;U_{i}^{(n)}\partial _i W_{1}^{(n)} \), \(C_{{4_{ij}}}=\int dy_1\ldots \int d{y_d} (\partial _i U_{j}^{(n)})^2\), \(C_{{5_{ij}}}=\int dy_1 \ldots \int d{y_d}(\partial _j U_{i})^2\), and \(C_{6_{ij}}{=}\int dy_1 \ldots \int d{y_d}(\partial _i U_{j})(\partial _j U_{i})\). For \(d=1\), \(C_{1_1},C_{2_1},C_{3_1}\) are just equal to \(C_1,C_2,C_3\) for the case with one extra dimension. We should sum all the terms for \(i,j=1,2,\ldots ,d\) in the effective action.
We also can check the the relationships between the coupling constants through the EOM of the KK modes. Firstly, from (37) we can get the EOM for the vector KK modes and scalar ones \(\phi _i^{(n)},\phi _j^{(n)}\):
On the other hand, substituted the KK decomposition into the EOM for the bulk field, there are
The EOM for the KK modes are unique, which indicates the relationships between the coupling constants.
-
Firstly, comparing the Eqs. (38) and (39) with (41) and(42), we have
$$\begin{aligned} \partial _i^2\;W_{1}^{(n)}= & {} -\frac{C_{1_i}}{I_1}W_{1}^{(n)}, \end{aligned}$$(44)and
$$\begin{aligned} -\frac{C_{3_i}}{I_1}W_{1}^{(n)}=\partial _i U_{i}^{(n)},~~~~~\frac{C_{3_i}}{C_{2_i}}U_{i}^{(n)}=\partial _i W_{1}^{(n)}, \end{aligned}$$(45)from which it is easy to deduce that
$$\begin{aligned} C_{3_i}^2=C_{1_i}C_{2_i}. \end{aligned}$$(46)For \(d=1\), we have seen this relationship in Sect. 3.1. For more extra dimensions, all through there are more scalar KK modes, each scalar interacts with vector by the same way. This means that for the scalar KK mode \(\phi ^{(n)}_j\) there is the relationship as \(C_{3_j}^2=C_{1_j}C_{2_j}\).
-
Secondly, with the Eqs. (39) , (40) and (42) ,(43), we obtain the following equations:
$$\begin{aligned} \partial _j^2\;U_{i}^{(n)}= & {} -\frac{C_{{4_{ij}}}}{C_{2_i}}U_{i}^{(n)}, \end{aligned}$$(47)$$\begin{aligned} \partial _i^2\;U_{j}^{(n)}= & {} -\frac{C_{{5_{ij}}}}{C_{2_j}}U_{j}^{(n)}, \end{aligned}$$(48)and
$$\begin{aligned} \partial _j\partial _i U_{j}^{(n)}= & {} \frac{C_{6_{ij}}}{C_{2_i}}U_{i}^{(n)}, \end{aligned}$$(49)$$\begin{aligned} \partial _i\partial _j U_{i}^{(n)}= & {} \frac{C_{6_{ij}}}{C_{2_j}}U_{j}^{(n)}. \end{aligned}$$(50)These equations will tell us more relationships between the coupling constants. For simplicity, we assume the orthornality conditions
$$\begin{aligned} I_1=1,~C_{2_i}=C_{2_j}=1. \end{aligned}$$(51)The Eqs. (47) and (48) show the constrains on the masses of the scalar KK modes. As the scalar and vector are not independent, the masses of them must be related. To find this, we consider the term \(\partial _j\partial _i\partial _j W_{1}^{(n)}\). Because \(\partial _j\partial _i\partial _j W_{1}^{(n)}=\partial _i\partial _j\partial _j W_{1}^{(n)}=-C_{1_j}\partial _i W_{1}^{(n)}=-C_{1_j}{C_{3_i}}U_{i}^{(n)}\) and \(\partial _j\partial _i\partial _j W_{1}^{(n)}=\) \({C_{3_i}}\partial _j\partial _j U_{i}^{(n)}=-{C_{3_i}}C_{{4_{ij}}}U_{i}^{(n)}\), there is
$$\begin{aligned} C_{1_j}=C_{{4_{ij}}}. \end{aligned}$$(52)Similarly, through the term \(\partial _i\partial _j\partial _i W_{1}^{(n)}\) we have
$$\begin{aligned} C_{1_i}=C_{{5_{ij}}}. \end{aligned}$$(53)Then we would like to find the relationships between \(C_{6_{ij}}\) and \(C_{5_i}, C_{6_j}\). As
$$\begin{aligned} C_{6_{ij}}U_{i}^{(n)}= & {} \partial _j\partial _i \left( \frac{1}{C_{3_j}}\partial _j W_{1}^{(n)}\right) =\frac{1}{C_{3_j}}\partial _i\partial _j\partial _j W_{1}^{(n)}\\= & {} \frac{C_{1_j}}{C_{3_j}}\partial _i W_{1}^{(n)} =\frac{C_{1_j}}{C_{3_j}}C_{3_i}U_{i}^{(n)} \end{aligned}$$it is clear that
$$\begin{aligned} C_{6_{ij}}=\frac{C_{1_j}}{C_{3_j}}C_{3_i} =\sqrt{C_{1_i}C_{1_j}}. \end{aligned}$$(54)
Ultimately the effective action can be rewritten as
which is just gauge invariant under the gauge transformation \(\hat{\mathcal {A}}\rightarrow \hat{\mathcal {A}}+\partial _{\mu }\gamma ^{( n )}\), \(\phi _i^{( n )} \rightarrow \phi _i^{( n )}+\sqrt{C_{1_i}}\gamma ^{( n )}\).
If all the extra dimensions are compact, there are massive bound vector KK modes. All of the extra dimensions contribute on the masses of the vector KK modes. However, for the scalar ones, they only obtain masses from \((d-1)\) extra dimensions.
4 Conclusion
In this work, we discussed the gauge invariance of massive vector KK modes for a massless bulk U(1) gauge field in different brane models. First we investigated whether the dimension of the brane affects the gauge invariance, and it was got that the dimension of the brane determines the effective potentials constraining the KK modes, but does not change the gauge invariance of the effective action. Second we considered the case in braneworld within flat space-time. Although the space-time is flat, there is also a gauge invariant effective action for a massless bulk U(1) gauge field, whatever the number of the extra dimensions is.
Throughout the paper, we found that no matter in the warped space-time or the flat case, the gauge invariance of the effective action is independent on the number of the dimension and the dimension of the brane. Nevertheless this is based on the orthornality conditions for the KK modes. Only the KK modes satisfying these orthornality conditions are stable. For the warped case, through solving the Schroding-like equation (20) or (21) under the orthornality conditions we can find the stable gauge invariant massive vector KK modes. Although whether there are solutions depends on the warp factor of a brane model; for the flat case, as the extra dimensions are compact, there are always stable KK modes. In a sense the gauge invariance is caused by the geometry of the space-time, i.e., the braneworld background. However, whether there is fundamental physics should be given more investigation.
Data Availability Statement
This manuscript has no associated data or the data will not be deposited. [Authors’ comment: All data generated or analysed during this study are included in this published article.]
References
O. Klein, Nature 118, 516 (1926)
N. Arkani-Hamed, S. Dimopoulos, G. Dvali, Phys. Lett. B 429, 263 (1998)
I. Antoniadis, N. Arkani-Hamed, S. Dimopoulos, G. Dvali, Phys. Lett. B 436, 257 (1998). https://doi.org/10.1016/S0370-2693(98)00860-0
L. Randall, R. Sundrum, Phys. Rev. Lett. 83, 3370 (1999). https://doi.org/10.1103/PhysRevLett.83.3370
L. Randall, R. Sundrum, Phys. Rev. Lett. 83, 4690 (1999). https://doi.org/10.1103/PhysRevLett.83.4690
M. Gremm, Phys. Rev. D 62, 044017 (2000). https://doi.org/10.1103/PhysRevD.62.044017
S. Kobayashi, K. Koyama, J. Soda, Phys. Rev. D 65, 064014 (2002). https://doi.org/10.1103/PhysRevD.65.064014
O. Arias, R. Cardenas, I. Quiros, Nucl. Phys. B 643, 187 (2002). https://doi.org/10.1016/S0550-3213(02)00691-0
D. Bazeia, A. Gomes, JHEP 0405, 012 (2004). https://doi.org/10.1088/1126-6708/2004/05/012
D. Bazeia, A.S. Lobao Jr., Phys. Lett. B 729, 127 (2014). https://doi.org/10.1016/j.physletb.2014.01.011
D. Bazeia, A.S. Lobao Jr., R. Menezes, Phys. Lett. B 743, 98 (2015). https://doi.org/10.1016/j.physletb.2015.02.037
C.E. Fu, Y.X. Liu, K. Yang, S.W. Wei, JHEP 1210, 060 (2012). https://doi.org/10.1007/JHEP10(2012)060
Y. Zhong, JHEP 2021(4), 118 (2021). https://doi.org/10.1007/jhep04(2021)118
R. Koley, S. Kar, Class. Quantum Gravity 24, 79 (2007). https://doi.org/10.1088/0264-9381/24/1/004
D.S.V. Dzhunushaliev, V. Folomeev, S. Aguilar-Rudametkin, Phys. Rev. D 77, 044006 (2008)
L.F.F. Freitas, G. Alencar, R.R. Landim, Eur. Phys. J. C 80(12), 1141 (2020). https://doi.org/10.1140/epjc/s10052-020-08670-9020-08670-9
L.J.S. Sousa, W. Cruz, C. Almeida, Phys. Rev. D 89(6) (2014). https://doi.org/10.1103/physrevd.89.064006
P.R. Archer, S.J. Huber, JHEP 1103, 018 (2011). https://doi.org/10.1007/JHEP03(2011)018
A. Moreira, J. Silva, F. Lima, C. Almeida, Phys. Rev. D 103(6) (2021). https://doi.org/10.1103/physrevd.103.064046
S.L. Parameswaran, S. Randjbar-Daemi, A. Salvio, Nucl. Phys. B 767, 54 (2007). https://doi.org/10.1016/j.nuclphysb.2006.12.020
A. Karch, L. Randall, JHEP 0105, 008 (2001)
D. Youm, Phys. Rev. D 64, 127501 (2001). https://doi.org/10.1103/PhysRevD.64.127501
S. Ichinose, Phys. Rev. D 66, 104015 (2002). https://doi.org/10.1103/PhysRevD.66.104015
A. Wang, Phys. Rev. D 66, 024024 (2002). https://doi.org/10.1103/PhysRevD.66.024024
D. Langlois, M. Sasaki, Phys. Rev. D 68, 064012 (2003). https://doi.org/10.1103/PhysRevD.68.064012
B. Mukhopadhyaya, S. Sen, S. Sen, S. SenGupta, Phys. Rev. D 70, 066009 (2004). https://doi.org/10.1103/PhysRevD.70.066009
A. Melfo, N. Pantoja, J.D. Tempo, Phys. Rev. D 73, 044033 (2006). https://doi.org/10.1103/PhysRevD.73.044033
R. Davies, D.P. George, Phys. Rev. D 76, 104010 (2007). https://doi.org/10.1103/PhysRevD.76.104010
Z.Q. Guo, B.Q. Ma, JHEP 0808, 065 (2008). https://doi.org/10.1088/1126-6708/2008/08/065
Y.X. Liu, X.H. Zhang, L.D. Zhang, Y.S. Duan, JHEP 0802, 067 (2008). https://doi.org/10.1088/1126-6708/2008/02/067
R. Koley, J. Mitra, S. SenGupta, Phys. Rev. D 79, 041902 (2009). https://doi.org/10.1103/PhysRevD.79.041902
G. Alencar, R. Landim, M. Tahim, C. Muniz, R. Costa Filho, Phys. Lett. B 693, 503 (2010). https://doi.org/10.1016/j.physletb.2010.09.005
A. Herrera-Aguilar, D. Malagon-Morejon, R.R. Mora-Luna, JHEP 1011, 015 (2010). https://doi.org/10.1007/JHEP11(2010)015
W. Cruz, M. Tahim, C. Almeida, Phys. Lett. B 686, 259 (2010). https://doi.org/10.1016/j.physletb.2010.02.064
K. Yang, Y.X. Liu, Y. Zhong, X.L. Du, S.W. Wei, Phys. Rev. D 86, 127502 (2012). https://doi.org/10.1103/PhysRevD.86.127502
A. Das, S. SenGupta, Phys. Lett. B 718, 1566 (2013). https://doi.org/10.1016/j.physletb.2012.12.056
P. Jones, G. Munoz, D. Singleton, Triyanta, Phys. Rev. D 88, 025048 (2013). https://doi.org/10.1103/PhysRevD.88.025048
S. Chakraborty, S. SenGupta, Phys. Rev. D 89(12), 126001 (2014). https://doi.org/10.1103/PhysRevD.89.126001
Z.H. Zhao, Q.Y. Xie, Y. Zhong, Class. Quantum Gravity 32(3) (2014)
L.J.S. Sousa, C.A.S. Silva, D.M. Dantas, C.A.S. Almeida, Phys. Lett. B 731, 64 (2014). https://doi.org/10.1016/j.physletb.2014.02.010
C.A. Vaquera-Araujo, O. Corradini, Eur. Phys. J. C 75(2), 48 (2015). https://doi.org/10.1140/epjc/s10052-014-3251-2
T.R. Govindarajan, S.D. Rindani, M. Sivakumar, Phys. Rev. D 32, 454 (1985). https://doi.org/10.1103/PhysRevD.32.454
S.D. Rindani, M. Sivakumar, Phys. Rev. D 32, 3238 (1985)
C.E. Fu, Y.X. Liu, H. Guo, S.L. Zhang, Phys. Rev. D 93(6), 064007 (2016). https://doi.org/10.1103/physrevd.93.064007
A. Smailagic, E. Spallucci, Phys. Rev. D 61, 067701 (2000). https://doi.org/10.1103/PhysRevD.61.067701
C.T. Hill, S. Pokorski, J. Wang, Phys. Rev. D 64, 105005 (2001). https://doi.org/10.1103/PhysRevD.64.105005
C.E. Fu, Y. Zhong, Y.X. Liu, JHEP 2019(1) (2019). https://doi.org/10.1007/jhep01(2019)021
C.E. Fu, Y. Zhong, H. Guo, L. Zhao, Z.Q. Chen, Phys. Lett. B 810, 135781 (2020). https://doi.org/10.1016/j.physletb.2020.135781
S. Pokorski, Gauge Field Theories (Cambridge University Press, Cambridge, 1987)
S. Weinberg, The Quantum Theory of Fields (Cambridge University Press, Cambridge, 1995)
R.P. Feynman, QED: The Strange Theory of Light and Matter (Princeton University Press, Princeton, 2006)
M. Novello, J.M. Salim, Phys. Rev. D 20, 377 (1979). https://doi.org/10.1103/PhysRevD.20.377
A. Khoudeir, Phys. Rev. D 59(2) (1998). https://doi.org/10.1103/physrevd.59.027702
A. Khoudeir, Phys. Rev. D 59, 027702 (1999). https://doi.org/10.1103/PhysRevD.59.027702
Acknowledgements
This work was supported by the Natural Science Foundation of Shaanxi Province (No. 2022JQ-037).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3. SCOAP3 supports the goals of the International Year of Basic Sciences for Sustainable Development.
About this article
Cite this article
Fu, CE. Gauge invariant massive vector Kaluza–Klein modes of U(1)gauge field in braneworld. Eur. Phys. J. C 82, 1013 (2022). https://doi.org/10.1140/epjc/s10052-022-10967-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1140/epjc/s10052-022-10967-w