Skip to main content
SpringerLink
Log in

A Piecewise-Linear Reconstruction to Reduce the Dissipation of the HLL Method in Solving the Gas Dynamics Equations

  • Published:
Numerical Analysis and Applications Aims and scope Submit manuscript

Abstract

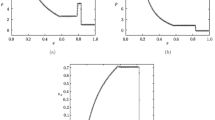
In this paper, the construction of the original “Harten–Lax–van Leer” method using a piecewise-linear reconstruction of physical variables is described. The thus obtained numerical method makes it possible to reproduce the pressure, density, and velocity profiles with low dissipation at the discontinuities. To verify the method, classical problems of discontinuity breakdown are used with analytical solutions based on various configurations of shock waves, contact discontinuities, and rarefaction waves. The order of accuracy of the numerical method is studied using a Sod-type problem. It is shown that the greatest decrease in the order of accuracy is when a rarefaction wave is reproduced. The numerical method is verified by means of a three-dimensional Sedov test of a point explosion and a problem of a supernova Ia type explosion with two symmetric ignition points leading to the formation of a G1.9+0.3-type remnant.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

REFERENCES

  1. Godunov, S.K., A Difference Method for Numerical Calculation of Discontinuous Solutions of the Equations of Hydrodynamics, Mat. Sb., 1959, vol. 47, pp. 271–306.

    MathSciNet  MATH  Google Scholar 

  2. Balsara, D., Higher-Order Accurate Space-Time Schemes for Computational Astrophysics—Part I: Finite Volume Methods, Liv. Rev. Comput. Astrophys., 2017, vol. 3, article no. 2.

    Article  Google Scholar 

  3. Harten, A., Lax, P., and van Leer, B., On Upstream Differencing and Godunov-Type Schemes for Hyperbolic Conservation Laws, SIAM Rev., 1983, vol. 25, pp. 289–315.

    Article  MathSciNet  Google Scholar 

  4. Simon, S. and Mandal, J.C., A Cure for Numerical Shock Instability in HLLC Riemann Solver Using Antidiffusion Control, Comp. Fluids, 2018, vol. 174, pp. 144–166.

    Article  MathSciNet  Google Scholar 

  5. Rusanov, V.V., The Calculation of the Interaction of Non-Stationary Shock Waves with Barriers, Comput. Math. Math. Phys., 1961, vol. 1, pp. 267–279.

    MathSciNet  Google Scholar 

  6. Einfeldt, B., On Godunov-Type Methods for Gas Dynamics, SIAM J. Num. An., 1988, vol. 25, pp. 294–318.

    Article  MathSciNet  Google Scholar 

  7. Einfeldt, B., Munz, C.-D., Roe, P., and Sjogreen, B., On Godunov-Type Methods near Low Densities, J. Comput. Phys., 1991, vol. 92, pp. 273–295.

    Article  MathSciNet  Google Scholar 

  8. Toro, E., Spruce, M., and Speares, W., Restoration of the Contact Surface in the Harten–Lax–van Leer Riemann Solver, Shock Waves, 1994, vol. 4, pp. 25–34.

    Article  Google Scholar 

  9. Mandal, J.C. and Panwar, V., Robust HLL-Type Riemann Solver Capable of Resolving Contact Discontinuity, Comp. Fluids, 2012, vol. 63, pp. 148–164.

    Article  MathSciNet  Google Scholar 

  10. Xie, W., Li, W., and Li, H., On Numerical Instabilities of Godunov-Type Schemes for Strong Shocks, J. Comput. Phys., 2017, vol. 350, pp. 607–637.

    Article  MathSciNet  Google Scholar 

  11. Dumbser, M. and Balsara, D., A New Efficient Formulation of the HLLEM Riemann Solver for General Conservative and Non-Conservative Hyperbolic Systems, J. Comput. Phys., 2016, vol. 304, pp. 275–319.

    Article  MathSciNet  Google Scholar 

  12. Miyoshi, T. and Kusano, K., A Multi-State HLL Approximate Riemann Solver for Ideal Magnetohydrodynamics, J. Comput. Phys., 2005, vol. 208, pp. 315–344.

    Article  MathSciNet  Google Scholar 

  13. Pandolfi, M. and D’Ambrosio, D., Numerical Instabilities in Upwind Methods: Analysis and Cures for the “Carbuncle” Phenomenon, J. Comput. Phys., 2000, vol. 166, pp. 271–301.

    Article  MathSciNet  Google Scholar 

  14. Chauvat, Y., Moschetta, J.-M., and Gressier, J., Shock Wave Numerical Structure and the Carbuncle Phenomenon, Int. J. Num. Meth. Fluids, 2005, vol. 47, pp. 903–909.

    Article  Google Scholar 

  15. Roe, P., Approximate Riemann Solver, Parameter Vectors and Difference Schemes, J. Comput. Phys., 1981, vol. 43, pp. 357–372.

    Article  MathSciNet  Google Scholar 

  16. Davis, S.F., A Rotationally Biased Upwind Difference Scheme for the Euler Equations, J. Comput. Phys., 1984, vol. 56, pp. 65–92.

    Article  Google Scholar 

  17. Levy, D.W., Powell, K.G., and van Leer, B., Use of a Rotated Riemann Solver for the Two-Dimensional Euler Equations, J. Comput. Phys., 1993, vol. 106, pp. 201–214.

    Article  Google Scholar 

  18. Nishikawa, H. and Kitamura, K., Very Simple, Carbuncle-Free, Boundary-Layer-Resolving, Rotated-Hybrid Riemann Solvers, J. Comput. Phys., 2008, vol. 227, pp. 2560–2581.

    Article  MathSciNet  Google Scholar 

  19. Kulikov, I.M., Chernykh, I.G., Glinskiy, B.M., and Protasov, V.A., An Efficient Optimization of HLL Method for the Second Generation of Intel Xeon Phi Processor, Lobachevskii J. Math., 2018, vol. 39, iss. 4, pp. 543–551.

    Article  MathSciNet  Google Scholar 

  20. Kulikov, I.M., Chernykh, I.G., and Tutukov, A.V., A New Parallel Intel Xeon Phi Hydrodynamics Code for Massively Parallel Supercomputers, Lobachevskii J. Math., 2018, vol. 39, iss. 9, pp. 1207–1216.

    Article  MathSciNet  Google Scholar 

  21. Kulikov, I., Chernykh, I., and Tutukov, A., A New Hydrodynamic Code with Explicit Vectorization Instructions Optimizations That Is Dedicated to the Numerical Simulation of Astrophysical Gas Flow. I. Numerical Method, Tests, and Model Problems, The Astrophys. J. Suppl. Ser., 2019, vol. 243, article no. 4.

    Article  Google Scholar 

  22. Chernykh, I., Kulikov, I., and Tutukov, A., Hydrogen–Helium Chemical and Nuclear Galaxy Collision: Hydrodynamic Simulations on AVX-512 Supercomputers, J. Comput. Appl. Math., 2021, vol. 391, article no. 113395.

    Article  MathSciNet  Google Scholar 

  23. Kim, S.D., Lee, B.J., Lee, H.J., and Jeung, I.S., Robust HLLC Riemann Solver with Weighted Average Flux Scheme for Strong Shock, J. Comput. Phys., 2009, vol. 228, pp. 7634–7642.

    Article  MathSciNet  Google Scholar 

  24. Simon, S. and Mandal, J.C., A Simple Cure for Numerical Shock Instability in the HLLC Riemann Solver, J. Comput. Phys., 2019, vol. 378, pp. 477–496.

    Article  MathSciNet  Google Scholar 

  25. Rodionov, A.V., Artificial Viscosity in Godunov-Type Schemes to Cure the Carbuncle Phenomenon, J. Comput. Phys., 2017, vol. 345, pp. 308–329.

    Article  MathSciNet  Google Scholar 

  26. Rodionov, A.V., Artificial Viscosity to Cure the Shock Instability in High-Order Godunov-Type Schemes, Comp. Fluids, 2019, vol. 190, pp. 77–97.

    Article  MathSciNet  Google Scholar 

  27. Kulikov, I.M., Chernykh, I.G., Sapetina, A.F., Lomakin, S.V., and Tutukov, A.V., A New Rusanov-Type Solver with a Local Linear Solution Reconstruction for Numerical Modeling of White Dwarf Mergers by Means Massive Parallel Supercomputers, Lobachevskii J. Math., 2020, vol. 41, iss. 8, pp. 1485–1491.

    Article  MathSciNet  Google Scholar 

  28. Guy, C., A HLL-Rankine–Hugoniot Riemann Solver for Complex Non-Linear Hyperbolic Problems, J. Comput. Phys., 2013, vol. 251, pp. 156–193.

    Article  MathSciNet  Google Scholar 

  29. Capdeville, G., A High-Order Multi-Dimensional HLL-Riemann Solver for Non-Linear Euler Equations, J. Comput. Phys., 2011, vol. 230, pp. 2915–2951.

    Article  MathSciNet  Google Scholar 

  30. Toro, E., Riemann Solvers and Numerical Methods for Fluid Dynamics: A Practical Introduction, Springer-Verlag, 2009.

    Book  Google Scholar 

  31. Kriksin, Yu.A. and Tishkin, V.F., Numerical Solution of the Einfeldt Problem Based on the Discontinuous Galerkin Method, Preprint of the Keldysh Institute of Applied Mathematics RAS, no. 90, Moscow, 2019.

  32. Kriksin, Y.A. and Tishkin, V.F., Variational Entropic Regularization of the Discontinuous Galerkin Method for Gasdynamic Equations, Math. Models Comp. Simulat., 2019, vol. 11, pp. 1032–1040.

    Article  MathSciNet  Google Scholar 

  33. Reinecke, M., Hillebrandt, W., and Niemeyer, J.C., Three-Dimensional Simulations of Type Ia Supernovae, Astron. Astrophys., 2002, vol. 391, pp. 1167–1172.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Computational Mathematics and Mathematical Geophysics, Siberian Branch, Russian Academy of Sciences, Novosibirsk, Russia

    I. M. Kulikov

Authors
  1. I. M. Kulikov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to I. M. Kulikov.

Additional information

Translated from Sibirskii Zhurnal Vychislitel’noi Matematiki, 2022, Vol. 25, No. 2, pp. 141-156. https://doi.org/10.15372/SJNM20220204.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kulikov, I.M. A Piecewise-Linear Reconstruction to Reduce the Dissipation of the HLL Method in Solving the Gas Dynamics Equations. Numer. Analys. Appl. 15, 112–124 (2022). https://doi.org/10.1134/S1995423922020045

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1995423922020045

Keywords

Search

Navigation