Service outsourcing under co-opetition and information asymmetry

Abstract

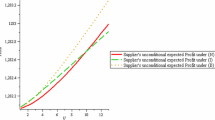
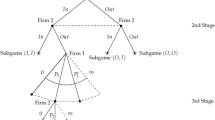
Co-opetition refers to the phenomenon that firms simultaneously cooperate and compete in order to maximize their profits. This paper studies the contracting for an outsourcing supply chain (a user company vs. a service provider) in the presence of co-opetition and information asymmetry. The user company outsources part of his service capacity at a discount price to the service provider for sale. The service provider charges a commission for doing outsourcing work and competes with the user company for the service capacity to satisfy their respective demands. We solve for the service provider’s optimal commission decision and the user company’s optimal outsourcing decisions (outsourcing volume and price discount) when the user company has private information about his service capacity. Specifically, we highlight the following observations. For the service provider, a menu of two-part tariffs that consist of a fixed commission and a per-volume commission can reveal the true type of the user company’s capacity; the user company’s optimal outsourcing proportion is quasi-convex and the optimal price discount is non-decreasing in his capacity volume, which is counterintuitive.

Appendix: Proofs of Lemmas and Theorems

Proof of Lemma 1

Under full information, the IC constraint in the optimization problem (4) can be removed. In this case, following the IR constraint we have

$$ r_{i}^{f} (\alpha ,\beta \lambda_{i} ) \le\,p_{0} \hbox{min} \{ (1 - \beta )\lambda_{i} ,d_{u} \} + \alpha p_{0} \hbox{min} \{ \beta \lambda_{i} ,d_{c} \} - p_{0} \hbox{min} \{ \lambda_{i} ,d_{u} \} + \bar{k}\beta \lambda_{i} . $$

Since the service provider’s profit is increasing in the commission level $r_{i}^{f} (\alpha ,\beta \lambda_{i} ),$ then the optimal commission level under full information satisfies Eq. (7).

Recall that $A_{1} \le A_{2} ,$ then $d_{u} \le d_{c} .$ Thus, the formulation of $r_{i}^{f} (\alpha ,\beta \lambda_{i} )$ can be simplified as follows:

Case 1 If $d_{u} \le {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {(1 - \beta )}}} \right. \kern-0pt} {(1 - \beta )}} \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta },$ then

$$ r_{i}^{f} (\alpha ,\beta \lambda_{i} ) = \left\{ {\begin{array}{*{20}l} {(\bar{k} - (1 - \alpha )p_{0} )\beta \lambda_{i} ,} \hfill & {{\text{if}}\;0 \le \lambda_{i} \le d_{u} } \hfill \\ (\bar{k} - (1 - \alpha )p_{0} )\beta \lambda_{i} &\\\quad + p_{0} (\lambda_{i} - d_{u} ), & {{\text{if}}\;d_{u} \le \lambda_{i} \le {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {(1 - \beta )}}} \right. \kern-0pt} {(1 - \beta )}}} \hfill \\ {(\bar{k} + \alpha p_{0} )\beta \lambda_{i} ,} \hfill & {{\text{if}}\;{{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {(1 - \beta )}}} \right. \kern-0pt} {(1 - \beta )}} \le \lambda_{i} \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta }} \hfill \\ {\bar{k}\beta \lambda_{i} + \alpha p_{0} d_{c} ,} \hfill & {{\text{if}}\;\lambda_{i} \ge {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta }} \hfill \\ \end{array} ;} \right. $$

Case 2 If $d_{u} \le {{{{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta } \le d_{u} } \mathord{\left/ {\vphantom {{{{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta } \le d_{u} } {(1 - \beta )}}} \right. \kern-0pt} {(1 - \beta )}},$ then

$$ r_{i}^{f} (\alpha ,\beta \lambda_{i} ) = \left\{ {\begin{array}{*{20}l} {(\bar{k} - (1 - \alpha )p_{0} )\beta \lambda_{i} ,} \hfill & {{\text{if}}\;0 \le \lambda_{i} \le d_{u} } \hfill \\ (\bar{k} - (1 - \alpha )p_{0} )\beta \lambda_{i} & \\ \quad+ p_{0} (\lambda_{i} - d_{u} ), & {{\text{if}}\;d_{u} \le \lambda_{i} \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta }} \hfill \\ {}[p_{0} + (\bar{k} - p_{0} )\beta ]\lambda_{i} &\\ \quad + \alpha p_{0} d_{c} - p_{0} d_{u} , & {{\text{if}}\;{{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } \beta }} \right. \kern-0pt} \beta } \le \lambda_{i} \le {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {(1 - \beta )}}} \right. \kern-0pt} {(1 - \beta )}}} \hfill \\ {\bar{k}\beta \lambda_{i} + \alpha p_{0} d_{c} ,} \hfill & {{\text{if}}\;\lambda_{i} \ge {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {(1 - \beta )}}} \right. \kern-0pt} {(1 - \beta )}}} \hfill \\ \end{array} } \right.. $$

Recall that $p_{0} - \bar{k} \le \alpha p_{0} - k$, then $r_{i}^{f} (\alpha ,\beta \lambda_{i} )$ is the increasing in $\lambda_{i}$ on each piecewise interval. Further, $r_{i}^{f} (\alpha ,\beta \lambda_{i} )$ is continuous at the points of each piecewise interval. Thus, Lemma 1 holds.  □

Proof of Theorem 1

Under asymmetric information, we should consider both the IR and the IC constraints. Following the proof of Lemma 1, under the IR constraint the commission level $r_{i}^{f} (\alpha ,\beta \lambda_{i} )$ is non-decreasing in $\lambda_{i}$. The IC constraint can be written as $r_{i} (\alpha ,\beta \lambda_{i} ) \le r_{i} (\alpha ,\beta \lambda_{j} )$, $i,j = L{\text{ or }}H$, $i \ne j$. When $i = L,$ since $\lambda_{L} \le \lambda_{H} ,$ we have $r_{L} (\alpha ,\beta \lambda_{L} ) \le r_{L} (\alpha ,\beta \lambda_{H} )$, i.e., the commission level described by Lemma 1 satisfies the IC constraint. When $i = H$, to satisfy the IC constraint, the commission level should be no higher than $r_{H} (\alpha ,\beta \lambda_{1} )$ with $\lambda_{L} \le \lambda_{1} \le \lambda_{H}$. Hence, the optimal commission contracts under asymmetric information follow Eq. (8).  □

Proof of Theorem 2

Due to $d_{u} = A_{1} - p_{0} + \theta\,\alpha p_{0} ,$ then

$$ \pi_{uL}^{s} = p_{0} \hbox{min} \{ \lambda_{L} ,A_{1} - p_{0} + \theta \alpha p_{0} \} - \bar{k}\lambda_{L} $$

We discuss the optimal result through the following cases.

Case 1 $\lambda_{L} \ge A_{1} - p_{0} + \theta \alpha p_{0} ,$ i.e., $\alpha {{ \le (\lambda_{L} - A_{1} + p_{0} )} \mathord{\left/ {\vphantom {{ \le (\lambda_{L} - A_{1} + p_{0} )} {(\theta p_{0} }}} \right. \kern-0pt} {(\theta p_{0} }}).$

$$ \pi_{uL}^{s} = p_{0} (A_{1} - p_{0} + \theta \alpha p_{0} ) - \bar{k}\lambda_{L} , $$

which is increasing in $\alpha$ and achieves its maximal value at $\alpha {{ = (\lambda_{L} - A_{1} + p_{0} )} \mathord{\left/ {\vphantom {{ = (\lambda_{L} - A_{1} + p_{0} )} {(\theta p_{0} )}}} \right. \kern-0pt} {(\theta p_{0} )}}.$ When $\alpha {{ = (\lambda_{L} - A_{1} + p_{0} )} \mathord{\left/ {\vphantom {{ = (\lambda_{L} - A_{1} + p_{0} )} {(\theta p_{0} )}}} \right. \kern-0pt} {(\theta p_{0} )}},\,\pi_{uL}^{s} = (p_{0} - \bar{k})\lambda_{L} ,$ which can be incorporated into the following case $\lambda_{L} \le A_{1} - p_{0} + \theta \alpha p_{0} .$.

Case 2 $\lambda_{L} \le A_{1} - p_{0} + \theta \alpha p_{0} ,$ i.e., $\alpha {{ \ge (\lambda_{L} - A_{1} + p_{0} )} \mathord{\left/ {\vphantom {{ \ge (\lambda_{L} - A_{1} + p_{0} )} {(\theta p_{0} }}} \right. \kern-0pt} {(\theta p_{0} }}).$

$$ \pi_{uL}^{s} = (p_{0} - \bar{k})\lambda_{L} , $$

which is independent of $\alpha$ and $\beta .$ Recall the condition that $p_{0} - \bar{k} \le \alpha p_{0} - k,$ i.e., $\alpha \ge 1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }}.$

Consequently, combining Cases 1 and 2 we have

$$ \left\{ {\begin{array}{*{20}l} {\hbox{max}} \{ {{(\lambda_{L} - A_{1} + p_{0} )} \mathord{\left/ {\vphantom {{(\lambda_{L} - A_{1} + p_{0} )} {(\theta p_{0} }}} \right. \kern-0pt} {(\theta p_{0} }}),1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }},0\} \\ \qquad \le \alpha^{*} \le 1 , \quad {{\text{if }}{{\theta < (\lambda_{L} - A_{1} + p_{0} )} \mathord{\left/ {\vphantom {{\theta < (\lambda_{L} - A_{1} + p_{0} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }}} \hfill \\ {\alpha^{*} = 1,} \qquad\qquad {\text{otherwise}} \hfill \\ \end{array} } \right., $$

and $0 \le \beta^{*} \le 1$.

Thus, Theorem 2 holds.  □

Proof of Theorem 3

Suppose that $A_{i} - p_{0} \ge \lambda_{1} ,$ then $d_{u} \ge \lambda_{1} ,\,d_{c} \ge \lambda_{1} ,$

$$ \pi_{uH}^{s} = p_{0} \left[ {\hbox{min} \{ (1 - \beta )\lambda_{H} ,d_{u} \} - (1 - \beta )\lambda_{1} } \right] + \alpha p_{0} \left[ {\hbox{min} \{ \beta \lambda_{H} ,d_{c} \} - \beta \lambda_{1} } \right] + (\lambda_{H} - \lambda_{1} )\bar{k}\beta + C $$

with $C = p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} - \bar{k}\lambda_{H} .$

We discuss the optimal result through the following cases.

Case 1 $(1 - \beta )\lambda_{H} \le d_{u} ,$ i.e., $\beta \ge 1 - {{(A_{1} - p_{0} + \theta \alpha p_{0} )} \mathord{\left/ {\vphantom {{(A_{1} - p_{0} + \theta \alpha p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$

• (1) If $\beta \lambda_{H} \ge d_{c} ,$ i.e., $\beta \ge {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},$ then

  $$ \pi_{uH}^{s} = p_{0} (\lambda_{H} - \lambda_{1} ) - \left[ {\alpha p_{0} \lambda_{1} + (\lambda_{H} - \lambda_{1} )(p_{0} - \bar{k})} \right]\beta + \alpha p_{0} (A_{2} - \alpha p_{0} + \theta p_{0} ) + C, $$

  which is decreasing in $\beta$ and achieves its maximal value at $\beta = {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$ Hence, Case (1) can be incorporated into the following case $\beta \lambda_{H} \le d_{c} .$

• (2) If $\beta \lambda_{H} \le d_{c} ,$ i.e., $\beta \le {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},$ then it requires that ${{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }} \ge 1 - {{(A_{1} - p_{0} + \theta \alpha p_{0} )} \mathord{\left/ {\vphantom {{(A_{1} - p_{0} + \theta \alpha p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},$ i.e.,

  $$ \alpha \le {{(A_{1} + A_{2} + \theta p_{0} - p_{0} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{1} + A_{2} + \theta p_{0} - p_{0} - \lambda_{H} )} {[p_{0} (1 - \theta )]}}} \right. \kern-0pt} {[p_{0} (1 - \theta )]}} $$

  (14)

  In this case,

  $$ \pi_{uH}^{s} = p_{0} (\lambda_{H} - \lambda_{1} ) + (\lambda_{H} - \lambda_{1} )(\alpha p_{0} + \bar{k} - p_{0} )\beta + C. $$

  (15)

  Recall the condition that $p_{0} - \bar{k} \le \alpha p_{0} - k,$ i.e.,

  $$ \alpha \ge 1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }}, $$

  (16)

  hence $\pi_{uH}^{s}$ is increasing in $\beta .$ As a result,

• (1) If $1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }} \ge {{(A_{1} + A_{2} + \theta p_{0} - p_{0} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{1} + A_{2} + \theta p_{0} - p_{0} - \lambda_{H} )} {[p_{0} (1 - \theta )]}}} \right. \kern-0pt} {[p_{0} (1 - \theta )]}},$ then

  $$ (\alpha^{*} ,\beta^{*} ) = ({{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} {[p_{0} (1 - \theta )]}}} \right. \kern-0pt} {[p_{0} (1 - \theta )]}},{{[\lambda_{H} + (1 - \theta^{2} )p_{0} - \theta A_{2} - A_{1} ]} \mathord{\left/ {\vphantom {{[\lambda_{H} + (1 - \theta^{2} )p_{0} - \theta A_{2} - A_{1} ]} {[\lambda_{H} (1 - \theta )]}}} \right. \kern-0pt} {[\lambda_{H} (1 - \theta )]}}). $$

• (2) If $1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }} \le {{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} {[p_{0} (1 - \theta )]}}} \right. \kern-0pt} {[p_{0} (1 - \theta )]}},$ then

  $\beta^{*} = \hbox{min} \{ 1,{{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}\} .$ By substituting $\beta^{*} = {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}$ into the profit function (15), we have $\pi_{cH}^{s}$ is concave in $\alpha$ and achieves its maximal value when $\alpha = \alpha_{0} = {{(A_{2} + p_{0} + \theta p_{0} - \bar{k})} \mathord{\left/ {\vphantom {{(A_{2} + p_{0} + \theta p_{0} - \bar{k})} {(2p_{0} )}}} \right. \kern-0pt} {(2p_{0} )}}.$

  Recall the condition (14) and that $\alpha \le 1,$ then $\alpha^{*}$ should satisfy

  $$ \alpha^{*} \le \bar{\alpha } = \hbox{min} \left\{ {1,{{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} {[p_{0} (1 - \theta )]}}} \right. \kern-0pt} {[p_{0} (1 - \theta )]}}} \right\}. $$

  (17)

Recall the condition (16) and that $\beta^{*} = {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }} \le 1,$ then $\alpha^{*}$ should also satisfy

$$ \alpha^{*} \ge \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\alpha } = \hbox{max} \{ {{(A_{2} - \lambda_{H} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{H} + \theta p_{0} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }},1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }}\} . $$

(18)

As a result,

$$ (\alpha^{*} ,\beta^{*} ) = \left\{ {\begin{array}{*{20}l} {(\hbox{min} \{ \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\alpha } ,1\} ,1),} \hfill & {{\text{if }}\alpha_{0} \le \hbox{min} \{ \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\alpha } ,1\} } \hfill \\ {(\hbox{min} \{ \alpha_{0} ,\bar{\alpha }\} ,{{(A_{2} - \alpha^{*} p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha^{*} p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}),} \hfill & {\text{otherwise}} \hfill \\ \end{array} } \right., $$

with $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\alpha } = \hbox{max} \{ {{(A_{2} - \lambda_{H} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{H} + \theta p_{0} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }},1 - ({{\bar{k} - k)} \mathord{\left/ {\vphantom {{\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }}\} ,$ $\bar{\alpha } = \hbox{min} \{ 1,{{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{1} + A_{2} - p_{0} + \theta p_{0} - \lambda_{H} )} {[p_{0} (1 - \theta )]}}} \right. \kern-0pt} {[p_{0} (1 - \theta )]}}\}$ and $\alpha_{0} = {{(A_{2} + p_{0} + \theta p_{0} - \bar{k})} \mathord{\left/ {\vphantom {{(A_{2} + p_{0} + \theta p_{0} - \bar{k})} {(2p_{0} )}}} \right. \kern-0pt} {(2p_{0} )}}.$

Case 2 $(1 - \beta )\lambda_{H} \ge d_{u} ,$ i.e., $\beta \le 1 - {{(A_{1} - p_{0} + \theta \alpha p_{0} )} \mathord{\left/ {\vphantom {{(A_{1} - p_{0} + \theta \alpha p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$

• (1) If $\beta \lambda_{H} \le d_{c} ,$ i.e., $\beta \le {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},$ then

  $$ \pi_{uH}^{s} = p_{0} (A_{1} - p_{0} - \lambda_{1} + \theta \alpha p_{0} ) + [p_{0} \lambda_{1} + (\lambda_{H} - \lambda_{1} )(\alpha p_{0} + \bar{k})]\beta + C, $$

  which is increasing in $\beta ,$ and achieves its maximal value at $\beta \le {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$

Hence, this case can be incorporated into the following case $\beta \lambda_{H} \ge d_{c} .$

• (2) If $\beta \lambda_{H} \ge d_{c} ,$ i.e., $\beta \ge {{(A_{2} - \alpha p_{0} + \theta p_{0} )} \mathord{\left/ {\vphantom {{(A_{2} - \alpha p_{0} + \theta p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},$ then

$$ \begin{aligned} \pi_{uH}^{s} & = p_{0} (A_{1} - p_{0} - \lambda_{1} + \theta \alpha p_{0} ) + \alpha p_{0} (A_{2} - \alpha p_{0} + \theta p_{0} ) \\ & \quad + [(1 - \alpha )p_{0} \lambda_{1} + (\lambda_{H} - \lambda_{1} )\bar{k}]\beta + C, \\ \end{aligned} $$

which is increasing in $\beta$, and achieves its maximal value at $\beta^{ *} = 1 - {{(A_{1} - p_{0} + \theta \alpha p_{0} )} \mathord{\left/ {\vphantom {{(A_{1} - p_{0} + \theta \alpha p_{0} )} {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$

Hence, Case 2 can be incorporated into Case 1.

As a result, Theorem 3 holds.  □

Proof of Corollary 3

When there is no demand competition and the high-type user company outsources all his remaining resources to the service provider, i.e., $\theta = 0$ and $\beta = 1,$ the commission he should pay to the service provider satisfies

$$ r_{H}^{f*} (\alpha ,\lambda_{1} ) = \alpha p_{0} \hbox{min} \{ \lambda_{1} ,d_{c} \} - p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} + \bar{k}\lambda_{1} . $$

Hence, the profit function of the high-type user company when $\beta = 1$ is

$$ \pi_{uH}^{s} = \alpha p_{0} [\hbox{min} \{ \lambda_{H} ,d_{c} \} - \hbox{min} \{ \lambda_{1} ,d_{c} \} ] + p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} - \bar{k}\lambda_{1} . $$

Recall that $d_{u} = A_{1} - p_{0}$ and $d_{c} = A_{2} - \alpha p_{0} ,$ thus we have the following cases.

Case 1 If $\lambda_{H} \le d_{c} ,$ i.e., $\alpha \le {{(A_{2} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{H} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }},$ then $\pi_{uH}^{s} = \alpha p_{0} (\lambda_{H} - \lambda_{1} ) + p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} - \bar{k}\lambda_{1} ,$ which is increasing in $\alpha .$

Case 2 If $\lambda_{1} \ge d_{c} ,$ i.e., $\alpha \le {{(A_{2} - \lambda_{1} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{1} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }},$ then $\pi_{uH}^{s} = p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} - \bar{k}\lambda_{1} ,$ which is independent of $\alpha .$

Case 3 If $\lambda_{1} \le d_{c} \le \lambda_{H} ,$ i.e., ${{(A_{2} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{H} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }} \le \alpha \le {{(A_{2} - \lambda_{1} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{1} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }},$ then $\pi_{uH}^{s} = \alpha p_{0} [A_{2} - \alpha p_{0} - \lambda_{1} ] + p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} - \bar{k}\lambda_{1} ,$ which is concave in $\alpha$ and achieves its maximal value at $\alpha = {{(A_{2} - \lambda_{1} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{1} )} {(2p_{0} )}}} \right. \kern-0pt} {(2p_{0} )}}.$

Further, recall the condition (14), then we have

$$ \alpha^{*} = \left\{ {\begin{array}{*{20}l} {1 - {{(\bar{k} - k)} \mathord{\left/ {\vphantom {{(\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }},} \hfill & {{\text{if }}\alpha_{1} < 1 - {{(\bar{k} - k)} \mathord{\left/ {\vphantom {{(\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }}} \hfill \\ {\alpha_{1} ,} \hfill & {{\text{if }}1 - {{(\bar{k} - k)} \mathord{\left/ {\vphantom {{(\bar{k} - k)} {p_{0} }}} \right. \kern-0pt} {p_{0} }} \le \alpha_{1} < 1} \hfill \\ {1,} \hfill & {{\text{if }}\alpha_{1} \ge 1} \hfill \\ \end{array} } \right., $$

with $\alpha_{1} = \hbox{max} \{ {{(A_{2} - \lambda_{1} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{1} )} {(2p_{0} )}}} \right. \kern-0pt} {(2p_{0} )}},{{(A_{2} - \lambda_{H} )} \mathord{\left/ {\vphantom {{(A_{2} - \lambda_{H} )} {p_{0} }}} \right. \kern-0pt} {p_{0} }}\} .$ Thus, Corollary 3 holds.  □

Proof of Corollary 4

When $\alpha = 1$, we have $r_{i}^{f*} (\beta \lambda_{1} ) = p_{0} \hbox{min} \{ (1 - \beta )\lambda_{1} ,d_{u} \} + p_{0} \hbox{min} \{ \beta \lambda_{1} ,d_{c} \} - p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} + \bar{k}\beta \lambda_{1} ,$ with $d_{u} = A_{1} - p_{0} + \theta p_{0}$ and $d_{c} = A_{2} - p_{0} + \theta p_{0} .$ Hence, the profit of the user company satisfies

$$ \begin{aligned} \pi_{uH}^{s} & = p_{0} \left[ {\hbox{min} \{ (1 - \beta )\lambda_{H} ,d_{u} \} - \hbox{min} \{ (1 - \beta )\lambda_{1} ,d_{u} \} + \hbox{min} \{ \beta \lambda_{H} ,d_{c} \} - \hbox{min} \{ \beta \lambda_{1} ,d_{c} \} } \right] \\ & \quad + (\lambda_{H} - \lambda_{1} )\bar{k}\beta + C. \\ \end{aligned} $$

Suppose that ${{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }} + {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }} \ge 1,$ i.e., we discuss the optimal result with the following cases.

Case 1 $(1 - \beta )\lambda_{1} \ge d_{u}$ and $\beta \lambda_{H} \le d_{c} ,$ i.e., $\beta \le 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }}.$ In this case, $\pi_{uH}^{s} = (\lambda_{H} - \lambda_{1} )(p_{0} + \bar{k})\beta + C,$ which is increasing in $\beta ,$ thus $\beta^{*} = 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }}.$ Thus, Case 1 can be incorporated into the following Case 2.

Case 2 $(1 - \beta )\lambda_{H} \ge d_{u}$ and $(1 - \beta )\lambda_{1} \le d_{u} ,$ i.e., $1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }} \le \beta \le 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$

• (1) If $\beta \lambda_{H} \le d_{c} ,$ i.e., $\beta \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},$ then $\pi_{uH}^{s} = p_{0} (d_{u} - \lambda_{1} )\, + [(\lambda_{H} - \lambda_{1} )(p_{0} + \bar{k}) + p_{0} \lambda_{1} ]\beta + C,$ which is increasing in $\beta .$ Thus, this case can be incorporated into the following Case (2).

• (2) If $\beta \lambda_{H} \ge d_{c}$ and $\beta \lambda_{1} \le d_{c} ,$ i.e., ${{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }} \le \beta \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }}$ and $d_{u} + d_{c} \ge \lambda_{H} ,$ then $\pi_{uH}^{s} = p_{0} (d_{u} + d_{c} - \lambda_{1} ) + C + \bar{k}(\lambda_{H} - \lambda_{1} )\beta ,$ which is increasing in $\beta .$ Thus, this case can be incorporated into the following Case (3).

• (3) If $\beta \lambda_{1} \ge d_{c} ,$ i.e., $\beta \ge {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }},$ then $\pi_{uH}^{s} = p_{0} (d_{u} - \lambda_{1} )\, + [p_{0} \lambda_{1} + \bar{k}(\lambda_{H} - \lambda_{1} )]\beta + C,$ which is increasing in $\beta .$

Combining (1), (2) and (3), we have when $1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }} \le \beta \le 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},\,\beta^{*} = 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$ Hence, Case 2 can be incorporated into the following Case (3).

Case 3 $(1 - \beta )\lambda_{H} \le d_{u} ,$ i.e., $\beta \ge 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}.$

• (1) If $\beta \lambda_{H} \le d_{c} ,$ i.e., $\beta \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}$ and $d_{u} + d_{c} \ge \lambda_{H} ,$ then $\pi_{uH}^{s} = p_{0} (\lambda_{H} - \lambda_{1} ) + p_{0} \hbox{min} \{ \lambda_{1} ,d_{u} \} - \bar{k}\lambda_{H} \, + (\lambda_{H} - \lambda_{1} )\bar{k}\beta ,$ which is increasing in $\beta$. Hence, this case can be incorporated into the following Case (2).

• (2) If $\beta \lambda_{H} \ge d_{c}$ and $\beta \lambda_{1} \le d_{c} ,$ i.e., ${{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }} \le \beta \le {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }}$ and $d_{u} + d_{c} \ge \lambda_{H} ,$ then $\pi_{uH}^{s} = p_{0} (\lambda_{H} - \lambda_{1} ) + C\, + p_{0} d_{c} - [(\lambda_{H} - \lambda_{1} )(p_{0} - \bar{k}) + p_{0} \lambda_{1} ]\beta ,$ which is decreasing in $\beta ,$ thus $\beta^{*} = {{\hbox{min} \{ 1,d_{c} } \mathord{\left/ {\vphantom {{\hbox{min} \{ 1,d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}\}$ if $d_{u} + d_{c} \ge \lambda_{H} .$

• (3) If $\beta \lambda_{1} \ge d_{c} ,$ i.e., $\beta \ge {{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{1} }}} \right. \kern-0pt} {\lambda_{1} }},$ then $\pi_{uH}^{s} = p_{0} (\lambda_{H} - \lambda_{1} ) - (p_{0} - \bar{k})(\lambda_{H} - \lambda_{1} )\beta + C,$ which is decreasing in $\beta ,$ thus this case can be incorporated into the above Case (2). As a result, we can obtain that, if

  $$ \beta \ge 1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},\,\beta^{*} = \left\{ {\begin{array}{*{20}l} {\hbox{min} \{ 1,{{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}\} ,} \hfill & {{\text{if }}d_{u} + d_{c} \ge \lambda_{H} } \hfill \\ {1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},} \hfill & {\text{otherwise}} \hfill \\ \end{array} } \right.. $$

Consequently, combining Cases 1, 2 and 3 we have

$\beta^{*} = \left\{ {\begin{array}{*{20}l} {\hbox{min} \{ 1,{{d_{c} } \mathord{\left/ {\vphantom {{d_{c} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }}\} ,} \hfill & {{\text{if }}d_{u} + d_{c} \ge \lambda_{H} } \hfill \\ {1 - {{d_{u} } \mathord{\left/ {\vphantom {{d_{u} } {\lambda_{H} }}} \right. \kern-0pt} {\lambda_{H} }},} \hfill & {\text{otherwise}} \hfill \\ \end{array} } \right..$ Thus, Corollary 4 holds.  □