1 Introduction

Contemporary engineered materials, including composites, concrete, bio tissues, and foams, commonly exhibit susceptibility to heat and moisture due to their porous, fractured, and atomic structure. Piezoelectric materials are a category of intelligent materials that hold promise for enhancing network performance by employing active dampening, stiffening, and vibration mitigation [1]. However, thermal stresses caused by aerodynamic heating, heat transfer, and moisture transfer can lead to buckling, instability, and decreased stiffness and strength in these materials over their service life. As the temperature increases, fluid can be absorbed into the perforations, enlarging voids and tiny flaws and causing defects to expand. Conversely, when the temperature is lowered, trapped fluid can further broaden defects, resulting in potential system failure. Hence, the inclusion of the impact of hygro-thermal, highlighting the interplay between moisture and temperature, is crucial when scrutinizing the mechanical characteristics of these materials and structures[2]. Alhashash et al. [3] have presented a novel approach to understanding the behavior of semiconductor materials under the reference of the two-temperature hygro-thermo-elasticity theory. Alenazi et al. [4]conducted research on the influence of moisture diffusivity in a semiconductor material within the framework of photo-thermoelasticity theory. El-Sapa et al. [5] delved into the study of moisture impact on the free surface of a semiconductor material. Their investigation utilized a one-dimensional deformation model to explore these interactions.

Piezoelectric materials are now widely used in many fields, such as medical imaging, energy harvesting, and vibration control. The term “piezoelectricity” describes the capability of certain crystals to generate a voltage when they are mechanically stressed, which can be used for various applications. The Curie brothers [6] were the first to discover this phenomenon, and their work laid the foundation for developing modern piezoelectric materials. Mindlin [7] developed an equation for the relationship between temperature and piezoelectric materials. The fundamental laws that govern thermo-piezo-elasticmaterials were explored by Nowacki [8, 9].In recent decades, there has been substantial progress made by researchers and engineers in enhancing the properties of piezoelectric materials, hence leading to an expansion of their potential uses. Numerous researchers [10,11,12,13] have conducted extensive research on wave propagation in different types of thermoelastic materials with piezoelectricity, diffusion, memory, and the two-temperature effect. Their findings have contributed significantly to understanding the behavior of plane waves in these mediums and finding practical applications in various fields, such as material engineering and acoustics. The energy transmission methods across several contact types, including elastic, thermo-elastic, and thermo-piezo-electric, have been thoroughly studied by Gupta and his team [14,15,16,17,18,19,20,21,22]. They have examined how numerous elements, including electric fields, phase delays, and memory derivatives, affect these energy transfer processes. This research holds significance in advancing the comprehension of interface behavior and in the development of novel applications in the realms of energy harvesting and vibration control. Gupta and his research team [23,24,25] conducted an in-depth investigation into diverse wave phenomena within a porous fiber-reinforced thermoelastic medium. Their study considered the influence of memory effects, magnetic fields, and nonlocality on the observed phenomena. Further research is necessary to understand how piezoelectric composite materials perform in hygro-thermal environments.

Hygro-piezo-thermo-elastic (HPTE) materials are of significant importance as they offer a diverse range of properties that can be customized to cater to specific engineering needs. The combination of mechanical, electrical, and hygro-thermal features makes these materials capable of actively reducing vibrations, enhancing stiffness and strength, and adapting to variations in temperature and humidity. Due to these exceptional characteristics, they have immense potential for applications in diverse fields, including aerospace, robotics, and environmental monitoring, where precise control over material properties is critical. Using the virtual work idea, Raja et al. [26] have demonstrated how active stiffness affects the dynamic behavior of HPTE laminates. Gupta et al. [27] carried out an extensive review, examining the impacts of hygro-thermal conditions and additional non-linear influences on the mathematical formulation of smart structures incorporating piezoelectric elements. This review provides valuable insights into the challenges and opportunities in developing more advanced and robust models for piezoelectric intelligent systems. Zenkour and Alghanmi [28] investigated the behavior of sandwich plates under electro-mechanical-hygro-thermo sinusoidal loadings using piezoelectric material faces and a functionally graded core.

Tiwari and her research team [29,30,31,32,33,34,35] conducted an in-depth investigation into wave propagation, examining various scenarios, including continuous line heat sources, magneto-thermoelasticity, and memory-dependent derivatives. Their research yielded valuable insights into material behaviors under specific conditions, with implications for applications ranging from thermal management to high-temperature environments. Through the integration of memory-dependent phenomena and nonlocal effects, Tiwari’s work contributes to the advancement of theoretical frameworks, offering support for material design and optimization in diverse technological domains. Kaur’s research group [36,37,38,39] has significantly advanced the understanding of thermoelastic damping and dynamic responses in nanobeams, primarily focused on generalized piezothermoelastic nanobeams with simply supported boundaries. Additionally, they examined the magneto-electro-piezo-thermoelastic nanobeam with two temperatures subjected to ramp-type heating, revealing insights into the behavior of nanobeam structures under the influence of magneto-electro-piezo-thermoelastic effects during ramp-type heating scenarios.

Piezoelectric materials are widely employed in diverse real-world applications, yet their behavior at the free surface of the hygro-thermo-piezo-elastic medium remains inadequately understood. The objective of this study is to develop an innovative mathematical model to analyze the behavior of plane waves in piezo-thermo-elastic materials. Additionally, the study aims to investigate the influence of moisture and temperature diffusivities, as well as moisture content, on the distribution of physical properties within the thermo-piezo-elasticmaterial. This study provides a deeper understanding of these materials’ behavior, aiding in optimizing their design for practical applications.

2 Fundamental Equations of Hygro-thermo-piezo-elastic Medium

Following Yadav et al. [40], Barak and Gupta [41], Szekeres [42, 43], and Hosseini and Ghadiri [44],the total moisture flux \(f_{i}\) and heat flux \(q_{i}\) and the basic relationship for a coupled anisotropic hygro-thermo-piezo-elasticmedium are:

$$q_{i} = - D_{ij}^{T} T,_{i} - q_{ij}^{m} m,_{i} ,$$
(1)
$$f_{i} = - D_{ij}^{m} m,_{i} - Q_{ij}^{T} T,_{i} .$$
(2)

The hygro-piezoelectric medium facilitates the transmission of elastic, moisture, electro-magnetic, and thermal waves. The constitutive equations pertaining to this medium are stated in the following manner:

$$D_{i} = \eta_{ijk} e_{jk} + \varepsilon_{ij} E_{j} + \tau_{i} T + d_{i} m,E_{i} = - \phi ,_{i}$$
(3)
$$\rho T_{0} \dot{S} = - q_{i} ,_{i} ,\rho T_{0} S = \frac{{\beta_{ij}^{T} T_{0} }}{{\rho C_{E} }}e_{kk} + T - T_{0} \tau_{i} \phi ,_{i} ,$$
(4)
$$- q_{i} ,_{i} = D_{ij}^{T} T,_{ii} + q_{ij}^{m} m,_{ii} ,$$
(5)
$$\left. {\begin{array}{*{20}c} {\dot{J} = - f_{i} ,_{i} \,,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {J = m + \frac{{\beta_{ij}^{m} D_{ij}^{m} m_{0} }}{{K_{m} }}e_{kk} - m_{0} d_{i} \phi ,_{i} } \\ \end{array} } \right\},$$
(6)
$$- f_{i} ,_{i} = D_{ij}^{m} m,_{ii} + Q_{ij}^{T} T,_{ii} ,$$
(7)
$$\sigma_{ij} = c_{ijkl} e_{kl} - \eta_{ijk} E_{k} + \beta_{ij}^{T} T + \beta_{ij}^{m} m,\,$$
(8)
$$\left. {\begin{array}{*{20}l} {\sigma_{11} = c_{11} u_{1} ,_{1} + c_{12} u_{2} ,_{2} + c_{13} u_{3} ,_{3} - \beta_{1}^{T} T - \beta_{1}^{m} m + \eta_{31`} \phi ,_{3} \,,} \hfill \\ {\sigma_{12} = c_{66} \left( {u_{1} ,_{2} + u_{2} ,_{1} } \right)\,,} \hfill \\ {\sigma_{13} = c_{55} \left( {u_{1} ,_{3} + u_{3} ,_{1} } \right) + \eta_{15} \phi ,_{1} \,,} \hfill \\ {\sigma_{22} = c_{12} u_{1} ,_{1} + c_{22} u_{2} ,_{2} + c_{23} u_{3} ,_{3} - \beta_{2}^{T} T - \beta_{2}^{m} m + \eta_{32`} \phi ,_{3} \,,} \hfill \\ {\sigma_{23} = c_{44} \left( {u_{2} ,_{3} + u_{3} ,_{2} } \right)\, + \eta_{24} \phi ,_{2} ,} \hfill \\ {\sigma_{33} = c_{13} u_{1} ,_{1} + c_{23} u_{2} ,_{2} + c_{33} u_{3} ,_{3} - \beta_{3}^{T} T - \beta_{3}^{m} m + \eta_{33`} \phi ,_{3} \,,} \hfill \\ \end{array} } \right\}$$
(9)
$$\left. {\begin{array}{*{20}l} {D_{1} = \eta_{15} \,\left( {u_{1} ,_{3} + u_{3} ,_{1} } \right)\, - \varepsilon_{11} \phi ,_{1} \,,\,\,} \hfill \\ {D_{2} = \eta_{24} \,\left( {u_{2} ,_{3} + u_{3} ,_{2} } \right) - \varepsilon_{22} \phi ,_{2} } \hfill \\ {D_{3} = \eta_{31} u_{1} ,_{1} + \eta_{32} u_{2} ,_{2} + \eta_{33} u_{3} ,_{3} - \varepsilon_{33} \phi ,_{3} + \tau_{3} T + d_{3} m\,.} \hfill \\ \end{array} } \right\}$$
(10)

Equation of motion:

$$\sigma_{ji} ,_{j} = \rho \ddot{u}_{i} .$$
(11)

The following is the outcome of Eqs. (1), (4), and (5):

$$D_{i}^{T} T,_{ii} + q_{i}^{m} m,_{ii} - \dot{T} - \frac{{\beta_{i}^{T} T_{0} }}{{\rho C_{E} }}\dot{u}_{j} ,_{j} + T_{0} \tau_{i} \dot{\phi },_{i} = 0.$$
(12)

The following is the outcome of Eqs. (2), (6), and (7):

$$D_{i}^{m} m,_{ii} + Q_{i}^{T} T,_{ii} - \dot{m} - \frac{{\beta_{i}^{m} D_{i}^{m} m_{0} }}{{K_{m} }}\dot{u}_{j} ,_{j} + m_{0} d_{i} \dot{\phi },_{i} = 0.$$
(13)

Equation (3) may be written as follows:

$$D_{i} ,_{i} = 0\,\, \Rightarrow \,\,D_{1} ,_{1} + D_{2} ,_{2} + D_{3} ,_{3} = 0,$$
(14)

where,\(\,\beta_{ij}^{T} = \beta_{i}^{T} \delta_{ij} ,\,\,\)\(\beta_{ij}^{m} = \beta_{i}^{m} \delta_{ij} ,\,\,\)\(D_{ij}^{T} = D_{i}^{T} \delta_{ij} \,,\)\(D_{ij}^{m} = D_{i}^{m} \delta_{ij} \,,\)\(q_{ij}^{m} = q_{i}^{m} \delta_{ij}\),\(Q_{ij}^{T} = Q_{i}^{T} \delta_{ij} \,,\) and, \(i,\,j\) is not summed.

3 Modeling of the Problem

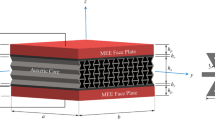
We assume a homogeneous orthotropic hygro-thermo-piezo-elastic half-space, and we adopt a Cartesian coordinate system \(\left( {x,\,y,\,z} \right)\). The half-space is characterized by uniform initial temperature \(T_{0}\) and moisture \(m_{0}\), as shown in Fig. 1. The propagation of hygro-thermal-elastic waves occurs within the \(xz\) plane, with all physical field variables function of \(t\), \(x,\) and \(z\).

Fig. 1
figure 1

Schematic representation of the problem

Full size image

The set of Eqs. (9)–(14) represents the governing equation that can be mathematically described in the \(xz -\) plane as:

$$c_{11} \frac{{\partial^{2} u}}{{\partial x^{2} }} + c_{55} \frac{{\partial^{2} u}}{{\partial z^{2} }} + \left( {c_{13} + c_{55} } \right)\frac{{\partial^{2} w}}{\partial x\partial z} + \left( {\eta_{31} + \eta_{15} } \right)\frac{{\partial^{2} \phi }}{\partial x\partial z} - \beta_{1}^{T} \frac{\partial T}{{\partial x}} - \beta_{1}^{m} \frac{\partial m}{{\partial x}} = \rho \ddot{u}\,,$$
(15)
$$\left( {c_{13} + c_{55} } \right)\frac{{\partial^{2} u}}{\partial x\partial z} + c_{55} \frac{{\partial^{2} w}}{{\partial x^{2} }} + c_{33} \frac{{\partial^{2} w}}{{\partial z^{2} }} + \eta_{15} \frac{{\partial^{2} \phi }}{{\partial x^{2} }} + \eta_{33} \frac{{\partial^{2} \phi }}{{\partial z^{2} }} - \beta_{3}^{T} \frac{\partial T}{{\partial z}} - \beta_{3}^{m} \frac{\partial m}{{\partial z}} = \rho \ddot{w}\,,$$
(16)
$$D_{1}^{T} \frac{{\partial^{2} T}}{{\partial x^{2} }} + D_{3}^{T} \frac{{\partial^{2} T}}{{\partial z^{2} }} + q_{1}^{m} \frac{{\partial^{2} m}}{{\partial x^{2} }} + q_{3}^{m} \frac{{\partial^{2} m}}{{\partial z^{2} }} - \frac{\partial T}{{\partial t}} - \frac{{T_{0} }}{{\rho C_{E} }}\left( {\beta_{1}^{T} \frac{{\partial^{2} u}}{\partial x\partial t} + \beta_{3}^{T} \frac{{\partial^{2} w}}{\partial z\partial t}} \right) + T_{0} \tau_{3} \frac{{\partial^{2} \phi }}{\partial z\partial t} = 0\,,$$
(17)
$$Q_{1}^{T} \frac{{\partial^{2} T}}{{\partial x^{2} }} + Q_{3}^{T} \frac{{\partial^{2} T}}{{\partial z^{2} }} + D_{1}^{m} \frac{{\partial^{2} m}}{{\partial x^{2} }} + D_{3}^{m} \frac{{\partial^{2} m}}{{\partial z^{2} }} - \frac{\partial m}{{\partial t}} - \frac{{m_{0} }}{{K_{m} }}\left( {\beta_{1}^{T} D_{1}^{m} \frac{{\partial^{2} u}}{\partial x\partial t} + \beta_{3}^{T} D_{3}^{m} \frac{{\partial^{2} w}}{\partial z\partial t}} \right) + m_{0} d_{3} \frac{{\partial^{2} \phi }}{\partial z\partial t} = 0\,,$$
(18)
$$\left( {\eta_{15} + \eta_{31} } \right)\frac{{\partial^{2} u}}{\partial x\partial z} + \eta_{15} \frac{{\partial^{2} w}}{{\partial x^{2} }} + \eta_{33} \frac{{\partial^{2} w}}{{\partial z^{2} }} - \varepsilon_{11} \frac{{\partial^{2} \phi }}{{\partial x^{2} }} - \varepsilon_{33} \frac{{\partial^{2} \phi }}{{\partial z^{2} }} + \tau_{3} \frac{\partial T}{{\partial z}} + d_{3} \frac{\partial m}{{\partial z}} = 0,$$
(19)

where, \(\vec{u} = \left( {u,\,0,\,w} \right)\), \(\beta_{1}^{T} = \left( {c_{11} + c_{13} } \right)\alpha_{11}^{T} + c_{13} \alpha_{11}^{T}\), \(\beta_{3}^{T} = 2c_{13} \alpha_{33}^{T} + c_{33} \alpha_{33}^{T}\), \(\beta_{1}^{m} = \left( {c_{11} + c_{13} } \right)\alpha_{11}^{m}\)\(+ c_{13} \alpha_{11}^{m}\), \(\beta_{3}^{m} = 2c_{13} \alpha_{33}^{m} + c_{33} \alpha_{33}^{m}\), the constants \(\alpha_{11}^{m} ,\,\alpha_{33}^{m}\) for the moisture expansion and \(\alpha_{11}^{T} ,\,\alpha_{33}^{T}\) for the linear thermal expansion.

4 Solution of the Problem

The technique of normal mode analysis has broad applications across several disciplines. It enables the calculation of solutions without imposing preconceptions on the studied variables, such as horizontal and vertical displacements, electric potential, temperature, and moisture. For the normal mode approach, the solution for these variables can be broken down as follows [45]:

$$\left( {u,\,w,\,\phi ,\,T,\,m} \right)\left( {x,z,t} \right) = \left( {u^{\prime},\,w^{\prime},\,\phi^{\prime},\,T^{\prime},\,m^{\prime}} \right)(z)\exp \left[ {ik\left( {x - ct} \right)} \right],$$
(20)

where,\(\omega = kc\) be the frequency,\(k\) be the wave number in the \(x -\) direction and \(u^{\prime}\), \(w^{\prime}\), \(\phi^{\prime}\)\(T^{\prime}\), and \(m^{\prime}\) are the function of \(z\) only,

Equations (15) through (19) and Eq. (20) together produce a homogeneous set of equations in five unknowns \(u^{\prime}\), \(w^{\prime}\), \(\phi^{\prime}\), \(T^{\prime}\), \(m^{\prime}\) as

$$\Omega R = 0$$
(21)

where, \(\Omega = \left[ {\begin{array}{*{20}c} {a_{11} + c_{55} D^{2} } & { - a_{12} D} & { - a_{13} D} & {a_{14} } & {a_{15} } \\ {a_{21} D} & {a_{22} - c_{33} D^{2} } & {a_{23} - \eta_{33} D^{2} } & {\beta_{3}^{T} D} & {\beta_{3}^{m} D} \\ {a_{31} } & {a_{32} D} & { - a_{33} D} & {a_{34} - D_{3}^{T} D^{2} } & {a_{35} - q_{3}^{m} D^{2} } \\ {a_{41} } & {a_{42} D} & { - a_{43} D} & {a_{44} - Q_{3}^{T} D^{2} } & {a_{45} - D_{3}^{m} D^{2} } \\ {a_{51} D} & {a_{52} - \eta_{33} D^{2} } & {\varepsilon_{33} D^{2} - a_{53} } & { - \tau_{3} D} & { - d_{3} D} \\ \end{array} } \right]\), \(R = \left[ {\begin{array}{*{20}c} {u^{\prime}} \\ {w^{\prime}} \\ {\phi^{\prime}} \\ {T^{\prime}} \\ {m^{\prime}} \\ \end{array} } \right]\), and \(a_{11} = \rho \omega^{2} - c_{11} k^{2}\), \(a_{12} = - \left( {c_{13} + c_{55} } \right)\iota k\), \(a_{13} = - \left( {\eta_{31} + \eta_{15} } \right)\iota k\), \(a_{14} = - \beta_{1}^{T} \iota k\), \(a_{15} = - \beta_{1}^{m} \iota k\), \(a_{21} = - \left( {c_{13} + c_{55} } \right)\iota k\), \(a_{22} = c_{55} k^{2} - \rho \omega^{2}\), \(a_{23} = \eta_{15} k^{2}\), \(a_{31} = - \frac{{T_{0} \beta_{1}^{T} k\omega }}{{\rho C_{e} }}\), \(a_{32} = \frac{{T_{0} \beta_{3}^{T} \iota \omega }}{{\rho C_{e} }}\), \(a_{33} = T_{0} \tau_{3} \iota \omega\), \(a_{34} = k^{2} D_{1}^{T} + \iota \omega\), \(a_{35} = k^{2} q_{1}^{m}\), \(a_{41} = - \frac{{m_{0} \beta_{1}^{T} D_{1}^{m} k\omega }}{{K_{m} }}\), \(a_{42} = \frac{{m_{0} \beta_{3}^{T} D_{3}^{m} \iota \omega }}{{K_{m} }}\), \(a_{43} = m_{0} d_{3} \iota \omega\), \(a_{44} = Q_{1}^{T} k^{2}\), \(a_{41} = \frac{{m_{0} \beta_{1}^{T} D_{1}^{m} k\omega }}{{K_{m} }}\), \(a_{51} = - \left( {\eta_{15} + \eta_{31} } \right)\iota k\), \(a_{52} = \eta_{15} k^{2}\), \(a_{53} = \varepsilon_{11} k^{2}\)\(D = {\partial \mathord{\left/ {\vphantom {\partial {\partial z}}} \right. \kern-0pt} {\partial z}}\).

The characteristic equation is obtained by nullifying the determinant of the matrix presented in Eq. (21) to find a non-trivial solution.

$$\left( {B_{0} D^{10} + B_{1} D^{8} + B_{2} D^{6} + B_{3} D^{4} + B_{4} D^{2} + B_{5} } \right)\left\{ {\phi^{\prime}(z),\,T^{\prime}(z)\,,u^{\prime}(z),\,w^{\prime}(z),\,m^{\prime}(z)} \right\} = 0$$
(22)

the coefficients \(B_{i} ,\,i = 1 - 5\) are provided in the Appendix.

The factorization of Eq. (22) may be expressed as.

$$\prod\limits_{j = 1}^{5} {\left( {D^{2} - \lambda_{j}^{2} } \right)} \left\{ {\phi^{\prime}(z),\,T^{\prime}(z)\,,u^{\prime}(z),\,w^{\prime}(z),\,m^{\prime}(z)} \right\} = 0.$$
(23)

The solutions of Eq. (23) are bounded as \(z \to \infty\) and given as follows:

$$\left( {u^{\prime},\,w^{\prime},\,\phi^{\prime},\,T^{\prime},m^{\prime}} \right) = \sum\limits_{n = 1}^{5} {\left( {M_{n} ,\,L_{1n} M_{n} ,\,L_{2n} M_{n} ,\,L_{3n} M_{n} ,\,\,L_{4n} M_{n} } \right)\exp \left( { - \lambda_{n} z} \right)} ,$$
(24)

where, \(\left[ {\begin{array}{*{20}c} {L_{1n} } \\ {L_{2n} } \\ {L_{3n} } \\ {L_{4n} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {a_{12} \lambda_{n} } & {a_{13} \lambda_{n} } & {a_{14} } & {a_{15} } \\ {a_{22} - c_{33} \lambda_{n}^{2} } & {a_{23} - \eta_{33} \lambda_{n}^{2} } & { - \beta_{3}^{T} \lambda_{n} } & { - \beta_{3}^{m} \lambda_{n} } \\ {a_{32} \lambda_{n} } & { - a_{33} \lambda_{n} } & { - a_{34} + D_{3}^{T} \lambda_{n}^{2} } & { - a_{35} + q_{3}^{m} \lambda_{n}^{2} } \\ {a_{42} \lambda_{n} } & { - a_{43} \lambda_{n} } & { - a_{44} + Q_{3}^{T} \lambda_{n}^{2} } & { - a_{45} + D_{3}^{m} \lambda_{n}^{2} } \\ \end{array} } \right]^{ - 1} \left[ {\begin{array}{*{20}c} { - a_{11} - c_{55} \lambda_{n}^{2} } \\ {\lambda_{n} a_{21} } \\ {a_{31} } \\ {a_{41} } \\ \end{array} } \right]\) and \(\lambda_{n}^{2} ,\,\left( {n = 1 - \,5} \right)\) are the solutions of the characteristics Eq. (23).

By using Eq. (24) in Eqs. (9)-(10) in the \(xz\) plane and applying the normal mode approach yields the subsequent outcomes:

$$\left( {\sigma_{xx} ,\,\sigma_{zz} ,\,\sigma_{xz} ,\,D_{x} ,\,D_{z} } \right) = \sum\limits_{n = 1}^{5} {\left( {L_{5n} ,\,L_{6n} ,\,L_{7n} ,\,L_{8n} ,\,\,L_{9n} } \right)\exp ( - \lambda_{n} x_{3} )} .$$
(25)

5 Boundary Conditions

The free surface (z = 0) of the considered model is subjected to mechanical load and thermal shock. Following Dutta et al. [46], the subsequent boundary conditions aid in solving the governing equations within a half-space domain \(\left( {x,\,y,\,z \ge 0} \right)\).

Mechanical boundary conditions:

$$\sigma_{zz} \left( {x,\,0,\,t} \right) = P_{1} \left( {x,\,t} \right) = - P_{1}^{*} \exp \left\{ {\iota k\left( {x - ct} \right)} \right\},\sigma_{xz} \left( {x,\,0,\,t} \right) = 0$$
(26)

Thermal boundary condition:

$$T\left( {x,\,0,\,t} \right) = P_{2} \left( {x,\,t} \right) = P_{2}^{*} \exp \left\{ {\iota k\left( {x - ct} \right)} \right\},$$
(27)

where, \(P_{1} \left( {x,\,t} \right)\) and \(P_{2} \left( {x,\,t} \right)\) indicated the arbitrary functions of \(\left( {x,\,t} \right)\), \(P_{1}^{*}\), \(P_{2}^{*}\) are constant.

Electrical boundary condition:

$$\phi ,_{z} \left( {x,\,0,\,t} \right) = 0.$$
(28)

Electrical displacement boundary condition:

$$D,_{z} \left( {x,\,0,\,t} \right) = 0.$$
(29)

Upon substitution of the postulated solutions into the prescribed boundary conditions (26)–(29), the coefficients \(M_{n} \left( {n = 1 - 5} \right)\), yield

$$\sum\limits_{n = 1}^{5} {L_{6n} M_{n} } = - P_{1}^{*} ,$$
(30)
$$\sum\limits_{n = 1}^{5} {L_{7n} M_{n} } = 0,$$
(31)
$$\sum\limits_{n = 1}^{5} {L_{3n} M_{n} } = P_{2}^{*} ,$$
(32)
$$\sum\limits_{n = 1}^{5} {\lambda_{n} L_{2n} M_{n} } = 0,$$
(33)
$$\sum\limits_{n = 1}^{5} {\lambda_{n} L_{9n} M_{n} } = 0,$$
(34)

where,\(L_{5n} = c_{11} \iota k - c_{13} \lambda_{n} L_{1n} - \eta_{31} \lambda_{n} L_{2n} - \beta_{1}^{T} L_{3n} - \beta_{1}^{m} L_{4n}\), \(L_{7n} = - c_{55} \left( {\lambda_{n} - \iota kL_{1n} } \right) + \eta_{15} \iota kL_{2n}\).

\(L_{6n} = c_{13} \iota k - c_{33} \lambda_{n} L_{1n} - \eta_{33} \lambda_{n} L_{2n} - \beta_{3}^{T} L_{3n} - \beta_{3}^{m} L_{4n}\), \(L_{8n} = - \eta_{15} \left( {\lambda_{n} - \iota kL_{1n} } \right) - \varepsilon_{11} \iota kL_{2n}\).

\(L_{9n} = \eta_{31} \iota k - \eta_{33} \lambda_{n} L_{1n} + \varepsilon_{33} \lambda_{n} L_{2n} + \tau_{3} L_{3n} + d_{3} L_{4n}\).

To obtain the value of \(M_{n} \,\left( {n = 1 - 5} \right)\), the Eqs. (30) to (34) can be solved using the inverse matrix method, which can be carried out as follows:

$$\left[ {\begin{array}{*{20}c} {M_{1} } \\ {M_{2} } \\ {M_{3} } \\ {M_{4} } \\ {M_{5} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {L_{61} } & {L_{62} } & {L_{63} } & {L_{64} } & {L_{65} } \\ {L_{71} } & {L_{72} } & {L_{73} } & {L_{74} } & {L_{75} } \\ {L_{31} } & {L_{32} } & {L_{33} } & {L_{34} } & {L_{35} } \\ {\lambda_{1} L_{21} } & {\lambda_{2} L_{22} } & {\lambda_{3} L_{23} } & {\lambda_{4} L_{24} } & {\lambda_{5} L_{25} } \\ {\lambda_{1} L_{91} } & {\lambda_{2} L_{92} } & {\lambda_{3} L_{93} } & {\lambda_{4} L_{94} } & {\lambda_{5} L_{95} } \\ \end{array} } \right]^{ - 1} \left[ {\begin{array}{*{20}c} { - P_{1}^{*} } \\ 0 \\ {P_{2}^{*} } \\ 0 \\ 0 \\ \end{array} } \right]$$
(35)

This approach enables the derivation of precise analytical expressions for the diverse components of the displacement vector, electric displacement, stresses, temperature, and electric potential.

6 Validation

Considering isotropy and neglecting piezo and pyro-electric effects, we have

\(c_{11} = \lambda + 2\mu\), \(c_{33} = \lambda + 2\mu\), \(c_{13} = \lambda\), \(c_{55} = \mu\), \(\beta_{1}^{T} = \beta_{3}^{T} = \beta^{T}\), \(\beta_{1}^{m} = \beta_{3}^{m} = \beta^{m}\), \(D_{1}^{T} = D_{3}^{T} = D^{T}\), \(D_{1}^{m} = D_{3}^{m} = D^{m}\), \(q_{1}^{m} = q_{3}^{m} = D_{T}^{m}\), \(Q_{1}^{T} = Q_{3}^{T} = D_{m}^{T}\), \(d_{3} = 0\), \(\tau_{3} = 0\), \(\varepsilon_{11} = \varepsilon_{33} = 0\), \(\eta_{31} = \eta_{33} = \eta_{15} = 0\), the above model transforms into a thermo-hygro-elastic model as developed by Yadav et al. [40].

The fundamental Eqs. (15) to (19) regulating the system may be simplified as

$$\left( {\lambda + 2\mu } \right)\frac{{\partial^{2} u}}{{\partial x^{2} }} + \mu \frac{{\partial^{2} u}}{{\partial z^{2} }} + \left( {\left( {\lambda + \mu } \right)} \right)\frac{{\partial^{2} w}}{\partial x\partial z} + - \beta^{T} \frac{\partial T}{{\partial x}} - \beta^{m} \frac{\partial m}{{\partial x}} = \rho \ddot{u}\,,$$
(36)
$$\left( {\lambda + \mu } \right)\frac{{\partial^{2} u}}{\partial x\partial z} + \mu \frac{{\partial^{2} w}}{{\partial x^{2} }} + \left( {\lambda + 2\mu } \right)\frac{{\partial^{2} w}}{{\partial z^{2} }} + - \beta^{T} \frac{\partial T}{{\partial z}} - \beta^{m} \frac{\partial m}{{\partial z}} = \rho \ddot{w}\,,$$
(37)
$$D^{T} \left( {\frac{{\partial^{2} T}}{{\partial x^{2} }} + \frac{{\partial^{2} T}}{{\partial z^{2} }}} \right) + D_{T}^{m} \left( {\frac{{\partial^{2} m}}{{\partial x^{2} }} + \frac{{\partial^{2} m}}{{\partial z^{2} }}} \right) - \frac{\partial T}{{\partial t}} - \frac{{\beta^{T} T_{0} }}{{\rho C_{E} }}\left( {\frac{{\partial^{2} u}}{\partial x\partial t} + \frac{{\partial^{2} w}}{\partial z\partial t}} \right) = 0\,,$$
(38)
$$D_{m}^{T} \left( {\frac{{\partial^{2} T}}{{\partial x^{2} }} + \frac{{\partial^{2} T}}{{\partial z^{2} }}} \right) + D^{m} \left( {\frac{{\partial^{2} m}}{{\partial x^{2} }} + \frac{{\partial^{2} m}}{{\partial z^{2} }}} \right) - \frac{\partial m}{{\partial t}} - \frac{{\beta^{m} m_{0} D^{m} }}{{K_{m} }}\left( {\frac{{\partial^{2} u}}{\partial x\partial t} + \frac{{\partial^{2} w}}{\partial z\partial t}} \right) = 0\,.$$
(39)

7 Numerical Results and Discussion

A numerical experiment has been conducted using MATLAB software to investigate the impact of hygrothermal conditions on the physical distribution of piezothermoelastic material.The values for hygro parameters and material constants for cadmium selenide, as presented in Table 1, are adopted based on the works of Yadav et al.[47].

Table 1 Values of the materials constants
Full size table

During the numerical method outlined previously, we focus on the real components of the stress tensor \(\left( {\sigma_{xx} ,\,\sigma_{zz} ,\,\sigma_{xz} } \right)\), electric displacement \(\left( {D_{x} ,\,D_{z} } \right)\), displacement vector \(\left( {u,\,w} \right)\), temperature \(T\), and electric potential \(\phi\). We calculated and plotted the physical distribution of these components by varying moisture concentrations, moisture diffusivity, and temperature diffusivity for fixed \(\beta_{1}^{m} = 0.8 \times 10^{ - 4}\), \(\beta_{3}^{m} = 1.1 \times 10^{ - 4}\). The sub-figures of the resulting graph present overlapping curves to clearly illustrate any subtle differences, thereby aiding in comprehending intricate details at a micro-scale.

The first category, Fig. 2a–i depicted the effect of various levels of moisture concentration \(\left( {m_{0} = 10\% ,\,\,20\% ,\,\,30\% ,\,\,40\% } \right)\) on the hygro-piezo-thermal-elastic-electric wave propagation with the distance \(z\). All calculations have been evaluated with time \(t = 1.5\) and assumed constants \(P_{1}^{*} = 0.01\) and \(P_{1}^{*} = 0.02\). The sky blue, orange, yellow, and purple linesrelated to the moisture concentration \(m_{0} = 10\% ,\,\,20\,\% ,\,\,30\% ,\,\,40\%\), respectively.

Fig. 2
figure 2figure 2

a–i Distribution of the physical quantities with respect to \(z\) for various values of moisture concentration \(m_{0} = 10\% ,\,\,\) \(20\% ,\,\,\) \(30\% ,\,\,\) \(40\% ,\) at \({\text{D}}_{{1}}^{{\text{m}}} = 0.8 \times 10^{ - 4}\), \({\text{D}}_{{3}}^{{\text{m}}} = 1.1 \times 10^{ - 4}\), \({\text{D}}_{{1}}^{{\text{T}}} = 50 \times 10^{ - 4}\), \({\text{D}}_{{1}}^{{\text{T}}} = 75 \times 10^{ - 4}\)

Full size image

The distribution of displacement expresses the elastic wave. Within the given range \(0 \le z \le 0.5\), the horizontal displacement component \(u\) exponentially decreases from a negative value to its minimum value. As shown in Fig. 2a, with a further increase of \(z\), the horizontal displacement progressively increases and tends towards zero. The vertical displacement component \(w\) starts with a positive value at the maximum point and decreases exponentially, converging to zero as \(z\) approaches infinity, as illustrated in Fig. 2b. The horizontal displacement component’s distribution is directly proportional to the concentration of the moisture, whereas the vertical displacement component’s distribution is inversely proportional to it.

The potential distribution field \(\phi\) represents the electric wave, starting from its minimum negative point and sharply increasing as \(z\) increases, ultimately vanishing as \(z\) approaches infinity, as illustrated in Fig. 2c. The thermal temperature \(T\) represents the thermal wave, decreasing exponentially within the range \(0 \le z \le 0.05\). Subsequently, with a further increment of \(z\) the \(u\) gradually increases and approaches zero, as shown in Fig. 2d. The amount of electric potential and temperature distributions is inversely proportional to the moisture concentration, with higher moisture concentrations resulting in lower amounts of both distributions.

The mechanical wave generated by the stress components is shown in Fig. 2e–g. Figure 2e illustrates the distribution profile of the stress component \(\sigma_{xx}\), which starts from a maximum value and decreases exponentially, approaching zero as \(z\) tends to infinity. Figure 2f reveals that the stress component \(\sigma_{zz}\) distribution starts from zero, increases exponentially within the range \(0 \le z \le 0.3\), and then tends to zero as \(z\) further increases. Figure 2g demonstrates that the distribution profile of the stress component \(\sigma_{xz}\) follows a pattern almost identical to that of the stress component \(\sigma_{zz}\). The \(\sigma_{xz}\) distribution curve starts from zero, satisfying the boundary condition (26), i.e., \(\sigma_{xz} \left( {x,0,t} \right) = 0\). The noted observation enhances assurance in the accuracy and reliability of our numerical coding, indicating the accurate implementation of the boundary condition (36). Figure 2e–g distinctly illustrate the considerable influence of moisture content on the mechanical wave.

The electrical displacement \(D_{x}\) and \(D_{z}\) are shown in Fig. 2h, i. The amplitude of \(D_{x}\) start from a positive value at the maximum point and reaches minima its negative value with a slight increase of \(z\). Further increase of \(z\) the amplitude increases and reaches to zero. The amplitude of the \(D_{z}\) start from positive value at the maximum point decreases exponentially and tends to zero with \(z\). Figure 2h, i suggest that the electrical displacement increases with the increase of moisture concentration.

The second category, Fig. 3a–i shows the effect of moisture diffusivity parameters \(\left( {{\text{D}}_{{1}}^{{\text{m}}} = 0.8 \times 10^{ - 4} ,\,} \right.\)\(\left. {D_{3}^{m} = 1.1 \times 10^{ - 4} } \right)\), \(\left( {{\text{D}}_{{1}}^{{\text{m}}} = 0.8 \times 10^{ - 3} ,\,} \right.\)\(\left. {D_{3}^{m} = 1.1 \times 10^{ - 3} } \right)\), \(\left( {{\text{D}}_{{1}}^{{\text{m}}} = 0.8 \times 10^{ - 2} ,\,} \right.\)\(\left. {D_{3}^{m} = 1.1 \times 10^{ - 2} } \right)\), \(\left( {{\text{D}}_{{1}}^{{\text{m}}} = 0.8 \times 10^{ - 1} ,\,} \right.\)\(\left. {D_{3}^{m} = 1.1 \times 10^{ - 1} } \right)\) on the profiles of the hygro-piezo-thermo-elastic model with a fixed time \(t = 1.5\) and assumed constant \(P_{1}^{*} = 0.01\) and \(P_{1}^{*} = 0.02\). From these figures, note that all physical field distributions exhibit similar behavior, as described in Fig. 2a–i.

Fig. 3
figure 3figure 3

a–i Distribution of the physical quantities with respect to \(z\) for various values of moisture diffusivity \(D_{1}^{m} = 0.8 \times 10^{ - 4}\), \(D_{3}^{T} = 1.1 \times 10^{ - 4}\), \(D_{1}^{m} = 0.8 \times 10^{ - 3}\), \(D_{3}^{m} = 1.1 \times 10^{ - 3}\),\(D_{1}^{m} = 0.8 \times 10^{ - 2}\), \(D_{3}^{m} = 1.1 \times 10^{ - 2}\),\(D_{1}^{m} = 0.8 \times 10^{ - 1}\), \(D_{3}^{m} = 1.1 \times 10^{ - 1}\), at \(m_{0} = 10\,\% ,\)\(D_{1}^{T} = 50 \times 10^{ - 4}\), \(D_{3}^{T} = 75 \times 10^{ - 4}\)

Full size image

The significant impact of moisture diffusivity observes a fascinating complexity that defies a simplistic direct or inverse proportionality with moisture diffusivity. Rather than adhering to predictable patterns, the relationship between moisture diffusivity and the distribution profiles unfolds in a nuanced manner, showcasing the richness of interactions at play. It becomes apparent that the impact of moisture diffusivity is not uniform, leading to intricate variations in the distribution profiles. This departure from conventional expectations adds a layer of sophistication to the understanding of the system. To enhance clarity, we employ descriptive language to highlight specific instances where the influence of moisture diffusivity manifests in unexpected ways. By navigating through these specific scenarios or depth ranges, the graphical discussion unveils a tapestry of complexities, offering a more profound and convincing interpretation of the observed phenomena.

In essence, this approach acknowledges the intricate and non-linear nature of the relationship between moisture diffusivity and physical distribution profiles and persuasively underscores the need to embrace the complexity inherent in the system under investigation. The physical properties of the hygro-piezo-thermo-elastic model always tend to the equilibrium state as \(z\) approaches infinity.

The third category, Fig. 4a–i shows the effect of temperature diffusivity parameters \(\left( {{\text{D}}_{{1}}^{{\text{T}}} = 50 \times 10^{ - 4} ,\,} \right.\)\(\left. {D_{3}^{T} = 75 \times 10^{ - 4} } \right)\), \(\left( {{\text{D}}_{{1}}^{{\text{T}}} = 50 \times 10^{ - 3} ,\,} \right.\)\(\left. {D_{3}^{T} = 75 \times 10^{ - 3} } \right)\), \(\left( {{\text{D}}_{{1}}^{{\text{T}}} = 50 \times 10^{ - 2} ,\,} \right.\)\(\left. {D_{3}^{T} = 75 \times 10^{ - 2} } \right)\), \(\left( {{\text{D}}_{{1}}^{{\text{T}}} = 50 \times 10^{ - 1} ,\,} \right.\)\(\left. {D_{3}^{T} = 75 \times 10^{ - 1} } \right)\) on the various distribution profiles of the hygro-piezo-thermo-elastic model with a fixed time \(t = 1.5\) and assumed constant \(P_{1}^{*} = 0.01\) and \(P_{1}^{*} = 0.02\). Upon examination of these figures, it becomes apparent that all of the field distributions exhibit a similar trend, as depicted in Fig. 2a–i. Notably, the impact of temperature diffusivity is prominently manifested in the aforementioned figures. It should also be pointed out that the physical properties of the hygro-piezo-thermo-elastic model invariably tend towards an equilibrium state as \(z\) approaches infinity.

Fig. 4
figure 4figure 4

a–i Distribution of the physical quantities with respect to \(z\) for various values of temperature diffusivity \(D_{1}^{T} = 50 \times 10^{ - 4}\), \(D_{3}^{T} = 75 \times 10^{ - 4}\), \(D_{1}^{T} = 50 \times 10^{ - 3}\), \(D_{3}^{T} = 75 \times 10^{ - 3}\),\(D_{1}^{T} = 50 \times 10^{ - 2}\), \(D_{3}^{T} = 75 \times 10^{ - 2}\),\(D_{1}^{T} = 50 \times 10^{ - 1}\), \(D_{3}^{T} = 75 \times 10^{ - 1}\), at \(m_{0} = 10\,\% ,\)\(D_{1}^{m} = 0.8 \times 10^{ - 4}\), \(D_{3}^{T} = 1.1 \times 10^{ - 4}\)

Full size image

The initial three categories illustrate the physical field distribution at a fixed value of \(x = 0.1\) across various profiles. Conversely, the fourth category, presented in Fig. 5a–i, showcases 3D plots of the solutions in the \(xz\) plane at a fixed time \(t = 1.5\), allowing an exploration of the influence of \(x\) on the distribution patterns. All figures were generated within the range of \(- 10 \le x \le 10\) and \(0 \le z \le 30\). The observation from Fig. 5a–i are as follows:

  • Across the entire range of \(x\), all function profiles display a nearly uniform distribution characterized by alternating peaks.

  • The green color region signifies displacement values approaching zero, affirming the theoretical assumption of boundedness in the solution as \(z \to \infty\).

  • By inspecting the contour plot on the \(xz\) plane, we can deduce that any significant alteration in \(x\) corresponds to an opposite change in the distribution’s direction.

  • All field variables adhere to the boundary constraints.

Fig. 5
figure 5figure 5

a–i Distribution of the physical quantities in \(\left( {x,z} \right)\) plane when, \(m_{0} = 10\,\% ,\)\(D_{1}^{T} = 50 \times 10^{ - 4}\), \(D_{3}^{T} = 75 \times 10^{ - 4}\), \(D_{1}^{m} = 0.8 \times 10^{ - 4}\), \(D_{3}^{m} = 1.1 \times 10^{ - 4}\)

Full size image

8 Conclusions

This research comprehensively analyzes the behavior of plane waves within a piezo-elastic medium subjected to hygro-thermal environments. Applying the rigorous mathematical approach of normal mode analysis, we reveal the nuanced dynamics of the thermo-piezo-elastic medium. Our examination sheds light on the interplay between moisture content, moisture diffusivity, and temperature diffusivity, which collectively shape the comprehensive physical field distribution of the CdSe material. Based on our detailed analysis, several notable outcomes emerge:

  • The field variables’ magnitudes decrease in the \(z\) direction, validating the assumption (24) and supporting previous research conducted by Mondal and Othman [48]. This phenomenon ensures the finite propagation speed of the thermal wave.

  • All physical quantities in the system have both continuity and boundary condition agreements.

  • The moisture content and moisture and temperature diffusivities significantly impact all the physical field distribution of the hygro-piezo-thermo-elastic model.

  • The distribution of physical fields in the hygro-piezo-thermo-elastic model is notably influenced by moisture and temperature diffusivities. Importantly, these impacts do not exhibit a straightforward direct proportionality, adding a layer of complexity to the interplay between these factors

  • The outcomes of this study contribute to enhancing the performance and durability of electronic devices, sensors, energy harvesting systems, materials for structural health monitoring, and infrastructure, particularly in the face of fluctuating moisture and temperature conditions.