Skip to main content
SpringerLink
Log in

Three-Dimensional Free Vibration Analysis and Critical Speed of Pressurized Rotating Functionally Graded Cylindrical Shells

  • Research Paper
  • Published:
Iranian Journal of Science and Technology, Transactions of Mechanical Engineering Aims and scope Submit manuscript

Abstract

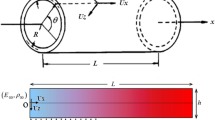
This paper presents an approximate solution for the free vibration analysis of rotating functionally graded cylindrical shells based on the three-dimensional theory, using layerwise theory. Equations of motion are derived by applying Hamilton’s principle. In order to accurately account for the thickness effects, the layerwise theory is used to discretize the equations of motion and the related boundary conditions through the thickness of the shells. The edges of the shell are restrained by simply supported boundary conditions. Material properties are assumed graded in the thickness direction according to a simple power-law distribution in terms of the volume fraction of the constituents. The results obtained include the relationship between frequency characteristics, different material properties, power-law index, rotating velocities and amplitude of internal pressure. In order to validate the present analysis, the comparison is made with the other published works for a cylindrical shell obtained from CLT to TSDT. In addition, another comparison is made with non-rotating isotropic cylindrical shell based on 3D theory. These comparisons confirm reliability of the present work as a measure to approximate solutions to the problem of rotating functionally graded cylindrical shells.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  • Bahadori R, Najafizadeh MM (2015) Free vibration analysis of two-dimensional functionally graded axisymmetric cylindrical shell on Winkler–Pasternak elastic foundation by First-order Shear Deformation Theory and using Navier-differential quadrature solution methods. Appl Math Model 39:4877–4894

    Article  MathSciNet  Google Scholar 

  • Dneshjou K, Talebitooti M, Talebitooti R (2013) Free vibration and critical speed of moderately thick rotating laminated composite conical shell using GDQ method. Appl Math Mech 34:437–456

    Article  MATH  Google Scholar 

  • Dong SB (1997) A block-stodola eigensolution technique for large algebraic systems with non-symmetrical matrices. Int J Numer Meth Eng 11:247–267

    Article  MathSciNet  MATH  Google Scholar 

  • Ebrahimi MJ, Najafizadeh MM (2013) Free vibration of two-dimensional functionally graded circular cylindrical shells on elastic foundation. Modares Mech Eng 38(1):308–324

    MATH  Google Scholar 

  • Ebrahimi F, Rastgo A (2008) An analytical study on the free vibration of smart circular thin FGM plate based on classical plate theory. Thin Walled Struct 46:1402–1408

    Article  Google Scholar 

  • Hua L, Lam KY (1998) Frequency characteristics of a thin rotating cylindrical shell using the generalized differential quadrature method. Int J Mech Sci 40:443–459

    Article  MATH  Google Scholar 

  • Lam KY, Hua L (1999) Influence of boundary conditions on the frequency characteristics of a rotating truncated circular conical shell. J Sound Vib 223(2):171–195

    Article  MATH  Google Scholar 

  • Lam KY, Loy CT (1994) On vibrations of thin rotating laminated composite cylindrical shells. Compos Eng 4:1153–1167

    Article  Google Scholar 

  • Lam KY, Loy CT (1995a) Free vibrations of a rotating multi-layered cylindrical shell. Int J Solids Struct 32:647–663

    Article  MATH  Google Scholar 

  • Lam KY, Loy CT (1995b) Analysis of rotating laminated cylindrical shells by different thin shell theories. J Sound Vib 186:23–35

    Article  MATH  Google Scholar 

  • Leissa AW (1973) Vibration of shells, SP-288, NASA

  • Loy CT, Lam KY (1999) Vibration of thick cylindrical shells on the basis of three-dimensional theory of elasticity. J Sound Vib 226(4):719–737

    Article  MATH  Google Scholar 

  • Loy CT, Lam KY, Reddy JN (1999) Vibration of functionally graded cylindrical shells. Int J Mech Sci 41:309–324

    Article  MATH  Google Scholar 

  • Malekzadeh P, Heydarpour Y (2012) Free vibration analysis of rotating functionally graded cylindrical shells in thermal environment. Compos Struct 94:2971–2981

    Article  Google Scholar 

  • Malekzadeh P, Bahranifard F, Ziaee S (2013) Three-dimensional free vibration analysis of functionally graded cylindrical panels with cut-out using Chebyshev–Ritz method. Compos Struct 105:1–13

    Article  Google Scholar 

  • Najafi A, Ahmadzadeh M (2014) Vibration dissipation of a shell containing fluid. Iran J Sci Technol Trans Mech Eng 38:337–349

    Google Scholar 

  • Najafizadeh MM, Eslami MR (2002) Buckling analysis of circular plates of functionally graded materials under uniform radial compression. Int J Mech Sci 44:2479–2493

    Article  MATH  Google Scholar 

  • Najafizadeh MM, Isvandzibaei MR (2007) Vibration of functionally graded cylindrical shells based on higher order shear deformation plate theory with ring support. Acta Mech 191:75–91

    Article  MATH  Google Scholar 

  • Najafizadeh MM, Isvandzibaei MR (2009) Vibration of functionally graded cylindrical shells based on different shear deformation shell theories with ring support under various boundary conditions. J Mech Sci Technol 23:2072–2084

    Article  Google Scholar 

  • Najafizadeh MM, Hasani A, Khazaeinejad P (2009) Mechanical stability of functionally graded stiffened cylindrical shells. Appl Math Model 33:1151–1157

    Article  MathSciNet  MATH  Google Scholar 

  • Nami MR, Janghrban M (2015) Free vibration of functionally graded size dependent nanoplates based on second order shear deformation theory using nonlocal elasticity theory. Iran J Sci Technol Trans Mech Eng 39:15–28

    Google Scholar 

  • Ng TY, Lam KY (1999) Vibration and critical speed of a rotating cylindrical shell subjected to axial loading. Appl Acoust 56(4):273–282

    Article  Google Scholar 

  • Pradhan SC, Loy CT, Lam KY, Reddy JN (2000) Vibration characteristics of functionally graded cylindrical shells under various boundary conditions. Appl Acoust 61:111–129

    Article  Google Scholar 

  • Reddy JN (2004) Mechanics of laminated composite plates and shells: theory and analysis, 2nd edn. CRC Press, Boca Raton

    Book  MATH  Google Scholar 

  • Shakeri M, Yas MH (2002) Three-dimensional axisymmetric vibrations of orthotropic and cross-ply laminated hemispherical shells. Iran J Sci Technol Trans Mech Eng 26:431–440

    MATH  Google Scholar 

  • Soedel W (1996) Vibrations of shells and plates. Revised and expanded, 2nd edn. Marcel Dekker, New York

    Google Scholar 

  • Sofiyev AH (2009) The vibration and stability behavior of freely supported FGM conical shells subjected to external pressure. Compos Struct 89:356–366

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Qom University of Technology, Qom, 1519-37195, Iran

    Mostafa Talebitooti

Authors
  1. Mostafa Talebitooti
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mostafa Talebitooti.

Appendix

Appendix

$$K^{1}_{11} = \left[ {C_{11} \left( {\frac{m\pi }{L}} \right)^{2} \alpha_{j}^{2} + C_{55} \left( {\frac{{\partial \alpha_{j} }}{\partial z}} \right)^{2} + \frac{{C_{66} n^{2} \alpha_{j}^{2} }}{{R_{j}^{2} }}} \right]\frac{\pi L}{2}$$
(39)
$$K^{1}_{12} = \left( { - \frac{{\left( {C_{66} + C_{12} } \right)}}{{R_{j} }}n\frac{m\pi }{L}\alpha_{j}^{2} } \right)\frac{\pi L}{2}$$
(40)
$$K^{1}_{13} = \left[ { - \left( { - C_{55} + C_{13} } \right)\frac{{\partial \alpha_{j} }}{\partial z}\frac{m\pi }{L}\alpha_{j} - \frac{{C_{12} }}{{R_{j} }}\frac{m\pi }{L}\alpha_{j}^{2} } \right]\frac{\pi L}{2}$$
(41)
$$K^{1}_{14} = \left[ {C_{11} \left( {\frac{m\pi }{L}} \right)^{2} \beta_{j} \alpha_{j} + C_{55} \left( {\frac{\partial \beta }{\partial z}} \right)\left( {\frac{\partial \alpha }{\partial z}} \right) + \frac{{C_{66} n^{2} \beta_{j} \alpha_{j} }}{{R_{j}^{2} }}} \right]\frac{\pi L}{2}$$
(42)
$$K^{1}_{15} = \left[ { - \left( {C_{66} + C_{12} } \right)\frac{n}{{R_{j} }}\frac{m\pi }{L}\beta_{j} \alpha_{j} } \right]\frac{\pi L}{2}$$
(43)
$$K^{1}_{16} = \left[ { - \left( {C_{55} + C_{13} } \right)\frac{m\pi }{L}\frac{{\partial \beta_{j} }}{\partial z}\alpha_{j} - \frac{{C_{12} }}{{R_{j} }}\frac{m\pi }{L}\beta_{j} \alpha_{j} } \right]\frac{\pi L}{2}$$
(44)
$$K^{1}_{22} = \left[ {\left( {C_{22} n^{2} + C_{44} } \right)\frac{{\alpha_{j}^{2} }}{{R_{j}^{2} }} + C_{44} \left( {\frac{{\partial \alpha_{j} }}{\partial z}} \right)^{2} + C_{66} \left( {\frac{m\pi }{L}} \right)^{2} \alpha_{j}^{2} - \frac{{C_{44} }}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial z}\alpha_{j} - \frac{{C_{44} }}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial Z}\alpha_{j} } \right]\frac{\pi L}{2}$$
(45)
$$K^{1}_{23} = \left[ {\left( {C_{22} + C_{44} } \right)\frac{n}{{R_{j}^{2} }}\alpha_{j}^{2} + \left( { - C_{44} + C_{23} } \right)\frac{n}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial z}\alpha_{j} } \right]\frac{\pi L}{2}$$
(46)
$$K^{1}_{24} = \left[ { - \left( {C_{66} + C_{12} } \right)\frac{n}{{R_{j} }}\frac{m\pi }{L}\beta_{j} \alpha_{j} } \right]\frac{\pi L}{2}$$
(47)
$$K^{1}_{25} = \left[ {\left( {C_{22} n^{2} + C_{44} } \right)\frac{{\beta_{j} \alpha_{j} }}{{R_{j}^{2} }} + C_{44} \left( {\frac{{\partial \beta_{j} }}{\partial Z}} \right)\left( {\frac{{\partial \alpha_{j} }}{\partial Z}} \right) + C_{66} \left( {\frac{m\pi }{L}} \right)^{2} \beta_{j} \alpha_{j} - \frac{{C_{44} }}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial Z}\beta_{j} - \frac{{C_{44} }}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial Z}\alpha_{j} } \right]\frac{\pi L}{2}$$
(48)
$$K^{1}_{26} = \left[ {\left( {C_{22} + C_{44} } \right)\frac{n}{{R_{j}^{2} }}\beta_{j} \alpha_{j} + \left( {C_{44} + C_{23} } \right)\frac{n}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial Z}\alpha_{j} } \right]\frac{\pi L}{2}$$
(49)
$$K^{1}_{33} = \left[ {\left( {C_{22} + C_{44} n^{2} } \right)\frac{{\alpha_{j}^{2} }}{{R_{j}^{2} }} + C_{33} \frac{{\partial \alpha_{j} }}{\partial z}\frac{{\partial \alpha_{j} }}{\partial z} + C_{55} \left( {\frac{m\pi }{L}} \right)^{2} \alpha_{j}^{2} + \frac{{C_{23} }}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial z}\alpha_{j} + \frac{{C_{23} }}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial z}\alpha_{j} } \right]\frac{\pi L}{2}$$
(50)
$$K^{1}_{36} = \left[ {\left( {C_{22} + C_{44} n^{2} } \right)\frac{{\beta_{j} \alpha_{j} }}{{R_{j}^{2} }} + C_{33} \frac{{\partial \beta_{j} }}{\partial z}\frac{{\partial \alpha_{j} }}{\partial z} + C_{55} \left( {\frac{m\pi }{L}} \right)^{2} \beta_{j} \alpha_{j} + \frac{{C_{23} }}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial z}\beta_{j} + \frac{{C_{23} }}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial z}\alpha_{j} } \right]\frac{\pi L}{2}$$
(51)
$$K_{42} = \left( { - \frac{{\left( {C_{66} + C_{12} } \right)}}{{R_{j} }}n\frac{m\pi }{L}\alpha_{j} \beta_{j} } \right)\frac{\pi L}{2}$$
(52)
$$K^{1}_{43} = \left[ { - \left( {C_{55} + C_{13} } \right)\frac{{\partial \alpha_{j} }}{\partial z}\frac{m\pi }{L}\beta_{j} - \frac{{C_{12} }}{{R_{j} }}\frac{m\pi }{L}\alpha_{j} \beta_{j} } \right]\frac{\pi L}{2}$$
(53)
$$K^{1}_{44} = \left[ {C_{11} \left( {\frac{m\pi }{L}} \right)^{2} \beta_{j}^{2} + C_{55} \left( {\frac{{\partial \beta_{j} }}{\partial z}} \right)^{2} + \frac{{C_{66} }}{{R_{j}^{2} }}n^{2} \beta_{j}^{2} } \right]\frac{\pi L}{2}$$
(54)
$$K_{45} = \left[ { - \left( {C_{66} + C_{12} } \right)\frac{n}{{R_{j} }}\frac{m\pi }{L}\beta_{j}^{2} } \right]\frac{\pi L}{2}$$
(55)
$$K^{1}_{46} = \left[ { - \left( { - C_{55} + C_{13} } \right)\frac{{\partial \beta_{j} }}{\partial z}\frac{m\pi }{L}\beta_{j} - \frac{{C_{12} }}{{R_{j} }}\frac{m\pi }{L}\beta_{j}^{2} } \right]\frac{\pi L}{2}$$
(56)
$$K^{1}_{53} = \left[ {\left( {C_{22} + C_{44} } \right)\frac{{n\alpha_{j} \beta_{j} }}{{R_{j}^{2} }} + \left( {C_{44} + C_{23} } \right)\frac{n}{{R_{j} }}\frac{{\partial \alpha_{j} }}{\partial z}\beta_{j} } \right]\frac{\pi L}{2}$$
(57)
$$K^{1}_{55} = \left[ {\left( {C_{22} n^{2} + C_{44} } \right)\frac{{\beta_{j}^{2} }}{{R_{j}^{2} }} + C_{44} \left( {\frac{{\partial \beta_{j} }}{\partial z}} \right)^{2} + C_{66} \left( {\frac{m\pi }{L}} \right)^{2} \beta_{j}^{2} - \frac{{C_{44} }}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial z}\beta_{j} - \frac{{C_{44} }}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial z}\beta_{j} } \right]\frac{\pi L}{2}$$
(58)
$$K^{1}_{56} = \left[ {\left( {C_{22} + C_{44} } \right)\frac{{n\beta_{j}^{2} }}{{R_{j}^{2} }} + \left( { - C_{44} + C_{23} } \right)\frac{n}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial z}\beta_{j} } \right]\frac{\pi L}{2}$$
(59)
$$K^{1}_{66} = \left[ {\left( {C_{22} + C_{44} n^{2} } \right)\frac{{\beta_{j}^{2} }}{{R_{j}^{2} }} + C_{33} \left( {\frac{{\partial \beta_{j} }}{\partial Z}} \right)^{2} + C_{55} \left( {\frac{m\pi }{L}} \right)^{2} \beta_{j}^{2} + \frac{{C_{23} }}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial Z}\beta_{j} + \frac{{C_{23} }}{{R_{j} }}\frac{{\partial \beta_{j} }}{\partial Z}\beta_{j} } \right]\frac{\pi L}{2}$$
(60)
$$K^{1}_{ij} = K^{1}_{ji}$$
(61)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Talebitooti, M. Three-Dimensional Free Vibration Analysis and Critical Speed of Pressurized Rotating Functionally Graded Cylindrical Shells. Iran J Sci Technol Trans Mech Eng 43, 113–126 (2019). https://doi.org/10.1007/s40997-017-0115-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40997-017-0115-z

Keywords

Search

Navigation