Introduction

Some materials such as water ice only exist at cryogenic temperatures. Some may be subjected to impact when in use at high temperatures, such as the alloys used in turbines [1, 2]. Yet others may be subjected to a heat pulse at the same time as a shock in, for example, blast loading of concrete [3] or rock [4]. If such events are going to be accurately modelled, a full constitutive relation for the material of interest is needed and this in turn requires mechanical data to be obtained for that material over a wide range of temperature and strain rate [5,6,7,8,9,10]. Thus it is necessary to be able to perform high rate split Hopkinson bar (SHPB) tests at both high and low temperatures. Heating and cooling techniques for achieving this have been reviewed by Chen & Song [11]. So the emphasis of this article will not be on the experimental methods for accomplishing this but on the effect of temperature gradients on elastic wave propagation in the bars themselves. For a comprehensive overview of the SHPB technique and its applications, the reader is referred to the book by Chen & Song [12].

If the specimen (and hence the Hopkinson bar ends) are at a different temperature to ambient, there are several problems that have to be addressed.

First

The elastic modulus, E, of the Hopkinson bar rods (and hence their mechanical impedance) changes with temperature (Fig. 1). As can be seen from this figure, temperature has a significant effect even for a metallic alloy such as Inconel 718 whose elastic properties are a weak function of temperature (the decrease in modulus of about 40 GPa for a temperature rise of 600 K corresponds to a change in impedance of about 10%). The problem is much worse for Hopkinson bars made from viscoelastic polymeric materials [13,14,15] as their mechanical properties depend on both frequency and temperature [16,17,18,19]. Polymers have an additional problem for high temperature testing as even the most heat-resistant ones cannot be used above a few hundred degrees Celsius due to them melting and decomposing. They can, however, be used in low temperature Hopkinson bar tests [15], unless they become brittle. As far as I know, no-one has so far used polymer bars at elevated temperatures. Instead low impedance elastic metals (such as titanium or magnesium alloys [20, 21]) are usually used for obtaining high rate mechanical data for low strength materials. The titanium alloy Ti6Al4V also has the additional advantage that it has a relatively low thermal conductivity (7.2 Wm−1K−1 as compared to 11.4 Wm−1K−1 for Inconel 718 and 16.5 Wm−1K−1 for stainless steel).

Fig. 1
figure 1

Young’s modulus as a function of temperature for Inconel 718 calculated from data supplied by the manufacturer. From [22]

Full size image

The change in elastic modulus has three effects:

  1. (i)

    the particle velocity, \(V=F/\left(AZ\right)\), at the end of a bar of cross-sectional area, A, for a given force, F, will change (Fig. 2; [23,24,25]);

    Fig. 2
    figure 2

    Plot of the ratio of particle velocity, V0, at the strain gauge to the particle velocity, VT, at the end of a high strength steel bar as a function of temperature of the heated end. From [23]

    Full size image
  2. (ii)

    some of the input pulse will be reflected from the section of the bar that is at a non-ambient temperature (Fig. 3; [25, 26]) due to the difference in impedance (the reflection coefficient, R, at an interface between two materials of different acoustic impedance is given by \(R=\frac{{Z}_{1}-{Z}_{2}}{{Z}_{1}+{Z}_{2}}\) ([27] p. 34), where \({Z}_{1}\) and \({Z}_{2}\) are the acoustic impedances of the bar at ambient and non-ambient temperatures respectively;

    Fig. 3
    figure 3

    Computed effect of reflection from a temperature gradient on the force measured at a strain gauge station ‘upstream’ of the gradient (input pulse was a Heaviside function). Solid line: heated rod. Dotted line: unheated rod. The rod was made from a refractory austenitic steel whose end was heated to 1000 °C [24]. From [25]

    Full size image
  3. (iii)

    elastic waves propagating through the temperature gradient will be distorted differently by dispersion compared to a rod that is all at the same temperature [26, 28,29,30,31,32,33,34].

Second

The strain gauges must be kept at ambient temperature, particularly if they are semiconductor gauges, or their response will be changed [11, 35, 36]. As most designers of Hopkinson bar systems position the strain gauges sufficiently far away from the specimen that they remain at ambient temperature, this issue is not normally a problem. However, if it is necessary to measure strains in the heated (or cooled) sections of the bars, a non-contact temperature insensitive optical technique could be used such as photon Doppler velocimetry [37,38,39], although this may be hard to implement close to the device used to heat or cool the specimen.

Third

If tests are to be carried out above ambient temperature, the heating rates should be as rapid as possible to avoid annealing the specimen and hence changing its internal structure [11, 40,41,42]. However, if the specimen is initially in a fully annealed state the heating rate will not matter. Cooling rates for cryogenic studies are not normally so critical since lowering the temperature has the effect of freezing the structure. Most researchers have, however, used conventional resistance furnaces rather than rapidly-acting induction or radiant heaters for high temperature studies e.g. [11, 23, 43,44,45,46,47].

Figure 3 shows the error that results in the calculation of the force on a specimen at 1000 °C if it is assumed that the temperature gradient has no effect. This systematic error rises from 1.5% for \({T}_{F}\)=125 °C to 12% for \({T}_{F}\)=1000 °C (\({T}_{F}\) is the temperature of the furnace). The calculation was performed using a simple form for the temperature distribution (\(T\left(x\right)={T}_{F}{\text{e}}^{-\mu x}\)) and dividing the rod into 80 equal segments [25].

The Solutions

There have been several approaches to these problems listed above.

The first is either to ignore it [48, 49] or to say that thermal gradients in the bars have only a small effect on the measured stress pulses so that their effects are less than the experimental error (unless the temperature excursion is large; [50,51,52,53]). This is usually the case for metallic bars from liquid helium temperatures (4.2 K) [53] up to around + 300 °C. For temperatures between 300 and 600 °C, Inconel Alloy 718 can be used. This alloy has a Young’s modulus (and hence mechanical impedance) which is a relatively weak function of temperature over the temperature range − 200 to + 600 °C (see Fig. 1 and also [54]).

The second is to seek to heat only the specimen and not the bar. This is essential if it is desired to test above 600 °C.

Several ways of doing this have been devised:

  1. (i)

    heat the specimen (and a short section of the ends of the quickly by induction [41], infrared pulse heating [55,56,57], or by passing a current through the specimen to raise its temperature by resistance heating [58]. Note that none of these rapid heating techniques work for materials with low thermal or electrical conductivity, such as polymers [59]. Also rapid heating can produce non-uniform (and usually unknown) temperature distributions within both the specimen and the bars [57]. If both the specimen and the ends of the bars are brought to temperature, the issue arises as to whether the bar ends will be damaged by the test. This would be the case if the flow stress of the bar material falls below about one third of that of the specimen [60,61,62] or if the loading pulse is sufficiently strong to produce plastic rather than elastic deformation of the bar material at the temperature of interest;

  2. (ii)

    construct a mechanical device to bring the bars into contact with the heated specimen a fraction of a second before it is loaded (Fig. 4) [45], or, alternatively, slide the heated specimen into position between the bars a short time before loading [22, 63]. Note that Lindholm and Yeakley [23] had previously reported that this method cannot be done manually fast enough to avoid cooling the specimen substantially. Even a few milliseconds contact of a hot specimen with cold bars can result in a significant transfer of heat energy (Figs. 5, 6, 7, 8, 9). This technique has been used successfully up to 1000 °C [64]. High temperature tension and torsion experiments cannot be performed this way as the specimen in these cases cannot be slid in as it has to be in mechanical (and hence thermal) contact with the bars at all times [65]. For compression experiments (usually performed using light gas-guns) there can be significant uncertainty in the timings of bar contact [55] and hence in the choreography of the mechanical loading and heating pulses [11, 57].

    Fig. 4
    figure 4

    Schematic diagram of a device developed at the Los Alamos National Laboratory to bring Hopkinson bar rods into contact with heated specimen. From [45]

    Full size image
    Fig. 5
    figure 5

    Calculation of the effect of cold contact time for a specimen of vanadium heated to 600 °C. From [55]

    Full size image
    Fig. 6
    figure 6

    Temperature–time plots for the heating of a specimen to 1000 °C using the technique shown in Fig. 7. From [22]

    Full size image
    Fig. 7
    figure 7

    Lamp heating method for heating specimen. From [22]

    Full size image
    Fig. 8
    figure 8

    Temperature–time plots of heating of the bar after bringing them into contact with a specimen heated to 1000 °C. From [22]

    Full size image
    Fig. 9
    figure 9

    Temperature–time plot of cooling of a specimen heated to 1025 °C after bringing into contact with cold bars. From [22]

    Full size image
  3. (iii)

    load the specimen through an insulator (such as alumina) which at room temperature has an impedance nearly equal to steel [66, 67] (as far as I know, this method so far has only been used for compression experiments);

  4. (iv)

    sandwich the specimen between two metal platens of sufficient thickness that when the bars are brought into contact with the specimen-platen sandwich, the platens rather than the specimen cool on the timescale of the test (Figs. 10, 11) [68];

    Fig. 10
    figure 10

    Metal platen system for shielding the specimen from the cooling effect of the cold bars. From [68]

    Full size image
    Fig. 11
    figure 11

    Temperature–time plot for a steel specimen loaded in the arrangement shown in Fig. 10. The specimen can be seen to remain at the desired test temperature up until loading at which point it rises in temperature due to adiabatic plastic work. From [68]

    Full size image
  5. (v)

    keep the impedance constant along the length of the bar by shaping it to compensate for the temperature gradient [44]. So far this has only been implemented for torsion testing and dynamic fracture testing of ceramics in an SHPB [69]. This method has the disadvantage that a bar of a particular profile can only be used for one particular (and known) temperature gradient;

  6. (vi)

    calculate the effect of the temperature gradient on the wave propagation [14, 23, 43]. One way of doing this is to determine the impedance as a function of position (see Fig. 12) [24, 25, 69] using the two-point measurement technique pioneered by Lundberg and colleagues for waves propagating down rods of changing cross-section [70, 71].

    Fig. 12
    figure 12

    Impedance as a function of position for an austenitic steel rod heated to 950 °C at the right-hand end. From [24]

    Full size image

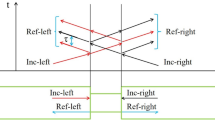
Empirical Check

I have performed an empirical check on whether stress pulses really do propagate from the input to the output bar through both a negative and a positive temperature gradient without significant distortion (see Figs. 13, 14). The bars were 12.7 mm in diameter and 0.5 m long. The strain gauges were positioned half way along the bars. I performed the check by comparing the output bar pulses obtained when the whole apparatus was at room temperature with those obtained where both bar ends were either cold or hot. The only visible effect of the temperature gradient is a slight time shift in the output pulses (a similar observation was made recently by Potter et al. [72]). Note that the ‘spikes’ that can be seen on the traces obtained at high temperatures are due to the induction heater used. No visible distortion of the pulses can be seen except for one experiment where the input pulse shows a ‘rounded knee’ at around 90 µs at high temperature (Fig. 14b). This is probably due to elastic wave energy being reflected from the temperature gradient (cf. Fig. 3). It should be noted that another test under the same conditions with stainless steel bars did not show this phenomenon. We may conclude therefore that stainless steel can be used for Hopkinson bar work at cryogenic temperatures but is slightly inferior to Inconel 718 for high temperature work.

Fig. 13
figure 13

Comparison of propagation of pulses from the input bar into the output bar when a the interface is at − 155 °C and b the interface is at + 550 °C with the same experiment carried out at room temperature when both bars are made from Inconel 718

Full size image
Fig. 14
figure 14

Comparison of propagation of pulses from the input bar into the output bar when a the interface is at − 120 °C and b the interface is at + 580 °C with the same experiment carried out at room temperature when both bars are made from stainless steel

Full size image

One way of calculating the impedance as a function of position and the amount of energy reflected from the temperature gradient is to determine the functional form of the temperature distribution, \(T\left(x\right)\), along the bar. As most researchers use resistance furnaces (which can take up to 30 min to heat the specimen [43]), the bar is assumed to be in a steady state thermally [24, 43]. These authors also checked the temperature distribution using thermocouples (see Figs. 15, 16). Then to turn this into an impedance versus position graph, the relationship between Young’s modulus and temperature must also be known for the bar material (the effect of temperature on the density of the bar is ignored as being a relatively small effect [24, 25]). This relationship has usually been taken to be linear [23, 24]:

$$E\left(T\right)={E}_{0}\left[1-\beta \left(T-{T}_{0}\right)\right],$$
(1)

where \({E}_{0}\) is Young’s modulus at ambient temperature, \(\beta\) is the measure of the dependence of modulus on temperature (ca. 4 \(\times\) 10−4 K−1 for steel [25]), and \({T}_{0}\) is the ambient temperature.

Fig. 15
figure 15

Temperature distribution in a Hopkinson pressure bar measured using thermocouples placed at 5 cm intervals. From [43]

Full size image
Fig. 16
figure 16

Temperature distribution in a Hopkinson pressure bar where the end temperature was 950 °C. Solid circles are measured values, the solid line was calculated. From [24]

Full size image

Chiddister and Malvern [43] found that approximating the smooth curve shown in Fig. 15 by a set of five discrete temperature steps allowed them to calculate the strain in the specimen to within 1% of the value measured by a strain gauge attached directly to the specimen at temperatures up to 480 °C and to within 3% at 650 °C (the reflection of a stress wave from a set of step changes in modulus can be calculated analytically). This procedure was further checked by comparing the predictions with the measured stress pulse reflected back down the input bar.

Bacon et al. [24] found that solving the one-dimensional heat equation gave almost the same answer as solving the two-dimensional heat equation (assuming that the heat flux down the bar is constant down the bar and that the temperature gradient inside the furnace is linear). Both solutions lay close to the temperature values they measured (see Fig. 16). They quote the 1D solution for \(T\left(x\right)\) as follows:

$${T}_{0} , \quad x\le {x}_{B} ,$$
$$T\left(x\right)=\left({T}_{E}-{T}_{0}\right)\frac{\text{sinh}\mu \left(x-{x}_{B}\right)}{\text{sinh}\mu \left({x}_{E}-{x}_{B}\right)}+{T}_{0},\quad {x}_{B}\le x\le {x}_{E },$$
(2)
$$\frac{{T}_{F}\left(x-{x}_{E}\right)+{T}_{E}\left({x}_{F}-x\right)}{{x}_{F}-{x}_{E}} ,\quad x\ge {x}_{E} ,$$

where the temperature and position variables are defined in Fig. 16. \(\mu\) is a parameter that the authors defined as \(\mu =2h/a\lambda\) where h is the heat flux, \(\lambda\) is the thermal conductivity, and a is the radius of the rod. The definition of mechanical impedance \(Z=\sqrt{\rho E}\), where \(\rho\) is the bar density (taken to be constant), and so

$$Z\left(x\right)={Z}_{0}{\left[1-\beta \left(T\left(x\right)-{T}_{0}\right)\right]}^{0.5},$$
(3)

where \({Z}_{0}\) is the impedance at room temperature.

A method that can be used to determine \(Z\left(x\right)\) directly is the two-point measurement technique developed by Lundberg et al. [70, 71, 73] whose original use was for bars having a cross-section that varies with position. The technique involves measuring the strain pulses at two points A and B remote from the furnace (see Fig. 16). Then by dividing up the bar between B and the end into equal segments (40 were used in the calculation performed by Bacon et al. [24]), expressions can be derived for the force and particle velocity at the entrance to each segment [making the assumption that the functional form of \(Z\left(x\right)\) is as given in Eqs. (2) and (3)] until the end of the bar is reached. If the end if the bar is free, the force there must be zero. This boundary condition was then applied in a minimization routine to determine \(Z\left(x\right),\) and this is plotted in Fig. 12.

Bacon et al. [24] also performed an experimental check to see whether their theory correctly predicted the force pulse measured on an extension rod brought into contact with the main rod just before a force pulse was launched down it. The agreement was found to much better than assuming the impedance did not vary with position, though there was a small residual error in calculating the time at which the particle velocity began to rise (the time at which the force pulse began to rise was correctly predicted). They attributed this discrepancy (equivalent to a displacement of 10 µm) to imperfect contact between the two bars.

Conclusions

The method developed by Bacon et al. [24] can be applied to any temperature distribution expressible in polynomial form. The problem with applying their technique to cases where the specimen is heated (or cooled) very rapidly is that the heat flux in the bar is not in a steady state. This means that the functional form of the temperature distribution may not be calculable from the heat diffusion equation: indeed it may vary from shot to shot. Whether a good enough approximation can be arrived at is a matter for future investigators to determine.