Abstract
This paper presents a modified multi-body dynamics model which can evaluate the performance of the steering and suspension systems simultaneously. The modified model investigates the effects of suspension geometry on the steering mechanism. Moreover, it evaluates the influence of the kingpin axis through steering process. This approach consists of three steps; in the first step, the effects of suspension geometry on steering returnability and ride quality are considered utilizing overturning moment and sprung mass acceleration. In other words, this model considers the suspension parameters as inputs of geometry suspension model and evaluates their influences on vehicle behavior. In the second step, the output data of the geometry model is considered as input data for steering system in order to evaluate the effects of suspension parameters on steering mechanism. The accuracy of the proposed model is verified by experimental tests. In this case, Renault Logan is subjected to a constant cornering test (ISO 7401) and double-lane change test (ISO 4138). In the third step, a probabilistic approach is used by developing NSGA II in order to design a robust Pareto multi-objective optimum algorithm. This modified algorithm is used for robust design of a modified suspension-steering mechanism, as an application, considering probabilistic and uncertain variables. The results achieved by the new model are compared with Adams Car simulation in order to evaluate the accuracy of the model.

















Similar content being viewed by others
Abbreviations
- m s :
-
Sprung mass (kg)
- m u 1 :
-
Unsprung mass of front left suspension (kg)
- m u 2 :
-
Unsprung mass of rear left suspension (kg)
- m u 3 :
-
Unsprung mass of front right suspension (kg)
- m u 4 :
-
Unsprung mass of rear right suspension (kg)
- c s 1 :
-
Damping coefficient of front left suspension (N s/m)
- c s 2 :
-
Damping coefficient of rear left suspension (N s/m)
- c s 3 :
-
Damping coefficient of front right suspension (N s/m)
- c s 4 :
-
Damping coefficient of rear right suspension (N s/m)
- k t 1 :
-
Tire stiffness of front left tire (N/m)
- k t 2 :
-
Tire stiffness of rear left tire (N/m)
- k t3 :
-
Tire stiffness of front right tire (N/m)
- k t 4 :
-
Tire stiffness of rear right tire (N/m)
- k s 1 :
-
Spring stiffness of front left suspension (N/m)
- k s 2 :
-
Spring stiffness of rear left suspension (N/m)
- k s 3 :
-
Spring stiffness of front right suspension (N/m)
- k s 4 :
-
Spring stiffness of rear right suspension (N/m)
- Ф:
-
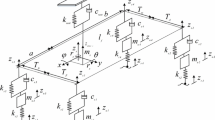
The angle between line ow- and y-axis in Fig. 5
- z r :
-
Random vibration road profile (m)
- c seat :
-
Damping coefficient of driver seat (N s/m)
- k seat :
-
Spring stiffness of driver seat (N/m)
- Ω :
-
Rotation of the control arm (deg)
- Ω 0 :
-
Initial rotation of the control arm (deg)
- z sm :
-
Vertical motion of sprung mass (m)
- z um :
-
Unsprung mass displacement (m)
- λA :
-
Length of suspension member (m) (Fig. 5)
- λB :
-
Length of suspension member (m) (Fig. 5)
- λC :
-
Length of control member (m) (Fig. 5)
- acc.zs :
-
Sprung mass acceleration (m/s2)
- acc.φ :
-
Control arm angular acceleration (deg/s2)
- φ :
-
Roll angle of control arm (deg)
- d :
-
Relative displacement of sprung mass and unsprung mass (m)
- \(\tilde{V}\) :
-
Relative velocity of sprung mass and unsprung mass (m/s)
- z w :
-
Value of point w in z direction (m) (see Fig. 5)
- V :
-
Value of point w in z direction (m) (see Fig. 5)
- z g :
-
Value of point g in z direction (m) (see Fig. 5)
- z f :
-
Value of point f in z direction (m) (see Fig. 5)
- y w :
-
Value of point w in y direction (m) (see Fig. 5)
- y g :
-
Value of point g in y direction (m) (see Fig. 5)
- y f :
-
Value of point f in y direction (m) (see Fig. 5)
- σ :
-
Angle between ow and y-axis (deg) (see Fig. 5)
- F xk :
-
The kth external force in x direction (N)
- F yk :
-
The kth external force in y direction (N)
- F zk :
-
The kth external force in z direction (N)
- q j :
-
The jth general coordinate in Lagrange equation
- V :
-
Potential energy in Lagrange equation
- T :
-
Kinetic energy in Lagrange equation
- D :
-
Energy of damping in Lagrange equation
- Q j(n):
-
The general force corresponding to the general coordinate qj
- t :
-
Time domain in Lagrange equation (s)
- λ(t):
-
White noise function
- ψ :
-
Spectral density of white noise
- σ 2 :
-
Variance of road roughness
- ζ 0 :
-
Constant value in linear regression equation
- ζ 1 :
-
Constant value in linear regression equation
- ν i :
-
The regression coefficients in linear regression equation
- p :
-
Vehicle yaw velocity
- φ :
-
Vehicle roll angle
- \(\dot{\varphi }\) :
-
Vehicle roll rate
- ν x :
-
Vehicle longitudinal velocity
- a 1 :
-
Distance of center of gravity from front tire
- a 2 :
-
Distance of center of gravity from rear tire
- c Tf :
-
Front overall torque coefficient
- c Tr :
-
Rear overall torque coefficient
- c α f :
-
Sum of left and right wheels sideslip coefficients for front wheels
- c α r :
-
Sum of left and right wheels sideslip coefficients for rear wheels
- k f :
-
Front spring stiffness of vehicle
- k r :
-
Rear spring stiffness of vehicle
- c f :
-
Front damping coefficient
- c r :
-
Rear damping coefficient
- F x :
-
Longitudinal force of the vehicle
- F y :
-
Lateral force of the vehicle
- M x :
-
Moment of the vehicle in x-axis
- M z :
-
Moment of the vehicle in z-axis
- β :
-
Caster angle (rad)
- ϑ :
-
Kingpin angle (rad)
- δ :
-
Steer angle (rad)
- δ i :
-
Steer angle of the inner wheel (rad)
- δ o :
-
Steer angle of the outer wheel (rad)
- w :
-
Vehicle track (m)
- l :
-
Wheel base of the vehicle (m)
References
Stoerkle J (2013) Lateral dynamics of multiaxle vehicles. Master Thesis Institute for Dynamic Systems and Control Swiss Federal Institute of Technology (ETH) Zurich
Hidehisa Y, Shuntaro S, Masao N (2009) Lane change steering manoeuvre using model predictive control theory. Veh Syst Dyn 46:669–681
Polack P, Altche F, d'Andréa-Novel B, de La Fortelle A (2017) The kinematic bicycle model: a consistent model for planning feasible trajectories for autonomous vehicles. In: 2017 IEEE intelligent vehicles symposium (IV). Los Angeles, CA, USA, 11–14
Yutan L, Chengjun W, Chuanjin Z (2022) Optimization of the suspension system and analysis of the ride performance of the crawler-type coal mine search and rescue robot. J Braz Soc Mech Sci 367
Bakker E, Pacejka HB (1989) A new tire model with an application in vehicle dynamics studies. SAE Paper, No. 890087
Zong C, Liang H, Tian C, Hu R (2010) Vehicle chassis coordinated control strategy based on model predictive control method. In: 2010 IEEE international conference on information and automation (ICIA). Harbin, China, 20–23
Falcone P, Tseng HE, Borrelli F, Asgari J, Hrovat D (2009) MPC-based yaw and lateral stabilization via active front steering and braking. Veh Syst Dyn 46:611–628
Ni J, Hu J (2017) Dynamics control of autonomous vehicle at driving limits and experiment on an autonomous formula racing car. Mech Syst Signal Process 90:154–174
Kirli A, Arslan MS (2016) Optimization of parameters in the hysteresis-based steering feel model for steer-by-wire systems. IFAC-Papers Online 49:129–134
Pauwelussen JP. Vehicle handling dynamics theory and application, 2nd edn. Chapter Five. Butterworth-Heinemann, pp 123–194
Mantaras DA, Luque P, Vera C (2004) Development and validation of a three-dimensional kinematic model for the Macpherson steering and suspension mechanisms. Mech Mach Theory 39:603–619
Chen K, Beale DG (2003) Base dynamic parameter estimation of a Macpherson suspension mechanism. Veh Syst Dyn 39:227–244
Hu Y, Chen MZQ, Shu Z (2014) Passive vehicle suspensions employing inerters with multiple performance requirements. J Sound Vib 333:2212–2225
Renukadevi V, Tamilselvi S (2022) Stronger forms of sensitivity in the dynamical system of abelian semigroup actions. J Dyn Control Syst 28:151–162
Bouazara M (1997) Etude et analyse de la suspension active et semi-active des vehicules routters. PhD thesis, Universite Laval, Canada
Yarmohammadisatri S, Shojaeefard MH, Khalkhali A (2017) A family base optimization of a developed nonlinear vehicle. Nonlinear Dyn 90:649–669
Jamali A, Salehpour M, Nariman-zadeh MN (2013) Robust Pareto active suspension design for vehicle vibration model with probabilistic uncertain parameters. Multibody Syst Dyn 30:265–285
Ahn C, Peng H (2013) Robust estimation of road frictional coefficient. IEEE Trans Control Syst Technol 21:1–13
Wang J, Wilson DA (2001) Mixed GL2/H2/GH2 control with pole placement and its application to vehicle suspension systems. Int J Control 74:1353–1369
Crespo LG, Kenny SP (2005) Robust control deign for systems with probabilistic uncertainty. NASA report, TP-2005-213531, March
Wang Q, Stengel RF (2001) Searching for robust minimal-order compensators. J Dyn Syst Meas Control 123:223–236
ISO 4138 (2004) Passenger cars—steady-state circular driving behaviour—open-loop test methods
ISO 7401 (2011) Road vehicles—lateral transient response test methods—open-loop test methods
Catia official website. https://www.3ds.com/products-services/catia/?gclid=Cj0KCQjwruPNBRCKARIsAEYNXIhPe17gaCSYgJSJosOZSjIwirHdE1Wuzc7SlAronQAFEs7eGZCcrUMaAkbkEALw_wcB
Fallah MS, Bhat R, Xie WF (2009) New model and simulation of Macpherson suspension system for ride control applications. Veh Syst Dyn 47:195–220
Vehicle standard website. https://www.techstreet.com/standards/din-70000?product_id=1055555
Jazara RN, Subica A, Zhang N (2012) Kinematics of a smart variable caster mechanism for a vehicle steerable wheel. Veh Syst Dyn 50:1861–1875
Gho YG (2009) Vehicle steering returnability with maximum steering wheel angle at low speeds. Int J Automot Technol 10:431–439
Tayyebi Sh (2021) An adaptive fuzzy sliding mode control under model uncertainties and disturbances: second-order non-linear system. J Braz Soc Mech Sci 533
Su L, Meng Zh, Sun Y, Ge X (2018) A reliability-based design framework for early stages of design process. J Braz Soc Mech Sci 205
Yang K, Chen E, Zhou X (2016) Time-restricted sensitivity and entropy for random dynamical systems. J Dyn Control Syst 37:145–174
Shojaeefard MH, Talebitooti R, YarmohammadiSatri S (2015) Optimum design of 1st gear ratio for 4WD vehicles based on vehicle dynamic behaviour. Adv Mech Eng 1–9
Shojaeefard MH, Talebitooti R, YarmohammadiSatri S, Amiryoon MH (2014) Investigation on natural frequency of an optimized elliptical container using real-coded genetic algorithm. Lat Am J Solid Struct 11:113–129
Funding
The authors did not receive support from any organization for the submitted work.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that there is no competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
Additional information
Technical Editor: Sadegh Yarmohammadisatri.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1
By using the triangle OWF, the following equation can be written as:
where
In Eq. (24), λ and \(\lambda^{\prime}\) represent the initial length of control member wf (illustrated in Fig. 9). Ω indicates the angle between the ow-axis and y-axis which is presented in Fig. 5.
Δλ and zg–zr (Eqs. 27 and 29) are calculated by considering the geometry of vehicle suspension as shown below:
Equations (1), (24)–(29) indicate the transformed of suspension key points, based on original variables of the vehicle suspension. These points are utilized to derive the state equations of the suspension geometry. The state equations of geometry suspension are then used to evaluate the vehicle suspension behavior under different conditions. Lagrangian mechanics are used to achieve the equations of motion of the Macpherson suspension, as indicated in Eq. (30).
where
where T, V and D, respectively, represent the kinetic energy, potential energy and the damping energy. The equations of motion and its associated parameters are as follows:
Appendix 2
By substitution of Eq. (32) into Eq. (3), f1 and f2 are calculated as:
where D1 and D2 are given by Eq. (35).
Λ(x3), H(x3), cλ and dλ are achieved by Eqs. (36)–(39).
Appendix 3
Appendix 4
The sum of the camber and slip moments generates the roll moment (Mx). This moment is dependent on the lateral force, as indicated in Fig. 6.
The rear and front coefficients of tire torque are indicated as follows:
The roll moments, which are generated by difference between the vertical forces exerted on the right and left wheels, are related to the roll angle of the car.
In these equations, cαf and cαr are sum of left and right wheels sideslip coefficients for front and rear wheels. CTf and CTr are front and rear overall torque coefficient. kϕ and cϕ are the roll stiffness and roll damping of the vehicle which are calculated as:
w is the vehicle track; kf, kr and cf, cr are front and rear spring stiffness and damping coefficient, respectively.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Yarmohammadisatri, S., Khalkhali, A. & Claudel, C. Robust design of multi-body model of steering mechanism based on uncertainties of suspension parameters. J Braz. Soc. Mech. Sci. Eng. 45, 482 (2023). https://doi.org/10.1007/s40430-023-04379-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s40430-023-04379-4