Introduction

The use of composite material for the structure/component design has grown significantly over the past few decades because their response characteristics can be tailored to meet specific design requirements. Furthermore, composite structures possess high specific stiffness and high specific strength which leads to overall reduction of weight, by increasing the efficiency of the structure. Laminated composite plates are widely used in industry and new fields of technology. Due to the high degrees of anisotropy and the low rigidity in transverse shear of the plates, the Kirchhoff hypothesis as a classical theory is no longer adequate. This hypothesis states that the normal to the midplane of a plate remains straight and normal after deformation because of the negligible transverse shear effects. Refined theories without this assumption have been used recently. The free vibration frequencies calculated by using the classical theory of thin plates are higher than those obtained by the Mindlin theory of plates (Mindlin 1951), in which the transverse shear and rotary inertia effects are included.

A number of shear deformation theories have been proposed to date. The first such theory for laminated isotropic plates was proposed apparently by Stavski (1965). This theory was generalized to laminated anisotropic plates in Yang et al. (1966), Ambartsumyan (1969) and Ambartsumyan and Gnuni (1961). It was shown in Srinivas and Rao (1970), Whitney and Sun (1973) and Bert et al. (1974) that the Yang–Norris–Stavski (YNS) theory (Yang et al. 1966) is adequate for predicting the flexural vibration response of laminated anisotropic plates in the first few modes. In Whitney and Pagano (1970), the YNS theory was employed to study the cylindrical bending of antisymmetric cross-ply and angle-ply plate-strips under sinusoidal loading and the free vibration of antisymmetric angle-ply plate-strips (see also (Fortier and Rossettos 1973; Shinha and Rath 1975). Using the YNS theory, a closed-form solution for the free vibration of simply supported rectangular plates of antisymmetric angle-ply laminates was obtained in (Bert and Chen 1978). In Noor (1973) were also presented exact three-dimensional elasticity solutions for the free vibration of isotropic and anisotropic composite laminated plates, which serve as benchmark solutions for comparison by many researchers. The free vibration of antisymmetric angle-ply laminated plates, with reference to transverse shear deformations, was investigated in Reddy (1979) using the finite-element method The author also derived a set of variationally consistent equilibrium equations for the kinematic models originally proposed by Levinson and Murthy (Reddy 1984). In Reddy and Khdeir (1989), analytical and finite- element solutions for the vibration and buckling of laminated composite plates were found using various theories of plates to prove the necessity for shear deformation theories to predict the behavior of composite laminates. Using a higher order shear deformation theory, finite-element solutions for free vibration analysis of laminated composite plates were also obtained in (Shankara and Iyengar 1996). The complete set of linear equations of a second-order theory was derived in Khdeir and Reddy (1999) to analyze the free vibration behavior of cross-ply and antisymmetric angle-ply laminated plates. In Singh et al. (2001), the natural frequencies of composite plates with random material properties were determined using a higher order shear deformation theory (including the rotatory inertia effect). The natural frequencies of laminated composite plates were also found in Rastgaar et al. (2006) by employing a third-order shear deformation theory. In Simsek (2010a), the dynamic deflections and the stresses of a functionally graded simply supported beam subjected to a moving mass were investigated using the Euler–Bernoulli, Timoshenko, and the parabolic shear deformation theory of beams. In Simsek (2010b), the free vibration of functionally graded beams with different boundary conditions was examined by using the classical, first-order, and different higher order shear deformation theories of beams. A stress analysis of a functionally graded plate subjected to thermal and mechanical loads was performed in Matsunaga (2009) using a two-dimensional higher order theory. A new trigonometric shear deformation theory for isotropic and composite laminated and sandwich plates was developed recently in Mantari et al. (2012), El and Chulkov (1973), where displacements of the middle surface were expanded in terms of tangential trigonometric functions of the thickness coordinate, and the transverse displacements were assumed to be constant across the thickness.

In this paper, a refined and simple theory of plates is presented and applied to the investigation of static, buckling, and free vibration behavior of laminated composite plates. This theory is based on the assumption that the in-plane and transverse displacements consist of bending and shear components where the bending components do not contribute to shear forces, and likewise, the shear components do not contribute to bending moments. The most interesting feature of this theory is that it allows for parabolic distributions of transverse shear stresses across the plate thickness and satisfies zero shear stress conditions at the top and bottom surfaces of the plate without using shear correction factors. The equations of motion are derived using Hamilton’s principle. The fundamental frequencies are found by solving an Eigen value equation. The results obtained by the present method are compared with solutions and results of the first-order and the other higher-order theories.

Theoretical formulations

Basic assumptions

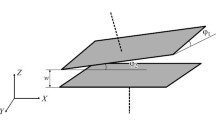
Consider a rectangular plate of total thickness h composed of n orthotropic layers with the coordinate system as shown in Fig. 1. The assumptions of the refined plate’s theory are as follows:

Fig. 1
figure 1

Coordinate system and layer numbering used for a typical laminated plate

Full size image

  • The displacements are small in comparison with the plate thickness and, therefore, strains involved are infinitesimal.

  • The transverse displacement w includes three components of bending w b and shear w s . These components are functions of coordinates x, y, and time t only.

    $$w(x,y,z,t) = w_{b} (x,y,t) + w_{s} (x,y,t)$$
    (1)

  • The transverse normal stress \(\sigma_{z}\) is negligible in comparison with in-plane stresses \(\sigma_{x}\) and \(\sigma_{y}\).

  • The displacements U in x-direction and V in y-direction consist of extension, bending, and shear components:

    $$U = u + u_{b} + u_{s} ,\;V = v + v_{b} + v_{s}$$
    (2)

  • The bending components \(u_{b}\) and \(v_{b}\) are assumed to be similar to the displacements given by the classical plate theory. Therefore, the expression for \(u_{b}\) and \(v_{b}\) can be given as

    $$u_{b} = - z\frac{{\partial w_{b} }}{\partial x},\quad v_{b} = - z\frac{{\partial w_{b} }}{\partial y}$$
    (3)

  • The shear components \(u_{s}\) and \(v_{s}\) give rise, in conjunction with \(w_{s}\), to the parabolic variations of shear strains \(\gamma_{xz}\), \(\gamma_{yz}\) and hence to shear stresses \(\sigma_{xz}\), \(\sigma_{yz}\) through the thickness of the plate in such a way that shear stresses \(\sigma_{xz}\), \(\sigma_{yz}\) are zero at the top and bottom faces of the plate. Consequently, the expression for \(u_{s}\) and \(v_{s}\) can be given as

    $$u_{s} = f(z)\frac{{\partial w_{s} }}{\partial x},\quad v_{s} = f(z)\frac{{\partial w_{s} }}{\partial y}$$
    (4)

Kinematics

Based on the assumptions made in the preceding section, the displacement field can be obtained using Eqs. (1)–(4):

$$\begin{aligned} &u(x,y,z,t) = u_{0} (x,y,t) - z\frac{{\partial w_{b} }}{\partial x} - f(z)\frac{{\partial w_{s} }}{\partial x} \hfill \\ &v(x,y,z,t) = v_{0} (x,y,t) - z\frac{{\partial w_{b} }}{\partial y} - f(z)\frac{{\partial w_{s} }}{\partial y} \hfill \\ &w(x,y,z,t) = w_{b} (x,y,t) + w_{s} (x,y,t), \hfill \\ \end{aligned}$$
(5a)

where \(u_{0}\) and \(v_{0}\) are the mid-plane displacements of the plate in the \(x\) and \(y\) direction, respectively; \(w_{b}\) and \(w_{s}\) are the bending and shear components of transverse displacement, respectively, while \(f(z)\) represents shape functions determining the distribution of the transverse shear strains and stresses along the thickness. This function ensures zero transverse shear stresses at the top and bottom surfaces of the plate. The parabolic distributions of transverse shear stresses through the plate thickness are taken into account for the analysis, by means of the hyperbolic and exponential function of the assumed displacement field.

Present model 1 HSDT The function f(z) is an hyperbolic shape function (Hassaine Daouadji et al. 2012, 2013) (Hyperbolic Shear Deformation Theory):

$$f(z) = z \, \left[ {1 + \frac{3\pi }{2}\sec h^{2} \left( {\frac{1}{2}} \right)} \right] - \frac{3\pi }{2}h\tanh \, \left( {\frac{z}{h}} \right)$$
(5b)

Present model 2 ESDT The function f(z) is an exponential shape function (Karama et al. 2003) (Exponential Shear Deformation Theory):

$$f(z) = z - ze^{{\left( { - 2\frac{{z^{2} }}{{h^{2} }}} \right)}}$$
(5c)

The strains associated with the displacements in Eq. (5a), (5b), (5c) are

$$\left\{ {\begin{array}{*{20}c} {\varepsilon_{x} } \\ {\varepsilon_{y} } \\ {\gamma_{xy} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} {\varepsilon_{x}^{0} } \\ {\varepsilon_{y}^{0} } \\ {\gamma_{xy}^{0} } \\ \end{array} } \right\} + z\left\{ {\begin{array}{*{20}c} {k_{x}^{b} } \\ {k_{y}^{b} } \\ {k_{xy}^{b} } \\ \end{array} } \right\} + f(z)\left\{ {\begin{array}{*{20}c} {k_{x}^{s} } \\ {k_{y}^{s} } \\ {k_{xy}^{s} } \\ \end{array} } \right\},\left\{ {\begin{array}{*{20}c} {\gamma_{yz} } \\ {\gamma_{xz} } \\ \end{array} } \right\} = g(z)\left\{ {\begin{array}{*{20}c} {\gamma_{yz}^{s} } \\ {\gamma_{xz}^{s} } \\ \end{array} } \right\},$$
(6a)

where

$$\left\{ {\begin{array}{*{20}c} {\varepsilon_{x}^{0} } \\ {\varepsilon_{y}^{0} } \\ {\gamma_{xy}^{0} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} {\frac{{\partial u_{0} }}{\partial x}} \\ {\frac{{\partial v_{0} }}{\partial y}} \\ {\frac{{\partial u_{0} }}{\partial y} + \frac{{\partial v_{0} }}{\partial x}} \\ \end{array} } \right\},\left\{ {\begin{array}{*{20}c} {k_{x}^{b} } \\ {k_{y}^{b} } \\ {k_{xy}^{b} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} { - \frac{{\partial^{2} w_{b} }}{{\partial x^{2} }}} \\ { - \frac{{\partial^{2} w_{b} }}{{\partial y^{2} }}} \\ { - 2\frac{{\partial^{2} w_{b} }}{\partial x\partial y}} \\ \end{array} } \right\},\left\{ {\begin{array}{*{20}c} {k_{x}^{s} } \\ {k_{y}^{s} } \\ {k_{xy}^{s} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} { - \frac{{\partial^{2} w_{s} }}{{\partial x^{2} }}} \\ { - \frac{{\partial^{2} w_{s} }}{{\partial y^{2} }}} \\ { - 2\frac{{\partial^{2} w_{s} }}{\partial x\partial y}} \\ \end{array} } \right\},\left\{ {\begin{array}{*{20}c} {\gamma_{yz}^{s} } \\ {\gamma_{xz}^{s} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} {\frac{{\partial w_{s} }}{\partial y}} \\ {\frac{{\partial w_{s} }}{\partial x}} \\ \end{array} } \right\}$$
(6b)

and: \(g(z) = 1 - f^{\prime}(z),f^{\prime}(z) = \frac{{{\text{d}}f(z)}}{{{\text{d}}z}}.\)

Constitutive equations

The stress state in each layer is given by Hooke’s law

$$\left\{ \begin{aligned} \sigma_{x} \hfill \\ \sigma_{y} \hfill \\ \tau_{xy} \hfill \\ \tau_{yz} \hfill \\ \tau_{xz} \hfill \\ \end{aligned} \right\} = \left[ {\begin{array}{*{20}c} {Q_{11} } & {Q_{12} } & 0 & 0 & 0 \\ {Q_{12} } & {Q_{22} } & 0 & 0 & 0 \\ 0 & 0 & {Q_{66} } & 0 & 0 \\ 0 & 0 & 0 & {Q_{44} } & 0 \\ 0 & 0 & 0 & 0 & {Q_{55} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\varepsilon_{x} } \\ {\varepsilon_{y} } \\ {\gamma_{xy} } \\ {\gamma_{yz} } \\ {\gamma_{xz} } \\ \end{array} } \right\},$$
(7a)

where \(Q_{ij}\) are the stiffnesses, which are defined in terms of engineering constants in the material axes of the layer:

$$Q_{11} = \frac{{E_{11} }}{{1 - \nu_{12} \nu_{21} }},Q_{22} = \frac{{E_{22} }}{{1 - \nu_{12} \nu_{21} }},Q_{12} = \frac{{\nu_{12} E_{22} }}{{1 - \nu_{12} \nu_{21} }},Q_{66} = G_{12} ,Q_{44} = G_{23} ,Q_{55} = G_{13} .$$
(7b)

Since the laminate is made of several orthotropic layers with their material axes oriented arbitrarily with respect to laminate coordinates, the constitutive equations of each layer must be transformed to the laminate coordinates x, y, and z. The stress–strain relations in the laminate coordinates of a kth layer are

$$\left\{ \begin{aligned} \sigma_{x} \hfill \\ \sigma_{y} \hfill \\ \tau_{xy} \hfill \\ \tau_{yz} \hfill \\ \tau_{xz} \hfill \\ \end{aligned} \right\}^{(k)} = \left[ {\begin{array}{*{20}c} {\bar{Q}_{11} } & {\bar{Q}_{12} } & {\bar{Q}_{16} } & 0 & 0 \\ {\bar{Q}_{12} } & {\bar{Q}_{22} } & {\bar{Q}_{26} } & 0 & 0 \\ {\bar{Q}_{16} } & {\bar{Q}_{26} } & {\bar{Q}_{66} } & 0 & 0 \\ 0 & 0 & 0 & {\bar{Q}_{44} } & {\bar{Q}_{45} } \\ 0 & 0 & 0 & {\bar{Q}_{45} } & {\bar{Q}_{55} } \\ \end{array} } \right]^{(k)} \left\{ {\begin{array}{*{20}c} {\varepsilon_{x} } \\ {\varepsilon_{y} } \\ {\gamma_{xy} } \\ {\gamma_{yz} } \\ {\gamma_{xz} } \\ \end{array} } \right\}^{(k)} ,$$
(7c)

where \(\bar{Q}_{ij}\) are the transformed material constants, which are given in (Karama et al. 2003) as

$$\begin{aligned} \bar{Q}_{11} = Q_{11} \cos^{4} \theta + 2(Q_{12} + 2Q_{66} )\sin^{2} \theta \cos^{2} \theta + Q_{22} \sin^{4} \theta \hfill \\ \bar{Q}_{12} = (Q_{11} + Q_{22} - 4Q_{66} )\sin^{2} \theta \cos^{2} \theta + Q_{12} (\sin^{4} \theta + \cos^{4} \theta ) \hfill \\ \bar{Q}_{22} = Q_{11} \sin^{4} \theta + 2(Q_{12} + 2Q_{66} )\sin^{2} \theta \cos^{2} \theta + Q_{22} \cos^{4} \theta \hfill \\ \bar{Q}_{16} = (Q_{11} - Q_{12} - 2Q_{66} )\sin \theta \cos^{3} \theta + (Q_{12} - Q_{22} + 2Q_{66} )\sin^{3} \theta \cos \theta \hfill \\ \bar{Q}_{26} = (Q_{11} - Q_{12} - 2Q_{66} )\sin^{3} \theta \cos \theta + (Q_{12} - Q_{22} + 2Q_{66} )\sin \theta \cos^{3} \theta \hfill \\ \bar{Q}_{66} = (Q_{11} + Q_{22} - 2Q_{12} - 2Q_{66} )\sin^{2} \theta \cos^{2} \theta + Q_{66} (\sin^{4} \theta + \cos^{4} \theta ) \hfill \\ \bar{Q}_{44} = Q_{44} \cos^{2} \theta + Q_{55} \sin^{2} \theta \hfill \\ \bar{Q}_{45} = (Q_{55} - Q_{44} )\cos \theta \sin \theta \hfill \\ \bar{Q}_{55} = Q_{55} \cos^{2} \theta + Q_{44} \sin^{2} \theta \hfill \\ \end{aligned}$$
(7d)

In which \(\theta\) is the angle between the global x-axis and the local x-axis of each layer.

Governing equations

Using Hamilton’s energy principle, we derive the equation of motion of the laminated composite plate

$$\delta \int_{{t_{1} }}^{{t_{2} }} {(U - V - T){\text{d}}t = 0} ,$$
(8a)

where U is the strain energy, T is the kinetic energy of the plate, and V is the work of external forces. Employing the principle of minimum total energy leads to the general equation of motion and boundary conditions. Taking the variation of the above equation and integrating by parts, we obtain

$$\begin{aligned} \int\limits_{{t_{2} }}^{{t_{1} }} {\left[ {\int\limits_{V} {(\sigma_{x} \delta \varepsilon_{x} } + \sigma_{y} \delta \varepsilon_{y} + \tau_{xy} \delta \gamma_{xy} + \tau_{yz} \delta \gamma_{yz} + \tau_{xz} \delta \gamma_{xz} )} \right.} \hfill \\ - \rho (\ddot{u}_{0} \delta u_{0} + \ddot{\nu }_{0} \delta v_{0} + (\ddot{w}_{b} + \ddot{w}_{s} )\delta (w_{b} + w_{s} )){\text{d}}V - \int\limits_{A} {q\delta (w_{b} + w_{s} ){\text{d}}A} \hfill \\ \left. { + \int_{A} {\left[ {N_{x}^{0} \frac{{\partial^{2} (w_{b} + w_{s} )}}{{\partial x^{2} }} + N_{y}^{0} \frac{{\partial^{2} (w_{b} + w_{s} )}}{{\partial y^{2} }} + 2N_{xy}^{0} \frac{{\partial^{2} (w_{b} + w_{s} )}}{\partial x\partial y}} \right]{\text{d}}A} } \right]{\text{d}}t = 0, \hfill \\ \end{aligned}$$
(8b)

where \(q\) and \(N_{x}^{0}\), \(N_{y}^{0}\), \(N_{xy}^{0}\) are transverse and in-plane distributed force, respectively.

Where two points above a variable means the second derivative with respect to time. Using the combination of Eqs. (6a), (6b), (8a) and (8b) which takes the form

$$\begin{aligned} &\int\limits_{{t_{2} }}^{{t{}_{1}}} {\left[ {\int\limits_{A} {\left( { - \delta u_{0} N_{x,x} - \delta v_{0} N_{y,y} - \delta u_{0} N_{xy,y} - \delta v_{0} N_{xy,x} - \delta w_{b} M_{x,xx}^{b} - \delta w_{b} M_{y,yy}^{b} } \right.} } \right.} - 2\delta w_{b} M_{xy,xy}^{b} \hfill \\ &\quad \left. { - \delta w_{s} M_{x,xx}^{s} - \delta w_{s} M_{y,yy}^{s} - 2\delta w_{s} M_{xy,xy}^{s} - \delta w_{s} S_{xz,x}^{s} - \delta w_{s} S_{yz,y}^{s} } \right){\text{d}}A - \int\limits_{A} {q\delta (w_{b} + w_{s} ){\text{d}}A} \hfill \\ &\quad + \int_{A} {\left[ {N_{x}^{0} (w_{b,xx} + w_{s,xx} ) + N_{y}^{0} (w_{b,yy} + w_{s,yy} ) + 2N_{xy}^{0} (w_{b,xy} + w_{s,xy} )} \right]{\text{d}}A} \hfill \\& \quad - \int\limits_{A} {\left\{ {\delta u_{0} } \right.} \left( {I_{1} \ddot{u}_{0} - I_{2} \ddot{w}_{b,x} - I_{4} \ddot{w}_{s,x} } \right) + \delta v_{0} \left( {I_{1} \ddot{\nu }_{0} - I_{2} \ddot{w}_{b,y} - I_{4} \ddot{w}_{s,y} } \right) \hfill \\ &\quad + \delta w_{b} \left[ {I_{1} (\ddot{w}_{b} + \ddot{w}_{s} ) + I_{2} (\ddot{u}_{0,x} + \ddot{v}{}_{o,y}) - I_{3} (\ddot{w}_{b,xx} + \ddot{w}_{b,yy} ) - I_{5} (\ddot{w}_{s,xx} + \ddot{w}_{s,yy} )} \right] \hfill \\ &\quad \left. {\left. { +\, \delta w_{s} \left[ {I_{1} (\ddot{w}_{b} + \ddot{w}_{s} ) + I_{4} (\ddot{u}_{0,x} + \ddot{v}{}_{o,y}) - I_{5} (\ddot{w}_{b,xx} + \ddot{w}_{b,yy} ) - I_{6} (\ddot{w}_{s,xx} + \ddot{w}_{s,yy} )} \right]} \right\}{\text{d}}A} \right]{\text{d}}t = 0 \hfill \\ \end{aligned}$$
(9)

The stress resultants N, M, and S are defined as

$$(N_{x} ,N_{y} ,N_{xy} ) = \int_{ - h/2}^{h/2} {(\sigma_{x} ,\sigma_{y} ,\tau_{xy} )\,\,{\text{d}}z} = \sum\limits_{k = 1}^{N} {\int_{{z_{k} }}^{{z_{k + 1} }} {(\sigma_{x} ,\sigma_{y} ,\tau_{xy} )\,{\text{d}}z} }$$
(10a)
$$(M_{x}^{b} ,M_{y}^{b} ,M_{xy}^{b} ) = \int_{ - h/2}^{h/2} {(\sigma_{x} ,\sigma_{y} ,\tau_{xy} )\,z\,{\text{d}}z} = \sum\limits_{k = 1}^{N} {\int_{{z_{k} }}^{{z_{k + 1} }} {(\sigma_{x} ,\sigma_{y} ,\tau_{xy} )\,z\,{\text{d}}z} }$$
(10b)
$$(M_{x}^{s} ,M_{y}^{s} ,M_{xy}^{s} ) = \int_{ - h/2}^{h/2} {(\sigma_{x} ,\sigma_{y} ,\tau_{xy} )\,f(z)\,{\text{d}}z} = \sum\limits_{k = 1}^{N} {\int_{{z_{k} }}^{{z_{k + 1} }} {(\sigma_{x} ,\sigma_{y} ,\tau_{xy} )\,f(z)\,{\text{d}}z} }$$
(10c)
$$(S_{xz}^{s} ,S_{yz}^{s} ) = \int_{ - h/2}^{h/2} {(\tau_{xz} ,\tau_{yz} )\,g(z)\,{\text{d}}z} = \sum\limits_{k = 1}^{N} {\int_{{z_{k} }}^{{z_{k + 1} }} {(\tau_{xz} ,\tau_{yz} )\,g(z)\,{\text{d}}z} }$$
(10d)

Inserting Eqs. (7a), (7b), (7c), (7d) into Eqs. (10a), (10b), (10c), (10d) and integrating across the thickness of the plate, the stress resultants are obtained:

$$\left\{ {\begin{array}{*{20}c} N \\ {M^{b} } \\ {M^{s} } \\ \end{array} } \right\} = \left[ {\begin{array}{*{20}c} A & B & {B^{s} } \\ B & D & {D^{s} } \\ {B^{s} } & {D^{s} } & {H^{s} } \\ \end{array} } \right] \, \left\{ {\begin{array}{*{20}c} \varepsilon \\ {k^{b} } \\ {k^{s} } \\ \end{array} } \right\},\;\left\{ {\begin{array}{*{20}c} {S_{yz}^{s} } \\ {S_{xz}^{s} } \\ \end{array} } \right\} = \left[ {\begin{array}{*{20}c} {A_{44}^{s} } & {A_{45}^{s} } \\ {A_{45}^{s} } & {A_{55}^{s} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\gamma_{yz}^{s} } \\ {\gamma_{xz}^{s} } \\ \end{array} } \right\},$$
(11a)

where

$$N = \left\{ {N_{x} ,N_{y} ,N_{xy} } \right\}^{t} ,\;M^{b} = \left\{ {M_{x}^{b} ,M_{y}^{b} ,M_{xy}^{b} } \right\}^{t} ,M^{s} = \left\{ {M_{x}^{s} ,M_{y}^{s} ,M_{xy}^{s} } \right\}^{t}$$
(11b)
$$\varepsilon = \left\{ {\varepsilon_{x}^{0} ,\varepsilon_{y}^{0} ,\gamma_{xy}^{0} } \right\},k^{b} = \left\{ {k_{x}^{b} ,k_{y}^{b} ,k_{xy}^{b} } \right\},k^{s} = \left\{ {k_{x}^{s} ,k_{y}^{s} ,k_{xy}^{s} } \right\}$$
(11c)
$$A = \left[ {\begin{array}{*{20}c} {A_{11} } & {A_{12} } & {A_{16} } \\ {A_{12} } & {A_{22} } & {A_{26} } \\ {A_{16} } & {A_{26} } & {A_{66} } \\ \end{array} } \right],B = \left[ {\begin{array}{*{20}c} {B_{11} } & {B_{12} } & {B_{16} } \\ {B_{12} } & {B_{22} } & {B_{26} } \\ {B_{16} } & {B_{26} } & {B_{66} } \\ \end{array} } \right],D = \left[ {\begin{array}{*{20}c} {D_{11} } & {D_{12} } & {D_{16} } \\ {D_{12} } & {D_{22} } & {D_{26} } \\ {D_{16} } & {D_{26} } & {D_{66} } \\ \end{array} } \right]$$
(11d)
$$B^{s} = \left[ {\begin{array}{*{20}c} {B_{11}^{s} } & {B_{12}^{s} } & {B_{16}^{s} } \\ {B_{12}^{s} } & {B_{22}^{s} } & {B_{26}^{s} } \\ {B_{16}^{s} } & {B_{26}^{s} } & {B_{66}^{s} } \\ \end{array} } \right],D^{s} = \left[ {\begin{array}{*{20}c} {D_{11}^{s} } & {D_{12}^{s} } & {D_{16}^{s} } \\ {D_{12}^{s} } & {D_{22}^{s} } & {D_{26}^{s} } \\ {D_{16}^{s} } & {D_{26}^{s} } & {D_{66}^{s} } \\ \end{array} } \right],H^{s} = \left[ {\begin{array}{*{20}c} {H_{11}^{s} } & {H_{12}^{s} } & {H_{16}^{s} } \\ {H_{12}^{s} } & {H_{22}^{s} } & {H_{26}^{s} } \\ {H_{16}^{s} } & {H_{26}^{s} } & {H_{66}^{s} } \\ \end{array} } \right]$$
(11e)

And the stiffness components and inertias are given as

$$\left( {A_{ij} ,B_{ij} ,D_{ij} ,B_{ij}^{s} ,D_{ij}^{s} ,H_{ij}^{s} } \right) = \int\limits_{ - h/2}^{h/2} {\bar{Q}_{ij} } (1,z,z^{2} ,f(z),zf(z),f^{2} (z)){\text{d}}z\,\,\,,\,\,\,\,(i,j) = (1,2,6)$$
(12a)
$$A_{ij}^{s} = \int\limits_{ - h/2}^{h/2} {\bar{Q}_{ij} \left[ {g(z)} \right]}^{2} \;{\text{d}}z,\;(i,j) = (4,5)$$
(12b)
$$(I_{1} ,I_{2} ,I_{3} ,I_{4} ,I_{5} ,I_{6} ) = \int\limits_{ - h/2}^{h/2} \rho (1,z,z^{2} ,f(z),zf(z),[f(z)]^{2} ){\text{d}}z.$$
(12c)

Collecting the coefficients of \(\delta u_{0}\), \(\delta v_{0}\), \(\delta w_{b}\), and \(\delta w_{s}\) in Eq. (9), the equations of motion are obtained as

$$\begin{aligned} \delta u_{0} :N_{x,x} + N_{xy,y} &= I_{1} {\ddot{u}}_{0} - I_{2} {\ddot{w}}_{b,x} - I_{4} {\ddot{w}}_{s,x} \hfill \\ \delta v_{0} :N_{xy,x} + N_{y,y}& = I_{1} {\ddot{v}}_{0} - I_{2} {\ddot{w}}_{b,y} - I_{4} {\ddot{w}}_{s,y} \hfill \\ \delta w_{b} :M_{x,xx}^{b} + 2M_{xy,xy}^{b} + M_{y,yy}^{b} + q + N & = I_{1} ({\ddot{w}}_{b} + {\ddot{w}}_{s} ) + I_{2} ({\ddot{u}}_{0,x} + {\ddot{v}}_{0,y} ) \hfill \\ & \quad - I_{3} ({\ddot{w}}_{b,xx} + {\ddot{w}}_{b,yy} ) - I_{5} ({\ddot{w}}_{s,xx} + {\ddot{w}}_{s,yy} ), \hfill \\ \delta w_{s} :M_{x,xx}^{s} + 2M_{xy,xy}^{s} + M_{y,yy}^{s} + S_{xz,x}^{s} + S_{yz,y}^{s} + q + N & = I_{1} ({\ddot{w}}_{b} + {\ddot{w}}_{s} ) + I_{4} ({\ddot{u}}_{0,x} + {\ddot{v}}_{0,y} ) \hfill \\ & \quad - I_{5} ({\ddot{w}}_{b,xx} + {\ddot{w}}_{b,yy} ) - I_{6} ({\ddot{w}}_{s,xx} + {\ddot{w}}_{s,yy} ) \hfill \\ \end{aligned}$$
(13a)

where \(N\) is defined by

$$N = N_{x}^{0} \frac{{\partial^{2} (w_{b} + w_{s} )}}{{\partial x^{2} }} + N_{y}^{0} \frac{{\partial^{2} (w_{b} + w_{s} )}}{{\partial y^{2} }} + 2N_{xy}^{0} \frac{{\partial^{2} (w_{b} + w_{s} )}}{\partial x\partial y} ,$$
(13b)

Clearly, when the effect of transverse shear deformation is neglected (\(w_{s}\) = 0), Eqs. (13a), and (13b) yield the equations of motion of a composite plate based on the classical theory of plates.

Analytical solutions for simply supported rectangular laminates

For antisymmetric cross-ply laminates

The Navier solutions can be developed for rectangular laminates with two sets of simply supported boundary conditions. For antisymmetric cross-ply laminates, the following plate stiffnesses are identically zero:

$$\begin{aligned} A_{16} = A_{26} = D_{16} = D_{26} = D_{16}^{s} = D_{26}^{s} = H_{16}^{s} = H_{26}^{s} = 0 \hfill \\ B_{12} = B_{26} = B_{16} = B_{66} = B_{12}^{s} = B_{16}^{s} = B_{26}^{s} = B_{66}^{s} = A_{45}^{s} = 0 \hfill \\ B_{22} = - B_{11} ,B_{22}^{s} = - B_{11}^{s} \hfill \\ \end{aligned}$$
(14)

The following boundary conditions for antisymmetric cross-ply laminates can be written as

$$\begin{aligned} v(0,y) = w_{b} (0,y) = w_{s} (0,y) = \frac{{\partial w_{b} }}{\partial y}(0,y) = \frac{{\partial w_{s} }}{\partial y}(0,y) = 0 \hfill \\ v(a,y) = w_{b} (a,y) = w_{s} (a,y) = \frac{{\partial w_{b} }}{\partial y}(a,y) = \frac{{\partial w_{s} }}{\partial y}(a,y) = 0 \hfill \\ N_{x} (0,y) = M_{x}^{b} (0,y) = M_{x}^{s} (0,y) = N_{x} (a,y) = M_{x}^{b} (a,y) = M_{x}^{s} (a,y) = 0 \hfill \\ u(x,0) = w_{b} (x,0) = w_{s} (x,0) = \frac{{\partial w_{b} }}{\partial x}(x,0) = \frac{{\partial w_{s} }}{\partial x}(x,0) = 0 \hfill \\ u(x,b) = w_{b} (x,b) = w_{s} (x,b) = \frac{{\partial w_{b} }}{\partial x}(x,b) = \frac{{\partial w_{s} }}{\partial x}(x,b) = 0 \hfill \\ N_{y} (x,0) = M_{y}^{b} (x,0) = M_{y}^{s} (x,0) = N_{y} (x,b) = M_{y}^{b} (x,b) = M_{y}^{s} (x,b) = 0 \hfill \\ \end{aligned}$$
(15)

The boundary conditions in Eq. (15) are satisfied by the following expansions:

$$\begin{aligned} u_{0} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {U_{mn} \,e^{i\omega t} \cos (\lambda \, x)\sin (\mu \, y)} } \hfill \\ v_{0} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {V_{mn} \,e^{i\omega t} \sin (\lambda \, x)\cos (\mu \, y)} } \hfill \\ w_{b} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {W_{bmn} \,e^{i\omega t} \sin (\lambda \, x)\sin (\mu \, y)} } \hfill \\ w_{s} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {W_{smn} \,e^{i\omega t} \sin (\lambda \, x)\sin (\mu \, y)} } \hfill \\ \end{aligned}$$
(16)

where U mn , V mn , W bmn , and W smn unknown parameters must be determined, ω is the Eigen frequency associated with (m, n) the Eigen-mode, and \(\lambda = \frac{m\pi }{a}\) and \(\mu = \frac{n\pi }{b}\).

The transverse load q is also expanded in the double-Fourier sine series as follows:

$$q(x,y) = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {Q_{mn} \sin (\lambda x)\sin (\mu y)} }$$
(17)

The coefficients Q mn are given below for some typical loads:

$$Q_{mn} = \frac{4}{ab}\int_{0}^{a} {\int_{0}^{b} {q(x,y)\sin (\lambda x)} } \sin (\mu y){\text{d}}x{\text{d}}y,\quad {\text{and}}\left\{ \begin{aligned}& Q_{mn} = q_{0} \hfill \\ &Q_{mn} = \frac{{16q_{0} }}{{mn\pi^{2} }} \hfill \\ \end{aligned} \right.$$
(18)

Substituting Eqs. (14), (16), and (17) into Eqs. (13a), (13b), the Navier solution of antisymmetric cross-ply laminates can be determined from equations

$$\left( {\left[ {\begin{array}{*{20}c} {a_{11} } & {a_{12} } & {a_{13} } & {a_{14} } \\ {a_{12} } & {a_{22} } & {a_{23} } & {a_{24} } \\ {a_{13} } & {a_{23} } & {a_{33} + S} & {a_{34} + S} \\ {a_{14} } & {a_{24} } & {a_{34} + S} & {a_{44} + S} \\ \end{array} } \right] - \omega^{2} \left[ {\begin{array}{*{20}c} {m_{11} } & 0 & 0 & 0 \\ 0 & {m_{22} } & 0 & 0 \\ 0 & 0 & {m_{33} } & {m_{34} } \\ 0 & 0 & {m_{34} } & {ms_{44} } \\ \end{array} } \right]} \right)\left\{ {\begin{array}{*{20}c} {U_{mn} } \\ {V_{mn} } \\ {W_{bmn} } \\ {W_{smn} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} 0 \\ 0 \\ q \\ q \\ \end{array} } \right\},$$
(19)

where

$$\begin{aligned} a_{11} = A_{11} \lambda^{2} + A_{66} \mu^{2} ,a_{12} = \lambda \mu \left( {A_{12} + A_{66} } \right),a_{13} = - B_{11} \lambda^{3} ,a_{14} = - B_{11}^{s} \lambda^{3} \hfill \\ a_{22} = A_{66} \lambda^{2} + A_{22} \mu^{2} ,a_{23} = B_{11} \mu^{3} ,a_{24} = B_{11}^{s} \mu^{3} \hfill \\ a_{33} = D_{11} \lambda^{4} + 2(D_{12} + 2D_{66} )\lambda^{2} \mu^{2} + D_{22} \mu^{4} \hfill \\ a_{34} = D_{11}^{s} \lambda^{4} + 2(D_{12}^{s} + 2D_{66}^{s} )\lambda^{2} \, \mu^{ \, 2} + D_{22}^{s} \, \mu^{ 4} \hfill \\ a_{44} = H_{11}^{s} \lambda^{4} + 2(H_{12}^{s} + 2H_{66}^{s} )\lambda^{2} \mu^{2} + H_{22}^{s} \mu^{4} + A_{55}^{s} \lambda^{2} + A_{44}^{s} \mu^{2} \hfill \\ m_{11} = m_{22} = I_{1} ,\;m_{33} = I_{1} + I_{3} (\lambda^{2} + \mu^{2} ) \hfill \\ m_{34} = I_{1} + I_{5} (\lambda^{2} + \mu^{2} ),m_{44} = I_{1} + I_{6} (\lambda^{2} + \mu^{2} ),S = N_{x}^{0} \lambda^{2} + N_{y}^{0} \mu^{2} \hfill \\ \end{aligned}$$
(20)

For antisymmetric angle-ply laminates

For antisymmetric angle-ply laminates, the following plate stiffnesses are identically zero:

$$\begin{aligned} A_{16} = A_{26} = D_{16} = D_{26} = D_{16}^{s} = D_{26}^{s} = H_{16}^{s} = H_{26}^{s} = 0 \hfill \\ B_{11} = B_{12} = B_{22} = B_{66} = B_{11}^{s} = B_{12}^{s} = B_{22}^{s} = B_{66}^{s} = A_{45}^{s} = 0. \hfill \\ \end{aligned}$$
(21)

The following boundary conditions for antisymmetric angle-ply laminates can be written as

$$\begin{aligned} u(0,y) = w_{b} (0,y) = w_{s} (0,y) = \frac{{\partial w_{b} }}{\partial y}(0,y) = \frac{{\partial w_{s} }}{\partial y}(0,y) = 0 \hfill \\ u(a,y) = w_{b} (a,y) = w_{s} (a,y) = \frac{{\partial w_{b} }}{\partial y}(a,y) = \frac{{\partial w_{s} }}{\partial y}(a,y) = 0 \hfill \\ N_{xy} (0,y) = M_{x}^{b} (0,y) = M_{x}^{s} (0,y) = N_{xy} (a,y) = M_{x}^{b} (a,y) = M_{x}^{s} (a,y) = 0 \hfill \\ v(x,0) = w_{b} (x,0) = w_{s} (x,0) = \frac{{\partial w_{b} }}{\partial x}(x,0) = \frac{{\partial w_{s} }}{\partial x}(x,0) = 0 \hfill \\ v(x,b) = w_{b} (x,b) = w_{s} (x,b) = \frac{{\partial w_{b} }}{\partial x}(x,b) = \frac{{\partial w_{s} }}{\partial x}(x,b) = 0 \hfill \\ N_{xy} (x,0) = M_{y}^{b} (x,0) = M_{y}^{s} (x,0) = N_{xy} (x,b) = M_{y}^{b} (x,b) = M_{y}^{s} (x,b) = 0. \hfill \\ \end{aligned}$$
(22)

The boundary conditions in Eq. (22) are satisfied by the following expansions:

$$\begin{aligned} u_{0} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {U_{mn} \,e^{i\omega t} \sin (\lambda \, x)\cos (\mu \, y)} } \hfill \\ v_{0} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {V_{mn} \,e^{i\omega t} \cos (\lambda \, x)\sin (\mu \,y)} } \hfill \\ w_{b} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {W_{bmn} \,e^{i\omega t} \sin (\lambda \, x)\sin (\mu \, y)} } \hfill \\ w_{s} = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {W_{smn} \,e^{i\omega t} \sin (\lambda \, x)\sin (\mu \, y)} } . \hfill \\ \end{aligned}$$
(23)

Substituting Eqs. (21), (17), and (23) into Eqs. (13a), (13b), the equations of the form in Eq. (19) are obtained with the following coefficients:

$$\begin{aligned} a_{11} = A_{11} \lambda^{2} + A_{66} \mu^{2} ;a_{12} = \lambda \mu \, \left( {A_{12} + A_{66} } \right);a_{13} = - (3B_{16} \lambda^{2} \mu + B_{26} \mu^{3} ),a_{22} = A_{66} \lambda^{2} + A_{22} \mu^{2} \hfill \\ a_{14} = - (3B_{16}^{s} \lambda^{2} \mu + B_{26}^{s} \mu^{3} );a_{23} = - (B_{16} \lambda^{3} + 3B_{26} \lambda \mu^{2} );a_{24} = - (B_{16}^{s} \lambda^{3} + 3B_{26}^{s} \lambda \mu^{2} ) \hfill \\ a_{33} = D_{11} \lambda^{4} + 2(D_{12} + 2D_{66} )\lambda^{2} \mu^{2} + D_{22} \mu^{4} ;a_{34} = D_{11}^{s} \lambda^{4} + 2(D_{12}^{s} + 2D_{66}^{s} )\lambda^{2} \, \mu^{ \, 2} + D_{22}^{s} \, \mu^{ 4} \hfill \\ a_{44} = H_{11}^{s} \lambda^{4} + 2(H_{12}^{s} + 2H_{66}^{s} )\lambda^{2} \mu^{2} + H_{22}^{s} \mu^{4} + A_{55}^{s} \lambda^{2} + A_{44}^{s} \mu^{2} ;S = N_{x}^{0} \lambda^{2} + N_{y}^{0} \mu^{2} \hfill \\ m_{11} = m_{22} = I_{1} ,m_{33} = I_{1} + I_{3} (\lambda^{2} + \mu^{2} );m_{34} = I_{1} + I_{5} (\lambda^{2} + \mu^{2} ),m_{44} = I_{1} + I_{6} (\lambda^{2} + \mu^{2} ) \hfill \\ \end{aligned}$$
(24)

Numerical results and discussion

In this study, various numerical examples are described and discussed for verifying the accuracy of the present models in predicting the static bending, critical buckling load, and free vibration behaviors of simply supported antisymmetric cross-ply and angle-ply laminates. To verify, the results achieved by current models are compared with those of Reddy (1984) and exact solution of elasticity in three dimensions (Pagano 1970). The effectiveness of these present′s theories is use of the extension component of transverse displacement. The following lamina properties are used:

Material 1 (Noor 1975): \(E_{1} = 40E_{2} ,\begin{array}{*{20}c} {} & {G_{12} = } \\ \end{array} G_{13} = 0.6E_{2} ,\begin{array}{*{20}c} {} & {G_{23} = 0.5E_{2} } \\ \end{array} ,\begin{array}{*{20}c} {} & {\nu_{12} = 0.25} \\ \end{array}\)

Material 2 (Ren 1990): \(E_{1} = 40E_{2} ,\begin{array}{*{20}c} {} & {G_{12} = } \\ \end{array} G_{13} = 0.5E_{2} ,\begin{array}{*{20}c} {} & {G_{23} = 0.6E_{2} } \\ \end{array} ,\begin{array}{*{20}c} {} & {\nu_{12} = 0.25} \\ \end{array}\)

Material 3 (Pagano 1970): \(E_{1} = 25E_{2} ,\begin{array}{*{20}c} {} & {G_{12} = } \\ \end{array} G_{13} = 0.5E_{2} ,\begin{array}{*{20}c} {} & {G_{23} = 0.2E_{2} } \\ \end{array} ,\begin{array}{*{20}c} {} & {\nu_{12} = 0.25} \\ \end{array}\)

For convenience, the following nondimensionalizations are used in presenting the numerical results in graphical and tabular forms:

$$\begin{aligned} \bar{w} = \frac{{100h^{3} E_{2} }}{{q_{0} a^{4} }}w(a/2,b/2),\bar{\sigma }_{x} = \frac{{h^{2} }}{{q_{0} a^{2} }}\sigma_{x} (a/2,b/2),\bar{\sigma }_{y} = \frac{{h^{2} }}{{q_{0} a^{2} }}\sigma_{y} (a/2,b/2) \hfill \\ \bar{\tau }_{xy} = \frac{{h^{2} }}{{q_{0} a^{2} }}\tau_{xy} (0,0),\bar{\tau }_{xz} = \frac{h}{{q_{0} a}}\tau_{xz} (0,b/2),\bar{\omega } = \omega \frac{{a^{2} }}{h}\sqrt {\frac{\rho }{{E_{2} }}} \;\bar{N} = N_{cr} \left( {\frac{{a^{2} }}{{E_{2} h^{3} }}} \right) \hfill \\ \end{aligned}$$
(25)

Numerical results for bending analysis

The static bending solution obtained by setting the time derivative terms and in-plane forces to zero and simplified as

$$\left[ {\begin{array}{*{20}c} {a_{11} } & {a_{12} } & {a_{13} } & {a_{14} } \\ {a_{12} } & {a_{22} } & {a_{23} } & {a_{24} } \\ {a_{13} } & {a_{23} } & {a_{33} } & {a_{34} } \\ {a_{14} } & {a_{24} } & {a_{34} } & {a_{44} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {U_{mn} } \\ {V_{mn} } \\ {W_{bmn} } \\ {W_{smn} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} 0 \\ 0 \\ {Q_{mn} } \\ {Q_{mn} } \\ \end{array} } \right\}.$$
(26)

A simply supported two-layer antisymmetric angle-ply (45°/−45°) laminate under sinusoidal transverse load is considered. Material set 2 is used. The numerical results of nondimensionalized deflection for the square and rectangular plates are shown in Table 1. In the case of thick plates, a considerable difference exists between the results obtained using the various models and the values reported by Ren’s model (Ren 1990). For a / h ratio equal to 4, the deflections predicted by Reddy’s (Reddy 1984), both these theories are 20–25 % lower for a square flat, and 15 % and 20 % lower for a rectangular flat as compared to the values, therefore, obtained by Ren model’s (Ren 1990). The results computed using all the five models are in good agreement with those reported by Reddy (Reddy 1984) and Ren (Ren 1990) for thin plates (a/h = 100). The nondimensionalized deflections of two-layer (45°/−45°) square laminates under sinusoidal transverse load are presented in Fig. 1 for various ratio of modulus E 1/E 2 (G 12 = G 13 = 0.5E 2, G 23 = 0.6E 2, m 12 = 0.25, a/h = 10).

Table 1 Nondimensionalized deflections of simply supported two-layer (45°/−45°) square and rectangular laminates under sinusoidal transverse load
Full size table

A simply supported two-layer (0°/90°) antisymmetric square laminate under sinusoidal transverse load is considered. The layers have equal thickness. Material set 3 is used. Numerical values of nondimensionalized transverse displacement and in plane stresses are shown in Table 2. Three-dimensional elasticity results are obtained using the method given by (Pagano 1970). The results clearly indicate that the percentage error with respect to three-dimensional elasticity solution in predicting the transverse displacement and in-plane stresses is very much lesser in the case of present models and the prediction of in-plane normal stresses, \(\bar{\sigma }_{x}\), \(\bar{\sigma }_{y}\), is very poor.

Table 2 Nondimensionalized deflections and stresses in two-layer (0°/90°) simply supported square laminated plate under sinusoidal transverse load
Full size table

To further illustrate the accuracy of present theory for wide range of thickness ratio a/h and material anisotropy E 1/E 2, the variations of dimensionless deflection with respect to thickness ratio and material anisotropy are illustrated in Figs. 2 and 3, respectively. The obtained results are compared with those predicted by (Reddy 1984). Again, the present’s models and existing FSDT give almost identical solutions, whereas CPT underestimates deflections of thick laminates with a/h <20 due to ignoring shear deformation effects (Fig. 3). The through thickness variations and corresponding values of the in-plane displacement, normal stresses (\(\bar{\sigma }_{x}\), \(\bar{\sigma }_{y}\)), and shear stresses (\(\bar{\sigma }_{xy}\), \(\bar{\sigma }_{xz}\)) are also given in Figs. 4, 5, 6, and 7, respectively, for a moderately thick laminate with a/h = 10. An excellent agreement between the results predicted by the present theories and results of the first-order and the other higher order theories is found in the literature.

Fig. 2
figure 2

The effect of modulus ratio on nondimensionalized deflection of simply supported two-layer (\(\theta\)/\(- \theta\)) square laminates under sinusoidal transverse load (a/h = 10)

Full size image
Fig. 3
figure 3

The effect of side-to-thickness ratio on nondimensionalized deflection of simply supported two-layer (45°/−45°) square laminates under sinusoidal transverse load

Full size image
Fig. 4
figure 4

Variation of normal stress \(\bar{\sigma }_{x}\) through the thickness of simply supported two-layer (0°/90°) square plate for different values of the aspect ratio

Full size image
Fig. 5
figure 5

Variation of normal stress \(\bar{\sigma }_{y}\) through the thickness of simply supported antisymmetric two-layer (0°/90°) square plate for different values of the aspect ratio

Full size image
Fig. 6
figure 6

Variation of longitudinal tangential stress \(\bar{\tau }_{xy}\) through the thickness of simply supported two-layer (0°/90°) square plate for different values of the aspect ratio

Full size image
Fig. 7
figure 7

Variation of tangential stress \(\bar{\tau }_{xz}\) through the thickness of simply supported two-layer (0°/90°) square plate for different values of the aspect ratio

Full size image

Numerical results for free vibration analysis

In the case of free vibration, the natural frequencies of the laminates can be obtained by setting the determinant of the coefficient of the following matrix to zero:

$$\left( {\left[ {\begin{array}{*{20}c} {a_{11} } & {a_{12} } & {a_{13} } & {a_{14} } \\ {a_{12} } & {a_{22} } & {a_{23} } & {a_{24} } \\ {a_{13} } & {a_{23} } & {a_{33} } & {a_{34} } \\ {a_{14} } & {a_{24} } & {a_{34} } & {a_{44} } \\ \end{array} } \right] - \omega^{2} \left[ {\begin{array}{*{20}c} {m_{11} } & 0 & 0 & 0 \\ 0 & {m_{22} } & 0 & 0 \\ 0 & 0 & {m_{33} } & {m_{34} } \\ 0 & 0 & {m_{34} } & {ms_{44} } \\ \end{array} } \right]} \right)\left\{ {\begin{array}{*{20}c} {U_{mn} } \\ {V_{mn} } \\ {W_{bmn} } \\ {W_{smn} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ 0 \\ \end{array} } \right\}$$
(27)

In Tables 3 and 4, the nondimensional fundamental frequencies of anti-symmetrically laminated cross-ply plates obtained using different shear deformation theories are shown for various values of a/h and modules ratios. It can be seen that, in general, the present model gives more accurate results in predicting the natural frequencies than the PSDT (Reddy 1984) and the three-dimensional elasticity solution given in (Noor 1973). It should be noted that unknown functions in the present model are four, while the unknown functions in the higher order shear deformation theories (Reddy 1984) are five. It can be concluded that the present model is not only accurate, but also simple in predicting the natural frequencies of laminated plates.

Table 3 nondimensional fundamental frequencies of antisymmetric square plates at various values of orthotropy ratio with a/h = 5
Full size table
Table 4 Nondimensional fundamental frequencies of antisymmetric square plates at various values of a/h with E 1/E 2 = 40
Full size table

The variation of natural frequencies with respect to side-to-thickness ratio a/h is presented in Table 5. The natural frequencies obtained using the present model is compared with Reddy’s theory PSDT Reddy (1984), Swaminathan and Patil (2008), and FSDT. In the case of thick plates (a/h ratios 2, 4, 5, and 10) there is a considerable difference between the results computed using the present and the theories of Reddy (1984), Swaminathan and Patil (2008), and (Xiang et al. (2011). The variation of natural frequencies with respect to side-to-thickness ratio a/h for different E 1/E 2 ratio is presented. For a four-layered thick plate with a/h ratio equal to 2 and E 1/E 2 ratio equal to 3 and 10, the percentage difference in values predicted by present theory is 0.15 and 3.50 % lower as compared to Reddy’s theory PSDT Reddy (1984) and Swaminathan and Patil (2008). At higher range of E 1/E 2 ratio equal to 20–40, the percentage difference in values between both the theories is very much higher, and Reddy’s theory very much overpredicts the natural frequency values. For a four-layered thick plate with a/h ratio equal to 2 and E 1/E 2 ratio equal to 20, 30, and 40, the percentage differences in values predicted by present theory are 6, 8, and 9.50 % lower as compared to the theories of Reddy (1984), Swaminathan and Patil (2008), and Xiang et al. (2011). The difference between the models tends to reduce for thin and relatively thin plates. Irrespective of the number of layers, the percentage difference in values between the two theories increases with the increase in the degree of anisotropy. As the number of layer increases, the percentage difference in values between the two theories decreases significantly.

Table 5 Non-dimensionalized fundamental frequencies for a simply supported antisymmetric angle-ply square laminated plate
Full size table

The obtained results of fundamental frequencies are compared with the exact 3D solutions reported by Reddy’s theory (Reddy 1984). Here also the results obtained by the present theories are almost identical with those predicted by existing FSDT. This statement is also firmly demonstrated in Figs. 8 and 9 in which the results obtained by the present theory and FSDT are in excellent agreement for a wide range of thickness ratio a/h. According to Table 6 the present results are in good agreement with the results of Reddy PSDT Reddy (1984), Swaminathan and Patil (2008) and Xiang et al. (2011).

Fig. 8
figure 8

Variation of dimensionless fundamental frequency of antisymmetric angle-ply (45/−45) n square laminates versus thickness ratio (Material 3, E 1/E 2 = 40)

Full size image
Fig. 9
figure 9

Variation of dimensionless fundamental frequency of antisymmetric cross-ply (0/90) n square laminates versus thickness ratio (Material 3, E 1/E 2 = 40)

Full size image
Table 6 The non-dimensional fundamental frequency of the simply supported square plate (\(\theta\)/\(- \theta\)/…) (E 1/E 2 = 40)
Full size table

Numerical results for buckling analysis

For buckling analysis, the applied loads are assumed to be in-plane forces

$$N_{x}^{0} = - N_{0} ,\begin{array}{*{20}c} {} & {N_{y}^{0} = \gamma N_{0} } \\ \end{array} ,\begin{array}{*{20}c} {} & {\gamma = \frac{{N_{x}^{0} }}{{N_{y}^{0} }}} \\ \end{array} ,\begin{array}{*{20}c} {} & {N_{xy}^{0} = 0} \\ \end{array} .$$
(28)

The buckling solution can be obtained from Eq. (19) by setting the time derivative terms and transverse forces to zero:

$$\left[ {\begin{array}{*{20}c} {a_{11} } & {a_{12} } & {a_{13} } & {a_{14} } \\ {a_{12} } & {a_{22} } & {a_{23} } & {a_{24} } \\ {a_{13} } & {a_{23} } & {a_{33} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} & {a_{34} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} \\ {a_{14} } & {a_{24} } & {a_{34} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} & {a_{44} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {U_{mn} } \\ {V_{mn} } \\ {W_{bmn} } \\ {W_{smn} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ 0 \\ \end{array} } \right\}$$
(29)

Following the condensation of variables procedure to eliminate the in-plane displacements U mn and V mn , the following system is obtained:

$$\left[ {\begin{array}{*{20}c} {\bar{a}_{33} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} & {\bar{a}_{34} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} \\ {\bar{a}_{43} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} & {\bar{a}_{44} - N_{0} (\lambda^{2} + \gamma \mu^{2} )} \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {W_{bmn} } \\ {W_{smn} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \right\},$$
(30)

where

$$\begin{aligned} \bar{a}_{33} = a_{33} - a_{13} \frac{{b_{1} }}{{b_{0} }} - a_{23} \frac{{b_{2} }}{{b_{0} }},\bar{a}_{34} = a_{34} - a_{14} \frac{{b_{1} }}{{b_{0} }} - a_{24} \frac{{b_{2} }}{{b_{0} }},\bar{a}_{43} = a_{34} - a_{13} \frac{{b_{3} }}{{b_{0} }} - a_{23} \frac{{b_{4} }}{{b_{0} }} \hfill \\ \bar{a}_{44} = a_{44} - a_{14} \frac{{b_{3} }}{{b_{0} }} - a_{24} \frac{{b_{4} }}{{b_{0} }},b_{0} = a_{11} a_{22} - a_{12}^{2} ,b_{1} = a_{13} a_{22} - a_{12} a_{23} \hfill \\ b_{2} = a_{11} a_{23} - a_{12} a_{13} ,b_{3} = a_{14} a_{22} - a_{12} a_{24} ,b_{4} = a_{11} a_{24} - a_{12} a_{14} \hfill \\ \end{aligned}$$
(31)

For nontrivial solution, the determinant of the coefficient matrix in Eq. (30) must be zero. This gives the following expression for buckling load:

$$N_{0} = \frac{1}{{\lambda^{2} + \gamma \mu^{2} }} \times \frac{{\bar{a}_{33} \bar{a}_{44} - \bar{a}_{34} \bar{a}_{43} }}{{\bar{a}_{33} + \bar{a}_{44} - \bar{a}_{34} - \bar{a}_{43} }}$$
(32)

A simply supported anti-symmetric cross-ply (0/90) n (n = 2, 3, 5) square laminate subjected to uniaxial compressive load is considered. Table 7 shows a comparison between the results obtained using the various models and the three-dimensional elasticity solutions given by Noor (1975). The results clearly indicate that the present model gives more accurate results in predicting the buckling loads when compared to Reddy (1984). Compared to the three-dimensional elasticity solution, the buckling loads predicted by present model, Reddy (1984), are 6–7 %, for four-layer antisymmetric cross-ply (0/90/0/90) square laminates. The effect of side-to-thickness ratio on buckling load of simply supported four-layer (0/90/0/90) square laminates is also presented in Figs. 10 and 11.

Table 7 Nondimensional uniaxial buckling load of simply supported antisymmetric cross-ply (0/90/…) square laminates (a/h = 10)
Full size table
Fig. 10
figure 10

The effect of side-to-thickness ratio on nondimensionalized uniaxial buckling load of simply supported antisymmetric cross-ply (0/90) n square laminates

Full size image
Fig. 11
figure 11

The effect of side-to-thickness ratio on nondimensionalized uniaxial buckling load of simply supported antisymmetric angle-ply square laminates

Full size image

In Table 8, a simply supported two-layer anti-symmetric angle-ply (\(\theta / - \theta\)) square laminate subjected to uniaxial compressive is considered. The numerical values of buckling. The results are compared with the values reported by Ren (1990). For all values of side-to-thickness ratio and fiber orientation, the buckling loads predicted by the present model and Reddy (1984) are almost identical. For a/h ratio equal to 4 and the fiber orientation equal to 30°, the buckling load values predicted by Reddy (1984), and present model are 18–2 % lower as compared to the values obtained by Ren (1990). The results computed using all the five models are in a good agreement with those reported by Ren (1990) for thin plates (a/h = 100). The effect of modulus ratio on nondimensionalized uniaxial buckling load of simply supported two-layer (45/−45) square laminate is presented in Fig. 12 (\(G_{12} = G_{13} = 0.6E_{2} , \, G_{23} = 0.5E_{2} , \, \nu_{12} = 0.25, \, a/h = 10\)).

Table 8 Nondimensional uniaxial buckling load of simply supported two-layer \((\theta/{-}\theta )\) square laminates
Full size table
Fig. 12
figure 12

The effect of modulus ratio on nondimensionalized uniaxial buckling load of simply supported two-layer antisymmetric angle-ply square laminates (a/h = 10)

Full size image

Conclusions

A refined higher order shear deformation theory of plates has been successfully developed for the static, buckling, and free vibration of simply supported laminated plates. The theory allows for a square-law variation in the transverse shear strains across the plate thickness and satisfies the zero-traction boundary conditions on the top and bottom surfaces of the plate without using shear correction factors. The equations of motion were derived from Hamilton’s principle. The accuracy and efficiency of the present models have been demonstrated for static and free vibration behaviors of anti-symmetric cross-ply and angle-ply laminates. The conclusions of this theory are as follows:

  • The deflection load obtained using present models (a simpler version of present theory with four unknowns) and other higher-order theories found in the literature (five unknowns) are almost identical.

  • Compared to the three-dimensional elasticity solution, the present models give more accurate results of static and dynamic load than the height order shear deformation theory.

  • Compared to the three-dimensional elasticity solution, the present theories give more accurate results of deflection and dynamic load than the height order shear deformation theory found in the literature.

  • The natural frequencies obtained by the proposed model with four unknowns are almost identical to those predicted by the shear deformation theories containing five unknowns.

  • The buckling load obtained using present′s model (a simpler version of present theory with four unknowns) and height order shear deformation Reddy’s theory (Reddy 1984) (five unknowns) are comparable.

  • Compared to the three-dimensional elasticity solution, the present model gives more accurate results of buckling load than the height order shear deformation theory.

It can be concluded that the present models proposed prove to be accurate in solving the static, buckling, and dynamic behaviors of anti-symmetric cross-ply and angle-ply laminated composite plates and efficient in predicting the vibration responses of composite plates.